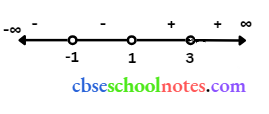Continuity And Differentiability Exercise 5.1
Question 1. Prove that the function f(x) = 5x-3 is continuous at x = 0, at x = -3 and x = 5.
Solution:
The given function is f(x) = 5x-3
At x= 0, f(0) =5×0-3=-3
⇒ \(\lim _{x \rightarrow 0} f(x)=\lim _{x \rightarrow 0}(5 x-3)=5 \times\) 0-3=-3
⇒ \(\lim _{x \rightarrow 0}\) f(x)=f(0)
Therefore, f is continuous at x =0
At x=-3, f(-3)=5 \(\times(-3)\)-3=-18
⇒ \(\lim _{x \rightarrow-3} f(x)=\lim _{x \rightarrow-3}(5 x-3)=5 \times(-3)-3=-18 \Rightarrow \lim _{x \rightarrow-3} f(x)^{-}=f(-3)\)
Therefore, f is continuous at x=-3
At x=5, f(x)=f(5)=5 \(\times\) 5-3=25-3=22
⇒ \(\lim _{x \rightarrow 5} f(x)=\lim _{x \rightarrow 5}(5 x-3)=5 \times 5-3=22 \Rightarrow \lim _{x \rightarrow 5} f(x)=f(5)\)
Therefore, is continuous at x = 5
Question 2. Examine the continuity of the function \(\mathrm{f}(\mathrm{x})=2 \mathrm{x}^2-1\) at x=3.
Solution:
The given function is \(\mathrm{f}(\mathrm{x})=2 \mathrm{x}^2-1\)
At x=3, f(x)=f(3)=2 \(\times 3^2-1=17\)
⇒ \(\lim _{x \rightarrow 3} f(x)=\lim _{x \rightarrow 3}\left(2 x^2-1\right)=2 \times 3^2-1=17 \lim _{x \rightarrow 3} f(x)=f(3)\)
Thus, f is continuous at x =3
Question 3. Examine the following functions for continuity.
f(x)=x-5
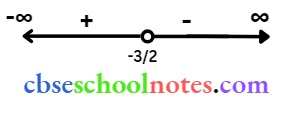
f(x)=\(\frac{1}{x-5}, x \neq 5\)
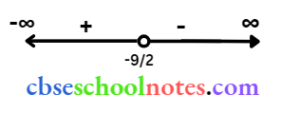
f(x)=\(\frac{x^2-25}{x+5}, x \neq-5\)
f(x)=|x-5|
Solution :
1. The given function is f(x) = x- 5
It is evident that f is defined at every real number k and its value at k is k – 5
It is also observed that, \(\lim _{x \rightarrow k} f(x)=\lim _{x \rightarrow k}(x-5)=k-5=f(k)\)
⇒ \(\lim _{x \rightarrow k} f(x)=f(k)\)
Hence, f is continuous at every real number and therefore, it is a continuous function
Read and Learn More Class 12 Maths Chapter Wise with Solutions
2. The given function is f(x)=\(\frac{1}{x-5}, x \neq 5\)
For any real number k \(\neq 5\), we obtain \(\lim _{x \rightarrow k} f(x)=\lim _{x \rightarrow k}\left(\frac{1}{x-5}\right)=\frac{1}{k-5}\)
Also, f(k)=\(\frac{1}{k-5}( At k \neq 5) \Rightarrow \lim _{x \rightarrow k} f(x)=f(k)\)
Hence,f is continuous at every point in the domain of f and therefore, it is a continuous function.
3. The given function is f(x)=\(\frac{x^2-25}{x+5}\), x \(\neq-5\)
For any real number c \(\neq-5\), we obtain
⇒ \(\lim _{x \rightarrow c} f(x)=\lim _{x \rightarrow c} \frac{x^2-25}{x+5}=\lim _{x \rightarrow c} \frac{(x+5)(x-5)}{x+5}=\lim _{x \rightarrow c}(x-5)=(c-5)\)
Also, f(c)=\(\frac{(c+5)(c-5)}{c+5}=(c-5)(as c \neq-5)\)
⇒ \(\lim _{x \rightarrow c} f(x)=f(c)\)
Hence, f is continuous at every point in the domain of f and therefore, it is a continuous function.
4. The given function is f(x)=|x-5|=\(\begin{cases}{l}5-x, \text { if } x<5 \\ x-5, \text { if } x \geq 5\end{cases}\).
This function f is defined at all points of the real line.
Let c be a point on a real line. Then, c < 5 or c =5 or c > 5
Case 1: c < 5
Then, f (c)=5 – c
⇒ \(\lim _{x \rightarrow c} f(x)=\lim _{x \rightarrow c}(5-x)=5-c \Rightarrow \lim _{x \rightarrow c} f(x)=f(c)\)
Therefore, f is continuous at all real numbers less than 5.
Case 2: c=5
Then, f(c)=f(5)=(5-5)=0
⇒ \(\lim _{x \rightarrow 5} f(x)=\lim _{x \rightarrow 5}(5-x)=(5-5)=0 \Rightarrow \lim _{x \rightarrow 5^{+}} f(x)=\lim _{x \rightarrow 5}\)(x-5)=0
⇒ \(\lim _{x \rightarrow c^{-}} f(x)=\lim _{x \rightarrow c^{+}} f(x)=f(c)\)
Therefore, f is continuous at x = 5
Case 3: c > 5
Then, f(c)=f(5)=c-5
⇒ \(\lim _{x \rightarrow c} f(x)=\lim _{x \rightarrow c}(x-5)=c-5 \Rightarrow \lim _{x \rightarrow c} f(x)=f(c)\)
Therefore, f is continuous at all real numbers greater than 5.
Hence, f is continuous at every real number and therefore, it is a continuous function.
Question 4. Prove that the function f {x} =\(\mathrm{x}^{\mathrm{n}}\) is continuous at x= n, where n is a positive integer.
Solution:
The given function is \(\mathrm{f}(\mathrm{x})=\mathrm{x}^{\mathrm{n}}\)
It is evident that f is defined at all positive integers, n, and its value at n is n.
Then, \(\lim _{x \rightarrow \pi} f(x)=\lim _{x \rightarrow n}\left(x^n\right)=n^n \Rightarrow \lim _{x \rightarrow \pi}\)
f(x)=f(n)
Therefore, f is continuous at n, where n is a positive integer.
Question 5. Is the function f defined by f(x)=\(\begin{cases}{l}x,\text { if } x \leq 1 \\ 5, \text { if } x>1\end{cases}\).continuous at x=0 ? At x=1? At x=2?
Solution:
The given function f(x)=\(\begin{cases}{l}x, \text { if } x \leq 1 \\ 5, \text { if } x>1\end{cases}\).
⇒ \(\mathrm{f}(\mathrm{x})\) may be discontinuous at doubtful point x=1 and except this point \(\mathrm{f}(\mathrm{x})\) be continuous.
Now, at x =1,
The left hand {limit} of f at x=1 is, \(\lim _{x \rightarrow 1^{-}} f(x)=\lim _{x \rightarrow 1^{-}}\) x=1
The right hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{+}} f(x)=\lim _{x \rightarrow 1^{+}}(5)\)=5
⇒ \(\lim _{x \rightarrow 1^{-}} f(x) \neq \lim _{x \rightarrow 1^{+}} f(x)\)
Hence, f is not continuous at x = 1
Question 6. Find all points of discontinuity of f, where f is defined by f(x)=\(\begin{cases}{l}2 x+3, \text { if } x \leq 2 \\ 2 x-3, \text { if } x>2\end{cases}\).
Solution:
The given function f(x)=\(\begin{cases}{l}2 x+3, \text { if } x \leq 2 \\ 2 x-3, \text { if } x>2\end{cases}\). may be discontinuous at doubtful point x=2 and except this point \(\mathrm{f}(\mathrm{x}) \)be continuous.
At x=2
The left hand limit of f at x=2 is, \(\lim _{x \rightarrow 2^{-}} f(x)=\lim _{x \rightarrow 2^{-}}(2 x+3)=2 \times\) 2+3=7
The right hand limit of f at x=2 is \(\lim _{x \rightarrow 2^{+}} f(x)=\lim _{x \rightarrow 2^{+}}(2 x-3)=2 \times\) 2-3=1
⇒ \(\lim _{x \rightarrow 2^{-}} f(x) \neq \lim _{x \rightarrow 2^{+}} f(x)\)
Therefore, f is not continuous at x=2. Hence, x=2 is the only point of discontinuity of f.
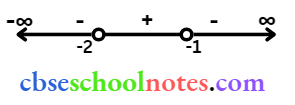
Question 7. Find all points of discontinuity of f, where f is defined by f(x)=\(\begin{cases}{l}|x|+3, \text { if } x \leq-3 \\ -2 x, \text { if }-3<x<3 \\ 6 x+2, \text { if } x \geq 3\end{cases}\).
Solution:
The given function f is f(x)=\(\begin{cases}{l}|x|+3=-x+3, \text { if } x \leq-3 \\ -2 x, \text { if }-3<x<3 \\ 6 x+2, \text { if } x \geq 3\end{cases}\).
Given fn. f(x) may be discontinuous at doubtful points x=-3 and x=3 and except these two points f(x) be continuous.
at x=-3, f(-3)=-(-3)+3=6
L.H.L =\(\lim _{x \rightarrow-3^{-}} f(x)=\lim _{x \rightarrow-3^{-}}(-x+3)=-(-3)+3=6\)
R.H.L =\(\lim _{x \rightarrow-3^{+}} f(x)=\lim _{x \rightarrow-3^{+}}(-2 x)=-2 \times(-3)\)=6
⇒ \(\lim _{x \rightarrow-3} f(x)=f(-3)\)
Therefore, f is continuous at x = -3
Now at x=3, the left hand limit of f at x=3 is, \(\lim _{x \rightarrow 3^{-}} f(x)=\lim _{x \rightarrow 3^{-}}(-2 x)=-2 \times 3\)=-6
The right hand limit of f at x=3 is, \(\lim _{x \rightarrow 3^{+}} f(x)=\lim _{x \rightarrow 3^{+}}(6 x+2)=(6 \times 3)+2=20\)
It is observed that the left and right-hand limits of f at x=3 do not coincide.
Therefore, f is not continuous at x=3. Hence, x=3 is the only point of discontinuity of f.
Question 8. Find all points of discontinuity of f, where f is defined by f(x)= \(\begin{cases}|x| & \text { if } x \neq 0 \\ 0, & \text { if } x=0\end{cases}\)
Solution:
The given function f is f(x)=\(\begin{cases}{l}|x| \text { if } x \neq 0 \\ 0, \text { if }x=0\end{cases}\).
It is known that, x<0 \(\Rightarrow|x|\)=-x and x>0 \(\Rightarrow|x|\)=x
Therefore, the given function can be rewritten as f(x)=\(\left\{\begin{array}{l}
\frac{|x|}{x}=\frac{-x}{x}=-1 \text { if } x<0 \\
0, \text { if } x=0 \\
\frac{|x|}{x}=\frac{x}{x}=1, \text { if } x>0
\end{array}\right.\).
Given f. f(x) may be discontinuous at doubtful point x=0 and except these point f(x) be continuous.
Now at x=0, then the left hand limit of f at x=0 is, \(\lim _{x \rightarrow 0^{-}} f(x)=\lim _{x \rightarrow 0^{-}}(-1)\)=-1
The right hand limit of f at x=0 is, \(\lim _{x \rightarrow 0^{+}} f(x)=\lim _{x \rightarrow 0^{+}}(1)\)=1
It is observed that the left and right-hand limit of f at x=0 do not coincide.
Therefore, f is not continuous at x=0
Hence, x=0 is the only point of discontinuity of f.
Question 9. Find all points of discontinuity of f, where f is defined by f(x)= \(\begin{cases}\frac{x}{|x|} & \text { if } x<0 \\ -1, & \text { if } x \geq 0\end{cases}\)
Solution:
The given function f is f(x)= \(\begin{cases}\frac{x}{|x|}, & \text { if } x<0 \\ -1, & \text { if } x \geq 0\end{cases}\) It is known that, x<0 \(\Rightarrow|x|\)=-x
⇒ \(\mathrm{f}(\mathrm{x})\)=-1 for all x \(\in \mathrm{R}\)
Let c be any real number. Then, \(\lim _{x \rightarrow c} f(x)=\lim _{x \rightarrow c}(-1)\)=-1
Also, f(c)=\(-1=\lim _{x \rightarrow c} f(x)\)
Therefore, the given function is a continuous function.
Hence, the given function has no point of discontinuity.
Question 10. Find all points of discontinuity of f, where f is defined by f(x)=\(\begin{cases}{l}x+1, \text { if } x \geq 1 \\ x^2+1, \text { if } x<1\end{cases}\).
Solution:
The given function f is f(x)=\(\begin{cases}{l}x+1, \text { if } x \geq 1 \\ x^2+1, \text { if } x<1\end{cases}\).
Function \(\mathrm{f}(\mathrm{x})\) may be discontinuous at doubtful point x=1 and except these point f(x) be continuous.
Now at x=1, f(1)=1+1=2
The left hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{-}} f(x)=\lim _{x \rightarrow 1^{-}}\left(x^2+1\right)=1^2+1=2\)
The right hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{+}} f(x)=\lim _{x \rightarrow 1^{+}}(x+1)\)=1+1=2
⇒ \(\lim _{x \rightarrow 1} \mathrm{f}(\mathrm{x})=\mathrm{f}(1)\)
Therefore, f is continuous at x=1. Hence, the given function f has no point of discontinuity.
Question 11. Find all points of discontinuity of f, where f is defined by f(x)=\(\begin{cases}{l}x^3-3, \text { if } x \leq 2 \\ x^2+1, \text { if } x>2\end{cases}\).
Solution:
The given function f is f(x)=\(\left\{\begin{array}{l}x^3-3, \text { if } x \leq 2 \\ x^2+1, \text { if } x>2\end{array}\right.\)
Function \(\mathrm{f}(\mathrm{x})\) may be discontinuous at doubtful point x = 2 and except these point \(\mathrm{f}(\mathrm{x})\) be continuous.
Now at x=2, f(2)=\(2^3-3=5\)
L.H.L =\(\lim _{x \rightarrow 2^{-}} f(x)=\lim _{x \rightarrow 2^{-}}\left(x^3-3\right)=2^3-3=5\)
R.H.L =\(\lim _{x \rightarrow 2^{+}} f(x)=\lim _{x \rightarrow 2^2}\left(x^2+1\right)=2^2+1=5\)
L.H.L = R.H.L =5
⇒ \(\lim _{x \rightarrow 2} f(x)=f(2)\)
Therefore, f is continuous at x = 2. Thus, the given function f is continuous at every point on the real line, Hence, f has no point of discontinuity.
Question 12. Find all points of discontinuity of f, where f is defined by f(x)= \(\begin{cases}x^{10}-1, & \text { if } x \leq 1 \\ x^2, & \text { if } x>1\end{cases}\)
Solution:
The given function is f(x)= \(\begin{cases}x^{10}-1, & \text { if } x \leq 1 \\ x^2, & \text { if } x>1\end{cases}\)
The function \(\mathrm{f}(\mathrm{x})\) may be discontinuous at doubtful point x=1 and except this point f(x) be continuous.
Now, at x=1, then the left hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{-}} f(x)=\lim _{x \rightarrow 1^{-}}\left(x^{10}-1\right)=1^{10}-1=1-1=0\)
The right hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{+}} f(x)=\lim _{x \rightarrow 1^{+}}\left(x^2\right)=1^2=1\)
It is observed that the left and right-hand limit of f at x=1 do not coincide.
Therefore,f is not continuous at x=1
Thus, from the above observation, it can be concluded that x=1 is the only point of discontinuity of f.
Question 13. Is the function defined by f(x)=\(\begin{cases}{l}x+5, \text { if } x \leq 1 \\ x-5, \text { if } x>1\end{cases}\).. a continuous function?
Solution:
The given function is f(x)=\(\begin{cases}{l}x+5, \text { if } x \leq 1 \\ x-5, \text { if } x>1\end{cases}\).
Function \(\mathrm{f}(\mathrm{x})\) may be discontinuous at doubtful point x=1 and except these point \(\mathrm{f}(\mathrm{x})\) be continuous.
Now at x=1, f(1)=1+5=6
The left hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{-}} f(x)=\lim _{x \rightarrow 1^{-}}(x+5)=1+5=6\)
The right hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{+}} f(x)=\lim _{x \rightarrow 1^{+}}(x-5)\)=1-5=-4
It is observed that the left and right-hand limits of f at x 1 do not coincide.
Therefore, f is not continuous at x- 1
Thus, from the above observation, it can be concluded that x- 1 is the only point of discontinuity of f.
Question 14. Discuss the continuity of the function f, where f is defined by f(x)= \(\begin{cases}3, & \text { if } 0 \leq x \leq 1 \\ 4, & \text { if } 1<x<3 \\ 5, & \text { if } 3 \leq x \leq 10\end{cases}\)
Solution:
The given function is \(f(\mathrm{x})=\{\begin{array}{l}3, \text { if } 0 \leq \mathrm{x} \leq 1 \\ 4, \text { if } 1<x<3 \\ 5, \text { if } 3 \leq \mathrm{x} \leq 10\end{array}\).
Function \(\mathrm{f}(\mathrm{x})\) may be discontinuous at doubtful points x=1 and x=3 and except these two points \(\mathrm{f}(\mathrm{x})\) be continuous.
Now at x=1, {f}(1)=3
The left hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{-}} f(x)=\lim _{x \rightarrow 1^{-}}(3)\)=3
The right hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{+}} f(x)=\lim _{x \rightarrow 1^{+}}(4)=4\)
It is observed that the left and right-hand limits of f at x=1 do not coincide.
Therefore, f is not continuous at x=1 and at x=3, f(3)=5
The left hand limit of f at x=3 is, \(\lim _{x \rightarrow 3^{-}} f(x)=\lim _{x \rightarrow 3^{-}}(4)=4\)
The right hand limit of f at x=3 is, \(\lim _{x \rightarrow 3^{+}} f(x)=\lim _{x \rightarrow 3^{+}}(5)=5\)
It is observed that the left and right-hand limits of f at x=3 do not coincide.
Therefore. f is not continuous at x=3. Hence, f is not continuous at x=1 and x =3
Question 15. Discuss the continuity of the function f, where f is defined by f(x)= \(\begin{cases}2 x, & \text { if } x<0 \\ 0, & \text { if } 0 \leq x \leq 1 \\ 4 x, & \text { if } x>1\end{cases}\)
Solution:
The given function is f(x)=\(\{\begin{array}{l}2 x, \text { if } x<0 \\ 0, \text { if } 0 \leq x \leq 1 \\ 4 x, \text { if } x>1\end{array}\).
Function \(\mathrm{f}(\mathrm{x})\) may be discontinuous at doubtful points x=0 and x = 1 and except these two points \mathrm{f}(\mathrm{x}) be continuous.
Now, at x=0, f(0)=0
The left hand limit of f at x=0 is, \(\lim _{x \rightarrow 0^{-}} f(x)=\lim _{x \rightarrow 0^{-}}(2 x)=2 \times 0=0\)
The right hand limit of f at x=0 is, \(\lim _{x \rightarrow 0^{+}} f(x)=\lim _{x \rightarrow 0^{+}}(0)=0\)
⇒ \(\lim _{x \rightarrow 0^{-}} f(x)=\lim _{x \rightarrow 0^{+}} f(x) \Rightarrow \text { limit exist }\)
⇒ \(\lim _{x \rightarrow 0} f(x)=f(0)\)
Therefore, f is continuous at x=0 and at x=1, f(1)=0
The left hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{-}} f(x)=\lim _{x \rightarrow 1^{-}}(0)=0\)
The right hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{+}} f(x)=\lim _{x \rightarrow 1^{+}}(4 x)=4 \times\) 1=4
It is observed that the left and right-hand limits of fat x = 1 do not coincide.
Therefore, f is not continuous at x = 1. Hence, f is not continuous only at x. = 1
Question 16. Discuss the continuity of the function f, where f is defined by f(x)= \(\begin{cases}-2, & \text { if } x \leq-1 \\ 2 x, & \text { if }-1<x \leq 1 \\ 2, & \text { if } x>1\end{cases}\)
Solution:
The given function f is f(x)= \(\begin{cases}-2, & \text { if } x \leq-1 \\ 2 x, & \text { if }-1<x \leq 1 \\ 2, & \text { if } x>1\end{cases}\)
Function \(\mathrm{f}(\mathrm{x})\) may be discontinuous at doubtful points x=-1 and x=1 and except these two points \(\mathrm{f}(\mathrm{x})\) be continuous.
Now, at x=-1, f(-1)=-2
The right hand limit of f at x=-1 is, \(\lim _{x \rightarrow-1^{+}} f(x)=\lim _{x \rightarrow-1^{+}}(2 x)=2 x(-1)=-2\)
⇒ \(\lim _{x \rightarrow-1^{-}} f(x)=\lim _{x \rightarrow-1^{+}} f(x) \Rightarrow \text { limit exist } \)
⇒ \(\lim _{x \rightarrow-1^{-}} f(x)=f(-1)\)
Therefore, f is continuous at x =-1 and at x=1, \(\mathrm{f}(1)\)=2 \times 1=2
The left hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{-}} f(x)=\lim _{x \rightarrow 1^{-}}(2 x)=2 \times 1\)=2
The right hand limit of f at x=1 is, \(\lim _{x \rightarrow 1^{+}} f(x)=\lim _{x \rightarrow 1^{+}}\) 2=2
⇒ \(\lim _{x \rightarrow 1^{-}} f(x)=\lim _{x \rightarrow 1^{+}} f(x) \Rightarrow \text { limit exist }\)
⇒ \(\lim _{x \rightarrow 1} f(x)=f(1)\)
Thus, from the above observations, it can be concluded that is continuous at all points of the real line.
Question 17. Find the relationship between a and b so that the function f defined by f(x)= \(\begin{cases} an x+1, & \text { if } x \leq 3 \\ b x+3, & \text { if } x>3\end{cases}\) is continuous at x=3.
Solution:
The given function f is f(x)=\(\{\begin{array}{ll}a x+1, & \text { if } x \leq 3 \\ b x+3, & \text { if } x>3\end{array}\).
If f is continuous at x=3, then
⇒ \(\lim _{x \rightarrow 3^{+}} f(x)=\lim _{x \rightarrow 3^{+}} f(x)=f(3)\)
Also, \(\lim _{x \rightarrow 3^{-}} f(x)=\lim _{x \rightarrow 3^{-}}(a x+1)=3 a+1\)
⇒ \(\lim _{x \rightarrow 3^{+}} f(x)=\lim _{x \rightarrow 3^{+}}(b x+3)=3 b+3 \Rightarrow f(3)\)=3 a+1
Therefore, from (1), we obtain.
3 a+1=3 b+3=3 a+1 \(\Rightarrow 3 a+1=3 b+3 \Rightarrow 3 a=3 b+2 \Rightarrow a-b=\frac{2}{3}\)
Therefore, the required relationship is given by a-b=\(\frac{2}{3}\)
Question 18. For what value of \(\lambda\) is the function defined by f(x)=\(\begin{cases} {cc}\lambda(x^2-2 x), & \text { if } x \leq 0 \\ 4 x+1, & \text { if } x>0\end{cases} \). continuous at x=0? What about continuity at x=1?
Solution:
The given function f is f(x)=\(\begin{cases}{cc}\lambda(x^2-2 x), & \text { if } x \leq 0 \\ 4 x+1, & \text { if } x>0\end{cases}\).
If f is continuous at x=0, then \(\lim _{x \rightarrow 0^{-}} f(x)=\lim _{x \rightarrow 0^{+}} f(x)=f(0)\)
\(\lim _{x \rightarrow 0^{-}} \lambda\left(x^2-2 x\right)=\lim _{x \rightarrow 0^{+}}(4 x+1)=\lambda\left(0^2-2 \times 0\right)\)
\(\lambda\left(0^2-2 \times 0\right)=4 \times 0+1=0 \Rightarrow 0=1=0\), which is not possible
Therefore, there is no value of \(\lambda\) for which f is continuous at x=0 At x=1, f(1)=4 x+1=4 \(\times \)1+1=5
⇒ \(\lim _{x \rightarrow 1}(4 x+1)=4 \times 1+1=5 \Rightarrow \lim _{x \rightarrow 1} f(x)=f(1)\)
Therefore, for any value of \lambda, f is continuous at x=1
Question 19. Show that the function defined by g(x)=x-[x] is discontinuous at all integral points. Here [x] denotes the greatest integer less than or equal to x.
Solution:
The given function is \(\mathrm{g}(\mathrm{x})=\mathrm{x}-[\mathrm{x}]\). It is evident that g is defined at all integral points.
Let n be an integer. Then, g(n)=n-[n]=n-n=0
The left hand limit of g at x=n is, \(\lim _{x \rightarrow n^{-}} g(x)=\lim _{x \rightarrow n^{-}}(x-[x])=\lim _{x \rightarrow n^{-}}(x)-\lim _{x \rightarrow n^{-}}[x]=n-(n-1)=1\)
The right hand limit of g at x=n is, \(\lim _{x \rightarrow n^{+}} g(x)=\lim _{x \rightarrow n^{+}}(x-[x])=\lim _{x \rightarrow n^{+}}(x)-\lim _{x \rightarrow n^{+}}[x]\)=n-n=0
It is observed that the left and right-hand limits of g at x=n do not coincide.
Therefore, g is not continuous at x=n
Hence,g is discontinuous at all integral points.
Question 20. Is the function defined by \(\mathrm{f}(\mathrm{x})=\mathrm{x}^2-\sin \mathrm{x}+5\) continuous at x=\(\pi\) ?
Solution:
The given function is f(x)=\(x^2-\sin x+5\)
At x=\(\pi, f(\pi)=\pi^2-\sin \pi+5=\pi^2-0+5=\pi^2+5\) and \(\lim _{x \rightarrow \pi} f(x)=\lim _{x \rightarrow \pi}\left(x^2-\sin x+5\right)\)
⇒ \(\lim _{x \rightarrow \pi} f(x)=\pi^2-\sin \pi+5 \Rightarrow \lim _{x \rightarrow \pi} f(x)=\pi^2+5\)
⇒ \(\lim _{x \rightarrow \pi} f(x)=f(\pi)=\pi^2+5\)
Question 21. Discuss the continuity of the following functions.
- f(x) = sin x + cos x
f(x) = sin x- cos x
f(x) = sin x x cos x
Solution:
By the property of continuity, we know that addition, subtraction, and multiplication of two continuous functions are always continuous.
Here, h(x) = sin x and g(x) = cos x are two continuous functions in R.
Therefore, sin x +cosx, sinx-cosx, and sin x.cosx are also continuous in R.
Question 22. Discuss the continuity of the cosine, cosecant, secant, and cotangent functions.
Solution:
It is known that if g and h are two continuous functions, then
⇒ \(\frac{\mathrm{h}(\mathrm{x})}{\mathrm{g}(\mathrm{x})}, \mathrm{g}(\mathrm{x}) \neq 0\) is continuous
⇒ \(\frac{1}{\mathrm{~g}(\mathrm{x})}, \mathrm{g}(\mathrm{x}) \neq 0\) is continuous
⇒ \(\frac{1}{h(x)}, h(x) \neq 0\) is continuous
It has to be proved first that g(x)=sin x and h(x)=cos x are continuous functions.
Let g(x)=sin x
It is evident that gx=sin x is defined for every real number.
Let c be a real number. Put x = c + h
If x \(\rightarrow\) c, then h \(\rightarrow\) 0
gc = sin c
⇒ \(\lim _{x \rightarrow c} g(x)=\lim _{x \rightarrow c} \sin x=\lim _{h \rightarrow 0} \sin (c+h)\)
= \(\lim _{h \rightarrow 0}[\sin c \cos h+\cos c \sin h]=\lim _{h \rightarrow 0}(\sin c \cos h)+\lim _{h \rightarrow 0}(\cos c \sin h) \)
= \(\sin \mathrm{c} \cos 0+\cos \mathrm{c} \sin 0=\sin \mathrm{c}+0=\sin \mathrm{c}\)
⇒ \(\lim _{x \rightarrow c} g(x)=g(c)\)
Therefore, g is a continuous function. Let \(\mathrm{h}(\mathrm{x})= cos x \)It is evident that h}(x)=cos x is defined for every real number.
Let c be a real number. Put x=c+h
If x \(\rightarrow\) c, then h \(\rightarrow\) 0, h{c}=cos c
⇒ \(\lim _{x \rightarrow c} h(x)=\lim _{x \rightarrow c} \cos x=\lim _{h \rightarrow 0} \cos (c+h)\)
=\(\lim _{h \rightarrow 0}[\cos c \cos h-\sin c \sin h]=\lim _{h \rightarrow 0} \cos c \cos h-\lim _{h \rightarrow 0} \sin c \sin h \)
=\(\cos c \cos 0-\sin c \sin 0=\cos c \times 1-\sin c \times 0=\cos c\)
⇒ \(\lim _{x \rightarrow c} h(x)=h(c)\)
Therefore, h(x)=cos x is a continuous function.
It can be concluded that,\({cosec} x=\frac{1}{\sin x}, \sin x \neq 0\) is continuous
⇒ \({cosec} x, x \neq n \pi(n \in Z)\) is continuous
Therefore, cosecant is continuous except at x=n p, n \(\in\) Z
sec x=\(\frac{1}{\cos x}, \cos x \neq 0\) is continuous
⇒ \(\sec x, x \neq(2 n+1) \frac{\pi}{2}(n \in Z)\) is continuous
Therefore, secant is continuous except at x=\((2 n+1) \frac{\pi}{2}(n \in Z)\)
⇒ \(\cot x=\frac{\cos x}{\sin x}, \sin x \neq 0\) is continuous
⇒ \(\cot x, x \neq n \pi(n \in Z)\) is continuous
Therefore, cotangent is continuous except at x=n \(\pi, n \in Z\)
Question 23. Find the points of discontinuity of f, where f(x)= \(\begin{cases}\frac{\sin x}{x}, & \text { if } x<0 \\ x+1, & \text { if } x \geq 0\end{cases}\)
Solution:
The given function f(x)= \(\begin{cases}\frac{\sin x}{x}, & \text { if } x<0 \\ x+1, & \text { if } x \geq 0\end{cases}\)
f(x) may be discontinuous at doubtful point x=0 and except this point $f(x)$ be continuous.
Now, at x=0, f(0)=0+1=1
The left hand limit of f at x=0 is, \(\lim _{x \rightarrow 0^{\circ}} f(x)=\lim _{x \rightarrow 0} \frac{\sin x}{x}=1\)
The right hand limit of f at x=0 is, \(\lim _{x \rightarrow 0} f(x)=\lim _{x \rightarrow 0^{+}}(x+1)=1\)
⇒ \(\lim _{x \rightarrow 0^{-}} f(x)=\lim _{x \rightarrow 0^{+}} f(x)=f(0)\)
Therefore, f is continuous at x =0
Thus, f has no point of discontinuity,
Question 24. Determine if f defined by f(x)=\(\begin{cases}{cl}x^2 \sin \frac{1}{x}, & \text { if } x \neq 0 \\ 0, & \text { if } x=0\end{cases}\). is a continuous function?
Solution:
The given function f is f(x)=\(\begin{cases}{cc}x^2 \sin \frac{1}{x}, & \text { if } x \neq 0 \\ 0 . & \text { if } x=0\end{cases}\).
f(x) may be discontinuous at doubtful point x=0 and except this point f(x) be continuous,
Now, at x =0, f(0)=0
⇒ \(\lim _{x \rightarrow 0} f(x)=\lim _{s \rightarrow 0} x^2 \sin \frac{1}{x}=0 \times(\text { Finite value from } 0 \text { to 1) }\)
⇒ \(\lim _{x \rightarrow 0} f(x)\)=0
Here, \(\lim _{x \rightarrow \infty} f(x)\)=f(0)
Therefore, f is continuous at x=0
Question 25. Examine the continuity of f, where f is defined by \(f(x)=\begin{cases}{cl}\sin x-\cos x & \text {, if } x \neq 0 \\ -1 & \text {, if } x=0\end{cases}\).
Solution:
The given function f(x)=\(\begin{cases}{cl}\sin x-\cos x & \text {, if } x \neq 0 \\ -1 & \text {, if } x=0\end{cases}\).
f(x) may be discontinuous at doubtful point x=0 and except this point f(x) be continuous. Now, at x=0, f(0)=-1
L.H.L =\(\lim _{x \rightarrow 0^{\circ}} f(x)=\lim _{x \rightarrow 0}(\sin x-\cos x)=\sin \theta-\cos 0=0-1=-1\)
R.H.L =\(\lim _{x \rightarrow x^2} f(x)=\lim _{x \rightarrow x^0}(\sin x-\cos x)=\sin 0-\cos 0=0-1=-1\)
⇒ \(\lim _{x \rightarrow 0^{-}} f(x)=\lim _{x \rightarrow+0^{+}} f(x)=f(0)\)
Therefore, f is continuous at x=0
Thus, f is a continuous function.
Question 26. Find the values of k so that the function f is continuous at the indicated point.
Solution:
f(x)=\(\begin{cases}{cc}\frac{k \cos x}{\pi-2 x} & \text {, if } x \neq \frac{\pi}{2} \\ 3 & \text {,if } x=\frac{\pi}{2}\end{cases}\). at x=\(\frac{\pi}{2}\)
The given function f(x)=\(\{\begin{cases}{cl}\frac{k \cos x}{\pi-2 x} & \text {, if } x \neq \frac{\pi}{2} \\ 3 & \text {, if } x=\frac{\pi}{2}\end{cases}\). is continuous at x=\(\frac{\pi}{2}\),
⇒ \(\lim _{x \rightarrow} f(x)=f\left(\frac{\pi}{2}\right)\)=3
Now, \(\lim _{\rightarrow \rightarrow-1} f(x)=\lim _{x \rightarrow \frac{\pi}{2}} \frac{k \cos x}{\pi-2 x}\)
Pet x=\(\frac{\pi}{2}+h, then, x \rightarrow \frac{\pi}{2} \Rightarrow h \rightarrow 0\)
⇒ \(\lim _{x \rightarrow+} f(x)=\lim _{x \rightarrow \frac{\pi}{2}} \frac{k \cos x}{\pi-2 x}=\lim _{x \rightarrow 4} \frac{k \cos \left(\frac{\pi}{2}+h\right)}{\pi-2\left(\frac{\pi}{2}+h\right)}\)
=k \(\lim _{b \rightarrow 0} \frac{-\sin h}{-2 h}=\frac{k}{2} \lim _{x \rightarrow \infty} \frac{\sin h}{h}=\frac{k}{2}, 1=\frac{k}{2}\)
⇒ \(\lim _{\rightarrow-\frac{1}{2}} f(x)=f\left(\frac{\pi}{2}\right) (From eq. (1))\)
⇒ \(\frac{k}{2}\)=3
k=6
Question 27. Find the values of k so that the function f is continuous at the indicated point:
Solution:
f(x)=\(\begin{cases}{l}
k x^2 & \text {, if } x \leq 2 \\
3 & \text {,if } x>2
\end{cases} \text { at } x=2t\).
The given function is f(x)=\(\begin{cases}{cl}k x^3 & \text { if } x \leq 2 \\ 3 & \text {, if } x>2\end{cases}\).
Is continuously at x=2,
⇒ \(\lim _{x \rightarrow x^2} f(x)=\lim _{x \rightarrow 0} f(x)=f(2) \Rightarrow \lim _{x \rightarrow 7}\left(k x^2\right)=\lim _{x \rightarrow 1}(3)=4 k\)
k \(\times 2^2=3=4 k \Rightarrow 4 k=3=4 k \Rightarrow 4 k=3 \Rightarrow k=\frac{3}{4}\)
Therefore, the required value of k is \(\frac{3}{4}\)
Question 28. Find the values of k so that the function f is continuous at the indicated point:
Solution:
f(x)=\(\begin{cases}{ll}
k x+1, & \text { if } x \leq \pi \\
\cos x, & \text { if } x>\pi
\end{cases} \text { at } x=\pi\).
The given function f(x)= \(\begin{cases}k x+1, & \text { if } x \leq \pi \\ \cos x, & \text { if } x>\pi\end{cases}\)
Is continuous at \(\mathrm{x}=\pi\)
⇒ \(\lim _{x \rightarrow 1^{-}} f(x)=\lim _{x \rightarrow m^{+}} f(x)=f(\pi) \Rightarrow \lim _{x \rightarrow z^{+}}(k x+1)=\lim _{x \rightarrow \pm^{-}} \cos x=k \pi+1 \)
k \(\pi+1=\cos \pi=k \pi+1 \Rightarrow k \pi+1=-1=k \pi+1 \Rightarrow\)
k=-\(\frac{2}{\pi}\)
Therefore, the required value of k is \(-\frac{2}{\pi}\)
Question 29. Find the values of k so that the function f is continuous at the indicated point:
Solution:
f(x)=\(\{\begin{array}{ll}
k x+1, & \text { if } x \leq 5 \\
3 x-5, & \text { if } x>5
\end{array}\) at x = 5
The given function f(x)= \(\begin{cases}k x+1, & \text { if } x \leq 5 \\ 3 x-5, & \text { if } x>5\end{cases}\)
Is continuous at x=5,
⇒ \(\lim _{x \rightarrow 5^5} f(x)=\lim _{x \rightarrow 5^5} f(x)=f(5) \Rightarrow \lim _{x \rightarrow 5^{(}}(k x+1)=\lim _{x \rightarrow 5^{(}}(3 x-5)=5 k+1\)
5 k+1=15-5=5 k+1 \(\Rightarrow 5 k+1=10 \Rightarrow 5 k=9 \Rightarrow k=\frac{9}{5}\)
Therefore, the required value of k is \(\frac{9}{5}\)
Question 30. Find the values of a and b such that the function defined by f(x)=\(\begin{cases}{cl}5, & \text { if } x \leq 2 \\ an x+b, & \text { if } 2<x<10 \\ 21, & \text { if } x \geq 10\end{cases}\) is a continuous function.
Solution:
The given function f(x)=\(\begin{cases}{cl}5, & \text { if } x \leq 2 \\ a x+b, & \text { if } 2<x<10 \text { is continuous function. } \\ 21, & \text { if } x \geq 10\end{cases}.\)
Since f is continuous at x=2, we obtain \(\lim _{x \rightarrow 2} f(x)=\lim _{x \rightarrow z^2} f(x)=f(2)\)
⇒ \(\lim _{x \rightarrow 5}(5)=\lim _{x \rightarrow 2}(a x+b)=5 \Rightarrow 5=2 a+b=5 \Rightarrow 2 a+b=5\) → Equation 1
Since f is continuous at x=10, we obtain
⇒ \(\lim _{x \rightarrow 10^{-}} f(x)=\lim _{x \rightarrow 10^{+}} f(x)=f(10) \Rightarrow \lim _{x \rightarrow 10^{-}}(a x+b)=\lim _{x \rightarrow 10^0}(21)=21\) → Equation 2
10 a+b=21=21 \(\Rightarrow\) 10 a+b=21
By subtracting equation (1) from equation (2), we obtain
8 \(\mathrm{a}=16 \Rightarrow \mathrm{a}=2\)
By putting a-2 in equation ( 1 ), we obtain
2 \(\approx 2+b=5 \Rightarrow 4+b=5 \Rightarrow\) b=1
Therefore, the values of a and b for which f is a continuous function are 2 and 1 respectively.
Question 31. Show that the function defined by f(x)=p\(\cos \left(x^2\right)\) is a continuous function.
Solution:
Given f(x)=\(\cos x^2\)
Let g(x)=\(\mathrm{x}^2 and h(x)=cos x\)
⇒ \(\mathrm{f}(\mathrm{x})=\cos (\mathrm{g}(\mathrm{x}))=\mathrm{h}(\mathrm{g}(\mathrm{x})) \Rightarrow f(\mathrm{x})={hog}(\mathrm{x})\)
g(x) is a polynomial function, which is continuous in its domain R so,g(x) is continuous.
Again h(x)=cos x is a trigonometric function that is continuous in {R}.
So, it is also continuous.
Since g(x) and h(x) is continuous.
i.e. h o g(x) is also continuous.
Hence, f(x) is a continuous function.
Question 32. Show that the function defined by f(x)=\(\mid \cos x\) is a continuous function.
Solution:
Given f(x)=|cos x|
Let g(x)= cos x and h(x)=|x|
⇒ \(\mathrm{f}(\mathrm{x})=|\mathrm{g}(\mathrm{x})|=\mathrm{h}(\mathrm{g}(\mathrm{x})) \Rightarrow \mathrm{f}(\mathrm{x})={hog}(\mathrm{x})\)
g(x) is a trigonometric function, which is continuous in its domain R.
So, g(x) is continuous
h}(x)=|x| is a modulus function, which is continuous in R.
So, it is also continuous.
Since g(x) and h(x) is continuous.
i.e. hog (x) is also continuous.
Hence, \(\mathrm{f}(\mathrm{x})=|\cos \mathrm{x}|\) is continuous function.
Question 33. Examine that sin x \(\mid\) is a continuous function.
Solution:
Let f(x)=sin |x|
This function f is defined for every real number and f can be written as the composition of two functions as,
Given f(x)=sin |x|
Let g(x)-|x| and h(x)=sin x
f(x)=sin (g(x))=h(g(x)) \(\Rightarrow\) f(x)=h o g(x)
g(x) is the module’s function, which is continuous in its domain R.
So, g(x) is continuous.
Again h (x) = sin x is a trigonometric function, which is continuous in R.
So, it is also continuous.
Since, g (x) and h (x) is continuous,
i.e. hog (x) is also continuous.
Hence, f (x)- sin jx] is a continuous function.
Question 34. Find all the points of discontinuity of f defined by f(x)=|x|-|x+1|
Solution:
Given that f(x)=|x|-|x+1|
f(x)=\(\begin{cases}{cc}
1 & \text { if } x<-1 \\
-2 x-1 & \text { if }-1 \leq x<0 \\
-1 & \text { if } x \geq 0
\end{cases}\)
Here, f(x) may be discontinuous at doubtful points x-1, x=0 and except these two points f(x) be continuous,
Now at x=-1
L.H.L =\(\lim _{x \rightarrow-1} f(x)=\lim _{x \rightarrow-1}(1)\)=1
R.H.L. =\(\lim _{x \rightarrow-1} f(x)=\lim _{x \rightarrow-1}(-2 x-1)\)=1
L.H.S. = R.H.S. \(\Rightarrow\) limit exist and {f}(-1)=1
Here \(\lim f(x)=f(-1)\)
So, f(x) is continuous at x=-1
Again at x=0
L.H.L. =\(\lim _{x \rightarrow 0^{+}} f(x)=\lim _{x \rightarrow 0^{+}}(-2 x-1)=-1 \)
R.H.L.=\(\lim _{x \rightarrow 0^{+}} f(x)=\lim _{x \rightarrow 0^{+}}(-1)=-1 \)
L.H.L = R.H.L \(\Rightarrow \text { limit exist and }\) f(0)=-1
Here \(\lim _{x \rightarrow 0} f(x)\)=f(0)
So, f(x) is continuous at x=0
Hence, there is no point of discontinuity for f(x).
Continuity And Differentiability Exercise 5.2
Question 1. \(\sin \left(x^2+5\right)\)
Solution:
⇒ \(\frac{d}{d x}\left[\sin \left(x^2+5\right)\right] =\cos \left(x^2+5\right) \cdot \frac{d}{d x}\left(x^2+5\right)\)
= \(\cos \left(x^4+5\right) \cdot\left[\frac{d}{d x}\left(x^2\right)+\frac{d}{d x}(5)\right]=\cos \left(x^2+5\right) \cdot[2 x+0]=2 x \cos \left(x^2+5\right)\)
Question 2. \(\cos (\sin x) \)
Solution:
⇒ \(\frac{d}{d x}[\cos (\sin x)]=-\sin (\sin x) \cdot \frac{d}{d x}(\sin x)\)
=-\(\sin (\sin x) \cdot \cos x=-\cos x \sin (\sin x)\)
Question 3. sin (a x+b)
Solution:
⇒ \(\frac{d}{d x}[\sin (a x+b)]=\cos (a x+b) \cdot \frac{d}{d x}(a x+b)\)
=\(\cos (a x+b) \cdot\left[\frac{d}{d x}(a x)+\frac{d}{d x}(b)\right]=\cos (a x+b) \cdot(a+0)=a \cos (a x+b)\)
Question 4. \(\sec (\tan (\sqrt{x}))\)
Solution:
⇒ \({\frac{d x}{d x}[\sec (\tan \sqrt{x})]} =\sec (\tan \sqrt{x}) \cdot \tan (\tan \sqrt{x}) \cdot \frac{d}{d x}(\tan \sqrt{x})\)
= \(\sec (\tan \sqrt{x}) \cdot \tan (\tan \sqrt{x}) \cdot \sec ^2(\sqrt{x}) \cdot \frac{d}{d x}(\sqrt{x}) \)
= \(\sec (\tan \sqrt{x}) \cdot \tan (\tan \sqrt{x}) \cdot \sec ^2(\sqrt{x}) \cdot \frac{1}{2 \sqrt{x}}\)
= \(\frac{\sec (\tan \sqrt{x}) \cdot \tan (\tan \sqrt{x}) \sec ^2(\sqrt{x})}{2 \sqrt{x}}\)
Question 5. \(\frac{\sin (a x+b)}{\cos (c x+d)}\)
Solution:
Let f(x)=\(\frac{\sin (a x+b)}{\cos (c x+d)}=\frac{u(x)}{v(x)}\)
⇒ \(f^{\prime}(x)=\frac{v(x)\left[u^{\prime}(x)\right]-u(x)\left[v^{\prime}(x)\right]}{[v(x)]^2}\)
⇒ \(\mathrm{f}(\mathrm{x})=\frac{\cos (c x+d) \cdot a \cos (a x+b)+\sin (a x+b) \cdot c \sin (c x+d)}{\cos ^2(c x+d)}\)
⇒ \(f^{\prime}(x)=a \cos (a x+b) \cdot \sec (c x+d)+c \sin (a x+b) \cdot \tan (c x+d) \cdot \sec (c x+d)\)
Question 6. \(\cos x^3 \cdot \sin ^2\left(x^5\right)\)
Solution:
The given function is \(\cos x^3-\sin ^2\left(x^3\right)\)
⇒ \(\frac{d}{d x}\left[\cos x^3 \cdot \sin ^2\left(x^5\right)\right]\)
= \(\sin ^2\left(x^5\right) \times \frac{d}{d x}\left(\cos x^3\right)+\cos x^3 \times \frac{d}{d x}\left[\sin ^2\left(x^5\right)\right]\)
= \(\sin ^2\left(x^5\right) \times\left(-\sin x^3\right) \times \frac{d}{d x}\left(x^3\right)+\cos x^5 \times 2 \sin \left(x^5\right) \cdot \frac{d}{d x}\left[\sin x^4\right]\)
=-\(\sin x^3 \sin ^2\left(x^5\right) \times 3 x^2+2 \sin x^5 \cos x^3 \cdot \cos x^5 \times \frac{d}{d x}\left(x^5\right)\)
=-\(3 x^2 \sin x^3 \cdot \sin ^2\left(x^5\right)+2 \sin x^5 \cos x^5 \cos x^3 \times 5 x^4 \)
= \(10 x^4 \sin x^4 \cos x^4 \cos x^3-3 x^7 \sin x^5 \sin ^2\left(x^5\right)\)
Question 7. 2 \(\sqrt{\cot \left(x^2\right)}\)
Solution:
⇒ \(\frac{d}{d x}\left[2 \sqrt{\cot \left(x^2\right)}\right]=2 \cdot \frac{1}{2 \sqrt{\cot \left(x^2\right)}} \times \frac{d}{d x}\left[\cot \left(x^2\right)\right]\)
=\(\sqrt{\frac{\sin \left(x^2\right)}{\cos \left(x^2\right)}} \times-{cosec}^2\left(x^2\right) \times \frac{d}{d x}\left(x^2\right)\)
=-\(\sqrt{\frac{\sin \left(x^2\right)}{\cos \left(x^2\right)}} \times \frac{1}{\sin ^2\left(x^2\right)} \times(2 x)\)
= \(\frac{-2 x}{\sqrt{\cos x^2 \sqrt{\sin x^2} \sin x^2}}=\frac{-2 \sqrt{2} x}{\sqrt{2 \sin x^2 \cos x^2}\left(\sin x^2\right)}\)
= \(\frac{-2 \sqrt{2} x}{\sin x^2 \sqrt{\sin 2 x^2}}\)
Question 8. \(\cos (\sqrt{x}\)
Solution:
⇒ \(\frac{d}{d x}[\cos (\sqrt{x})]=-\sin (\sqrt{x}) \cdot \frac{d}{d x}(\sqrt{x})=\frac{-\sin \sqrt{x}}{2 \sqrt{x}}\)
Question 9. Prove that the function f is given by f(x)=|x-1|, x \(\in \mathbf{R}\) is not differentiable at x=1.
Solution:
The given function is f(x)=\(|\mathrm{x}-1|, \mathrm{x} \in \mathbf{R}\)
It is known that a function f is differentiable at a point x=c in its domain if both \(\lim _{\mathrm{h} \rightarrow 0}\)
⇒ \(\frac{\mathrm{f}(\mathrm{c}-\mathrm{h})-\mathrm{f}(\mathrm{c})}{-\mathrm{h}} and \lim _{\mathrm{h} \rightarrow 0}\)
⇒ \(\frac{\mathrm{f}(\mathrm{c}+\mathrm{h})-\mathrm{f}(\mathrm{c})}{\mathrm{h}}\)are finite and equal.
To check the differentiability of the given function at x=1, consider the left-hand derivative of f at x=1
L.H.D. =\(\lim _{h \rightarrow 0} \frac{f(1-h)-f(1)}{-h}=\lim _{h \rightarrow 0} \frac{|1-h-1|-|1-1|}{-h}=\lim _{h \rightarrow 0}\left(\frac{h-0}{-h}\right)=-1\)
Consider the right-hand derivative of f at x=1
R.H.D. =\(\lim _{h \rightarrow 0} \frac{f(1+h)-f(1)}{h}=\lim _{h \rightarrow 0} \frac{|1+h-1|-|1-1|}{h}=\lim _{h \rightarrow 0}\left(\frac{h-0}{h}\right)=1\)
Since the left and right-hand derivatives of f at x=1 are not equal, f is not differentiable at x-1
Question 10. Prove that the greatest integer function defined by \(\mathrm{f}(\mathrm{x})=[\mathrm{x}], 0<\mathrm{x}<3\), is not differentiable at x-1 and x-2.
Solution:
The given function f is f(x)=[x], 0<x<3
It is known that a function f is differentiable at a point x=c in its domain if both \(\lim _{\mathrm{h} \rightarrow 0} \frac{f(c-h)-f(c)}{-h} and \lim _{x \rightarrow \infty} \frac{f(c+h)-f(c)}{h}\) are finite and equal.
To check the differentiability of the given function at x=1, consider the left-hand derivative of f at x=1,
L.H.D. =\(\lim _{h \rightarrow 0} \frac{f(1-h)-f(1)}{-h}=\lim _{h \rightarrow 0} \frac{[1-h]-[1]}{-h}=\lim _{h \rightarrow 0} \frac{0-1}{-h}=\infty\)
Consider the right-hand derivative of f at x=1
R.H.D. =\(\lim _{h \rightarrow h} \frac{f(1+h)-f(h)}{h}=\lim _{h \rightarrow 0} \frac{[1+h]-[t]}{h}=\lim _{h \rightarrow 1} \frac{1-r}{h}=\lim _{h \rightarrow 0}\) 0=0
Since the left and right-hand derivatives of f at x=1 are not equal, f is not differentiable at x=1
To check the differentiability of the given function at x=2, consider the left-hand derivative of f at x-2
L.H.D. =\(\lim _{h \rightarrow h} \frac{f(2-h)-f(2)}{-h}=\lim _{h \rightarrow 0} \frac{[2-h]-[2]}{-h}=\lim _{h \rightarrow 0} \frac{1-2}{-h}=\lim _{h \rightarrow 0} \frac{-1}{-h}=\infty\)
Consider the right-hand derivative of f at x-1.
R.H.D \(-\lim _{h \rightarrow 0} \frac{f(2+h)-f(2)}{h}=\lim _{h \rightarrow h} \frac{[2+h]-[2]}{h}=\lim _{h \rightarrow 0} \frac{2-2}{h}=\lim _{h \rightarrow 0}\) 0=0
Since the left and right-hand derivatives of f at x=2 are not equal, f is not differentiable at x=2
Continuity And Differentiability Exercise 5.3
Question 1.1. 2 x+3 y = sin x
Solution:
⇒ \(\frac{d}{d x}(2 x+3 y)=\frac{d}{d x}(\sin x)\)
⇒ \(\frac{d}{d x}(2 x)+\frac{d}{d x}(3 y)=\cos x\)
2+3 \(\frac{d y}{d x}=\cos x \)
3 \(\frac{d y}{d x}=\cos x-2 \)
⇒ \(\frac{d y}{d x}=\frac{\cos x-2}{3}\)
Question 2. 2 x+3 y=sin y
Solution:
⇒ \(\frac{d}{d x}(2 x)+\frac{d}{d x}(3 y)=\frac{d}{d x}(\sin y)\)
⇒ \(2+3 \frac{d y}{d x}=\cos y \frac{d y}{d x}\) [By using chain rule] }
⇒ \(2=(\cos y-3) \frac{d y}{d x} \Rightarrow \frac{d y}{d x}=\frac{2}{\cos y-3}\)
Question 3. a x+b \(y^2=\cos y\)
Solution:
⇒ \(\frac{d}{d x}(a x)+\frac{d}{d x}\left(b y^2\right)=\frac{d}{d x}(\cos y)\)
a+b \(\frac{d}{d x}\left(y^2\right)=\frac{d}{d x}(\cos y)\)
Using the chain rule, we obtain
⇒ \(\frac{d}{d x}\left(y^2\right)=2 y \frac{d y}{d x} and \frac{d}{d x}(\cos y)=-\sin y \frac{d y}{d x}\)
From (1) and (2), we obtain
a+b \(\times 2 y \frac{d y}{d x}=-\sin y \frac{d y}{d x}\)
⇒ \((2 b y+\sin y) \frac{d y}{d x}=-a\)
⇒ \(\frac{d y}{d x}=\frac{-a}{2 b y+\sin y}\)
Question 4. \(x y+y^2=\tan x+y\)
Solution :
⇒ \(\frac{d}{d x}\left(x y+y^2\right)=\frac{d}{d x}(\tan x+y) \Rightarrow \frac{d}{d x}(x y)+\frac{d}{d x}\left(y^2\right)\)
= \(\frac{d}{d x}(\tan x)+\frac{d y}{d x}\)
⇒ \(\left[y \cdot \frac{d}{d x}(x)+x \cdot \frac{d y}{d x}\right]+2 y \frac{d y}{d x}\)
= \(\sec ^2 x+\frac{d y}{d x}\) [Using product rule and chain rule]
y \(\cdot 1+x \cdot \frac{d y}{d x}+2 y \frac{d y}{d x}=\sec ^2 x+\frac{d y}{d x} \Rightarrow(x+2 y-1) \frac{d y}{d x}=\sec ^2 x-y\)
⇒ \(\frac{d y}{d x}=\frac{\sec ^2 x-y}{(x+2 y-1)}\)
Question 5. \( x^2+x y+y^2\)=100
Solution:
⇒ \(\frac{d}{d x}\left(x^2+x y+y^2\right)=\frac{d}{d x}(100)\)
⇒ \(\frac{d}{d x}\left(x^2\right)+\frac{d}{d x}(x y)+\frac{d}{d x}\left(y^2\right)\)=0 [Derivative of constant function is 0 ]
⇒ \(2 x+\left[y \cdot \frac{d}{d x}(x)+x \cdot \frac{d y}{d x}\right]+2 y \frac{d y}{d x}\)=0 [Using product rule and chain rule]
⇒ \(2 x+y \cdot 1+x \cdot \frac{d y}{d x}+2 y \frac{d y}{d x}\)=0
⇒ \(2 x+y+(x+2 y) \frac{d y}{d x}\)=0
⇒ \(\frac{d y}{d x}=-\frac{2 x+y}{x+2 y}\)
Question 6. \(x^3+x^2 y+x y^2+y^3=81\)
Solution:
⇒ \(\frac{d}{d x}\left(x^3+x^2 y+x y^2+y^3\right)=\frac{d}{d x}(81)\)
⇒ \(\frac{d}{d x}\left(x^3\right)+\frac{d}{d x}\left(x^2 y\right)+\frac{d}{d x}\left(x y^2\right)+\frac{d}{d x}\left(y^3\right)\)=0
⇒ \(3 x^2+\left[y \frac{d}{d x}\left(x^2\right)+x^2 \frac{d y}{d x}\right]+\left[y^2 \frac{d}{d x}(x)+x \frac{d}{d x}\left(y^2\right)\right]+3 y^2 \frac{d y}{d x}\)=0
⇒ \(3 x^2+\left[y \cdot 2 x+x^2 \frac{d y}{d x}\right]+\left[y^2 \cdot 1+x \cdot 2 y \cdot \frac{d y}{d x}\right]+3 y^2 \frac{d y}{d x}\)=0
⇒ \(\left(x^2+2 x y+3 y^2\right) \frac{d y}{d x}+\left(3 x^2+2 x y+y^2\right)\)=0
⇒ \(\frac{d y}{d x}=\frac{-\left(3 x^2+2 x y+y^2\right)}{\left(x^2+2 x y+3 y^2\right)}\)
Question 7. \(\sin ^2 y+\cos x y=k\)
Solution:
⇒ \(\frac{d}{d x}\left(\sin ^2 y+\cos x y\right)=\frac{d}{d x}(k)\)
⇒ \(\frac{d}{d x}\left(\sin ^2 y\right)+\frac{d}{d x}(\cos x y)=0\)
Using the chain rule, we obtain
⇒ \(\frac{d}{d x}\left(\sin ^2 y\right) =2 \sin y \frac{d}{d x}(\sin y)=2 \sin y \cos y \frac{d y}{d x}\)
⇒ \(\frac{d}{d x}(\cos x y)=-\sin x y \frac{d}{d x}(x y)=-\sin x y\left[y \frac{d}{d x}(x)+x \frac{d y}{d x}\right]\)
=-\(\sin x y\left[y \cdot 1+x \frac{d y}{d x}\right]=-y \sin x y-x \sin x y \frac{d y}{d x}\)
From (1), (2), and (3), we obtain
2 \(\sin y \cos y \frac{d y}{d x}-y \sin x y-x \sin x y \frac{d y}{d x}=0 \)
⇒ \((2 \sin y \cos y-x \sin x y) \frac{d y}{d x}=y \sin x y\)
⇒ \((\sin 2 y-x \sin x y) \frac{d y}{d x}=y \sin x y\)
⇒ \(\frac{d y}{d x}=\frac{y \sin x y}{\sin 2 y-x \sin x y}\)
Question 8. \(\sin ^2 x+\cos ^2 y=1\)
Solution:
⇒ \(\frac{d}{d x}\left(\sin ^2 x+\cos ^2 y\right)=\frac{d}{d x}(1)\)
⇒ \(\frac{d}{d x}\left(\sin ^2 x\right)+\frac{d}{d x}\left(\cos ^2 y\right)=0 \Rightarrow 2 \sin x \cdot \frac{d}{d x}(\sin x)+2 \cos y \cdot \frac{d}{d x}(\cos y)=0\)
2 \(\sin x \cos x+2 \cos y(-\sin y) \cdot \frac{d y}{d x}\)=0
⇒ \(\sin 2 x-\sin 2 y \frac{d y}{d x}\)=0
⇒ \(\frac{d y}{d x}=\frac{\sin 2 x}{\sin 2 y}\)
Question 9. y=\(\sin ^{-1}\left(\frac{2 x}{1+x^2}\right)\)
Solution:
y=\(\sin ^{-1}\left(\frac{2 x}{1+x^2}\right)\)
Put x=\(\tan \theta\)
⇒ \(\theta=\tan ^{-t}\) x
⇒ \(y=\sin ^{-1}\left(\frac{2 x}{1+x^2}\right)=\sin ^{-1}\left(\frac{2 \tan \theta}{1+\tan ^2 \theta}\right)\)
y=\(\sin ^{-1}(\sin 2 \theta)=2 \theta\)
y=2 \(\tan ^{-1} x\)
∴ \(\frac{d y}{d x}=\frac{2}{1+x^2}\)
Question 10. y=\(\tan ^{-1}\left(\frac{3 x-x^3}{1-3 x^2}\right),-\frac{1}{\sqrt{3}}<x<\frac{1}{\sqrt{3}} \)
Solution:
y=\(\tan ^{-1}\left(\frac{3 x-x^3}{1-3 x^2}\right)\)
Put x=\(\tan \theta \Rightarrow \theta=\tan ^{-1}x\)
y=\(\tan ^{-1}\left(\frac{3 \tan \theta-\tan ^3 \theta}{1-3 \tan ^2 \theta}\right)=\tan ^{-1}(\tan 3 \theta)\)
y=3 \(\theta=3 \tan ^{-1} x\)
⇒ \(\frac{d y}{d x}=\frac{3}{1+x^2}\)
Question 11. y=\(\cos ^{-1}\left(\frac{1-x^2}{1+x^2}\right), 0<x<1\)
Solution:
y=\(\cos ^{-1}\left(\frac{1-x^2}{1+x^2}\right)\)
Put x=\(\tan \theta \Rightarrow \theta=\tan ^{-1}\) x Equation 1
y=\(\cos ^{-1}\left(\frac{1-\tan ^2 \theta}{1+\tan ^2 \theta}\right) \left[ \cos 2 \theta=\frac{1-\tan ^2 \theta}{1+\tan ^2 \theta}\right]\)
y=\(\cos ^{-1}(\cos 2 \theta)=2 \theta \quad \Rightarrow y=2 \tan ^{-1}\) x From Equation 1
⇒ \(\frac{d y}{d x}=2 \cdot \frac{1}{1+x^2}\)
Question 12. y=\(\sin ^{-1}\left(\frac{1-x^2}{1+x^2}\right)\), 0<x<1
Solution:
y=\(\sin ^{-1}\left(\frac{1-x^2}{1+x^2}\right)\)
Put x=\(\tan \theta \Rightarrow \theta=\tan ^{-1} x\)
y=\(\sin ^{-1}\left(\frac{1-\tan ^2 \theta}{1+\tan ^2 \theta}\right) \Rightarrow y=\sin ^{-1}(\cos 2 \theta)\)
y=\(\sin ^{-1}\left\{\sin \left(\frac{\pi}{2}-2 \theta\right)\right\}\)
y=\(\frac{\pi}{2}-20=\frac{\pi}{2}-2 \tan ^{-1} x\)
⇒ \(\frac{d y}{d x}=-2 \cdot\left(\frac{1}{1+x^2}\right)=\frac{-2}{1+x^2}\)
Question 13. y=\(\cos ^{-1}\left(\frac{2 x}{1+x^2}\right),-1<x<1 \)
Solution:
y=\(\cos ^{-1}\left(\frac{2 x}{1+x^2}\right)\)
Put x=\(\tan \theta \Rightarrow \theta=\tan ^{-1} x\) Equation 1
y=\(\cos ^{-1}\left(\frac{2 \tan \theta}{1+\tan ^2 \theta}\right)\) \(\left( \sin 2 \theta=\frac{2 \tan \theta}{1+\tan ^2 \theta}\right)\)
y=\(\cos ^{-1}(\sin 2 \theta)\)
y=\(\cos ^{-1}\left\{\cos \left(\frac{\pi}{2}-2 \theta\right)\right\} \Rightarrow y=\frac{\pi}{2}-2 \theta\)
⇒ \( y=\frac{\pi}{2}-2 \tan ^{-1} x\) [From eq. (1)]
⇒ \(\frac{d y}{d x}=\frac{-2}{1+x^2}\)
Question 14. y=\(\sin ^{-1}\left(2 x \sqrt{1-x^2}\right),-\frac{1}{\sqrt{2}}<x<\frac{1}{\sqrt{2}}\)
Solution:
y=\(\sin ^{-1}\left(2 x \sqrt{1-x^2}\right)\)
Put x=\(\sin \theta \Rightarrow \theta=\sin ^{-1}\) x Equation 1
y=\(\sin ^{-1}\left(2 \sin \theta \sqrt{1-\sin ^2 \theta}\right)=\sin ^{-1}(2 \sin \theta \cos \theta)\)
y=\(\sin ^{-1}(\sin 2 \theta)=2 \theta \Rightarrow y=2 \sin ^{-1} x\) From Equation 1
⇒ \(\frac{d y}{d x}=\frac{2}{\sqrt{1-x^2}}\)
Question 15. y=\(\sec ^{-1}\left(\frac{1}{2 x^2-1}\right), 0<x<\frac{1}{\sqrt{2}}\)
Solution:
y=\(\sec ^{-1}\left(\frac{1}{2 x^2-1}\right)\)
Put x=\(\cos \theta \Rightarrow \theta=\cos ^{-1}\) x
y=\(\sec ^{-1}\left(\frac{1}{2 \cos ^2 \theta-1}\right)=\sec ^{-1}\left(\frac{1}{\cos 2 \theta}\right)=\sec ^{-1}(\sec 2 \theta)=2 \theta \)
y=2 \(\cos ^{-1}\) x (From eq. (1))
∴ \(\frac{d y}{d x}=\frac{-2}{\sqrt{1-x^2}}\)
Continuity And Differentiability Exercise 5.4
Question 1. \(\frac{e^x}{\sin x}\)
Solution:
Let y=\(\frac{e^4}{\sin x}\)
By using the quotient rule, we obtain
⇒ \(\frac{d y}{d x} =\frac{\sin x \frac{d}{d x}\left(e^x\right)-e^x \frac{d}{d x}(\sin x)}{\sin ^2 x}\)
=\(\frac{e^x(\sin x-\cos x)}{\sin ^2 x}, x \neq n \pi, n \in Z\) \(\left[\frac{d}{d x}\left(\frac{u}{v}\right)=\frac{v \frac{d}{d x}(u)-u \frac{d}{d x}(v)}{v^2}\right]\)
Question 2. \(e^{sin^{-1} x}\)
Solution:
Let y=\(e^{sin x^{-1}}\)
By using the chain rule, we obtain
⇒ \(\frac{d y}{d x}=\frac{d}{d x}\left(e^{n=-1}\right)\)
= \(\frac{d y}{d x}=e^{n+1}+\frac{d}{d x}\left(\sin ^{-1} x\right)\)
= \(\frac{e^{-n^{-1}} x}{\sqrt{1-x^2}}\)
⇒ \(\frac{d y}{d x}=\frac{e^{m n^{-1} x}}{\sqrt{1-x^2}}, x \in(-1,1)\)
Question 3. \(e^{x^2}\)
Solution:
Let y=\(e^{x^2}\) (By using the chain rule, we obtain)
⇒ \(\frac{d y}{d x}=\frac{d}{d x}\left(e^{x^{\prime}}\right)=e^{x^3} \cdot 3 x^2=3 x^2 e^{x^{\prime}}\)
Question 4. \(\sin \left(\tan ^{-1} e^{-x}\right)\)
Solution:
Let y=\(\sin \left(\tan ^{-1} e^{-\pi}\right)\) (By using chain rule, we obtain)
⇒ \(\frac{d y}{d x}=\frac{d}{d x}\left[\sin \left(\tan ^{-1} e^{-x}\right)\right]\)
=\(\cos \left(\tan ^{-1} e^{-x}\right) \cdot \frac{d}{d x}\left(\tan ^{-t} e^{-1}\right)\)
=\(\cos \left(\tan ^{-1} e^{-x}\right) \frac{1}{1+\left(e^{-1}\right)^2} \cdot \frac{d}{d x}\left(e^{-x}\right)\)
=\(\frac{\cos \left(\tan ^{-1} e^{-1}\right)}{1+e^{-2 x}} \cdot e^{-x} \cdot \frac{d}{d x}(-x)\)
=\(\frac{e^{-3} \cos \left(\tan ^{-1} e^{-1}\right)}{1+e^{-t x}} \times(-1)=\frac{-e^{-1} \cos \left(\tan ^{-1} e^{-t}\right)}{1+e^{-5 x}}\)
Question 5. \(\sin \left(\tan ^{-1} e^{-x}\right)\)
Solution:
Let y=\(\sin \left(\tan ^{-1} e^{-x}\right)\)(By using chain rule, we obtain)
⇒ \(\frac{d y}{d x} =\frac{d}{d x}\left[\sin \left(\tan ^{-1} e^{-1}\right)\right]\)
= \(\cos \left(\tan ^{-1} e^{-x}\right) \cdot \frac{d}{d x}\left(\tan ^{-1} e^{-1}\right)\)
= \(\cos \left(\tan ^{-1} e^{-1}\right) \frac{1}{1+\left(e^{-x}\right)} \cdot \frac{d}{d x}\left(e^{-x}\right)\)
= \(\frac{\cos \left(\tan ^{-1} e^{-1}\right)}{1+e^{-2 x}} \cdot e^{-x} \cdot \frac{d}{d x}(-x)\)
= \(\frac{e^{-1} \cos \left(\tan ^{-1} e^{-1}\right)}{1+e^{-3 x}} \times(-1)=\frac{-e^{-1} \cos \left(\tan ^{-1} e^{-x}\right)}{1+e^{-3 x}}\)
Question 6. \(e^x+e^{x^2}+\ldots .+e^{x^2}\)
Solution:
⇒ \(\frac{d}{d x}\left(e^x+e^{x^2}+\ldots .+e^{x^1}\right)\)
= \(\frac{d}{d x}\left(e^x\right)+\frac{d}{d x}\left(e^{x^2}\right)+\frac{d}{d x}\left(e^{x^3}\right)+\frac{d}{d x}\left(e^{x^4}\right)+\frac{d}{d x}\left(e^{x^2}\right)\)
=\(e^x+\left[e^{x^2} \times \frac{d}{d x}\left(x^2\right)\right]+\left[e^{x^1} \cdot \frac{d}{d x}\left(x^3\right)\right]+\left[e^{x^4} \cdot \frac{d}{d x}\left(x^4\right)\right]+\left[e^{x^3} \cdot \frac{d}{d x}\left(x^5\right)\right]\)
= \(e^x+\left(e^{x^1} \times 2 x\right)+\left(e^{x^3} \times 3 x^2\right)+\left(e^{x^4} \times 4 x^3\right)+\left(e^{x^3} \times 5 x^4\right)\)
=\(e^x+2 e^{x^1}+3 x^2 e^{x^1}+4 x^3 e^{x^1}+5 x^4 e^{x^5}\)
Question 7. \(\sqrt{e^{\sqrt{x}}}, x>0\)
Solution:
Let y=\(\sqrt{e^{\sqrt{x}}}\)
Then, \(y^2=e^{\sqrt{x}}\) (By differentiating this relationship with respect to x, we obtain)
2 y \(\frac{d y}{d x}=e^{\sqrt{x}} \frac{d}{d x}(\sqrt{x})\) [By applying chain rule]
2 y \(\frac{d y}{d x}=e^{\sqrt{x}} \frac{1}{2} \cdot \frac{1}{\sqrt{x}}\)
⇒ \(\frac{d y}{d x}\)
= \(\frac{e^{\sqrt{x}}}{4 y \sqrt{x}}\)
⇒ \(\frac{d y}{d x}=\frac{e^{\sqrt{x}}}{4 \sqrt{e^{\sqrt{x}}} \sqrt{x}}\)
⇒ \(\frac{d y}{d x}=\frac{e^{\sqrt{x}}}{4 \sqrt{x e^{\sqrt{x}}}}\), x>0
Question 8. \(\log (\log x)\), x>1
Solution:
Let y =\(\log (\log \mathrm{x})\) (By using chain rule, we obtain)
⇒ \(\frac{d y}{d x}=\frac{d}{d x}[\log (\log x)]=\frac{1}{\log x} \cdot \frac{d}{d x}(\log x)\)
=\(\frac{1}{\log x} \cdot \frac{1}{x}=\frac{1}{x \log x}, x>1\)
Question 9. \(\frac{\cos x}{\log x}\), x>0
Solution:
Let y=\(\frac{\cos x}{\log x}\)
By using the quotient rule, we obtain
⇒ \(\frac{d y}{d x}=\frac{\log x \frac{d}{d x}(\cos x)-\cos x \times \frac{d}{d x}(\log x)}{(\log x)^2}\)
=\(\frac{-\sin x \log x-\cos x \times \frac{1}{x}}{(\log x)^2}\)
=\(\frac{-[x \log x \cdot \sin x+\cos x]}{x(\log x)^2}\), x>0
Question 10. \(\cos \left(\log x+e^x\right)\), x>0
Solution:
Let y=\(\cos \left(\log x+e^x\right)\) (By using chain rule, we obtain)
⇒ \(\frac{d y}{d x} =\frac{d}{d x}\left\{\cos \left(\log x+e^x\right)\right\}\)
⇒ \(\frac{d y}{d x} =-\sin \left(\log x+e^x\right) \cdot \frac{d}{d x}\left(\log x+e^x\right)\)
=-\(\sin \left(\log x+e^x\right) \cdot\left(\frac{1}{x}+e^x\right)\)
=-\(\left(\frac{1}{x}+e^x\right) \sin \left(\log x+e^x\right)\), x>0
Continuity And Differentiability Exercise 5.5
Question 1. \(\cos \mathrm{x} \cdot \cos 2 \mathrm{x} \cdot \cos 3 \mathrm{x}\)
Solution:
Let y=\(\cos \mathrm{x} \cdot \cos 2 \mathrm{x} \cdot \cos 3 \mathrm{x}\)
Taking logarithm on both the sides \(\log y=\log (\cos x \cdot \cos 2 x \cdot \cos 3 x)\)
⇒ \(\log y=\log (\cos x)+\log (\cos 2 x)+\log (\cos 3 x)\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{y d x}=\frac{1}{\cos x} \cdot \frac{d}{d x}(\cos x)+\frac{1}{\cos 2 x} \cdot \frac{d}{d x}(\cos 2 x)+\frac{1}{\cos 3 x} \cdot \frac{d}{d x}(\cos 3 x)\)
⇒ \(\frac{d y}{d x}=y\left[-\frac{\sin x}{\cos x}-\frac{\sin 2 x}{\cos 2 x} \cdot \frac{d}{d x}(2 x)-\frac{\sin 3 x}{\cos 3 x} \cdot \frac{d}{d x}(3 x)\right]\)
⇒ \(\frac{d y}{d x}=-\cos x \cdot \cos 2 x \cdot \cos 3 x[\tan x+2 \tan 2 x+3 \tan 3 x]\)
Question 2. \(\sqrt{\frac{(x-1)(x-2)}{(x-3)(x-4)(x-5)}}\)
Solution:
Let y=\(\sqrt{\frac{(x-1)(x-2)}{(x-3)(x-4)(x-5)}}\)
Taking logarithm on both the sides \(\log y=\log \sqrt{\frac{(x-1)(x-2)}{(x-3)(x-4)(x-5)}}\)
⇒ \(\log y=\frac{1}{2} \log \left[\frac{(x-1)(x-2)}{(x-3)(x-4)(x-5)}\right]\)
⇒ \(\log y=\frac{1}{2}[\log \{(x-1)(x-2)\}-\log \{(x-3)(x-4)(x-5)\}]\)
⇒ \(\log y=\frac{1}{2}[\log (x-1)+\log (x-2)-\log (x-3)-\log (x-4)-\log (x-5)]\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{y} \frac{d y}{d x}=\frac{1}{2}\left[\begin{array}{l}
\frac{1}{x-1} \cdot \frac{d}{d x}(x-1)+\frac{1}{x-2} \cdot \frac{d}{d x}(x-2)-\frac{1}{x-3} \cdot \frac{d}{d x}(x-3) \\
-\frac{1}{x-4} \cdot \frac{d}{d x}(x-4)-\frac{1}{x-5} \cdot \frac{d}{d x}(x-5)
\end{array}\right]\)
⇒ \(\frac{d y}{d x}=\frac{y}{2}\left(\frac{1}{x-1}+\frac{1}{x-2}-\frac{1}{x-3}-\frac{1}{x-4}-\frac{1}{x-5}\right)\)
⇒ \(\frac{d y}{d x}=\frac{1}{2} \sqrt{(x-1)(x-2)}\left[\frac{1}{(x-3)(x-4)(x-5)}+\frac{1}{x-2}-\frac{1}{x-3}-\frac{1}{x-4}-\frac{1}{x-5}\right]\)
Question 3. \((\log x)^{\cos x}\)
Solution:
Let y=\((\log x)^{\cos x}\)
Taking logarithm on both the sides \(\log y=\cos x \cdot \log (\log x)\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{y} \cdot \frac{d y}{d x}=\frac{d}{d x}(\cos x) \times \log (\log x)+\cos x \times \frac{d}{d x}[\log (\log x)]\)
⇒ \(\frac{1}{y} \cdot \frac{d y}{d x}=-\sin x \log (\log x)+\cos x \times \frac{1}{\log x} \cdot \frac{d}{d x}(\log x)\)
⇒ \(\frac{d y}{d x}=y\left[-\sin x \log (\log x)+\frac{\cos x}{\log x} \times \frac{1}{x}\right]\)
∴ \(\frac{d y}{d x}=(\log x)^{\cos x}\left[\frac{\cos x}{x \log x}-\sin x \log (\log x)\right]\)
Question 4. \(x^x-2^{\sin x}\)
Solution:
Let y=\(x^x-2^{\sin x}\)
Also, let \(\mathrm{x}^{\mathrm{x}}=\mathrm{u} and 2^{\sin \mathrm{x}}=\mathrm{v}\)
y=\(\mathrm{u}-\mathrm{v}\)
⇒ \(\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\mathrm{du}}{\mathrm{dx}}-\frac{\mathrm{dv}}{\mathrm{dx}}\) → Equation 1
⇒ \(\mathrm{u}=\mathrm{x}^{\mathrm{x}}\)
Taking logarithm on both sides log u = x log x
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{\mathrm{u}} \frac{\mathrm{du}}{\mathrm{dx}}=\left[\frac{\mathrm{d}}{\mathrm{dx}}(\mathrm{x}) \times \log \mathrm{x}+\mathrm{x} \times \frac{\mathrm{d}}{\mathrm{dx}}(\log x)\right]\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=\mathrm{u}\left[1 \times \log \mathrm{x}+\mathrm{x} \times \frac{1}{\mathrm{x}}\right]\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=\mathrm{x}^{\mathrm{x}}(1+\log \mathrm{x})\) → Equation 2
⇒ \(\mathrm{v}=2^{\sin x}\)
⇒ \(\frac{1}{\mathrm{u} d \mathrm{du}}=\left[\frac{\mathrm{d}}{\mathrm{dx}}(\mathrm{x}) \times \log \mathrm{x}+\mathrm{x} \times \frac{\mathrm{d}}{\mathrm{dx}}(\log x)\right]\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=\mathrm{u}\left[1 \times \log \mathrm{x}+\mathrm{x} \times \frac{1}{\mathrm{x}}\right]\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=\mathrm{x}^{\mathrm{x}}(1+\log \mathrm{x})\)
⇒ \(\mathrm{v}=2^{\sin x}\)
Taking logarithm on both the sides \(\log v=\sin x \cdot \log 2\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{v} \cdot \frac{d v}{d x}=\log 2 \cdot \frac{d}{d x}(\sin x)\)
⇒ \(\frac{d v}{d x}=v(\log 2 \cos x) \Rightarrow \frac{d v}{d x}=2^{\sin x}(\cos x \log 2)\) Equation 3
Therefore, from equations (1), (2) and (3), we obtain
⇒ \(\frac{\mathrm{dy}}{\mathrm{dx}}=\mathrm{x}^{\mathrm{x}}(1+\log \mathrm{x})-2^{\sin \mathrm{x}} \cos \mathrm{x} \log 2\)
Question 5. \((x+3)^2 \cdot(x+4)^3 \cdot(x+5)^4\)
Solution:
Let \(y=(x+3)^2 \cdot(x+4)^3 \cdot(x+5)^4\)
Taking logarithms on both sides
⇒ \(\log y=\log (x+3)^2+\log (x+4)^3+\log (x+5)^4\)
∴ \(\log y=2 \log (x+3)+3 \log (x+4)+4 \log (x+5)\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{y} \cdot \frac{d y}{d x}=2 \cdot \frac{1}{x+3} \cdot \frac{d}{d x}(x+3)+3 \cdot \frac{1}{x+4} \cdot \frac{d}{d x}(x+4)+4 \cdot \frac{1}{x+5} \cdot \frac{d}{d x}(x+5)\)
⇒ \(\frac{d y}{d x}=y\left[\frac{2}{x+3}+\frac{3}{x+4}+\frac{4}{x+5}\right]\)
⇒ \(\frac{d y}{d x}=(x+3)^2(x+4)^3(x+5)^4 \cdot\left[\frac{2}{x+3}+\frac{3}{x+4}+\frac{4}{x+5}\right]\)
⇒ \(\frac{d y}{d x}=(x+3)^2(x+4)^3(x+5)^4 \cdot\left[\frac{2(x+4)(x+5)+3(x+3)(x+5)+4(x+3)(x+4)}{(x+3)(x+4)(x+5)}\right]\)
⇒ \(\frac{d y}{d x}=(x+3)(x+4)^2(x+5)^3 \cdot\left[2\left(x^2+9 x+20\right)+3\left(x^2+8 x+15\right)+4\left(x^2+7 x+12\right)\right]\)
⇒ \(\frac{d y}{d x}=(x+3)(x+4)^2(x+5)^3\left(9 x^2+70 x+133\right)\)
∴ \(\left.\left(x+\frac{1}{x}\right)^x+x^x+\frac{1}{x}\right)\)
Question 6. \(\left(x+\frac{1}{x}\right)^x+x^{\left(1+\frac{1}{x}\right)}\)
Solution:
Let y=\(\left(x+\frac{1}{x}\right)^x+x^{\left(1+\frac{1}{x}\right)}\)
Also, let \(mathrm{u}=\left(\mathrm{x}+\frac{1}{\mathrm{x}}\right)^{\mathrm{x}} and \mathrm{v}=\mathrm{x}^{\left(1+\frac{1}{\mathrm{x}}\right)}\)
y=u+v
⇒ \(\frac{d y}{d x} =\frac{d u}{d x}+\frac{d v}{d x}\)
u =\(\left(x+\frac{1}{x}\right)^x\)
Taking logarithms on both sides
⇒ \(\log u=\log \left(x+\frac{1}{x}\right)^x \Rightarrow \log u=x \log \left(x+\frac{1}{x}\right)\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{u} \cdot \frac{d u}{d x}=\frac{d}{d x}(x) \times \log \left(x+\frac{1}{x}\right)+x \times \frac{d}{d x}\left[\log \left(x+\frac{1}{x}\right)\right]\)
⇒ \(\frac{1}{u} \frac{d u}{d x}=1 \times \log \left(x+\frac{1}{x}\right)+x \times \frac{1}{\left(x+\frac{1}{x}\right)} \cdot \frac{d}{d x}\left(x+\frac{1}{x}\right)\)
⇒ \(\frac{d u}{d x}=u\left[\log \left(x+\frac{1}{x}\right)+\frac{x}{\left(x+\frac{1}{x}\right)} \times\left(1-\frac{1}{x^2}\right)\right]\)
⇒ \(\frac{d u}{d x}=\left(x+\frac{1}{x}\right)^x\left[\log \left(x+\frac{1}{x}\right)+\frac{\left(x-\frac{1}{x}\right)}{\left(x+\frac{1}{x}\right)}\right]\)
⇒ \(\frac{d u}{d x}=\left(x+\frac{1}{x}\right)^x\left[\log \left(x+\frac{1}{x}\right)+\frac{x^2-1}{x^2+1}\right]\)
⇒ \(\frac{d u}{d x}=\left(x+\frac{1}{x}\right)^x\left[\frac{x^2-1}{x^2+1}+\log \left(x+\frac{1}{x}\right)\right]\)
⇒ \(x^{\left(1+\frac{1}{x}\right)}\)
Taking logarithms on both the sides \(\log v=\log \left[x^{\left(1+\frac{1}{x}\right)}\right]\)
⇒ \(\log v=\left(1+\frac{1}{x}\right) \log x\) Differentiating both the sides with respect to x, we obtain
⇒ \(\frac{1}{v} \cdot \frac{d v}{d x}=\left[\frac{d}{d x}\left(1+\frac{1}{x}\right)\right] \times \log x+\left(1+\frac{1}{x}\right) \cdot \frac{d}{d x}(\log x)\)
⇒ \(\frac{1}{v} \frac{d v}{d x}=\left(-\frac{1}{x^2}\right) \log x+\left(1+\frac{1}{x}\right) \cdot \frac{1}{x} \Rightarrow \frac{1}{v} \frac{d v}{d x}=-\frac{\log x}{x^2}+\frac{1}{x}+\frac{1}{x^2}\)
⇒ \(\frac{d v}{d x}=v\left[\frac{-\log x+x+1}{x^2}\right] \Rightarrow \frac{d v}{d x}=x^{\left(1+\frac{1}{v}\right)}\left(\frac{x+1-\log x}{x^2}\right)\)
Therefore, from (1), (2), and (3), we obtain
∴ \(\frac{d y}{d x}=\left(x+\frac{1}{x}\right)^x\left[\frac{x^2-1}{x^2+1}+\log \left(x+\frac{1}{x}\right)\right]+x^{\left(1+\frac{1}{x}\right)}\left(\frac{x+1-\log x}{x^2}\right)\)
Question 7. \((\log x)^x+x^{\log x}\)
Solution:
Let y=\((\log x)^x+x^{\log x}\)
Also, let u=\((\log x)^x and v=x^{\log x}\)
y=u+v
⇒ \(\frac{d y}{d x}=\frac{d u}{d x}+\frac{d v}{d x}\)
u=\((\log x)^x\)
Taking logarithm on both the sides \(\log u=\log \left[(\log x)^x\right]\)
⇒ \(\log u=x \log (\log x)\) Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{u} \frac{d u}{d x}=\frac{d}{d x}(x) \times \log (\log x)+x \cdot \frac{d}{d x}[\log (\log x)]\)
⇒ \(\frac{d u}{d x}=u\left[1 \times \log (\log x)+x \cdot \frac{1}{\log x} \cdot \frac{d}{d x}(\log x)\right]\)
⇒ \(\frac{d u}{d x}=(\log x)^x\left[\log (\log x)+\frac{x}{\log x} \cdot \frac{1}{x}\right]=(\log x)^x\left[\log (\log x)+\frac{1}{\log x}\right]\)
⇒ \(\frac{d u}{d x}=(\log x)^x\left[\frac{\log (\log x) \cdot \log x+1}{\log x}\right]=(\log x)^{x-1}[1+\log x \cdot \log (\log x)]\)
v=\(x^{\log x}\)
Taking logarithm on both the sides \(\log v=\log \left(x^{\log x}\right)\)
⇒ \(\log v=\log x \log x=(\log x)^2\)
Differentiating both sides w.r.t.x, we obtain
⇒ \(\frac{1}{v} \cdot \frac{d v}{d x}=\frac{d}{d x}\left[(\log x)^2\right]\)
⇒ \(\frac{1}{v} \cdot \frac{d v}{d x}=2(\log x) \cdot \frac{d}{d x}(\log x) \Rightarrow \frac{d v}{d x}=2 v(\log x) \cdot \frac{1}{x}\)
⇒ \(\frac{d v}{d x}=2 x^{\log x} \frac{\log x}{x} \Rightarrow \frac{d v}{d x}=2 x^{\log x-1} \cdot \log x\)
Therefore, from (1), (2), and (3), we obtain
∴ \(\frac{d y}{d x}=(\log x)^{x-1}[1+\log x \cdot \log (\log x)]+2 x^{\log x-1} \cdot \log x\)
Question 8. \((\sin x)^x+\sin ^{-1} \sqrt{x}\)
Solution:
Let y=\((\sin x)^x+\sin ^{-1} \sqrt{x}\)
⇒Also, let u=\((\sin x)^x and v=\sin ^{-1} \sqrt{x}\)
\(\mathrm{y}=\mathrm{u}+\mathrm{v}\)
⇒ \(\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\mathrm{du}}{\mathrm{dx}}+\frac{\mathrm{dv}}{\mathrm{dx}}\) → Equation 1
⇒ \(\mathrm{u}=(\sin \mathrm{x})^{\mathrm{x}}\)
Taking logarithm on both the sides \(\log \mathrm{u}=\log (\sin \mathrm{x})^{\mathrm{x}} \Rightarrow \log \mathrm{u}=\mathrm{x} \log (\sin \mathrm{x})\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{u} \frac{d u}{d x}=\frac{d}{d x}(x) \times \log (\sin x)+x \times \frac{d}{d x}[\log (\sin x)]\)
⇒ \(\frac{d u}{d x}=u\left[1 \cdot \log (\sin x)+x \cdot \frac{1}{\sin x} \cdot \frac{d}{d x}(\sin x)\right]\)
⇒ \(\frac{d u}{d x}=(\sin x)^x\left[\log (\sin x)+\frac{x}{\sin x} \cdot \cos x\right]=(\sin x)^x(x \cot x+\log \sin x)\) → Equation 2
v=\(\sin ^{-1} \sqrt{x}\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{\mathrm{dv}}{\mathrm{dx}}=\frac{1}{\sqrt{1-(\sqrt{\mathrm{x}})^2}} \cdot \frac{\mathrm{d}}{\mathrm{dx}}(\sqrt{\mathrm{x}})\)
=\(\frac{1}{\sqrt{1-\mathrm{x}}} \cdot \frac{1}{2 \sqrt{\mathrm{x}}}=\frac{1}{2 \sqrt{\mathrm{x}-\mathrm{x}^2}}\) ⇒ Equation 3
Therefore, from (1), (2), and (3), we obtain \(\frac{d y}{d x}=(\sin x)^x(x \cot x+\log \sin x)+\frac{1}{2 \sqrt{x-x^2}}\)
Question 9. \(x^{\sin x}+(\sin x)^{\cos x}\)
Solution:
Let y=\(x^{\sin x}+(\sin x)^{\cos x}\)
Also, let \(\mathrm{u}=\mathrm{x}^{\sin \mathrm{x}}\) and \(\mathrm{v}=(\sin \mathrm{x})^{\cos \mathrm{x}}\)
⇒ \(\mathrm{y}=\mathrm{u}+\mathrm{v}\)
⇒ \(\frac{d y}{d x}=\frac{d u}{d x}+\frac{d v}{d x}\) → Equation 1
⇒ \(u=x^{\sin x}\)
Taking logarithm on both the sides \(\log \mathrm{u}=\log \left(\mathrm{x}^{\sin \mathrm{x}}\right)\)
⇒ \(\log \mathrm{u}=\sin \mathrm{x} \log \mathrm{x}\) Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{\mathrm{u} d \mathrm{du}}=\frac{\mathrm{d}}{\mathrm{dx}}(\sin \mathrm{x}) \cdot \log \mathrm{x}+\sin x \cdot \frac{\mathrm{d}}{\mathrm{dx}}(\log x)\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=\mathrm{u}\left[\cos x \log x+\sin x \cdot \frac{1}{x}\right]\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=x^{\sin x}\left[\cos x \log x+\frac{\sin x}{x}\right]\) . Equation 2
⇒ \(\mathrm{v}=(\sin x)^{\cos x}\)
Taking logarithm on both the sides \(\log \mathrm{v}=\log (\sin x)^{\cos x} \log \mathrm{y}=\cos x \log (\sin x)\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{v d v}=\frac{d}{d x}(\cos x) \times \log (\sin x)+\cos x \times \frac{d}{d x}[\log (\sin x)]\)
⇒ \(\frac{d v}{d x}=v\left[-\sin x \cdot \log (\sin x)+\cos x \cdot \frac{1}{\sin x} \cdot \frac{d}{d x}(\sin x)\right]\)
⇒ \(\frac{d v}{d x}=(\sin x)^{\cos x}\left[-\sin x \log \sin x+\frac{\cos x}{\sin x} \cos x\right]=(\sin x)^{\cos x}[-\sin x \log \sin x+\cot x \cos x]\) → Equation 3
⇒ \(\frac{d v}{d x}=(\sin x)^{\cos x}[\cot x \cos x-\sin x \log \sin x]]\)
From (1), (2), and (3), we obtain
∴ \(\frac{d y}{d x}=x^{\sin x}\left(\cos x \log x+\frac{\sin x}{x}\right)+(\sin x)^{\cos x}[\cos x \cot x-\sin x \log \sin x]\)
Question 10. \(x^{x \cos x}+\frac{x^2+1}{x^2-1}\)
Solution:
Let y=\(x^{x \cos x}+\frac{x^2+1}{x^2-1}\)
Also, let \(\mathrm{u}=\mathrm{x}^{\mathrm{x} \cos \mathrm{x}} and \mathrm{v}=\frac{\mathrm{x}^2+1}{\mathrm{x}^2-1}\) → Equation 1
⇒ \(\mathrm{y}=\mathrm{u}+\mathrm{v}\)
⇒ \(\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\mathrm{du}}{\mathrm{dx}}+\frac{\mathrm{dv}}{\mathrm{dx}}\)
⇒ \(\mathrm{u}=\mathrm{x}^{\mathrm{xec} x}\)
Taking logarithm on both the sides \(\log \mathrm{u}=\log \left(\mathrm{x}^{\mathrm{x} {cosx}}\right)\)
⇒ \(\log \mathrm{u}=\mathrm{x} \cos \mathrm{x} \log \mathrm{x} \)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{u} \frac{d u}{d x}=\frac{d}{d x}(x) \cdot \cos x \cdot \log x+x \cdot \frac{d}{d x}(\cos x) \cdot \log x+x \cos x \cdot \frac{d}{d x}(\log x)\)
⇒ \(\frac{d u}{d x}=u\left[1 \cdot \cos x \cdot \log x+x \cdot(-\sin x) \log x+x \cos x \cdot \frac{1}{x}\right]\)
⇒ \(\frac{d u}{d x}=x^{x \cos x}(\cos x \log x-x \sin x \log x+\cos x)\) → Equation 2
⇒ \(\frac{d u}{d x}=x^{x \cos x}[\cos x(1+\log x)-x \sin x \log x]\)
v =\(\frac{x^2+1}{x^2-1}\)
Taking logarithm on both the sides \(\log v=\log \left(x^2+1\right)-\log \left(x^2-1\right)\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{v} \frac{d v}{d x}=\frac{2 x}{x^2+1}-\frac{2 x}{x^2-1}\)
⇒ \(\frac{d v}{d x}=v\left[\frac{2 x\left(x^2-1\right)-2 x\left(x^2+1\right)}{\left(x^2+1\right)\left(x^2-1\right)}\right]\)
⇒ \(\frac{d v}{d x}=\frac{x^2+1}{x^2-1} \times\left[\frac{-4 x}{\left(x^2+1\right)\left(x^2-1\right)}\right]\)
⇒ \(\frac{d v}{d x}=\frac{-4 x}{\left(x^2-1\right)^2}\)
Therefore, from (1), (2), and (3), we obtain
⇒ \(\frac{d y}{d x}=x^{x \cos x}[\cos x(1+\log x)-x \sin x \log x]-\frac{4 x}{\left(x^2-1\right)^2}\)
Question 11. \((x \cos x)^x+(x \sin x)^{\frac{1}{x}}\)
Solution:
Let y=\((x \cos x)^x+(x \sin x)^{\frac{1}{x}}\)
Also, let u=\((x \cos x)^x and v=(x \sin x)^{\frac{1}{x}}\)
⇒ \(\mathrm{y}=\mathrm{u}+\mathrm{v}\)
⇒ \(\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\mathrm{du}}{\mathrm{dx}}+\frac{\mathrm{dv}}{\mathrm{dx}}\)
⇒ \(\mathrm{u}=(\mathrm{x} \cos \mathrm{x})^{\mathrm{x}}\)(Differentiating both sides w.r.t. x, we obtain)
Taking logarithms on both sides
⇒ \(\log u =\log (x \cos x)^x\)
⇒ \(\log u =x \log (x \cos x) \Rightarrow \log u=x[\log x+\log \cos x] \log u=x \log x+x \log \cos x\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{\mathrm{u}}\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=\frac{\mathrm{d}}{\mathrm{dx}}(\mathrm{x} \log \mathrm{x})+\frac{\mathrm{d}}{\mathrm{dx}}(\mathrm{x} \log \cos \mathrm{x})\) .
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=\mathrm{u}\left[\left\{\log \mathrm{x} \cdot \frac{\mathrm{d}}{\mathrm{dx}}(\mathrm{x})+\mathrm{x} \cdot \frac{\mathrm{d}}{\mathrm{dx}}(\log \mathrm{x})\right\}+\left\{\log \cos \mathrm{x} \cdot \frac{\mathrm{d}}{\mathrm{dx}}(\mathrm{x})+\mathrm{x} \cdot \frac{\mathrm{d}}{\mathrm{dx}}(\log \cos \mathrm{x})\right\}\right]\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=(\mathrm{x} \cos \mathrm{x})^{\mathrm{x}}\left[\left(\log \mathrm{x} \cdot 1+\mathrm{x} \cdot \frac{1}{\mathrm{x}}\right)+\left\{\log \cos \mathrm{x} \cdot 1+\mathrm{x} \cdot \frac{1}{\cos \mathrm{x}} \cdot \frac{\mathrm{d}}{\mathrm{dx}}(\cos \mathrm{x})\right\}\right]\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=(\mathrm{x} \cos \mathrm{x})^{\mathrm{x}}\left[(\log \mathrm{x}+1)+\left\{\log \cos \mathrm{x}+\frac{\mathrm{x}}{\cos \mathrm{x}} \cdot(-\sin \mathrm{x})\right\}\right]\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=(\mathrm{x} \cos \mathrm{x})^{\mathrm{x}}[(1+\log \mathrm{x})+(\log \cos \mathrm{x}-\mathrm{x} \tan \mathrm{x})]\)
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}=(\mathrm{x} \cos \mathrm{x})^{\mathrm{x}}[1-\mathrm{x} \tan \mathrm{x}+(\log \mathrm{x}+\log \cos \mathrm{x})]\)
⇒ \(\frac{d u}{d x}=(x \cos x)^x[1-x \tan x+\log (x \cos x)]\)
⇒ \(\mathrm{v}=(\mathrm{x} \sin \mathrm{x})^{\frac{1}{\mathrm{x}}}\)
Taking logarithms on both sides
⇒ \(\log \mathrm{v}=\log (\mathrm{x} \sin \mathrm{x})^{\frac{1}{\mathrm{x}}}\)
⇒ \(\log \mathrm{v}=\frac{1}{\mathrm{x}} \log (\mathrm{x} \sin \mathrm{x}) \Rightarrow \log \mathrm{v}\)
=\(\frac{1}{\mathrm{x}}(\log \mathrm{x}+\log \sin \mathrm{x})\)
⇒ \(\log \mathrm{v}=\frac{1}{\mathrm{x}} \log \mathrm{x}+\frac{1}{\mathrm{x}} \log \sin \mathrm{x}\)
Differentiating both sides with respect to x
⇒ \(\frac{1}{v} \frac{d v}{d x}=\frac{d}{d x}\left(\frac{1}{x} \log x\right)+\frac{d}{d x}\left[\frac{1}{x} \log (\sin x)\right]\)
⇒ \(\frac{1}{v} \frac{d v}{d x}=\left[\log x \cdot \frac{d}{d x}\left(\frac{1}{x}\right)+\frac{1}{x} \cdot \frac{d}{d x}(\log x)\right]+\left[\log (\sin x) \cdot \frac{d}{d x}\left(\frac{1}{x}\right)+\frac{1}{x} \cdot \frac{d}{d x}\{\log (\sin x)\}\right]\)
⇒ \(\frac{1}{v} \frac{d v}{d x}=\left[\log x \cdot\left(-\frac{1}{x^2}\right)+\frac{1}{x} \cdot \frac{1}{x}\right]+\left[\log (\sin x) \cdot\left(-\frac{1}{x^2}\right)+\frac{1}{x} \cdot \frac{1}{\sin x} \cdot \frac{d}{d x}(\sin x)\right]\)
⇒ \(\frac{1}{v} \frac{d v}{d x}=\frac{1}{x^2}(1-\log x)+\left[-\frac{\log (\sin x)}{x^2}+\frac{1}{x \sin x} \cdot \cos x\right]\)
⇒ \(\frac{d v}{d x}=(x \sin x)^{\frac{1}{x}}\left[\frac{1-\log x}{x^2}+\frac{-\log (\sin x)+x \cot x}{x^2}\right]\)
⇒ \(\frac{d v}{d x}=(x \sin x)^{\frac{1}{x}}\left[\frac{1-\log x-\log (\sin x)+x \cot x}{x^2}\right]\)
⇒ \(\frac{d v}{d x}=(x \sin x)^{\frac{1}{x}}\left[\frac{1-\log (x \sin x)+x \cot x}{x^2}\right]\)
From (1), (2), and (3), we obtain
∴ \(\frac{d y}{d x}=(x \cos x)^x[1-x \tan x+\log (x \cos x)]+(x \sin x)^{\frac{1}{x}}\left[\frac{x \cot x+1-\log (x \sin x)}{x^2}\right]\)
Question 12. \(x^y+y^x=1\)
Solution:
The given function is \(\mathrm{x}^y+\mathrm{y}^{\mathrm{x}}\)=1.
Let \(\mathrm{x}^{\mathrm{y}}=\mathrm{u} and\mathrm{y}^{\mathrm{x}}=\mathrm{v}\)
Then, the function becomes \mathrm{u}+\mathrm{v}=1
⇒ \(\frac{\mathrm{du}}{\mathrm{dx}}+\frac{\mathrm{dv}}{\mathrm{dx}}\)=0
⇒ \(\mathrm{u} =\mathrm{x}^{\mathrm{y}}\)
Taking logarithm on both the sides \(\log u=\log \left(x^y\right) \Rightarrow \log u=y \log x \)
Differentiating both sides with respect to x, we obtain
⇒ \(\mathrm{v}=\mathrm{y}^{\mathrm{x}}\)
⇒ \(\mathrm{v}=\mathrm{y}^{\mathrm{x}}\)
Taking logarithm on both the sides \(\log v=\log \left(y^x\right) \Rightarrow \log v=x \log y\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1 \mathrm{du}}{\mathrm{u} x}=\log x \frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{y} \cdot \frac{\mathrm{d}}{\mathrm{dx}}(\log \mathrm{x}) \Rightarrow \frac{\mathrm{du}}{\mathrm{dx}}\)
= \(\mathrm{u}\left[\log \mathrm{x} \frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{y} \cdot \frac{1}{\mathrm{x}}\right] \Rightarrow \frac{\mathrm{du}}{\mathrm{dx}}\)
= \(\mathrm{x}^y\left(\log \mathrm{x} \frac{\mathrm{dy}}{\mathrm{dx}}+\frac{\mathrm{y}}{\mathrm{x}}\right)\)
⇒ \(\mathrm{v}=\mathrm{y}^{\mathrm{x}}\)
Taking logarithm on both the sides \(\log v=\log \left(y^x\right) \Rightarrow \log v=x \log y\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{v} \cdot \frac{d v}{d x}=\log y \cdot \frac{d}{d x}(x)+x \cdot \frac{d}{d x}(\log y)\)
⇒ \(\frac{d v}{d x}=v\left(\log y \cdot 1+x \cdot \frac{1}{y} \cdot \frac{d y}{d x}\right)\)
⇒ \(\frac{d v}{d x}=y^x\left(\log y+\frac{x d y}{y}\right)\)
From (1), (2), and (3), we obtain
⇒ \(x^y\left(\log x \frac{d y}{d x}+\frac{y}{x}\right)+y^x\left(\log y+\frac{x d y}{y d x}\right)\)=0
⇒ \(\left(x^y \log x+x y^{x-1}\right) \frac{d y}{d x}=-\left(y x^{y-1}+y^x \log y\right)\)
∴ \(\frac{d y}{d x}=-\frac{y x^{y-1}+y^x \log y}{x^y \log x+x y^{x-1}}\)
Question 13. \(\mathrm{y}^{\mathrm{x}}=\mathrm{x}^y\)
Solution:
The given function is \(\mathrm{y}^{\mathrm{x}}=\mathrm{x}^y\)
Taking logarithm on both sides, we obtain \(\log y^\pi=\log x^5 \Rightarrow x \log y=y \log x\)
Differentiating both sides with respect to x, we obtain
⇒ \(\log y \cdot \frac{d}{d x}(x)+x \cdot \frac{d}{d x}(\log y)=\log x \cdot \frac{d}{d x}(y)+y \cdot \frac{d}{d x}(\log x)\)
⇒ \(\log y \cdot 1+x \cdot \frac{1}{y} \cdot \frac{d y}{d x}=\log x \cdot \frac{d y}{d x}+y \cdot \frac{1}{x}\)
⇒ \(\log y+\frac{x}{y} \frac{d y}{d x}=\log x \frac{d y}{d x}+\frac{y}{x}\)
⇒ \(\left(\frac{x}{y}-\log x\right) \frac{d y}{d x}=\frac{y}{x}-\log y \Rightarrow\left(\frac{x-y \log x}{y}\right) \frac{d y}{d x}=\frac{y-x \log y}{x}\)
∴ \(\frac{d y}{d x}=\frac{y}{x}\left(\frac{y-x \log y}{x-y \log x}\right)\)
Question 14. \((\cos x)^y=(\cos y)^x\)
Solution:
The given function is \((\cos x)^y=(\cos y)^x\)
Taking logarithms on both sides, we obtain
y \(\log \cos x=x \log \cos y\)
⇒ \(\log \cos x \cdot \frac{d y}{d x}+y \cdot \frac{d}{d x}(\log \cos x)=\log \cos y \cdot \frac{d}{d x}(x)+x \cdot \frac{d}{d x}(\log \cos y)\)
⇒ \(\log \cos x \frac{d y}{d x}+y \cdot \frac{1}{\cos x} \cdot \frac{d}{d x}(\cos x)=\log \cos y \cdot 1+x \cdot \frac{1}{\cos y} \cdot \frac{d}{d x}(\cos y)\)
⇒ \(\log \cos x \frac{d y}{d x}+\frac{y}{\cos x} \cdot(-\sin x)=\log \cos y+\frac{x}{\cos y}(-\sin y) \cdot \frac{d y}{d x}\)
⇒ \(\log \cos x \frac{d y}{d x}-y \tan x=\log \cos y-x \tan y \frac{d y}{d x}\)
⇒ \((\log \cos x+x \tan y) \frac{d y}{d x}=y \tan x+\log \cos y\)
∴ \(\frac{d y}{d x}=\frac{y \tan x+\log \cos y}{x \tan y+\log \cos x}\)
Question 15. \(x y=e^{(x-y)}\)
Solution:
The given function is x y=\(e^{(x-y)}\)
⇒ Taking logarithm on both the sides \(\log (x y)=\log \left(e^{x-y}\right)\)
⇒ \(\log x+\log y=(x-y) \log e \Rightarrow \log x+\log y=(x-y) \times 1\)
⇒ \(\log x+\log y=x-y\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{d}{d x}(\log x)+\frac{d}{d x}(\log y)=\frac{d}{d x}(x)-\frac{d y}{d x}\)
⇒ \(\frac{1}{x}+\frac{1}{y} \frac{d y}{d x}=1-\frac{d y}{d x}\)
⇒ \(\left(1+\frac{1}{y}\right) \frac{d y}{d x}=1-\frac{1}{x}\)
⇒ \(\left(\frac{y+1}{y}\right) \frac{d y}{d x}=\frac{x-1}{x}\)
∴ \(\frac{d y}{d x}=\frac{y(x-1)}{x(y+1)}\)
Equation 16. Find the derivative of the function given by \(f(x)=(1+x)\left(1+x^2\right)\left(1+x^4\right)\left(1+x^8\right)\) and hence find \(\mathrm{f}^{\prime}(1)\).
Solution:
The given relationship is \(f(x)=(1+x)\left(1+x^2\right)\left(1+x^4\right)\left(1+x^3\right)\)
Taking logarithms on both sides
⇒ \(\log f(x)=\log (1+x)+\log \left(1+x^2\right)+\log \left(1+x^4\right)+\log \left(1+x^x\right)\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{f(x)} \cdot \frac{d}{d x}[f(x)]=\frac{d}{d x} \log (1+x)+\frac{d}{d x} \log \left(1+x^2\right)+\frac{d}{d x} \log \left(1+x^4\right)+\frac{d}{d x} \log \left(1+x^8\right)\)
⇒ \(\frac{1}{f(x)} \cdot f^{\prime}(x)=\frac{1}{1+x} \cdot \frac{d}{d x}(1+x)+\frac{1}{1+x^2} \cdot \frac{d}{d x}\left(1+x^2\right)+\frac{1}{1+x^4} \cdot \frac{d}{d x}\left(1+x^4\right)+\frac{1}{1+x^8} \cdot \frac{d}{d x}\left(1+x^3\right)\)
⇒ \(f^{\prime}(x)=f(x)\left[\frac{1}{1+x}+\frac{1}{1+x^2} \cdot 2 x+\frac{1}{1+x^4} \cdot 4 x^3+\frac{1}{1+x^3} \cdot 8 x^7\right]\)
⇒ \(f^{\prime}(x)=(1+x)\left(1+x^2\right)\left(1+x^4\right)\left(1+x^8\right)\left[\frac{1}{1+x}+\frac{2 x}{1+x^7}+\frac{4 x^3}{1+x^4}+\frac{8 x^7}{1+x^5}\right]\)
Hence,\(f^{\prime}(1)=(1+1)\left(1+1^2\right)\left(1+1^4\right)\left(1+1^3\right)\left[\frac{1}{1+1}+\frac{2 \times 1}{1+1^2}+\frac{4 \times 1^3}{1+1^4}+\frac{8 \times 1^7}{1+1^1}\right]\)
=\(2 \times 2 \times 2 \times 2\left[\frac{1}{2}+\frac{2}{2}+\frac{4}{2}+\frac{8}{2}\right]=16 \times\left(\frac{1+2+4+8}{2}\right)=16 \times \frac{15}{2}=120\)
Question 17. If u, v and w are functions of x, then show that \(\frac{d}{d x}(u, v, w)=\frac{d u}{d x} v \cdot w+u, \frac{d v}{d x}, w+u, v, \frac{d w}{d x}\) in two ways-first by repeated application of product rule, second by logarithmic differentiation.
Solution:
Let y= u.v.w. =\(\mathrm{u} .(\mathrm{v} . \mathrm{w})\)
By applying the product rule, we obtain
⇒ \(\frac{d y}{d x}=\frac{d u}{d x} \cdot(v \cdot w)+u \cdot \frac{d}{d x}(v, w)\)
⇒ \(\frac{d y}{d x}=\frac{d u}{d x} v \cdot w+u\left[\frac{d v}{d x} \cdot w+v \cdot \frac{d w}{d x}\right]\) (Again applying product rule)
⇒ \(\frac{d y}{d x}=\frac{d u}{d x} \cdot v \cdot w+u \cdot \frac{d v}{d x} \cdot w+u \cdot v \cdot \frac{d w}{d x}\)
By taking logarithm on both sides of the equation y = u.v.w, we obtain
⇒ \(\log y=\log u+\log v+\log w\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{y} \cdot \frac{d y}{d x}=\frac{d}{d x}(\log u)+\frac{d}{d x}(\log v)+\frac{d}{d x}(\log w)\)
⇒ \(\frac{1}{y} \cdot \frac{d y}{d x}=\frac{1}{u} \frac{d u}{d x}+\frac{1}{v} \frac{d v}{d x}+\frac{1}{w} \frac{d w}{d x}\)
⇒ \(\frac{d y}{d x}=y\left(\frac{1}{u} \frac{d u}{d x}+\frac{1}{v} \frac{d v}{d x}+\frac{1}{w} \frac{d w}{d x}\right)\)
⇒ \(\frac{d y}{d x}=u \cdot v \cdot w \cdot\left(\frac{1}{u d x}+\frac{1 d v}{v d x}+\frac{1}{w} \frac{d w}{d x}\right)\)
∴ \(\frac{d y}{d x}=\frac{d u}{d x} \cdot v \cdot w+u \cdot \frac{d v}{d x} \cdot w+u \cdot v \cdot \frac{d w}{d x}\)
Continuity And Differentiability Exercise 5.6
Question 1. \(\mathrm{x}=2 \mathrm{at} \mathrm{t}^2, \mathrm{y}=\mathrm{at}^4\)
Solution:
The given equations are \(\mathrm{x}=2 \mathrm{at}^2\) and \(\mathrm{y}=a \mathrm{t}^4\)
Then, \(\frac{\mathrm{dx}}{\mathrm{dt}}=\frac{\mathrm{d}}{\mathrm{dt}}\left(2 \mathrm{at}^2\right)=2 \mathrm{a} \cdot \frac{\mathrm{d}}{\mathrm{dt}}\left(\mathrm{t}^2\right)\)
=\(2 \mathrm{a} \cdot 2 \mathrm{t}=4 \mathrm{at}\) and \(\frac{\mathrm{dy}}{\mathrm{dt}}=\frac{\mathrm{d}}{\mathrm{dt}}\left(\mathrm{at}^4\right)\)
=\(\mathrm{a} \cdot \frac{\mathrm{d}}{\mathrm{dt}}\left(\mathrm{t}^4\right)=\mathrm{a} \cdot 4 \cdot \mathrm{t}^3=4 \mathrm{at}^5\)
∴ \(\frac{d y}{d x}=\frac{\left(\frac{d y}{d t}\right)}{\left(\frac{d x}{d t}\right)}=\frac{4 a t^3}{4 a t}=t^2\)
Question 2. \(x=a \cos \theta, y=b \cos \theta\)
Solution:
The given equations are x=a \(\cos \theta and y=b \cos \theta\)
Then, \(\frac{d x}{d \theta}=\frac{d}{d \theta}(a \cos \theta)=a(-\sin \theta)=-a \sin \theta and \frac{d y}{d \theta}\)
=\(\frac{d}{d \theta}(b \cos \theta)=b(-\sin \theta)=-b \sin \theta\)
∴ \(\frac{d y}{d x}=\frac{\left(\frac{d y}{d \theta}\right)}{\left(\frac{d x}{d \theta}\right)}=\frac{-b \sin \theta}{-a \sin \theta}=\frac{b}{a}\)
Question 3. x=\(\sin t, y=\cos\) 2 t
Solution:
The given equations are x=\(\sin t and y=\cos\) 2 t
Then, \(\frac{\mathrm{dx}}{\mathrm{dt}}=\frac{\mathrm{d}}{\mathrm{dt}}(\sin \mathrm{t})=\cos \mathrm{t}\) and
⇒ \(\frac{\mathrm{dy}}{\mathrm{dt}}=\frac{\mathrm{d}}{\mathrm{dt}}(\cos 2 \mathrm{t})=-\sin 2 t \cdot \frac{\mathrm{d}}{\mathrm{dt}}(2 \mathrm{t})=-2 \sin 2 \mathrm{t}\)
∴ \(\frac{d y}{d x}=\frac{\left(\frac{d y}{d t}\right)}{\left(\frac{d x}{d t}\right)}=\frac{-2 \sin 2 t}{\cos t}=\frac{-2 \cdot 2 \sin t \cos t}{\cos t}=-4 \sin t\)
Question 4. x=4 t, y=\(\frac{4}{t}\)
Solution:
The given equations are x=4 t and y=\(\frac{4}{t}\)
⇒ \(\frac{d x}{d t}=\frac{d}{d t}(4 t)=4 and \frac{d y}{d t}=\frac{d}{d t}\left(\frac{4}{t}\right)\)
=\(4 \cdot \frac{d}{d t}\left(\frac{1}{t}\right)=4 \cdot\left(\frac{-1}{t^2}\right)=\frac{-4}{t^2}\)
∴ \(\frac{d y}{d x}=\frac{\left(\frac{d y}{d t}\right)}{\left(\frac{d x}{d t}\right)}=\frac{\left(\frac{-4}{t^2}\right)}{4}=\frac{-1}{t^2}\)
Question 5. x=\(\cos \theta-\cos 2 \theta, y=\sin \theta-\sin 2 \theta\)
Solution:
The given equations are x=\(\cos \theta-\cos 2 \theta and y=\sin \theta-\sin 2 \theta\)
Then,\(\frac{d x}{d \theta}=\frac{d}{d \theta}(\cos \theta-\cos 2 \theta)=\frac{d}{d \theta}(\cos \theta)-\frac{d}{d \theta}(\cos 2 \theta)\)
=-\(\sin \theta-(-2 \sin 2 \theta)=2 \sin 2 \theta-\sin \theta\)
and \(\frac{d y}{d \theta} =\frac{d}{d \theta}(\sin \theta-\sin 2 \theta)=\frac{d}{d \theta}(\sin \theta)-\frac{d}{d \theta}(\sin 2 \theta)\)
=\(\cos \theta-2 \cos 2 \theta\)
\(\frac{d y}{d x} =\frac{\left(\frac{d y}{d \theta}\right)}{\left(\frac{d x}{d \theta}\right)}\)
= \(\frac{\cos \theta-2 \cos 2 \theta}{2 \sin 2 \theta-\sin \theta}\)
Question 6. x=a\((\theta-\sin \theta), y=a(1+\cos \theta)\)
Solution:
The given equations are x=a\((\theta-\sin \theta) and y=a(1+\cos \theta)\)
Then, \(\frac{d x}{d \theta}=a\left[\frac{d}{d \theta}(\theta)-\frac{d}{d \theta}(\sin \theta)\right]=a(1-\cos \theta)\)
and \(\left.\frac{d y}{d \theta}=a\left[\frac{d}{d \theta}(1)+\frac{d}{d \theta}(\cos \theta)\right]=a[0+(-\sin \theta)]\right]=-a \sin \theta\)
⇒ \(\frac{d y}{d x}=\frac{\left(\frac{d y}{d \theta}\right.}{\left(\frac{d x}{d \theta}\right)}\)
=\(\frac{-a \sin \theta}{a(1-\cos \theta)}=\frac{-2 \sin \frac{\theta}{2} \cos \frac{\theta}{2}}{2 \sin ^2 \frac{\theta}{2}}\)
= \(\frac{-\cos \frac{\theta}{2}}{\sin \frac{\theta}{2}}=-\cot \frac{\theta}{2}\)
Question 7. x=\(\frac{\sin ^3 t}{\sqrt{\cos 2 t}}, y=\frac{\cos ^3 t}{\sqrt{\cos 2 t}}\)
Solution:
The given equations are x=\(\frac{\sin ^3 t}{\sqrt{\cos 2 t}} and y=\frac{\cos ^3 t}{\sqrt{\cos 2 t}}\)
Then,\(\frac{d x}{d t}=\frac{d}{d t}\left[\frac{\sin ^3 t}{\sqrt{\cos 2 t}}\right]\)
=\(\frac{\sqrt{\cos 2 t} \cdot \frac{d}{d t}\left(\sin ^3 t\right)-\sin ^3 t \cdot \frac{d}{d t} \sqrt{\cos 2 t}}{(\sqrt{\cos 2 t})^2}\)
=\(\frac{\sqrt{\cos 2 t} \cdot 3 \sin ^2 t \cdot \frac{d}{d t}(\sin t)-\sin ^3 t \times \frac{1}{2 \sqrt{\cos 2 t}} \cdot \frac{d}{d t}(\cos 2 t)}{\cos 2 t}\)
=\(\frac{3 \sqrt{\cos 2 t} \cdot \sin ^2 t \cos t-\frac{\sin ^3 t}{2 \sqrt{\cos 2 t}} \cdot(-2 \sin 2 t)}{\cos 2 t}\)
= \(\frac{3 \cos 2 t \sin ^2 t \cos t+\sin ^3 t \sin 2 t}{\cos ^2 t \sqrt{\cos 2 t}}\)
and \(\frac{d y}{d t} =\frac{d}{d t}\left[\frac{\cos ^3 t}{\sqrt{\cos 2 t}}\right]=\frac{\sqrt{\cos 2 t} \cdot \frac{d}{d t}\left(\cos ^3 t\right)-\cos ^3 t \cdot \frac{d}{d t}(\sqrt{\cos 2 t})}{\cos 2 t}\)
= \(\frac{\sqrt{\cos 2 t} \cdot 3 \cos ^2 t \cdot \frac{d}{d t}(\cos t)-\cos ^3 t \cdot \frac{1}{2 \sqrt{\cos 2 t}} \cdot \frac{d}{d t}(\cos 2 t)}{\cos 2 t}\)
=\(\frac{3 \sqrt{\cos 2 t} \cdot \cos ^2 t(-\sin t)-\cos ^3 t \cdot \frac{1}{2 \sqrt{\cos 2 t}} \cdot(-2 \sin 2 t)}{\cos 2 t}\)
=\(\frac{-3 \cos 2 t \cdot \cos ^2 t \cdot \sin t+\cos ^3 t \sin 2 t}{\cos ^2 t \cdot \sqrt{\cos 2 t}}\)
\(\frac{d y}{d x} =\frac{\left(\frac{d y}{d t}\right)}{\left(\frac{d x}{d t}\right)}=\frac{-3 \cos 2 t \cdot \cos ^2 t \cdot \sin t+\cos ^3 t \sin 2 t}{3 \cos 2 t \sin ^2 t \cos t+\sin ^3 t \sin 2 t}\)
=\(\frac{-3 \cos 2 t \cdot \cos ^2 t \cdot \sin t+\cos ^2 t(2 \sin t \cos t)}{3 \cos 2 t \sin ^2 t \cos t+\sin ^3 t(2 \sin t \cos t)}\)
=\(\frac{\sin t \cos t\left[-3 \cos 2 t \cdot \cos t+2 \cos ^3 t\right]}{\sin t \cos t\left[3 \cos 2 t \sin t+2 \sin ^3 t\right]}\)
=\(\frac{\left[-3\left(2 \cos ^2 t-1\right) \cos t+2 \cos ^3 t\right]}{\left[3\left(1-2 \sin ^2 t\right) \sin t+2 \sin ^3 t\right]}\)
⇒ \(\left[\begin{array}{l}
\cos 2 t=\left(2 \cos ^2 t-1\right)
\cos 2 t=\left(1-2 \sin ^2 t\right)
\end{array}\right]\)
=\(\frac{-4 \cos ^3 t+3 \cos t}{3 \sin t-4 \sin ^3 t}\)
∴ \(\frac{d y}{d x}=\frac{-\cos 3 t}{\sin 3 t}\left[\begin{array}{l}
\cos 3 t=4 \cos ^3 t-3 \cos t \sin 3 t=3 \sin t-4 \sin ^3 t
\end{array}\right]=-\cot 3 t\)
Question 8. x=a\(\left(\cos t+\log \tan \frac{t}{2}\right), y=a \sin t\)
Solution:
The given equations are x=a\(\left(\cos t+\log \tan \frac{t}{2}\right) and y=a \sin t\)
Then, \(\frac{\mathrm{dx}}{\mathrm{dt}}=\mathrm{a} \cdot\left[\frac{\mathrm{d}}{\mathrm{dt}}(\cos \mathrm{t})+\frac{\mathrm{d}}{\mathrm{dt}}\left(\log \tan \frac{\mathrm{t}}{2}\right)\right]\)
=\(\mathrm{a}\left[-\sin \mathrm{t}+\frac{\mathrm{t}}{\tan \frac{\mathrm{t}}{2}} \cdot \frac{\mathrm{d}}{\mathrm{dt}}\left(\tan \frac{\mathrm{t}}{2}\right)\right]\)
=a\(\left[-\sin t+\cot \frac{t}{2} \cdot \sec ^2 \frac{t}{2} \cdot \frac{d}{d t}\left(\frac{t}{2}\right)\right]=a\left[-\sin t+\frac{\cos \frac{t}{2}}{\sin \frac{t}{2}} \times \frac{1}{\cos ^2 \frac{t}{2}} \times \frac{1}{2}\right]\)
=a\(\left[-\sin t+\frac{1}{2 \sin \frac{t}{2} \cos \frac{t}{2}}\right]\)
=a\(\left(-\sin t+\frac{1}{\sin t}\right)=a\left(\frac{-\sin ^2 t+1}{\sin t}\right)\)
=a\(\left(\frac{\cos ^2 t}{\sin t}\right)\)
and \(\frac{d y}{d t}=a \frac{d}{d t}(\sin t)=a \cos t\)
∴ \(\frac{d y}{d x}=\frac{\left(\frac{d y}{d t}\right)}{\left(\frac{d x}{d t}\right)}=\frac{a \cos t}{\left(a \frac{\cos ^2 t}{\sin t}\right)}=\frac{\sin t}{\cos t}=\tan t\)
Question 9. x=a \(\sec \theta, y=b \tan \theta\)
Solution:
The given equations are x=a \(\sec \theta and y=b \tan \theta\)
Then, \(\frac{d x}{d \theta}=a \cdot \frac{d}{d \theta}(\sec \theta)=a \sec \theta \tan \theta\)
⇒ \(\frac{d y}{d \theta}=b \cdot \frac{d}{d \theta}(\tan \theta)=b \sec ^2 \theta\)
⇒ \(\frac{d y}{d x}=\frac{\left(\frac{d y}{d \theta}\right)}{\left(\frac{d x}{d \theta}\right)}\)
= \(\frac{b \sec ^2 \theta}{{asec} \theta \tan \theta}=\frac{b}{a} \sec \theta \cot \theta\)
= \(\frac{b \cos \theta}{a \cos \theta \sin \theta}=\frac{b}{a} \times \frac{1}{\sin \theta}=\frac{b}{a} {cosec} \theta\)
Question 10. x=a\((\cos \theta+\theta \sin \theta), y=a(\sin \theta-\theta \cos \theta)\)
Solution:
The given equations are x=a\((\cos \theta+\theta \sin \theta) and y=a(\sin \theta-\theta \cos \theta)\)
Then, \(\frac{d x}{d \theta} =a\left[\frac{d}{d \theta}(\cos \theta)+\frac{d}{d \theta}(\theta \sin \theta)\right]\)
=a\(\left[-\sin \theta+\theta \frac{d}{d \theta}(\sin \theta)+\sin \theta \frac{d}{d \theta}(\theta)\right]\)
=\(a(-\sin \theta+\theta \cos \theta+\sin \theta)=a \theta \cos \theta \)
and \(\frac{d y}{d \theta} =a\left[\frac{d}{d \theta}(\sin \theta)-\frac{d}{d \theta}(\theta \cos \theta)\right]\)
=\(a\left[\cos \theta-\left\{\theta \frac{d}{d \theta}(\cos \theta)+\cos \theta \cdot \frac{d}{d \theta}(\theta)\right\}\right]\)
=\(a[\cos \theta+\theta \sin \theta-\cos \theta]=a \theta \sin \theta \)
∴ \(\frac{d y}{d x} =\frac{\left(\frac{d y}{d \theta}\right)}{\left(\frac{d x}{d \theta}\right)}=\frac{a \theta \sin \theta}{a \theta \cos \theta}=\tan \theta\)
Question 11. If x=\(\sqrt{a^{\sin ^{-1} 1}}\), y=\(\sqrt{a^{\alpha x^{-1} t}}\), show that \(\frac{d y}{d x}=-\frac{y}{x}\) ?
Solution:
The given equations are x=\(\sqrt{a^{\sin ^{-1} t}}\) and y=\(\sqrt{a^{\cos ^{-1} t}}\)
x=\(\left(a^{\sin ^{-1} t}\right)^{\frac{1}{2}}\) and y=\(\left(a^{\cos ^{-1} t}\right)^{\frac{1}{2}}\)
x=\(a^{\frac{1}{2} {tin}^{-1} t}\) and y=\(a^{\frac{1}{2} \cos ^{-1} t}\)
Consider \(\mathrm{x}=\mathrm{a}^{\frac{1}{2} \sin ^{-1} \mathrm{t}}\)
Taking logarithms on both sides, we obtain
⇒ \(\log x=\frac{1}{2} \sin ^{-1} t \log a\)
⇒ \(\frac{1}{x} \cdot \frac{d x}{d t}=\frac{1}{2} \log a \cdot \frac{d}{d t}\left(\sin ^{-1} t\right)\)
⇒ \(\frac{d x}{d t}=\frac{x}{2} \log a \cdot \frac{1}{\sqrt{1-t^2}}\)
⇒ \(\frac{d x}{d t}=\frac{x \log a}{2 \sqrt{1-t^2}}\)
Then, consider y=\(a^{\frac{1}{2} \cos ^{-1} t}\)
Taking logarithms on both sides, we obtain
⇒ \(\log y=\frac{1}{2} \cos ^{-1} t \log a\)
⇒ \(\frac{1}{y} \cdot \frac{d y}{d t}=\frac{1}{2} \log a \cdot \frac{d}{d t}\left(\cos ^{-1} t\right)\)
⇒ \(\frac{d y}{d t}=\frac{y \log a}{2} \cdot\left(\frac{-1}{\sqrt{1-t^2}}\right)\)
⇒ \(\frac{d y}{d t}=\frac{-y \log a}{2 \sqrt{1-t^2}}\)
⇒ \(\frac{d y}{d x}=\frac{\left(\frac{d y}{d t}\right)}{\left(\frac{d x}{d t}\right)}\)
=\(\frac{\left(\frac{-y \log a}{2 \sqrt{1-t^2}}\right)}{\left(\frac{x \log a}{2 \sqrt{1-t^2}}\right)}=-\frac{y}{x}\)
Hence, proved.
Continuity And Differentiability Exercise 5.7
Question 1. \(x^2+3 x+2\)
Solution:
Let y=\(x^2+3 x+2\)
Differentiating w.r.t. x on both the sides
Then,\(\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{x}^2\right)+\frac{\mathrm{d}}{\mathrm{dx}}(3 \mathrm{x})+\frac{\mathrm{d}}{\mathrm{dx}}(2)\)
=2 \(\mathrm{x}+3+0=2 \mathrm{x}+3\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2}=\frac{d}{d x}(2 x+3)=\frac{d}{d x}(2 x)+\frac{d}{d x}(3)=2+0=2\)
Question 2. \(\mathrm{x}^{20}\)
Solution:
Let \(\mathrm{y}=\mathrm{x}^{20}\)
Then,\(\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{x}^{20}\right)=20 \mathrm{x}^{19}\)
Again differentiating with respect to x on both sides
∴ \(\frac{d^2 y}{d x^2}=\frac{d}{d x}\left(20 x^{19}\right)=20 \frac{d}{d x}\left(x^{19}\right)=20 \cdot 19 \cdot x^{18}=380 x^{18}\)
Question 3. x \(\cdot \cos x\)
Solution:
Let y=x \(\cdot \cos x\)
Then, \(\frac{d y}{d x}=\frac{d}{d x}(x \cdot \cos x)=\cos x \cdot \frac{d}{d x}(x)+x \frac{d}{d x}(\cos x)=\cos x \cdot 1+x(-\sin x)=\cos x-x \sin x\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2} =\frac{d}{d x}[\cos x-x \sin x]=\frac{d}{d x}(\cos x)-\frac{d}{d x}(x \sin x)\)
=-\(\sin x-\left[\sin x \cdot \frac{d}{d x}(x)+x \cdot \frac{d}{d x}(\sin x)\right]=-\sin x-(\sin x+x \cos x)=-(x \cos x+2 \sin x)\)
Question 4. log x
Solution:
Let y=\(\log \mathrm{x}\)
Then, \(\frac{d y}{d x}=\frac{d}{d x}(\log x)=\frac{1}{x}\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2}=\frac{d}{d x}\left(\frac{1}{x}\right)=\frac{-1}{x^2}\)
Question 5. \(x^3 \log x\)
Solution:
Let y=\(x^3 \log x\)
Then,\(\frac{d y}{d x} =\frac{d}{d x}\left[x^3 \log x\right]=\log x \cdot \frac{d}{d x}\left(x^3\right)+x^3 \cdot \frac{d}{d x}(\log x)\)
=\(\log x \cdot 3 x^2+x^3 \cdot \frac{1}{x}=\log x \cdot 3 x^2+x^2=x^2(1+3 \log x)\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2} =\frac{d}{d x}\left[x^2(1+3 \log x)\right]=(1+3 \log x) \cdot \frac{d}{d x}\left(x^2\right)+x^2 \frac{d}{d x}(1+3 \log x)\)
= \((1+3 \log x) \cdot 2 x+x^2 \cdot \frac{3}{x}\)
= 2 x+6 x \(\log x+3 x=5 x+6 x \log x=x(5+6 \log x)\)
Question 6. \(e^x \sin 5 x\)
Solution:
Let y=\(e^x \sin 5 x\)
⇒ \(\frac{d y}{d x}= \frac{d}{d x}\left(e^x \sin 5 x\right)\)
=\(\sin 5 x \cdot \frac{d}{d x}\left(e^x\right)+e^x \frac{d}{d x}(\sin 5 x)\)
=\(\sin 5 x \cdot e^x+e^x \cdot \cos 5 x \cdot \frac{d}{d x}(5 x)\)
=\(e^x \sin 5 x+e^x \cos 5 x \cdot 5=e^x(\sin 5 x+5 \cos 5 x)\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2} =\frac{d}{d x}\left[e^x(\sin 5 x+5 \cos 5 x)\right] \)
=\((\sin 5 x+5 \cos 5 x) \cdot \frac{d}{d x}\left(e^x\right)+e^x \cdot \frac{d}{d x}(\sin 5 x+5 \cos 5 x)\)
= \((\sin 5 x+5 \cos 5 x) e^x+e^x\left[\cos 5 x \cdot \frac{d}{d x}(5 x)+5(-\sin 5 x) \cdot \frac{d}{d x}(5 x)\right]\)
=\(e^x(\sin 5 x+5 \cos 5 x)+e^x(5 \cos 5 x-25 \sin 5 x)\)
=\(e^x(10 \cos 5 x-24 \sin 5 x)=2 e^x(5 \cos 5 x-12 \sin 5 x)\)
Question 7. \(e^{4 x} \cos 3 x\)
Solution:
Let y=\(e^{6 x} \cos 3 x\)
Then, \(\frac{d y}{d x}=\frac{d}{d x}\left(e^{6 x} \cdot \cos 3 x\right)\)
=\(\cos 3 x \cdot \frac{d}{d x}\left(e^{6 x}\right)+e^{4 x} \cdot \frac{d}{d x}(\cos 3 x)\)
=\(\cos 3 x \cdot e^{6 x} \cdot \frac{d}{d x}(6 x)+e^{6 x} \cdot(-\sin 3 x) \cdot \frac{d}{d x}(3 x)\)
=\(6 e^{6 x} \cos 3 x-3 e^{6 x} \sin 3 x\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2} =\frac{d}{d x}\left(6 e^{6 x} \cos 3 x-3 e^{6 x} \sin 3 x\right)\)
= \(6 \cdot \frac{d}{d x}\left(e^{6 x} \cos 3 x\right)-3 \cdot \frac{d}{d x}\left(e^{6 x} \sin 3 x\right)\)
=\(6 \cdot\left[6 e^{6 x} \cos 3 x-3 e^{6 x} \sin 3 x\right]-3 \cdot\left[\sin 3 x \cdot \frac{d}{d x}\left(e^{6 x}\right)+e^{6 x} \cdot \frac{d}{d x}(\sin 3 x)\right] [U {sing}(1)]\)
=\(36 e^{6 x} \cos 3 x-18 e^{6 x} \sin 3 x-3\left[\sin 3 x \cdot e^{6 x} \cdot 6+e^{6 x} \cdot \cos 3 x \cdot 3\right]\)
=\(36 e^{6 x} \cos 3 x-18 e^{6 x} \sin 3 x-18 e^{6 x} \sin 3 x-9 e^{6 x} \cos 3 x\)
=\(27 e^{6 x} \cos 3 x-36 e^{6 x} \sin 3 x=9 e^{6 x}(3 \cos 3 x-4 \sin 3 x)\)
Question 8. \(\tan ^{-1} x\)
Solution:
Let y=\(\tan ^{-1} x\)
Then, \(\frac{d y}{d x}=\frac{d}{d x}\left(\tan ^{-1} x\right)=\frac{1}{1+x^2}\)
Again differentiating with respect to x on both sides, use obtain
⇒ \(\frac{d^2 y}{d x^2}=\frac{d}{d x}\left(\frac{1}{1+x^2}\right)=\frac{d}{d x}\left(1+x^2\right)^{-1}\)
= \((-1) \cdot\left(1+x^2\right)^{-2} \cdot \frac{d}{d x}\left(1+x^2\right)\)
= \(\frac{-1}{\left(1+x^2\right)^2} \times 2 x=\frac{-2 x}{\left(1+x^2\right)^2}\)
Question 9. \(\log (\log x)\)
Solution:
Let y=\(\log (\log x)\)
Then, \(\frac{d y}{d x}=\frac{d}{d x}[\log (\log x)]=\frac{1}{\log x} \cdot \frac{d}{d x}(\log x)\)
=\(\frac{1}{x \log x}=(x \log x)^{-1}\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2}=\frac{d}{d x}\left[(x \log x)^{-1}\right]=(-1) \cdot(x \log x)^{-2} \cdot \frac{d}{d x}(x \log x)\)
=-\(\frac{1}{(x \log x)^2}\left[\log x \frac{d(x)}{d x}+x \cdot \frac{d}{d x}(\log x)\right]\)
=-\(\frac{1}{(x \log x)^2} \cdot\left[\log x \cdot 1+x \cdot \frac{1}{x}\right]=\frac{-(1+\log x)}{(x \log x)^2}\)
Question 10. \(\sin (\log x)\)
Solution:
Let \(\mathrm{y}=\sin (\log \mathrm{x})\)
Then, \(\frac{d y}{d x}=\frac{d}{d x}[\sin (\log x)]=\cos (\log x) \cdot \frac{d}{d x}(\log x)=\frac{\cos (\log x)}{x}\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2} =\frac{d}{d x}\left[\frac{\cos (\log x)}{x}\right]\)
= \(\frac{x \cdot \frac{d}{d x}[\cos (\log x)]-\cos (\log x) \frac{d}{d x}(x)}{x^2}\)
= \(\frac{x \cdot\left[-\sin (\log x) \cdot \frac{d}{d x}(\log x)\right]-\cos (\log x) \cdot 1}{x^2}\)
=\(\frac{-x \sin (\log x) \cdot \frac{1}{x}-\cos (\log x)}{x^2}\)
=\(\frac{-[\sin (\log x)+\cos (\log x)]}{x^2}\)
Question 11. If y=5 \(\cos x-3 \sin x, prove that \frac{d^2 y}{d x^2}+y=0\)
Solution:
It is given that, y=5 \(\cos x-3 \sin x\)
Then,\(\frac{d y}{d x}=\frac{d}{d x}(5 \cos x)-\frac{d}{d x}(3 \sin x)=5 \frac{d}{d x}(\cos x)-3 \frac{d}{d x}(\sin x)\)
=\(5(-\sin x)-3 \cos x=-(5 \sin x+3 \cos x)\)
Again differentiating with respect to \(\mathrm{x}\) on both the sides
⇒ \(\frac{d^2 y}{d x^2} =\frac{d}{d x}[-(5 \sin x+3 \cos x)]\)
=-\(\left[5 \cdot \frac{d}{d x}(\sin x)+3 \cdot \frac{d}{d x}(\cos x)\right]\)
=-\([5 \cos x+3(-\sin x)]=-[5 \cos x-3 \sin x]\)=-y
⇒ \(\frac{d^2 y}{d x^2}+y\) =0
Hence, proved.
Question 12. If y=\(\cos ^{-1}\) x, find \(\frac{d^2 y}{d x^2}\) in terms of y alone?
Solution:
It is given that, y=\(\cos ^{-1} x \Rightarrow x=\cos y\)
⇒ \(\frac{d y}{d x}=-\frac{1}{\sqrt{1-x^2}}=-\frac{1}{\sqrt{1-\cos ^2 y}}=-{cosec} y \)
⇒ \(\frac{d^2 y}{d x^2}=\frac{d}{d x}(-{cosec} y)\)
⇒ \(\frac{d^2 y}{d x^2}={cosec} y \cot y \frac{d y}{d x}\)
=-\({cosec} y \cot y\)
Alternate Method:
It is given that, y=\(\cos ^{-1} x\)
Then, \(\frac{d y}{d x}=\frac{d}{d x}\left(\cos ^{-1} x\right)=\frac{-1}{\sqrt{1-x^2}}=-\left(1-x^2\right)^{\frac{-1}{2}}\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2}=\frac{d}{d x}\left[-\left(1-x^2\right)^{\frac{-1}{2}}\right]\)
=-\(\left(-\frac{1}{2}\right) \cdot\left(1-x^2\right)^{\frac{-3}{2}} \cdot \frac{d}{d x}\left(1-x^2\right)\)
=\(\frac{1}{2 \sqrt{\left(1-x^2\right)^3}} \times(-2 x)\)
⇒ \(\frac{d^2 y}{d x^2}=\frac{-x}{\sqrt{\left(1-x^2\right)^2}}\)
⇒ \(y=\cos ^{-1} x \Rightarrow x=\cos y\)
Putting x=\(\cos y\) in equation (1), we obtain
⇒ \(\frac{d^2 y}{d x^2}=\frac{-\cos y}{\sqrt{\left(1-\cos ^2 y\right)^3}}=\frac{-\cos y}{\sqrt{\left(\sin ^2 y\right)^3}}\)
=\(\frac{-\cos y}{\sin ^3 y}=\frac{-\cos y}{\sin y} \times \frac{1}{\sin ^2 y}\)
∴ \(\frac{d^2 y}{d x^2}=-\cot y \cdot {cosec}^2 y\)
Question 13. If y=3 \(\cos (\log x)+4 \sin (\log x)\), show that \(x^2 y_2+x y_1+y\)=0
Solution:
It is given that, y=3 \(\cos (\log x)+4 \sin (\log x)\)
Then, \(y_1 =3 \cdot \frac{d}{d x}[\cos (\log x)]+4 \cdot \frac{d}{d x}[\sin (\log x)]\)
=3 \(\cdot\left[-\sin (\log x) \cdot \frac{d}{d x}(\log x)\right]+4 \cdot\left[\cos (\log x) \cdot \frac{d}{d x}(\log x)\right]\)
⇒ \(y_1 =\frac{-3 \sin (\log x)}{x}+\frac{4 \cos (\log x)}{x}=\frac{4 \cos (\log x)-3 \sin (\log x)}{x}\)
⇒ \(y_1 =4 \cos (\log x)-3 \sin (\log x)\)
Again differentiating with respect to x on both sides
x \(\frac{d\left(y_1\right)}{d x}+y_1 \frac{d(x)}{d x}=4(-\sin (\log x)) \frac{d}{d x}(\log x)-3 \cos (\log x) ⇒ \frac{d}{d x}(\log x)\)
⇒ \([latex]x y_2+y_1=\frac{-4 \sin (\log x)}{x}-\frac{3 \cos (\log x)}{x} \)
⇒ \(x^2 y_2+x y_1=-(4 \sin (\log x)+3 \cos (\log x))\)
⇒ \(x^2 y_2+x y_1=-y or x^2 y_2+x y_1+y=0\) [From eq. (1)]
Hence, proved.
Question 14. If y=500 \(e^{7 x}+600 e^{-7 x}\), show that \(\frac{d^2 y}{d x^2}\)=49 y
Solution:
It is given that, y=500 \(e^{1 x}+600 e^{-3 x}\)
Then, \(\frac{d y}{d x} =500 \cdot \frac{d}{d x}\left(e^{7 x}\right)+600 \cdot \frac{d}{d x}\left(e^{-7 x}\right)\)
=500 \(\cdot e^{7 x} \cdot \frac{d}{d x}(7 x)+600 \cdot e^{-7 x} \cdot \frac{d}{d x}(-7 x)=3500 e^{7 x}-4200 e^{-7 x}\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2} =3500 \cdot \frac{d}{d x}\left(e^{7 x}\right)-4200 \cdot \frac{d}{d x}\left(e^{-7 x}\right)\)
=3500 \(\cdot e^{7 x} \cdot \frac{d}{d x}(7 x)-4200 \cdot e^{-7 x} \cdot \frac{d}{d x}(-7 x)\)
=7 \(\times 3500 \cdot e^{7 x}+7 \times 4200 \cdot e^{-7 x}=49 \times 500 e^{7 x}+49 \times 600 e^{-7 x} \)
=49\(\left(500 e^{7 x}+600 e^{-7 x}\right)\)=49 y
Hence, proved.
Question 15. If \(\mathrm{e}^y(\mathrm{x}+1)=1\), show that \(\frac{\mathrm{d}^2 \mathrm{y}}{\mathrm{dx}^2}=\left(\frac{\mathrm{dy}}{\mathrm{dx}}\right)^2\)
Solution:
The given relationship is \(e^y(x+1)=1 \Rightarrow e^y=\frac{1}{x+1}\)
Taking logarithm on both the sides \(\log e^y=\log \frac{1}{(x+1)} \Rightarrow y=\log \frac{1}{(x+1)}\)
Differentiating this relationship with respect to x
⇒ \(\frac{\mathrm{dy}}{\mathrm{dx}}=(\mathrm{x}+1) \frac{\mathrm{d}}{\mathrm{dx}}\left(\frac{1}{\mathrm{x}+1}\right)\)
=\((\mathrm{x}+1) \cdot \frac{-1}{(\mathrm{x}+1)^2}=\frac{-1}{\mathrm{x}+1}\)
Again differentiating with respect to x on both sides
⇒ \(\frac{d^2 y}{d x^2}=-\frac{d}{d x}\left(\frac{1}{x+1}\right)=-\left(\frac{-1}{(x+1)^2}\right)\)
=\(\frac{1}{(x+1)^2}=\left(\frac{-1}{x+1}\right)^2=\left(\frac{d y}{d x}\right)^2\)
Hence, proved.
Question 16. If y=\(\left(\tan ^{-1} x\right)^2\), show that \(\left(x^2+1\right)^2 y_2+2 ⇒ x\left(x^2+1\right) y_1\)=2
Solution:
The given relationship is y=\(\left(\tan ^{-1} x\right)^2\).
Then, \(y_1=2 \tan ^{-1} x \frac{d}{d x}\left(\tan ^{-1} x\right)\)
⇒ \(y_1=2 \tan ^{-1} x \cdot \frac{1}{1+x^2}\)
⇒ \(\left(1+x^2\right) y_1=2 \tan ^{-1} x\)
Again differentiating with respect to x on both sides
⇒ \(\left(1+x^2\right) y_2+2 x y_1=2\left(\frac{1}{1+x^2}\right)\)
⇒\(\left(1+x^2\right)^2 y_2+2 x\left(1+x^2\right) y_1=2\)
Hence, proved.
Continuity And Differentiability Miscellaneous Exercise
Question 1. \(\left(3 x^2-9 x+5\right)^6\)
Solution:
Let y=\(\left(3 x^2-9 x+5\right)^{\prime}\)
Using the chain rule, we obtain
⇒ \(\frac{d y}{d x} =\frac{d}{d x}\left(3 x^2-9 x+5\right)^9=9\left(3 x^2-9 x+5\right)^1 \cdot \frac{d}{d x}\left(3 x^2-9 x+5\right)\)
=\(9\left(3 x^2-9 x+5\right)^3 \cdot(6 x-9)\)
=9\(\left(3 x^2-9 x+5\right)^3 \cdot 3(2 x-3)=27\left(3 x^2-9 x+5\right)^3(2 x-3)\)
Question 2. \(\sin ^3 x+\cos ^6 x\)
Solution:
Let y=\(\sin ^3 x+\cos ^4 x \)
⇒ \(\frac{d y}{d x} =\frac{d}{d x}\left(\sin ^3 x\right)+\frac{d}{d x}\left(\cos ^6 x\right)\)
=\(3 \sin ^2 x \cdot \frac{d}{d x}(\sin x)+6 \cos ^5 x \cdot \frac{d}{d x}(\cos x)\)
=3 \(\sin ^2 x \cdot \cos x+6 \cos ^5 x \cdot(-\sin x)\)
=3 \(\sin x \cos x\left(\sin x-2 \cos ^4 x\right)\)
Question 3. \((5 x)^{3 \mathrm{mon} t}\)
Solution:
Let y=\((5 x)^{2 \cos 2 x}\)
Taking logarithm on both sides, we obtain, \(\log y=3 \cos 2 x \log 5 x\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{y} \frac{d y}{d x}=3\left[\log 5 x \cdot \frac{d}{d x}(\cos 2 x)+\cos 2 x \cdot \frac{d}{d x}(\log 5 x)\right]\)
⇒ \(\frac{d y}{d x}=3 y\left[\log 5 x(-\sin 2 x) \cdot \frac{d}{d x}(2 x)+\cos 2 x-\frac{1}{5 x} \cdot \frac{d}{d x}(5 x)\right]\)
⇒ \(\frac{d y}{d x}=3 y\left[-2 \sin 2 x \log 5 x+\frac{\cos 2 x}{x}\right]\)
⇒ \(\frac{d y}{d x}=y\left[\frac{3 \cos 2 x}{x}-6 \sin 2 x \log 5 x\right]\)
∴ \(\frac{d y}{d x}=(5 x)^{3 \cos 2 x}\left[\frac{3 \cos 2 x}{x}-6 \sin 2 x \log 5 x\right]\)
Question 4. \(\sin ^{-1}(x \sqrt{x}), 0 \leq x \leq 1\)
Solution:
Let \(y=\sin ^{-1}(x \sqrt{x})\)
Using the chain rule, we obtain
⇒ \(\frac{d y}{d x} =\frac{d}{d x} \sin ^{-1}(x \sqrt{x})=\frac{1}{\sqrt{1-(x \sqrt{x})^2}} \times \frac{d}{d x}(x \sqrt{x})\)
=\(\frac{1}{\sqrt{1-x^3}} \cdot \frac{d}{d x}\left(x^{\frac{3}{2}}\right)\)
=\(\frac{1}{\sqrt{1-x^2}} \times \frac{3}{2} \cdot x^{\frac{1}{2}}=\frac{3 \sqrt{x}}{2 \sqrt{1-x^3}}\)
=\(\frac{3}{2} \sqrt{\frac{x}{1-x^3}}\)
Question 5. \(\frac{\cos ^{-1} \frac{x}{2}}{\sqrt{2 x+7}},-2<x<2\)
Solution:
Let \(\mathrm{y}=\frac{\cos ^{-1} \frac{\mathrm{x}}{2}}{\sqrt{2 \mathrm{x}+7}}\)
By the quotient rule, we obtain,
⇒ \(\frac{d y}{d x} =\frac{\sqrt{2 x+7} \frac{d}{d x}\left(\cos ^{-1} \frac{x}{2}\right)-\left(\cos ^{-1} \frac{x}{2}\right) \frac{d}{d x}(\sqrt{2 x+7})}{(\sqrt{2 x+7})^2}\)
=\(\frac{\sqrt{2 x+7}\left[\frac{-1}{\sqrt{1-\left(\frac{x}{2}\right)^2}} \cdot \frac{d}{d x}\left(\frac{x}{2}\right)\right]-\left(\cos ^{-1} \frac{x}{2}\right) \frac{1}{2 \sqrt{2 x+7}} \cdot \frac{d}{d x}(2 x+7)}{2 x+7}\),
=\(\frac{\sqrt{2 x+7} \frac{-1}{\sqrt{4-x^2}}-\left(\cos ^{-1} \frac{x}{2}\right) \frac{2}{2 \sqrt{2 x+7}}}{2 x+7}\)
=-\(\frac{\sqrt{2 x+7}}{\sqrt{4-x^2} \times(2 x+7)}-\frac{\cos ^{-1} \frac{x}{2}}{(\sqrt{2 x+7})(2 x+7)}\)
=-\(\left[\frac{1}{\sqrt{4-x^2} \sqrt{2 x+7}}+\frac{\cos ^{-1} \frac{x}{2}}{(2 x+7)^{\frac{3}{2}}}\right]\)
Question 6. \(\cot ^{-1}\left[\frac{\sqrt{1+\sin x}+\sqrt{1-\sin x}}{\sqrt{1+\sin x}-\sqrt{1-\sin x}}\right], 0<x<\frac{\pi}{2}\)
Solution:
Let y=\(\cot ^{-1}\left[\frac{\sqrt{1+\sin x}+\sqrt{1-\sin x}}{\sqrt{1+\sin x}-\sqrt{1-\sin x}}\right]\)
Then,\(\left[\frac{\sqrt{1+\sin x}+\sqrt{1-\sin x}}{\sqrt{1+\sin x}-\sqrt{1-\sin x}}\right]\)
=\(\frac{(\sqrt{1+\sin x}+\sqrt{1-\sin x})^2}{(\sqrt{1+\sin x}-\sqrt{1-\sin x})(\sqrt{1+\sin x}+\sqrt{1-\sin x})}\)
=\(\frac{(1+\sin x)+(1-\sin x)+2 \sqrt{(1-\sin x)(1+\sin x)}}{(1+\sin x)-(1-\sin x)}\)
=\(\frac{2+2 \sqrt{1-\sin ^2 x}}{2 \sin x}=\frac{1+\cos x}{\sin x}\)
=\(\frac{2 \cos ^2 \frac{x}{2}}{2 \sin \frac{x}{2} \cos \frac{x}{2}}=\cot \frac{x}{2}\)
Therefore, equation (1) becomes, y=\(\cot ^{-1}\left(\cot \frac{x}{2}\right)\)
⇒ \(y=\frac{x}{2}\)
⇒ \(\frac{d y}{d x}=\frac{1}{2} \frac{d}{d x}(x)\)
∴ \(\frac{d y}{d x}=\frac{1}{2}\)
Question 7. \((\log x)^{\log x}, x>1\)
Solution:
Let y=\((\log x)^{\log x}\)
Taking logarithms on both sides, we obtain
⇒ \(\log y=\log (\log x)^{\log x}\)
⇒ \(\log y=\log x \cdot \log (\log x)\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{y d y}=\frac{d}{d x}[\log x \cdot \log (\log x)]\)
⇒ \(\frac{1}{y d y}=\log (\log x) \cdot \frac{d}{d x}(\log x)+\log x \cdot \frac{d}{d x}[\log (\log x)]\)
⇒ \(\frac{d y}{d x}=y\left[\log (\log x) \cdot \frac{1}{x}+\log x \cdot \frac{1}{\log x} \cdot \frac{d}{d x}(\log x)\right]\)
⇒ \(\frac{d y}{d x}=y\left[\frac{1}{x} \cdot \log (\log x)+\frac{1}{x}\right] \Rightarrow \frac{d y}{d x}\)
=\((\log x)^{\log x}\left[\frac{1}{x}+\frac{\log (\log x)}{x}\right]\)
Question 8. \(\cos (a \cos x+b \sin x)\), for some constant a and b.
Solution:
Let y=\(\cos (a \cos x+b \sin x)\)
By using the chain rule, we obtain
⇒ \(\frac{d y}{d x} =\frac{d}{d x} \cos (a \cos x+b \sin x)\)
⇒ \(\frac{d y}{d x} =-\sin (a \cos x+b \sin x) \cdot \frac{d}{d x}(a \cos x+b \sin x)\)
=-\(\sin (a \cos x+b \sin x) \cdot[a(-\sin x)+b \cos x]=(a \sin x-b \cos x) \cdot \sin [a \cos x+b \sin x]\)
Question 9. \((\sin x-\cos x)^{(\sin x-\cos x)}, \frac{\pi}{4}<x<\frac{3 \pi}{4}\)
Solution:
Let y=\((\sin x-\cos x)^{(\sin x-606 x)}\)
Taking logarithms on both sides, we obtain
⇒ \(\log y=\log \left[(\sin x-\cos x)^{\sin x-\cos x)}\right]\)
⇒ \(\log y=(\sin x-\cos x) \cdot \log (\sin x-\cos x)\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{y d y}=\frac{d}{d x}[(\sin x-\cos x) \log (\sin x-\cos x)]\)
⇒ \(\frac{1 d y}{y d x}=\log (\sin x-\cos x) \cdot \frac{d}{d x}(\sin x-\cos x)+(\sin x-\cos x) \cdot \frac{d}{d x} \log (\sin x-\cos x)\)
⇒ \(\frac{1}{y} \frac{d y}{d x}=\log (\sin x-\cos x) \cdot(\cos x+\sin x)+(\sin x-\cos x) \cdot \frac{1}{(\sin x-\cos x)} \cdot \frac{d}{d x}(\sin x-\cos x)\)
⇒ \(\frac{d y}{d x}=y[(\cos x+\sin x) \cdot \log (\sin x-\cos x)+(\cos x+\sin x)]\)
∴ \(\frac{d y}{d x}=(\sin x-\cos x)^{(\sin x-\cos x)}(\cos x+\sin x)[1+\log (\sin x-\cos x)]\)
Question 10. Find \(\frac{d y}{d x}, if y=12(1-\cos t), x=10(t-\sin t),-\frac{\pi}{2}<t<\frac{\pi}{2}\)
Solution:
It is given that, y=12\((1-\cos t), x=10(t-\sin t)\)
⇒ \(\frac{\mathrm{dx}}{\mathrm{dt}}=\frac{\mathrm{d}}{\mathrm{dt}}[10(\mathrm{t}-\sin t)]=10 \cdot \frac{\mathrm{d}}{\mathrm{dt}}(\mathrm{t}-\sin t)=10(1-\cos \mathrm{t})\)
and\(\frac{\mathrm{dy}}{\mathrm{dt}}=\frac{\mathrm{d}}{\mathrm{dt}}[12(1-\cos \mathrm{t})]\)
=12 \(\cdot \frac{\mathrm{d}}{\mathrm{dt}}(1-\cos \mathrm{t})=12 \cdot[0-(-\sin t)]=12 \sin t\)
⇒ \(\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\left(\frac{\mathrm{dy}}{\mathrm{dt}}\right)}{\left(\frac{\mathrm{dx}}{\mathrm{dt}}\right)}\)
=\(\frac{12 \sin \mathrm{t}}{10(1-\cos \mathrm{t})}=\frac{12 \cdot 2 \sin \frac{\mathrm{t}}{2} \cdot \cos \frac{\mathrm{t}}{2}}{10 \cdot 2 \sin ^2 \frac{\mathrm{t}}{2}}\)
= \(\frac{6}{5} \cot \frac{\mathrm{t}}{2}\)
Question 11. If x \(\sqrt{1+y}+y \sqrt{1+x}=0, for -1<x<1\), prove that \(\frac{d y}{d x}=-\frac{1}{(1+x)^2}\) It is given that, x \(\sqrt{1+y}+y \sqrt{1+x}=0 \Rightarrow x \sqrt{1+y}=-y \sqrt{1+x}\)
Solution:
Squaring both sides, we obtain
⇒ \(x^2(1+y)=y^2(1+x) \Rightarrow x^2+x^2 y=y^2+x y^2\)
⇒ \(x^2-y^2=x y^2-x^2 y\)
⇒ \(x^2-y^2=x y(y-x)\)
(x+y)(x-y)=x y(y-x)
⇒ \(x+y=-x y \)
(1+x) y=-x
⇒ \(y=\frac{-x}{(1+x)}\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{d y}{d x}=-\frac{(1+x) \frac{d}{d x}(x)-x \frac{d}{d x}(1+x)}{(1+x)^2}=-\frac{(1+x)-x}{(1+x)^2}=-\frac{1}{(1+x)^2}\)
Hence, proved.
Question 12. If \(\cos y=x \cos (a+y) with \cos a \neq \pm 1\), prove that \(\frac{d y}{d x}=\frac{\cos ^2(a+y)}{\sin a}\).
Solution:
⇒ \(\cos y=x \cos (a+y) \Rightarrow x=\frac{\cos y}{\cos (a+y)}\)
Differentiating both sides with respect to y. we obtain
⇒ \(\frac{d x}{d y}=\frac{\cos (a+y) \frac{d}{d y}(\cos y)-\cos y \frac{d}{d y}(\cos (a+y))}{\cos ^2(a+y)}\)
=\(\frac{-\cos (a+y) \sin y+\cos y \sin (a+y)}{\cos ^2(a+y)} \)
⇒ \(\frac{d x}{d y}=\frac{\sin (a+y-y)}{\cos ^2(a+y)}=\frac{\sin a}{\cos ^2(a+y)} (\sin (A-B)=\sin A \cos B-\cos A \sin B)\)
∴ \(\frac{d y}{d x}=\frac{\cos ^2(a+y)}{\sin a}\)
Question 13. If x=a\((\cos t+t \sin t)\) and y=a\((\sin t-t \cos t)\), find \(\frac{d^2 y}{d x^2}\)
Solution:
It is given that, x=a\((\cos t+t \sin t) and y=a(\sin t-t \cos t)\)
⇒ \(\frac{\mathrm{dx}}{\mathrm{dt}}=\mathrm{a} \cdot \frac{\mathrm{d}}{\mathrm{dt}}(\cos \mathrm{t}+\mathrm{t} \sin \mathrm{t})\)
=a\(\left[-\sin t+\sin t \cdot \frac{d}{d t}(t)+t \cdot \frac{d}{d t}(\sin t)\right]=a[-\sin t+\sin t+t \cos t]=a t \cos t \)
and \(\frac{d y}{d t}=a \cdot \frac{d}{d t}(\sin t-t \cos t)\)
=a\(\left[\cos t-\left\{\cos t \cdot \frac{d}{d t}(t)+t \cdot \frac{d}{d t}(\cos t)\right\}\right]=a[\cos t-\{\cos t-t \sin t\}]=a t \sin t \)
⇒ \(\frac{d y}{d x}=\frac{\left(\frac{d y}{d t}\right)}{\left(\frac{d x}{d t}\right)}=\frac{a t \sin t}{a t \cos t}=\tan t\)
Then, \(\frac{d^2 y}{d x^2}=\frac{d}{d x}\left(\frac{d y}{d x}\right)=\frac{d}{d x}(\tan t)\)
=\(\sec ^2 t \cdot \frac{d t}{d x}=\sec ^2 t \cdot \frac{1}{a t \cos t}[\frac{d x}{d t}=a t \cos t\)
⇒ \(\frac{d t}{d x}=\frac{1}{a t \cos t}]\)
=\(\frac{\sec ^3 \mathrm{t}}{\mathrm{at}}, 0<\mathrm{t}<\frac{\pi}{2}\)
Question 14. If f(x)=\(|x|^3\), show that \(f^{\prime \prime}(x)\) exists for all real x, and find it.
Solution:
It is known that, \(|\mathrm{x}|=\begin{cases}{cl}\mathrm{x}, & \text { if } \mathrm{x} \geq 0 \\ -\mathrm{x}, & \text { if } \mathrm{x}<0\end{cases}\).
Therefore when x \(\geq 0, f(x)=|x|^3=x^3\).
In this case, \(f(x)=3 x^2\) and hence, \(f^{\prime}(x)=6 x\)
When x<0, \(f(x)=|x|^3=(-x)^2=-x^3\)
In this case, \(f(x)=-3 x^2 and hence, f^{\prime \prime}(x)=-6 x\)
Thus, for \(f(x)=|x|^3, f^{\prime}(x)\) exists for all real x and is given by, \(f^{\prime \prime}(x)=\begin{cases}{cl}6 x, & \text { if } x \geq 0 \\ -6 x, & \text { if } & x<0\end{cases}\).
Question 15. Using the fact that \(\sin (A+B)=\sin A \cos B+\cos A \sin B\) and the differentiation, obtain the sum formula for cosines.
Solution:
⇒ \(\sin (A+B)=\sin A \cos B+\cos A \sin B\)
Differentiating both sides with respect to X, we obtain
⇒ \(\frac{d}{d x}[\sin (A+B)]=\frac{d}{d x}(\sin A \cos B)+\frac{d}{d x}(\cos A \sin B)\)
⇒ \(\cos (A+B) \cdot \frac{d}{d x}(A+B)\)
= \(\cos B \cdot \frac{d}{d x}(\sin A)+\sin A \cdot \frac{d}{d x}(\cos B)+\sin B \cdot \frac{d}{d x}(\cos A)+\cos A \cdot \frac{d}{d x}(\sin B)\)
⇒ \(\cos (A+B) \cdot \frac{d}{d x}(A+B)\)
=\(\cos B \cdot \cos A \frac{d A}{d x}+\sin A(-\sin B) \frac{d B}{d x}+\sin B(-\sin A) \cdot \frac{d A}{d x}+\cos A \cos B \frac{d B}{d x}\)
⇒ \(\cos (A+B) \cdot\left[\frac{d A}{d x}+\frac{d B}{d x}\right]=(\cos A \cos B-\sin A \sin B) \cdot\left[\frac{d A}{d x}+\frac{d B}{d x}\right]\)
⇒ \(\cos (A+B)=\cos A \cos B-\sin A \sin B\)
Question 16. If y=\(\left|\begin{array}{ccc}f(x) & g(x) & h(x) \\ \ell & m & n \\ a & b & c\end{array}\right|\), prove that \(\frac{d y}{d x}=\left|\begin{array}{ccc}f^{\prime}(x) & g^{\prime}(x) & h^{\prime}(x) \\ \ell & m & n \\ a & b & c\end{array}\right|\)
Solution:
y=\(\left|\begin{array}{ccc}
f(x) & g(x) & h(x) \\
\ell & m & n \\
a & b & c
\end{array}\right|\)
y=\((m c-n b) f(x)-(\ell c-n a) g(x)+(c b-m a) h(x)\)
Then, \(\frac{d y}{d x} =\frac{d}{d x}[(m c-n b) f(x)]-\frac{d}{d x}[(c-n a) g(x)]+\frac{d}{d x}[(f b-m a) h(x)]\)
=\((m c-n b) f^{\prime}(x)-(f c-n a) g^{\prime}(x)+((b-m a) h^{\prime}(x)\).
=\(\left|\begin{array}{ccc}
f^{\prime}(x) & g^{\prime}(x) & h^{\prime}(x) \\
f & m & n \\
a & b & c
\end{array}\right|\)
Thus, \(\frac{d y}{d x}=\left|\begin{array}{ccc}
f^{\prime}(x) & g^{\prime}(x) & h^{\prime}(x) \\
c & m & n \\
a & b & c
\end{array}\right|\)
Question 17. If y=\(e^{3 \cos ^{-1} x},-1 \leq x \leq 1\), show that \(\left(1-x^2\right) \frac{d^2 y}{d x^2}-x \frac{d y}{d x}-a^2 y\)=0.
Solution:
It is given that, y=\(e^{0.06 E^{-1} x}\)
Taking logarithms on both sides, we obtain.
⇒ \(\log y=\log e^{a \cos ^{-1} x}\)
⇒ \(\log y=a \cos ^{-1} x \log e \Rightarrow \log y=a \cos ^{-1} x\)
Differentiating both sides with respect to x, we obtain
⇒ \(\frac{1}{y} \frac{d y}{d x}=a \times \frac{-1}{\sqrt{1-x^2}}\)
⇒ \(\frac{d y}{d x}=\frac{-a y}{\sqrt{1-x^2}}\)
By squaring both the sides, we obtain
⇒ ⇒ \(\left(\frac{d y}{d x}\right)^2=\frac{a^2 y^2}{1-x^2}\)
⇒ \(\left(1-x^2\right)\left(\frac{d y}{d x}\right)^2=a^2 y^2\)
Again differentiating both sides with respect to x, we obtain
⇒ \(\left(\frac{d y}{d x}\right)^2 \frac{d}{d x}\left(1-x^2\right)+\left(1-x^2\right) \times \frac{d}{d x}\left[\left(\frac{d y}{d x}\right)^2\right]\)
=\(a^2 \frac{d}{d x}\left(y^2\right)\)
⇒ \(\left(\frac{d y}{d x}\right)^2(-2 x)+\left(1-x^2\right) \times 2 \frac{d y}{d x} \cdot \frac{d^2 y}{d x^2}=a^2 \cdot 2 y \cdot \frac{d y}{d x}\)
-x\( \frac{d y}{d x}+\left(1-x^2\right) \frac{d^2 y}{d x^2}=a^2 \cdot y \left[\frac{d y}{d x} \neq 0\right]\)
⇒ \(\left(1-x^2\right) \frac{d^2 y}{d x^2}-x \frac{d y}{d x}-a^2 y\) = 0
Hence Proved