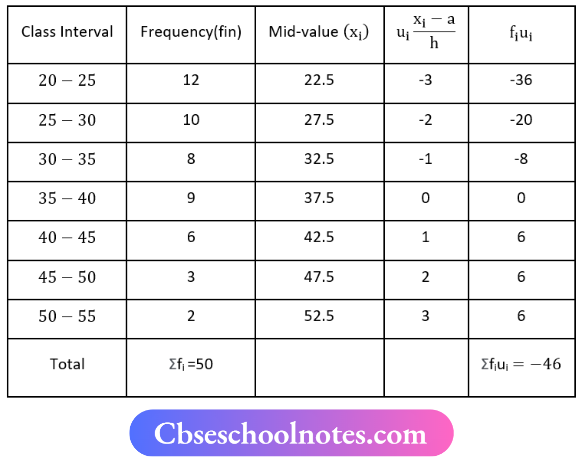
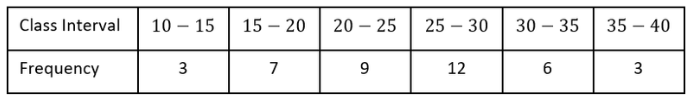
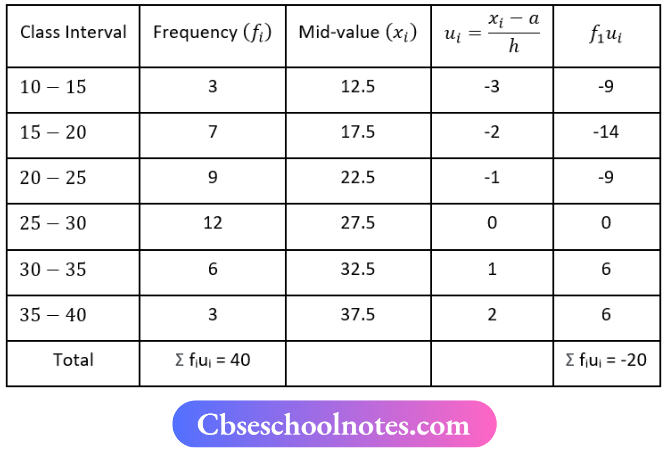
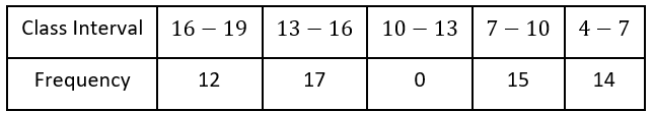
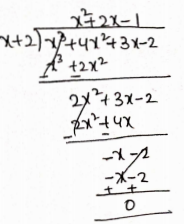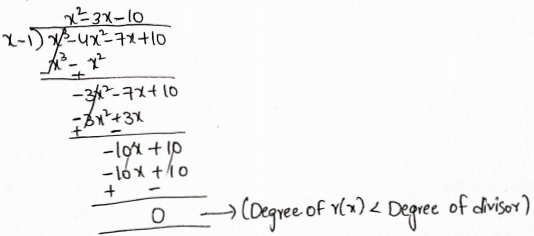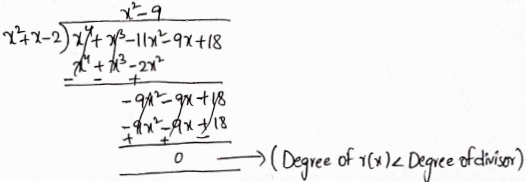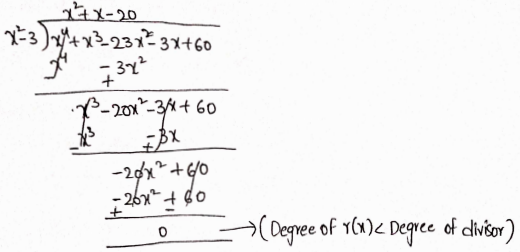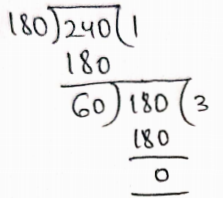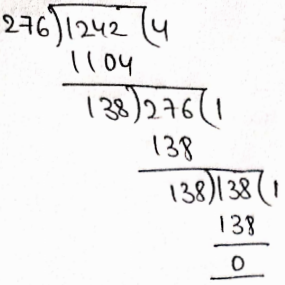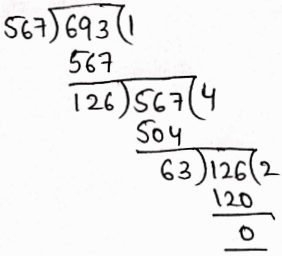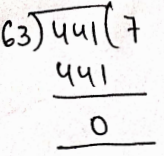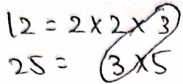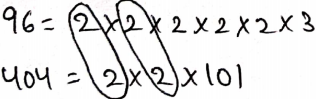Class 10 Maths

Class 10 Maths Introduction To Trigonometry

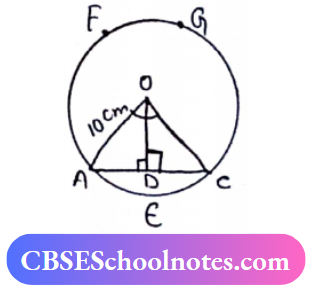
Question 1. In ΔABC, ∠B = 90° and, Sin A = \(\frac{3}{5}\) , then find all other trigonometric rotiss for ∠A.
Solution:

Sin A = \(\frac{3}{5}=\frac{perpendicular}{\text { hypo }}\)

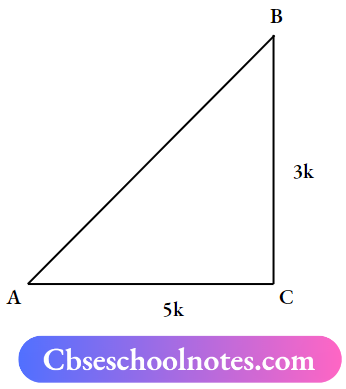
ΔABC

∠B=90°, BC=3t, AC=5k

AB² + BC²= AC²

AB² = AC² – BC²

= (25k²) – (9k²)

AB² = 16k²

AB = 4K

Read and Learn More Class 10 Maths

Cos A = \(=\frac{\text { base }}{\text { hypotenuse }}=\frac{A B}{A C}=\frac{4 k}{5 k}=\frac{4}{5}\)

tan A = \(\frac{\text {perpendicular }}{\text {base }}=\frac{B C}{A B}=\frac{3 k}{4 k}=\frac{3}{4}\)

Cosec A = \(\frac{\text { hypotenuse }}{\text { perpendicular }}=\frac{A C}{B C}=\frac{5 k}{3 k}=\frac{5}{3}\)

Sec A = \(\frac{\text { hypotenuse }}{\text { base }}=\frac{A C}{A B}=\frac{5 k}{4 k}=\frac{5}{4}\)

Cot A = \(\frac{\text { base }}{\text { perpendicular }}=\frac{A B}{B C}=\frac{4 k}{3 k}=\frac{4}{3}\)

Question 2. In ΔABC, ∠B = 90° and Cos A = \(\frac{9}{41}\), then find all other trigonometric ratios for ∠A.

Solution:

Cos A = \(\frac{9}{41}\)

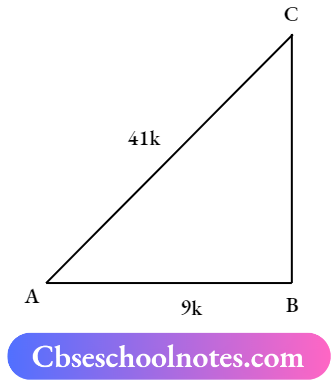
ΔABC

∠B=90°, AB=9k, AC = 41k

AB² + BC² = AC²

BC² = AC² – AB²

BC² = (41k) = (9k)²

BC² = 1681k² – 81k²

BC² = 1600k²

BC = 40k

Sin A = \(\frac{\text { perpendicular }}{\text { hypotemuse }}=\frac{B C}{A C}=\frac{40}{41}\)

tan A = \(\frac{\text { perpendicular }}{\text { base }}=\frac{B C}{A B}=\frac{40}{9}\)

Cosec A = \(\frac{\text { hypotenuse }}{\text { perpendicular }}=\frac{A C}{B C}=\frac{41}{40}\)

SecA = \(\frac{\text { hypotenusc }}{\text { base }}=\frac{A C}{A B}=\frac{41}{9}\)

Cot A = \(\frac{\text { base }}{\text { perpendicular }}=\frac{A B}{B C}=\frac{9}{40}\)

Question 3. In ΔABC, ∠A= 90° and tan B= \(\frac{5}{6}\), then find all other trigonometric ratios for ∠B.

Solution:

ΔABC, ∠Ạ = 90°

tan B = \(\frac{\text { Perpendicular }}{\text { Base }}=\frac{5}{6}\)

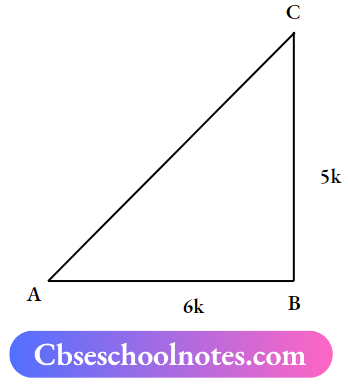
AC = 6K, BC=5K

AB² = AC²+BC²

AB² = (6k)² + (5k)²

AB² = 36k² +25k²

AB² = 61k²

AB = \(\sqrt{61 k}\)

Sin B = \(\frac{\text { Perpendicular }}{\text { hyotenuse }}=\frac{B C}{A B}=\frac{5 K}{\sqrt{61 K}}=\frac{5}{\sqrt{61}}\)

Cos B = \(\frac{\text { base }}{\text { hypotenuse }}=\frac{A C}{A B}=\frac{6 k}{\sqrt{61 k}}=\frac{6}{\sqrt{61}}\)

Cosec B = \(\frac{\text { hypotenuse }}{\text {Perpendicular}}=\frac{A B}{B C}=\frac{\sqrt{61}}{5}\)

Sec B = \(\frac{\text { hypotenuse }}{\text { base }}=\frac{A B}{A C}=\frac{\sqrt{61}}{6}\)

Cot B = \(\frac{\text { base }}{\text { perpendicular }}=\frac{A C}{B C}=\frac{6}{5}\)

Question 4. In ΔPQR, ∠R = 90° and Cosec P =\(\frac{13}{5}\), then find all trignometric ratio for ∠Q.

Solution:

ΔPQR, ∠R=90°

Cosec P = \(=\frac{\text { hypotenuse }}{\text { perpendicular }}=\frac{13}{5}=\frac{P Q}{Q R}\)

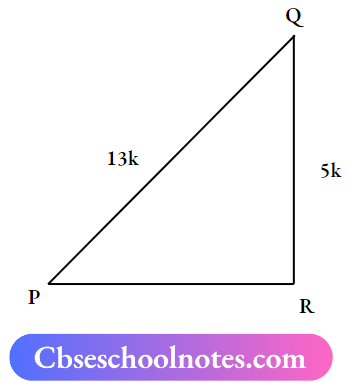
PR² + QR² = PQ²

PQ = 13K, QR = 5k

PR² = PQ² – QR²

= (13k)²– (5k)²

PR² = 169k²-25k²

PR² = 144k²

PR = 12k

Sin Q = \(\frac{\text { perpendicular }}{\text { hypotenuesc }}=\frac{Q R}{P Q}=\frac{5 K}{13 K}=\frac{5}{13}\)

Cos Q = \(\frac{\text { base }}{\text { hypdenuse }}=\frac{P R}{P Q}=\frac{12 k}{13 k}=\frac{12}{13}\)

tan Q = \(\frac{\text { perpendicular }}{\text { base }}=\frac{Q R}{P R}=\frac{5 k}{12 k}=\frac{5}{12}\)

Sec Q = \(\frac{\text { hypotenuse }}{\text { base }}=\frac{P Q}{P R}=\frac{13 k}{12 k}=\frac{13}{12}\)

Cot Q = \(=\frac{\text { base }}{\text { perpendicular }}=\frac{P R}{Q R}=\frac{12 k}{5 k}=\frac{12}{5}\)

Question 5. In ΔABC, ∠C = 90° and Sec B = \(\frac{5}{4}\), then find all other trigonometric ratios for ∠B.

Solution:

SecB = \(\frac{\text { hypo }}{\text { base }}=\frac{5}{4}=\frac{A B}{A C}\)

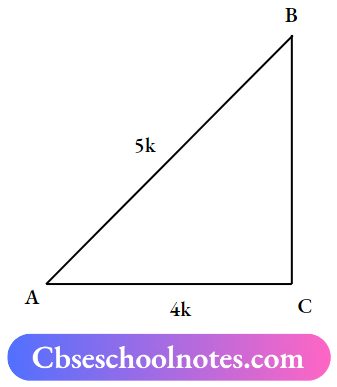
AB = 5K, AC = 4K

BC² = AB² – AC²

BC² = (5k)² = (4k)²

BC² = 25k² – 16k²

BC² = 9k²

BC = 3K

SinB = \(\frac{\text { perpendiular }}{\text { hypo }}=\frac{B C}{A C}=\frac{3 k}{4 k}=\frac{3}{4}\)

COS B = \(\frac{\text { base }}{\text { hypo }}=\frac{A B}{A C}=\frac{5 k}{4 k}=\frac{5}{4}\)

tan B = \(=\frac{\text { perpendicular }}{\text { base }}=\frac{B C}{A B}=\frac{3 k}{5 k}=\frac{3}{5}\)

Cosec B = \(\frac{\text { hypo }}{\text { base }}=\frac{A C}{B C}=\frac{4 k}{3 k}=\frac{4}{3}\)

Cot B = \(=\frac{\text { base }}{\text { perpendicular }}=\frac{A B}{B C}=\frac{5 k}{3 k}=\frac{5}{3}\)

Question 6. If tanθ = 2, then they find the value of \(\frac{2 \sin \theta \cos \theta}{\cos ^2 \theta-\sin ^2 \theta}\)

Solution:

tanθ = 2 = \(\frac{\sin \theta}{\cos \theta}=\frac{2}{1}\)

⇒ \(\frac{2 \sin \theta \cos \theta}{\cos ^2 \theta-\sin ^2 \theta}\)

⇒ \(\frac{2(2)(1)}{(1)^2-(2)^2}\)

⇒ \(\frac{4}{1-4}\)

⇒ \(\frac{-4}{3}\)

Question 7. If tanθ = \(\tan \theta=\frac{a}{b},\), then find the value of \(\frac{2 \sin \theta-3 \cos \theta}{2 \sin \theta+3 \cos \theta}\)

Solution:

⇒ \(\tan \theta=\frac{a}{b}\)

⇒ \(\frac{\sin \theta}{\cos \theta}=\frac{a}{b}\)

⇒ \(\frac{2 \sin \theta-3 \cos \theta}{2 \sin \theta+3 \cos \theta}\)

⇒ \(\frac{2 a-3 b}{2 a+3 b}\)

Question 8. If tanθ = \(\frac{3}{5}\), then find the Value of \(\frac{2 \sin \theta-3 \cos \theta}{2 \sin \theta+3 \cos \theta}\)

Solution:

⇒ \(\tan \theta=\frac{3}{5}\)

⇒ \(\frac{\sin \theta}{\cos \theta}=\frac{3}{5}\)

⇒ \(\frac{3 \sin \theta+4 \cos \theta}{3 \sin \theta-4 \cos \theta}\)

⇒ \(\frac{3(3)+4(5)}{3(3)-4(5)}\)

⇒ \(\frac{9+20}{9-20}\)

⇒ \(\frac{-29}{11}\)

Question 9. Find the value of Sin 60° Cos 30° – Cos 60° sin 30°.

Solution:

Sin 60° Cos 30°- Cos 60° Sin 30°

⇒ \(\left(\frac{\sqrt{3}}{2}\right)\left(\frac{\sqrt{3}}{2}\right)-\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)\)

⇒ \(\left(\frac{\sqrt{3}}{2}\right)^2-\left(\frac{1}{2}\right)^2\)

⇒ \(\frac{3}{4}-\frac{1}{4}\)

⇒ \(\frac{12-4}{16}\)

⇒ \(\frac{8}{16}\)

⇒ \(\frac{1}{2}\)

Question 10. Find the Value of \(\frac{2 \tan 30^{\circ}}{1-\tan ^2 30^{\circ}}\)

Solution:

⇒ \(\frac{2 \tan 30^{\circ}}{1-\tan ^2 30^{\circ}}\)

⇒ \(\frac{2\left(\frac{1}{\sqrt{3}}\right)}{1-\left(\frac{1}{\sqrt{3}}\right)^2}\)

⇒ \(\frac{\frac{2}{\sqrt{3}}}{1-\frac{1}{3}}\)

⇒ \(\frac{\frac{2}{\sqrt{3}}}{\frac{3-1}{3}}\)

⇒ \(\frac{\frac{2}{\sqrt{3}}}{\frac{2}{3}}\)

⇒ \(\sqrt{3}\)

Question 11. Find the value of 2sin 30° Cos30°

Solution:

2 sin 30° Cos 30°

⇒ \(2\left(\frac{1}{2}\right)\left(\frac{\sqrt{3}}{2}\right)\)

⇒ \(2\left(\frac{\sqrt{3}}{4}\right)\)

⇒ \(\frac{\sqrt{3}}{2}\)

Question 12. Show that: 5 Cos² 60° + 4 sec² 30° tan² 4s° + Cos² 90° = \(\frac{67}{12}\)

Solution:

5 Cos² 60° + 4 Sec² 30° – tan² 45° + Cos² 90°

⇒ \(5\left(\frac{1}{2}\right)^2+4\left(\frac{2}{\sqrt{3}}\right)^2-1+(0)^2\)

⇒ \(5\left(\frac{1}{4}\right)+4\left(\frac{4}{3}\right)-1\)

⇒ \(\frac{5}{4}+\frac{16}{3}-1\)

⇒ \(\frac{3 \times 5+4 \times 16-12}{12}\)

⇒ \(\frac{15+64-12}{12}\)

⇒ \(\frac{67}{12}\)

Question 13. Find the value of 4sin² 30° + tan² 60° + Sec² 45°

Solution:

4sin² 30°+ tan² 60°+ Sec² 45°.

⇒ \(4\left(\frac{1}{2}\right)^2+(\sqrt{3})^2+(\sqrt{2})^2\)

⇒ \(4\left(\frac{1}{4}\right)+3+2\)

= 1+3+2

= 6

Question 14. Find the Value of \(\frac{\sin 30^{\circ}}{\cos ^2 45^{\circ}}-\tan ^2 60^{\circ}+3 \cos 90^{\circ}+\sin 0^{\circ}\)

Solution:

⇒ \(\frac{\sin 30^{\circ}}{\cos ^2 45^{\circ}}-\tan ^2 60^{\circ}+3 \cos 90^{\circ}+\sin 0^{\circ}\)

⇒ \(\frac{\frac{1}{2}}{\left(\frac{1}{\sqrt{2}}\right)^2}-(\sqrt{3})^2+3(0)+0\)

⇒ \(\frac{\frac{1}{2}}{\frac{1}{2}}-3\)

⇒ 1 – 3 = -2

Question 15. Evaluate: \(\frac{\sin ^2 30^{\circ}+\sin ^2 45^{\circ}-4 \cot ^2 60^{\circ}}{2 \sin 30^{\circ} \cos 30^{\circ}+\frac{1}{2} \tan 60^{\circ}}\)

Solution:

⇒ \(\frac{\sin ^2 30^{\circ}+\sin ^2 45^{\circ}-4 \cot ^2 60^{\circ}}{2 \sin 30^{\circ} \cos 30^{\circ}+\frac{1}{2} \tan 60^{\circ}}\)

⇒ \(\frac{\left(\frac{1}{2}\right)^2+\left(\frac{1}{\sqrt{2}}\right)^2-4\left(\frac{1}{\sqrt{3}}\right)^2}{2\left(\frac{1}{2}\right)\left(\frac{\sqrt{3}}{2}\right)+\frac{1}{2}(\sqrt{3})}\)

⇒ \(\frac{\frac{1}{4}+\frac{1}{2}-4\left(\frac{1}{3}\right)}{\frac{\sqrt{3}}{2}+\frac{\sqrt{3}}{2}}\)

⇒ \(\frac{\frac{1}{4}+\frac{1}{2}-\frac{4}{3}}{\frac{2 \sqrt{3}}{4}}\)

⇒ \(\frac{\frac{1}{6}-\frac{4}{3}}{\frac{2 \sqrt{3}}{4}}\)

⇒ \(\frac{\frac{1-8}{6}}{\frac{2 \sqrt{3}}{4}}\)

⇒ \(\frac{\frac{-7}{6}}{\frac{2 \sqrt{3}}{4}}\)

⇒ \(\frac{\frac{-7}{6}}{\frac{\sqrt{3}}{2}}\)

⇒ \(\frac{-7}{12 \sqrt{3}}\)

Question 16. Show that: \(\sin 60^{\circ}=\frac{2 \tan 30^{\circ}}{1+\tan ^2 30^{\circ}}\)

Solution:

⇒ \(\sin 60^{\circ}=\frac{2 \tan 30^{\circ}}{1+\tan ^2 30^{\circ}}\)

⇒ \(\frac{\sqrt{3}}{2}=\frac{2\left(\frac{1}{\sqrt{3}}\right)}{1+\left(\frac{1}{\sqrt{3}}\right)^2}\)

⇒ \(\frac{\sqrt{3}}{2}=\frac{\frac{2}{\sqrt{3}}}{1+\frac{1}{3}}\)

⇒ \(\frac{\sqrt{3}}{2}=\frac{\frac{2}{\sqrt{3}}}{\frac{4}{3}}\)

⇒ \(\frac{\sqrt{3}}{2}=\frac{1}{\frac{2}{\sqrt{3}}}\)

⇒ \(\frac{\sqrt{3}}{2}=\frac{\sqrt{3}}{2}\)

Question 17. Show that: Cos² 60°- Sin² 60° = -Sin30°

Solution:

Cos² 60°-sin²60°= -Sin30°

⇒ \(\frac{1}{4}-\frac{3}{4}= -\frac{1}{2}\)

⇒ \(\frac{-2}{4}=\frac{-1}{2}\)

⇒ \(\frac{-1}{2}=\frac{-1}{2}\)

Question 18. Show that: tan 60° = \(\tan 60^{\circ}=\frac{2 \tan 30^{\circ}}{1-\tan ^2 30^{\circ}}\)

Solution:

⇒ \(\tan 60^{\circ}=\frac{2 \tan 30^{\circ}}{1-\tan ^2 30^{\circ}}\)

⇒ \(\sqrt{3}=\frac{2\left(\frac{1}{\sqrt{3}}\right)}{1-\left(\frac{1}{\sqrt{3}}\right)^2}\)

⇒ \(\sqrt{3}=\frac{2 / \sqrt{3}}{1-\frac{1}{3}}\)

⇒ \(\sqrt{3}=\frac{2/ \sqrt{3}}{\frac{3-1}{3}}\)

⇒ \(\sqrt{3}=\frac{2 / \sqrt{3}}{2 / 3}\)

⇒ \(\sqrt{3}=\frac{1}{1 / \sqrt{3}}\)

⇒ \(\sqrt{3}=\sqrt{3}\)

Question 19. Show that: \({Cos} 30^{\circ}=\sqrt{\frac{1+\cos 60^{\circ}}{2}}\)

Solution:

⇒ \({Cos} 30^{\circ}=\sqrt{\frac{1+\cos 60^{\circ}}{2}}\)

⇒ \(\frac{\sqrt{3}}{2}=\sqrt{\frac{1+\frac{1}{2}}{2}}\)

⇒ \(\frac{\sqrt{3}}{2}=\sqrt{\frac{2+1}{2}}\)

⇒ \(\frac{\sqrt{3}}{2}=\sqrt{\frac{\frac{2+1}{2}}{2}}\)

⇒ \(\frac{\sqrt{3}}{2}=\sqrt{\frac{\frac{3}{2}}{2}}\)

Question 20. Evaluate: \(\frac{\tan 45^{\circ}}{2 \sin 30^{\circ}-\cos 60^{\circ}}\)

Solution:

⇒ \(\frac{\tan 45^{\circ}}{2 \sin 30^{\circ}-\cos 60^{\circ}}\)

⇒ \(\frac{1}{2\left(\frac{1}{2}\right)-\frac{1}{2}}\)

⇒ \(\frac{1}{1-\frac{1}{2}}\)

⇒ \(\frac{1}{\frac{2-1}{2}}\)

⇒ \(\frac{1}{\frac{1}{2}}\)

= 2

Question 21. If A = 45°, then show that: Cos 2A = Cos²A – Sin²A

Solution:

Given A = 45°

Cos2(45°) = Cos²(45°)-Sin²(45°)

Cos 90° = \(\left(\frac{1}{\sqrt{2}}\right)^2-\left(\frac{1}{\sqrt{2}}\right)^2\)

0 = \(\frac{1}{2}-\frac{1}{2}\)

0 = 0

Question 22. If A=30° and B=60°, then show that: Cos (A+B) = CosA CosB – Sin A Sin B

Solution:

Given A= 30°, B=60°

Cos (30°+60°) = Cos 30° Cos 60° – Sin 30° Sin60°

⇒ \(\cos 90^{\circ}=\frac{\sqrt{3}}{2}\left(\frac{1}{2}\right)-\left(\frac{1}{2}\right) \frac{\sqrt{3}}{2}\)

0 = \(\frac{\sqrt{3}}{4}-\frac{\sqrt{3}}{4}\)

0=0

Question 23. If A = 30°, then show that: \(\tan 2 A=\frac{2 \tan A}{1-\tan ^2 A}\)

Solution:

Given A = 30°

tan 2(30°) = \(\frac{2 \tan 30^{\circ}}{1-\tan ^2 30^{\circ}}\)

tan 60° = \(\frac{2\left(\frac{1}{\sqrt{3}}\right)}{1-\left(\frac{1}{\sqrt{3}}\right)^2}\)

⇒ \(\sqrt{3}=\frac{2 / \sqrt{3}}{1-\frac{1}{3}}\)

⇒ \(\sqrt{3}=\frac{2 / \sqrt{3}}{\frac{3-1}{3}}\)

⇒ \(\sqrt{3}=\frac{2/\sqrt{3}}{2 / 3}\)

⇒ \(\sqrt{3}=\sqrt{3}\)

Question 24. If \(\cos \theta=\frac{\sqrt{3}}{2}\), then find the value of Sin 3θ.

Solution:

⇒ \(\cos \theta=\frac{\sqrt{3}}{2}\)

Cos θ = Cos30°

θ = 30°

= Sin 3θ

= Sin 3(30°)

= Sin 90°

= 1

Question 25. If tan (A+B) = \(\sqrt{3}\) and Sin (A-B) = \(\frac{1}{2}\), then find the value of tan (2A-3B)

Solution:

tan (A+B)= \(\sqrt{3}\)

Sin (A-B)= \(\frac{1}{2}\)

tan (2A-3B)

tan (A+B)= \(\sqrt{3}\)

A+B = 60°

Sin (A-B) = Sin 30° =) A-B = 30°

Acting equations

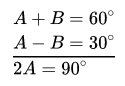
⇒ \(A=\frac{90^{\circ}}{2}=45^{\circ}\)

Subtracting equations

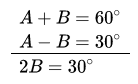
⇒ \(B=\frac{30^{\circ}}{2}\)

B = 15°

tan (2A-3B)

= tan (2(45°)-3((5°))

= tan (90°-45°)

= tan 45° = 1

Question 26. If A+B = 90° and tan A = \(\sqrt{3}\), then find the Value of B.

Solution:

A+B=90°

tan A = \(\sqrt{3}\)

tan A = tan 60°

A = 60°

60°+B=90°

B = 30°

Question 27. If A-B = 30° and Sin A = \(\frac{\sqrt{3}}{2}\), then find the value of B.

Solution:

A-B = 30°

Sin A = \(\frac{\sqrt{3}}{2}\)

Sin A = Sin 60°

A = 60°

60°-B = 30°

B = 30°

Question 28. \(\frac{1}{1+\tan ^2 \theta}+\frac{1}{1+\cos ^2 \theta}=1\)

Solution:

⇒ \(\frac{1}{1+\tan ^2 \theta}+\frac{1}{1+\cot ^2 \theta}\)

⇒ \(\frac{1}{1+\frac{\sin ^2 \theta}{\cos ^2 \theta}}+\frac{1}{1+\frac{\cos ^2 \theta}{\sin ^2 \theta}}\)

⇒ \(\frac{1}{\frac{\cos ^2 \theta+\sin ^2 \theta}{\cos ^2 \theta}}+\frac{1}{\frac{\sin ^2 \theta+\cos ^2 \theta}{\sin ^2 \theta}}\)

⇒ \(\frac{\cos ^2 \theta+\sin ^2 \theta}{1}\)

= 1 = R.H.S

Question 29. \(\sin ^2 \theta+\frac{1}{1+\tan ^2 \theta}=1\)

Solution:

⇒ \(\sin ^2 \theta+\frac{1}{1+\tan ^2 \theta}\)

⇒ \(\sin ^2 \theta+\frac{1}{1+\frac{\sin ^2 \theta}{\cos ^2 \theta}}\)

⇒ \(\sin ^2 \theta+\frac{\cos ^2 \theta}{1}\)

⇒ \(\sin ^2 \theta+\cos ^2 \theta\)

= 1 = R.H.S

Question 30. \(1+\frac{\cos ^2 \theta}{\sin ^2 \theta}-{cosec}^2 \theta=0\)

Solution:

⇒ \(1+\frac{\cos ^2 \theta}{\sin ^2 \theta}-{cosec}^2 \theta \Rightarrow \text { L.H.S }\)

⇒ \(1+\frac{\cos ^2 \theta}{\sin ^2 \theta}-\frac{1}{\sin ^2 \theta}\)

⇒ \(\frac{\sin ^2 \theta+\cos ^2 \theta-1}{\sin ^2 \theta}\)

⇒ \(\frac{1-1}{\sin ^2 \theta}=0=\text { R.H.S }\)

Question 31. \(\frac{1+\tan ^2 \theta}{{cosec}^2 \theta}=\tan ^2 \theta\)

Solution:

⇒ \(\text { L.H.S }=\frac{1+\tan ^2 \theta}{{cosec}^2 \theta}\)

⇒ \(\frac{1+\frac{\sin ^2 \theta}{\cos ^2 \theta}}{\frac{1}{\sin ^2 \theta}}\)

⇒ \(\frac{\frac{\cos ^2 \theta+\sin ^2 \theta}{\cos ^2 \theta}}{\frac{1}{\sin ^2 \theta}}\)

⇒ \(=\frac{1}{\cos ^2 \theta} \times \frac{\sin ^2 \theta}{1}\)

⇒ \(\tan ^2 \theta=\text { R.HS }\)

Question 32. Prove that: Cosec A-Cot A = \(\frac{1}{{cosec} A+\cot A}\)

Solution:

L.H.S = CosecA – Cota = \(({cosec} A-\cot A) \cdot \frac{({cosec} A+\cot A)}{({cosec} A+\cot A)}\)

⇒ \(\frac{{cosec}^2 A-\cot ^2 A}{{cosec} A+\cot A}\)

⇒ \(\frac{1}{{cosec} A+\cot A}\)

= R.H.S

Question 33. Prove that \(\frac{\sec A+1}{\tan A}=\frac{\tan A}{{Sec} A-1}\)

Solution:

L.H.S = \(\frac{\sec A+1}{\tan A}=\frac{\sec A+1}{\tan A} \times \frac{\sec A-1}{\sec A-1}\)

⇒ \(\frac{\sec ^2 A-1}{\tan A(\sec A-1)}=\frac{\tan ^2 A}{\tan A(\sec A-1)}\)

⇒ \(\frac{\tan A}{(\sec A-1)}\)

= R.H.S

Question 34. Check whether the equation \(\frac{\tan \phi+\sin \phi}{\tan \phi-\sin \phi}=\frac{\sec \phi+1}{\sec \phi-1}\) is an identity or not?

Solution:

L.H.S = \(\frac{\tan \phi+\sin \phi}{\tan \phi-\sin \phi}\)

⇒ \(\frac{\frac{\sin \phi}{\cos \phi}+\sin \phi}{\frac{\sin \phi}{\cos \phi}-\sin \phi}\)

⇒ \(\frac{\sin \phi \sec \phi+\sin \phi}{\sin \phi \sec \phi-\sin \phi}\)

⇒ \(\frac{\sin \phi(\sec \phi+1)}{\sin \phi(\sec \phi-1)}\)

⇒ \(\frac{\sec \phi+1}{\sec \phi-1}\)

= R.H.S

Question 35. If \(\cos ^2 30^{\circ}+\cos ^2 45^{\circ}+\cos ^2 60^{\circ}=x\), then find the value of “x”.

Solution:

⇒ \(\cos ^2 30^{\circ}+\cos ^2 45^{\circ}+\cos ^2 60^{\circ}=x\)

⇒ \(x=\left(\frac{\sqrt{3}}{2}\right)^2+\left(\frac{1}{\sqrt{2}}\right)^2+\left(\frac{1}{2}\right)^2\)

⇒ \(x=\frac{3}{4}+\frac{1}{2}+\frac{1}{4}\)

⇒ \(x=\frac{6+4+2}{8}\)

⇒ \(x=\frac{12}{8}\)

⇒ \(x=\frac{3}{2}\)

Question 36. Without using trigonometric tables, evaluate:

1. \(\frac{\sin 11^{\circ}}{\cos 79^{\circ}}\)

Solution:

⇒ \(\frac{\sin 11^{\circ}}{\cos 79^{\circ}}=\frac{\sin 11^{\circ}}{\left.\cos \left(90^{\circ}-79\right)^{\circ}\right)}=\frac{\sin 11^{\circ}}{\sin 11^{\circ}}=1\)

2. \(\frac{\sec 15^{\circ}}{{cosec} 75^{\circ}}\)

Solution:

⇒ \(\frac{\sec 15^{\circ}}{{cosec} 75^{\circ}}=\frac{\sec 15^{\circ}}{{cosec}\left(90^{\circ}-75^{\circ}\right)}=\frac{\sec 15^{\circ}}{{cosec} 15^{\circ}}=1\)

3. \(\frac{\tan 54^{\circ}}{\cot 36^{\circ}}\)

Solution:

⇒ \(\frac{\tan 54^{\circ}}{\cot 36^{\circ}}=\frac{\tan 54^{\circ}}{\cot \left(90^{\circ}-36^{\circ}\right)}=\frac{\tan 54^{\circ}}{\cot \left(90^{\circ}-36^{\circ}\right)}=\frac{\tan 54^{\circ}}{\tan 54{ }^{\circ}}=1\)

Question 37. Evaluate: tan42° – Cot 48°

Solution:

tan 42°-Cot 48° = tan 42°- Cot (90°- 42°)

tan42°-tan 42°

= 0

Question 38. Evaluate: Sex 36° – Cosec 54°

Solution:

Sec 36°- Cosec 54° = Sec 36° – Cosec (90°-36°)

= Sec 36° – Sec 36°

= 0

Question 39. Prove that: Sin 42°Cos 48°+ Sin 48° Cos42° = 1

Solution:

L.H.S= Sin 42° Cos48° + Sin48° Cos 42°

= Sin 42°cos (90°-42°)+ Sin(90° – 42°) Cos 42°

= Sin 42° Sin 42° + Cos42° Cos42°

Sin² 42° + Cos² 42°

= 1

Question 40. If Sin 3A = Cos(A-26°) where 3A is an acute angle, then find the value of A.

Solution:

Given that,

Sin 3A = Cos (A-26°)

Cos (90°-3A) = Cos (A-26°)

90°-3A = A-26°

-4A = -116°

A = 29°

Class 10 Maths Some Applications of Trigonometry

1. The length of the Shadow of a vertical pole is \(\frac{1}{\sqrt{3}}\) times its height. Show that the angle of elevation of the Sun is 60°.

Solution:

Let PQ be a vertical pole whose height is h. Its Shadow is OQ whose height is \(\frac{h}{\sqrt{3}}\)

Let the angle of elevation of the Sun is ∠POQ = 0

In APOQ,

⇒ \(\tan \theta=\frac{P Q}{O Q}=\frac{h}{h / \sqrt{3}}=\sqrt{3}=\tan 60^{\circ}\)

θ = 60°

∴ The angle of elevation of Sun = 60°

Read and Learn More Class 10 Maths

2. If a tower 30m high, casts a shadow \(10 \sqrt{3} \mathrm{~m}\) long on the ground, then what is the angle of elevation of the Sun?

Solution:

It is given that AB = 30m be the tower and BC = \(10 \sqrt{3} \mathrm{~m}\) m be its shadow on the ground.

Let θ be the angle of elevation.

In a right triangle,

tan θ = \(\frac{AB}{BC}\)

⇒ \(\frac{30}{10 \sqrt{3}}=\frac{3}{\sqrt{3}}=\sqrt{3}\)

= tan 60°

θ = 60°

∴ Hence, the angle of elevation θ = 60°

3. A ladder 15 meters long just reaches the top of a vertical wall. If the ladder makes an angle of 60° with the wall, find the height of the wall.

Solution:

Let PR be a ladder of length 15m and QR, a wall of height h.

Given that ∠PRQ = 60°

In ΔPQR,

Cos 60° = \(\frac{h}{PR}\) = \(\frac{1}{2}\) = \(\frac{h}{15}\)

⇒ h = \(\frac{15}{2}\)m

∴ Height of the wall = \(\frac{15}{2} m\)

4. A Circus artist is climbing a 20m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the Pole, if the angle made by the rope with the ground level is 30°.

Solution:

In ΔABC,

Sin 30°= \(\frac{A B}{A C}\)

⇒ \(\frac{1}{2}=\frac{A B}{20}\)

AB = 10

∴ Height of pole = 10m

5. A tree breaks due to stom and the broken part bends so that the top of the tree touches the ground making an angle 30° with it. The distance between the foot of the tree to the point where the top touches the ground is 8m. Find the height of the tree.

Solution:

Let the part CD of the tree BD broken in air and touches the ground at point A.

According to the problem,

AB = 8M

and ∠BAC = 30°

In ΔABC,

tan 30° = \(\frac{BC}{AB}\)

⇒ \(\frac{1}{\sqrt{3}}=\frac{B C}{8}\)

⇒ \(B=\frac{8}{\sqrt{3}} \mathrm{~m}\)

and Cos 30° = \(\frac{A B}{A C} \Rightarrow \frac{\sqrt{3}}{2}=\frac{8}{A C}\)

AC = \(\frac{16}{\sqrt{3}} m\)

CD = \(\frac{16}{\sqrt{3}} m\) (∵ AC = CD)

Now, the height of tree = BC + CD

⇒ \(\frac{8}{\sqrt{3}}+\frac{16}{\sqrt{3}}=\frac{24}{\sqrt{3}}=8 \sqrt{3} \mathrm{~m}\)

6. The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30°: Find the height of the tower.

Solution:

Let AB be the tower.

The angle of elevation of the top of the tower from Point C, 30m away from A is 30°

∴ In ABAC,

tan 30°= \(\frac{A B}{A C}\)

⇒ \(\frac{1}{\sqrt{3}}=\frac{A B}{A C}\)

AB = \(\frac{30}{\sqrt{3}}=10 \sqrt{3} \mathrm{~m}\)

∴ Height of the tower = \(10 \sqrt{3} \mathrm{~m}\)

7. From a point on the ground, the angles of elevation of the bottom and the top of a transmission tower fixed at the top of a 20m high building and 60° respectively. Find the height of the tower.

Solution:

Let, CD be the height of the transmission tower.

Here, the height of the building

BC = 20m

In ΔABC,

tan 45° = \(\frac{B C}{A B}\)

1 = \(\frac{20}{A B}\)

AB = 20m

In ΔABD,

tan 60° = \(\frac{B D}{A B} \Rightarrow \sqrt{3}=\frac{B D}{20}\)

⇒ \(B D=20 \sqrt{3} \mathrm{~m}\)

⇒ \(B C+C D=20 \sqrt{3}\)

⇒ \(20+C D=20 \sqrt{3}\)

⇒ \(C D=20(\sqrt{3}-1) m\)

∴ Height of transmission tower = \(20(\sqrt{3}-1) m\)

8. The Shadow of a tower Standing on a level plane is found to be much longer when the Sun’s elevation is 30° than when it is 60°. Find the height of the tower.

Solution:

Let AB be a tower of height ‘h’ meters and BD and BC be its shadows when the angles of elevation of the sun are 30° and 60° respectively.

∴ ∠ADB =30°, ∠ACB = 60° and CD = 50m

Let BC = X meters.

In ΔABC

tan 60° = \(\frac{A B}{B C} \Rightarrow \sqrt{3}=\frac{h}{x}\)

⇒ \(x=\frac{h}{\sqrt{3}}\)

In ΔABD

tan 30° = \(\frac{A B}{B D} \Rightarrow \frac{1}{\sqrt{3}}=\frac{h}{x+50}\)

⇒ \(\sqrt{3} h=x+50 \Rightarrow \sqrt{3} h=\frac{h}{\sqrt{3}}+50\)

2h= \(50 \sqrt{3}\)

h = \(25 \sqrt{3}\)

∴ Height of the tower = \(25 \sqrt{3}\)m

9. The angle of elevation of the top of a tower from a point on the ground is 30: After walking nom towards the tower, the angle of elevation becomes 60° Find the height of the tower.

Solution:

Let AB be a tower of height ‘h’ meters. From points D and c on the ground, the angle of elevation of top A of the tower is 30° and 60° respectively.

Given that CD = 40m

let BC = x meters

In ΔABC

tan 60° = \(\frac{A B}{B C} \Rightarrow \sqrt{3}=\frac{h}{x}\)

⇒ \(x=\frac{h}{\sqrt{3}}\)

In ΔABD

tan 30° =\(\frac{A B}{B D} \Rightarrow \frac{1}{\sqrt{3}}=\frac{h}{40+x}\)

⇒ \(\sqrt{3} h=40+x\)

⇒ \(\sqrt{3} h=40+\frac{h}{\sqrt{3}}\) [from (1)]

⇒ \(3 h=40 \sqrt{3}+h\)

⇒ \(2 h=40 \sqrt{3}\)

⇒ \(h=20 \sqrt{3}\)

∴ Height of the tower = \(20 \sqrt{3} \mathrm{~m}\) m

Class 10 Maths Probability

Question 1. In any situation that has only two possible outcomes, each outcome will have Probability. Find whether it is true or false.
Solution: False.

The probability of each outcome will be \(\frac{1}{2}\), only when the two outcomes are equally likely.

Question 2. A Marble is chosen at random from 6 marbles numbered 1 to 6. Find the Probability of getting a marble having number 2 and 6 on it.
solution:

The favourable Case is to get a marble on which both numbers 2 and are written. But there is no such marble.

So, N(E) = 0 and n(S) = 6

∴ Required probability = \(\frac{n(E)}{n(S)}=\frac{0}{6}=0\)

Question 3. A marble is chosen at random from 6 marbles numbered Ito 6. Find the Probability of getting a marble having number 2 or 6 on it.
Solution:

Here N(E) = 2

and n(S) = 6

∴ Required Probability = \(\frac{2}{6}=\frac{1}{3}\)

Read and Learn More Class 10 Maths

 

Question 4. It is given that in a group of 3 Students, the probability of 2 students not having the Same birthday is 0.992. What is the probability that the 2 students have the Same birthday.
Solution:

The probability of 2 students not having the Same birthday = 0.992

∴ Probability of 2 students having the same birthday

= 1-0.992

= 0.008

Question 5. A bag Contains 3 red balls and 5 black balls. A ball is drawn at random from the bag. What is the probability that the ball drawn is

1. Red?
2. Not ved?

Solution:

Total balls = 3+5 = -8

Total possible outcomes of drawing a ball at random from the bag = 8

1. Favorable outcomes of drawing a batt at random red ball =3

∴ The probability of drawing a red ball

⇒ \(\frac{\text { Favourable outcomes of drawing a red ball }}{\text { Total possible outcomes }}\)

⇒ \(\frac{3}{8}\)

2. Probability that the ball drawn is not red = 1- probability that the ball drawn

⇒ \(1-\frac{3}{8}=\frac{5}{8}\)

6. A die is thrown once. Find the probability of getting:

1. A prime number
2. A number lying between 2 and 6
3. An odd number.

Solution:

Possible outcomes in one throw of a die = {1,2,3,4,5,6}

Total possible outcomes = 6

1. Prime numbers = {2,3,5}=3

∴ Probability of getting prime number = \(\frac{3}{6}=\frac{1}{2}\)

2. Numbers lying between 2 to 6 = {3, 4, 5} = 3

∴ Probability of this event = \(\frac{3}{6}=\frac{1}{2}\)

3. Odd numbers = {1,3,5}=3

∴ Probability of this event = \(\frac{3}{6}=\frac{1}{2}\)

Question 7. 12 defective pens are accidentally mixed with 132 good to just look at a pen and tell whether or not it is taken out at random from this lot. Determine the probability Out is a good one.
Solution:

No. of good pens = 132

No. of defective pens = 12

Total pens = 132 +12 = 144

Total Favourable outcomes of drawing a pen = 144

Favourable outcomes of drawing a good pen = 132

∴ Probability of drawing a good pen = \(\frac{132}{144}=\frac{11}{12}\)

Question 8. A child has a die whose Six faces show the letters as

given below; The die is thrown once. what is the probability of getting

1. A?
2. D?

Solution:

Total possible outcomes in a throw of die = 6

1. Favourable outcome of getting A = 2

∴ Probability of getting \(A=\frac{2}{6}=\frac{1}{3}\)

2. Favourable outcomes of getting D = 1

∴ Probability of getting D = \(\frac{1}{6}\)

Question 9. A box Contains 90 discs which are numbered from 1 to 90. If one disc is drawn at random from the box, find the probability that it bears

1. A two-digit number,
2. A number divisible by 5.

Solution:

We have, n(S) = 90

1. Let A be the event of getting “a two-digit number”.

∴ Favourable cases are 10,11,12,13,14, …….., 90

∴ n(A) = 81

∴ P(A) = \(\frac{n(A)}{n(S)}=\frac{81}{90}=\frac{9}{10}\)

2. let B be the event of getting ་་ a number divisible by 5″.

∴ Favourable Cases are 10, 15, 20, 25, 30, …… 90.

Let there be n in numbers.

∴ T_n = 90

∴ 10+ (n-1)5 = 90

⇒ (n-1)5=80

⇒ n-1 = 16

⇒ n = 17

∴ n(B) = 17

P(B) = \(\frac{n(B)}{n(S)}=\frac{17}{90}\)

Question 10. It is known that a box of 600 electric bulbs Contains 12 defective bulbs. One bulb is taken out at random from this box, what is the probability that it is a non-defective bulb?
Solution:

The number of non-defective bulbs in the box = 600-12=588

So, probability of taking out a non-defective bulb = \(\frac{588}{600}=\frac{49}{50}\)

=0.98

Class 10 Maths Statistics

Question 1. Find the mean by the direct method:

Solution:

Now,

mean \(\bar{x}=\frac{\sum f_{i x_i}}{\sum f_i}=\frac{1100}{50}=22\)

Question 2. Find the mean using the Direct Method:

Read and Learn More Class 10 Maths

Solution:

Now, mean \(\bar{x}=\frac{\sum f_{i x i}}{\sum f_i}=\frac{13,200}{50}=264\)

Question 3. The mean of the following frequency distribution is 25. Find the value of P using the direct method:

Solution:

Now, Mean

⇒ \(\bar{x}=\frac{\sum f_{i x i}}{\sum f_i}\)

⇒ \(\bar{x}=\frac{1230+15 p}{42+p}\)

⇒ \(25=\frac{1230+15 P}{42+P}\)

⇒ 1050 + 25P = 1230 + 15P

⇒ 25P – 15P = 1230 + 15P

⇒ 10P = 180

⇒ P = \(\frac{180}{10}\)

P = 18

Question 4. The mean of the following distribution is 54. Find the value of P using the direct method:

Solution:

Now, Mean \(\bar{x}=54\)

⇒ \(\bar{x}=\frac{\sum f_{i x_i}}{\sum f_i}\)

54 = \(\frac{2070+70 p}{41+P}\)

2214+54p=2070+70P

70P-54P = 2214-2070

16P = 144

P = \(\frac{144}{16}\)

P = 9

Question 5. Find the mean from the following table using the Short Cut Method:

Solution:

Now, Mean a = 22.5

Mean \(\bar{x}=a+\frac{\sum f_{i d i}}{\sum f_i}\)

⇒ \(\bar{x}=22.5+\frac{0}{67}\)

⇒ \(\bar{x}=22.5\)

Question 6. Find the mean from the following table using the step. deviation method:

Solution:

Now, a = 37.5, h = 5

Mean \(\bar{x}=a+\frac{\sum f_{i u_i}}{\sum f_i} \times h\)

⇒ \(\bar{x}=37.5+\frac{-46}{50} \times 5\)

⇒ \(\bar{x}=37.5-\frac{46}{10}\)

⇒ \(\bar{x}=\frac{375-46}{10}\)

⇒ \(\bar{x}=\frac{329}{10}\)

⇒ \(\bar{x}=32.9\)

Question 7. Find the mean from the following table using the step deviation method:

Solution:

Now, a = 27.5, h = 5

mean \(\bar{x}=a+\frac{\sum f_i u_i}{\sum f_i} \times h\)

⇒ \(\bar{x}=27.5+\frac{-20}{40} \times 5\)

⇒ \(\bar{x}=\frac{220-20}{8}\)

⇒ \(\bar{x}=\frac{200}{8}\)

⇒ \(\bar{x}=25\)

Question 8. Find the median from the following data:

Solution:

Here, N = 58

∴ For Median class \(\frac{N}{2}=\frac{58}{2}=29\)

∴ Median class = 10-13

Here l₁ = 10, l₂ = 13

⇒ i = 13-10 = 3

f = 0, C.f = 29

∴ Median M = \(l_1+\frac{\left(\frac{N}{2}-c\right)}{f} \times i\)

M = \(10+\frac{29-29}{0} \times 3\)

M = \(10+\frac{0}{0} \times 3\)

M = 10

Question 9. Find the Median from the following data:

Solution:

Here, N = 50

⇒ \(\frac{N}{2}=\frac{50}{2}=25\)

=25

∴ Median Class = 20-30

Now, l₁ = 20, l₂ = 30

i = 30-20 = 10

f = 12, C = 15

and Median M = \(l_1+\frac{\left(\frac{N}{2}-C\right)}{f} \times i\)

M = \(20+\frac{(25-15)}{12} \times 10\)

M = \(20+\frac{10}{6} \times 5\)

M = \(\frac{120+50}{6}\)

M = \(\frac{170}{6}\)

M = 28.33

Question 10. Find the median from the following data:

Solution:

Here N = 50

⇒ \(\frac{N}{2}=\frac{50}{2}=25\)

∴ The median class is 15-20

Now, l₁ = 15, l₂ = 20, i = 20-15 = 5, f = 15, C = 20

and Median M = \(l_1+\frac{\left(\frac{N}{2}-C\right)}{f} \times i\)

M = \(15+\frac{25-20}{15} \times 5\)

M = \(\frac{45+5}{3}\)

M = \(\frac{50}{3}\)

M = 16.67

Question 11. Find the Median for the following frequency distribution:

Solution:

Now, N=340

⇒ \(\frac{N}{2}=\frac{340}{2}=170\)

∴ Median class = 39.5-46.5

∴ l₁ = 39.5, l₂ = 46.5, i= 46.5-39.5 = 7, f = 102, C = 199

Median M = \(l_1+\frac{\left(\frac{N}{2}-c\right)}{f} \times i\)

M = \(39.5+\frac{(170-199)}{102} \times 7\)

M = \(39.5-\frac{29}{102} \times 7\)

M = \(39.5-\frac{203}{102}\)

M = \(\frac{4029-203}{102}\)

M = \(\frac{3826}{102}\)

M = 36.5

Question 12. Find the Median for the following frequency distribution:

Solution:

Here N = 123

⇒ \(\frac{N}{2}=\frac{123}{2}=61.5\)

∴ Median Class = 20,5-25.5

l₁ = 20.5, l₂ = 25.5, i = 25.5-20.5 = 5, f = 24, C = 65

Median M = \(l_1+\frac{\left(\frac{N}{2}-C\right)}{F} \times i\)

M = \(20.5+\frac{(61.5-65)}{24} \times 5\)

M = \(20.5-\frac{3.5}{24} \times 5\)

M = \(\frac{492-17.5}{24}\)

M = \(\frac{474.5}{24}\)

M = 20.1

Question 13. If the Median of the following frequency distribution is 32.5. Find the value of F.

Solution:

Here N = 34+ P

⇒ \(\frac{N}{2}=\frac{34+P}{2}\)

Median = 325 ⇒ Median class=30-40

∴ 1₁ = 30, l₂ =40, i = 40-30=10

f = 12, C = 17

M = \(l_1+\frac{\left(\frac{N}{2}-c\right)}{f} \times i\)

32.5 = \(30+\frac{\left(\frac{34+p}{2}-17\right)}{12} \times 10\)

32.5-30 = \(\frac{10}{12}\left(\frac{34+P}{2}-17\right)\)

2.5 = \(\frac{5}{6}\left(\frac{34+p-34}{2}\right)\)

2.5 = \(\frac{5 P}{12}\)

30 = 5P

P = \(\frac{30}{5}\)

P = 6

Question 14. Determine the median for the following income distribution:

Solution:

Here N = 100

⇒ \(\frac{N}{2}=\frac{100}{2}=50\)

∴ Medion class = 300-400

∴ l₁ = 300, l₂ = 400, i= 400-300 = 100, f = 30, C = 33

M = \(l_1+\frac{\left(\frac{N}{2}-C\right)}{f} \times i\)

M = \(300+\frac{50-33}{30} \times 100\)

M = \(300+\frac{17}{30} \times 100\)

M = \(\frac{9000+1700}{30}\)

M = \(\frac{10700}{30}\)

M = 356.67

Question 15. Find the mode of the following frequency distribution:

Solution:

Clearly, the modal class is 60-80 as it has the maximum frequency

∴ l = 60, f₁ = 12, f₀ = 10, f₂ = 6, h = 20

Mode M = \(l+\frac{f_1-f_0}{2 f_1-f_0-f_2} \times h\)

M = \(60+\frac{12-10}{2(12)-10-6} \times 20\)

M = \(60+\frac{2}{24-16} \times 20\)

M = \(60+\frac{2}{8} \times 20\)

M = \(\frac{480+40}{8}\)

M = \(\frac{520}{8}\)

M = 65

Question 16. Given below is the frequency distribution of the heights of players in a school;

Solution:

Clearly, the modal class is 165.5-1685 as it has a maximum frequency

∴ l = 165.5, f₁ = 142, f₀ =118, f₂ = 127, h=3

Mode = \(l+\frac{f_1-f_0}{2 f_1-f_0-f_2} \times h\)

M = \(165.5+\frac{142-118}{2(142)-118-127} \times 3\)

M = \(165.5+\frac{24}{284-245} \times 3\)

M = \(165.5+\frac{24}{39} \times 3\)

M = \(\frac{6454.5+72}{39}\)

M = \(\frac{6526.5}{39}\)

M = 167.35

Question 17. Find the mode of the following frequency distribution:

Solution:

Clearly, the modal class is 50-60, as it has the maximum frequency

∴ l = 50, f₁ = 11, f₀ = 9, f₂ = 6, h = 10

Mode = \(\ell+\frac{f_1-f_0}{2 f_1-f_0-f_2} \times h\)

M = \(50+\frac{11-9}{2(11)-9-6} \times 10\)

M = \(50+\frac{2}{22-15} \times 10\)

M = \(50+\frac{2}{7} \times 10\)

M = \(\frac{350+20}{7}\)

M = \(\frac{370}{7}\)

M = 52.86

Question 18. The following distribution represents the height of 160 students in a class;

Solution:

Clearly, the modal class is 155-160 as it has the maximum

∴ l = 155, f₁ = 38, f₀ =30, f₂ =24, h=5

Mode = \(l+\frac{f_1-f_0}{2 f_1-f_0-f_2} \times h\)

M = \(155+\frac{38-30}{2(38)-30-24} \times 5\)

M = \(155+\frac{8}{76-54} \times 5\)

M = \(155+\frac{40}{22}\)

M = \(\frac{3410+40}{22}\)

M = \(\frac{3450}{22}\)

M = 156.82

Question 19. The following table gives the weekly wage of workers in a factory:

Find (1) the mean (2) the modal class (3) the mode

Solution:

1. Mean:

Mean = \(\frac{\sum f_{i x i}}{\sum f_i}=\frac{5520}{80}\)

= 69

2. Modal class = 55-60

3. Mode: clearly, the Modal class is 55-60 it has the maximum frequency

l = 55, f₁ = 20, f₀ = 5, f₂ = 10, h = 5

M = \(l+\frac{f_1-f_0}{2 f_1-f_0-f_2} \times h\)

M = \(55+\frac{20-5}{2(20)-5-10} \times 5\)

M = \(55+\frac{15}{40-15} \times 5\)

M = \(55+\frac{15}{25} \times 5\)

M = \(\frac{1375+75}{25}\)

M = \(\frac{1450}{25}\)

M = 58

Question 20. The mode of the following Series is 36. Find the missing frequency in it:

Solution:

Clearly, 30-40 is the modal class as mode 36 lies in this class

Here l = 30, f₁ = 16, f₀ = x (Say), f₂ = 12 and h = 10 and mode 36

Mode (M) = \(\ell+\frac{f_1-f_0}{2 f_1-f_0-f_2} \times h\)

36 = \(30+\frac{16-x}{2(16)-x-12} \times 10\)

36 = \(30+\frac{16-x}{32-x-12} \times 10\)

36 = \(30+\frac{16-x}{20-x} \times 10\)

36 – 30 = \(\frac{16-x}{20-x} \times 10\)

6(20-x) = 10(16-x)

120-6x = 160-10x

10x-6x = 160-120

4x = 40

x = \(\frac{40}{4}\)

x = 10

Question 21. Compute the mode of the following data:

Solution:

Clearly, Modal class 29.5 – 29.5 as it has the maximum frequency

∴ l = 19.5, f₁ = 23, f₀ = 16, f₂ = 15, h = 10

M = \(\ell+\frac{f_1-f_0}{2 f_1-f_0-f_2} \times h\)

M = \(19.5+\frac{23-16}{2(23)-16-15} \times 10\)

M = \(19.5+\frac{7}{46-31} \times 10\)

M = \(\frac{292.5+70}{15}\)

M = 24.17

Question 22. Find the mean, Median, and mode of the following data:

Solution:

let assumed mean A = 70, h = 20, Ef = 50, and Σfu = -19

Mean \(\bar{x}=A+\left[h \times \frac{\sum f_u}{\sum f}\right]\)

⇒ \(\bar{x}=70+\left[20 \times \frac{-19}{50}\right]\)

⇒ \(\bar{x}=70+[20 x-0.38]\)

⇒ \(\bar{x}=70-7.6\)

⇒ \(\bar{x}=62.4\)

Here N = 50

⇒ \(\frac{N}{2}=\frac{50}{2}\) = 25

Cumulative frequency just greater than 25 is 36 and the Corresponding class is 60-80.

∴ l₁ = 60, f = 12, l₂ = 80, C = 24, i = 80-60 = 20

Now, median (M) = \(l_1+\frac{\left(\frac{N}{2}-C\right)}{f} \times i\)

M = \(60+\frac{(25-24)}{12} \times 20\)

M = \(60+\frac{1}{12} \times 20\)

M = \(\frac{720+20}{12}\)

M = \(\frac{740}{12}\)

M = 61.66

Mode = 3(Median)-2(Mean)

Mode = 3(61.66)-2(62.4)

= 184.98-124.8

M = 60.18

Question 23. 100 Surnames were randomly picked from a local directory and the distribution of a number of letters of the English alphabet in the Surname was obtained as follows:

Solution:

let assumed mean A = 11.5, h = 3, Σf = 100, Σfu = -106

Mean \(\bar{x}=A+\left[h \times \frac{\sum(f u)}{\sum f}\right]\)

⇒ \(\bar{x}=11.5+\left[3 \times \frac{-106}{100}\right]\)

⇒ \(\bar{x}=11.5+[3 \times-1.06]\)

⇒ \(\bar{x}=11.5-3.18\)

⇒ \(\bar{x}=8.32\)

Here, N = 100

⇒ \(\frac{N}{2}=\frac{100}{2}\)

= 50

Cumulative frequency just greater than 50 is 76 and the corresponding class is 10-13

∴ l₁ = 7, f = 40, l₂ = 10, C = 36, i = 10-7 = 3

Median (M) = \(\ell+\frac{\left(\frac{N}{2}-C\right)}{f} \times i\)

M = \(7+\frac{50-36}{40} \times 3\)

M = \(7+\frac{14}{40} \times 3\)

M = \(\frac{280+42}{40}\)

M = \(\frac{322}{40}\)

M = 8.05

Mode = 3(Median)-2(Mean)

= 3(8.05)-2(8-32)

= 24.15-16.64

M = 7.51

Question 24. The following table gives the daily income of such workers of a factory:

Find the mean, mode, and median of the above data.

Solution:

let assumed mean A = 150, h = 20, Σf = 50, Σfu = -12

Mean \(\bar{x}=A+\left[h \times \frac{\sum f_4}{\sum P}\right]\)

⇒ \(\bar{x}=150+\left[20 \times \frac{-12}{50}\right]\)

⇒ \(\bar{x}=150+[20 x-0.24]\)

⇒ \(\bar{x}=150-4.8\)

⇒ \(\bar{x}=145.2\)

Here N = 50

⇒ \(\frac{N}{2}=\frac{50}{2}=25\)

Cumulative frequency just greater than 25 is 36 and the Corresponding is 120-140

∴ l₁ = 120, f = 14, l₂ = 140, C = 12, i = 140-120 = 20

Now, Median = \(l_1+\frac{\left(\frac{N}{2}- C\right)}{f} \times i\)

⇒ \(120+\frac{(25-12)}{14} \times 20\)

⇒ \(120+\frac{13}{14} \times 20\)

⇒ \(\frac{1680+260}{14}\)

⇒ \(\frac{1940}{14}\)

M = 138.57

Mode = 3(Median) – 2 (Mean)

M = 3(138.57)-2((45.2)

= 415.71-290.4

= 125.31

Question 25. A Survey regarding the heights (in cm) of so girls in a class was conducted and the following data was obtained:

Solution:

Let assumed Mean A=145, h=10, Σf=50, and Σfu=24

Mean \(\bar{x}=A+\left[h \times \frac{\sum f_u}{\sum f}\right]\)

⇒ \(\bar{x}=145+\left[10 \times \frac{24}{50}\right]\)

⇒ \(\bar{x}=145+[10 \times 0.48]\)

⇒ \(\bar{x}=145+4.8\)

⇒ \(\bar{x}=149.8 \mathrm{~cm}\)

Here N = 50

⇒ \(\frac{N}{2}=\frac{50}{2}=25\)

Cumulative frequency just greater than 25 is 42 and the Corresponding is 150-160

l₁ = 150, f = 20, l₂ = 160, C = 22, i = 160-150 = 10

Now, Median (M) = \(l_1+\frac{\left(\frac{N}{2}-c\right)}{F} \times i\)

⇒ \(150+\frac{(25-22)}{20} \times 10\)

⇒ \(150+\frac{30}{20}\)

⇒ \(\frac{3000+30}{20}\)

⇒ \(\frac{3030}{20}\)

= 151.5 cm

Mode = 3(Median)-2(Mean)

Mode = 3(151.5)-2(149.8)

= 454.5-299.6

= 154.9

Question 26. The table below shows the daily expenditure on food of 30 households in a locality:

Solution:

Let assumed mean A=225, h = 50, Σf=30, Σfu = -12

Mean \(\bar{x}=A+\left[h \times \frac{\sum f u}{\sum f}\right]\)

⇒ \(\bar{x}=225+\left[50 \times \frac{-12}{30}\right]\)

⇒ \(\bar{x}=225+[50 \times -0.4]\)

⇒ \(\bar{x}=225-20\)

⇒ \(\bar{x}=205\)

Here, N = 30

⇒ \(\frac{N}{2}=\frac{30}{2}=15\)

Median Class = 200-250

l₁ = 200, f = 12, l₂ = 250, C = 13, i = 250-200 = 50.

Median (M) = \(l_1+\frac{\left(\frac{N}{2}-c\right)}{F} \times i\)

M = \(200+\frac{15-13}{12} \times 50\)

M = \(\frac{2400+100}{12}\)

M = \(\frac{2500}{12}\)

M = 208.33

 

• Chapter 1 Real Numbers
• Chapter 2 Polynomials
• Chapter 3 Linear Equation In Two Variables
• Chapter 4 Quadratic Equations
• Chapter 5 Arithmetic Progression

• Chapter 7 Co-Ordinate Geometry

• Chapter 8 Introduction To Trigonometry
  Chapter 9 Some Applications of Trigonometry

• Chapter 10 Circles

• Chapter 12 Area Related To Circles
• Chapter 13 Volume And Surface Area Of Solids
• Chapter 14 Statistics

• Chapter 15 Probability

Class 10 Maths Volume And Surface Area Of Solids

Question 1. A tent of cloth is Cylindrical upto I’m height and Conical above it of the Same radius of base. If the diameter of the tent is 6m and the slant height of the Conical part is 5m, find the cloth required to make this tent.
Solution:

Diameter of base 2r = 6m

r = \(\frac{6}{2}\) = 3m

Height of Cylindrical path h = 1m

The slant height of Conical part l = 5m

Cloth required in tent = 2πrh +πrl

= πr(2h+1)

⇒ \(\frac{22}{7}\) x 3 (2×1 +5)

⇒ \(\frac{22}{7}\) x 3(10)

= 66m²

Read and Learn More Class 10 Maths

Question 2. The volume and Surface area of a Solid hemisphere are numerically equal. What is the diameter of the hemisphere?
Solution:

We have,

Volume of hemisphere = Surface area of hemisphere

⇒ \(\frac{2}{3}\) = 3πr²

⇒ \(\frac{2}{3}\) r = 3

2r = 9

Hence, the diameter of the hemisphere = 9 units.

Question 3. 2 Cubes each of volume 64 cm3 are joined end to end. Find the Surface area of the resulting Cuboid.
Solution:

Given,

volume of cube = 64 cm³

(Side)³ = 64

(Side)³ = 43

Side = 4cm

Side of cube = 4cm

A Cuboid is formed by joining two Cubes together.

∴ For Cuboid

length l = 4+4=8cm,

breadth b = 4cm

height b = 4cm

Now, the total surface area of the cuboid.

= 2(l.b+b.h+l.h)

2(8×4+ 4×4+8×4)

= 2(32+16+32)

2(80)

= 160 cm²

Question 4. From a Solid Cylinder whose height is 2.4cm and diameter is 1.4 cm, a Conical Cavity of the Same height and diameter is hollowed out. Find the total Surface area of the remaining Solid to the nearest Cm².
Solution:

Diameter of Cylinder 2r = 1.4cm

r = 0.7cm

∴ Radius of Cylinder = radius of cone = r= 0.70m

Height of Cylinder = height of cone

h = 2.40m

If the slant height of a cone is l, then

l² = h²+r² = (2.4)² + (0.7)²

5.76 +0.49 = 6.25

1 = \(\sqrt{6.25}\) = 2.5cm

The surface area of the remaining solid = area of the base of the cylinder + curved Surface of the cylinder + curved surface of the cone

πr² + 2πrh + πrl

=πr (r+2h+1)

= \(\frac{22}{7}\) × 0.7 (0.7+2×2.4+2.5)

= \(\frac{22}{7}\) × 0.7 × (5.86) 223×0.7

= \(\frac{22}{7}\): × 4.102

= 17.6cm²

Question 5. The radius and height of a solid right Circular Cone are in the ratio of 5:12. If its volume is 314 cm³, find its total Surface area. [Take π = 3.14]
Solution:

Let the radius of Cone = 5x

∴ Height of Cone =12x

l² = (5x)²+ (12x)²

l² = 25x² +144x²

l² = 169x²

l = \(\sqrt{169 x^2}\)

l = 13x

It is given that volume = 314 cm²

∴ \(\frac{1}{3}\)π(5x)2 (12x) = 314

⇒ \(\frac{1}{3}\) × 3.14 x 25×12 × x3 = 314

⇒  x³ = \(\frac{314 \times 3}{3.14 \times 25 \times 12}\) = 1

∴ x = 1cm

∴ Radius r = 5×1 = 5cm

Height h = 12×1 = 12cm

and slant height 1 = 13×1 = 13cm

Now, total surface area of Cone = πr(l+r) = 3.14 × 5(13+5) = 3.14×5×18

= 282.60cm²

Hence, the total surface area of cone is 282.60cm²

Question 6. The Curved Surface area of a Cone of height 8m is 188.4m². Find the volume of Cone.
Solution:

πrl = 188.4

⇒ rl = \(\frac{188.4}{3.14}\) = 60

r²l² = 3600

r² (h² +r²)=3600

r² (64+r²)=3600

r⁴+64r² = 3600 = 0

(r² +100) (r² – 36)=0

∴ r² = -100 or r²=36

r = 6

(∵ r² = -100 is not possible)

∴ Volume of a cone = \(\frac{1}{3} \pi r^2 h=\frac{1}{3} \times 3.14 \times 36 \times 8=301.44 \mathrm{~m}^3\)

Question 7. A Conical tent is required to accommodate 157 persons, each person must have 2m² of space on the ground and 15m³ of air to breathe. Find the height of the tent” Also Calculate the slant height.
Solution:

1 person needs 2m² of Space.

∴ 157 Persons needs 2 × 157 m² of Space on the ground.

πr² = 2 × 157

∴ r² = \(\frac{2 \times 157}{3.14}\)

r² = 100

r = 10m

Also, 1 person needs 15m3 of air.

∴ 157 Persons need 15×157 m2 of air.

∴ \(\frac{1}{3}\) πr2h = 15×157

⇒ h = \(\frac{15 \times 157 \times 3}{3.14 \times 100}\) = 22.5m

∴ l² = h² + r² = (225)² + (10)² = 606.25

∴ l = \(\sqrt{606.25}\) = 24.62m

Question 8. Three Cubes of metal whose edges are in the ratio 3:4:5 are melted down into a single cube whose diagonal is \(12 \sqrt{3}\) cm. Find the edges of the three cubes.
Solution:

The ratio in the edges = 3:4:5

Let edges be 3x, 4x and 5x respectively.

∴ Volumes of three cubes will be 27x³, 64x³, and 125x³ in cm³ respectively.

Now, the Sum of the Volumes of these three Cubes = 27x³ + 64x³+125x³

216x³ cm³

Let the edge of the new cube be a cm.

∴ Diagonal of new Cube = \(a \sqrt{3}\) cm

∴ \(a \sqrt{3}=12 \sqrt{3}\)

⇒ a = 12

∴ Volume of new Cube = (12)³ = 1728 cm³

Now by the given Condition

216x³ = 1728

x³ = 8

x = 2

∴ Edge of 1 Cube = 3×2 = 6cm

Edge of 2 Cube = 4×2 = 8 Cm

Edge of 3 Cube = 5×2 = 10cm

Question 9. A Solid is in the shape of a Cone Standing on a hemisphere with both their radii being equal to Icm and the height of the cone is equal to its radius. Find the volume of the Solid in terms of π.
Solution:

Rodius of hemisphere = radius of Cone = r = 1 cm

Height of Cone h = radius of Cone = 1 cm

Volume of hemisphere = \(\frac{2}{3}\) πr3

volume of Come = \(\frac{1}{3}\) πr2h

∴ Volume of Solid = Volume of hemisphere + volume of Come

⇒ \(\frac{2}{3} \pi r^3+\frac{1}{3} \pi r^2 h\)

⇒ \(\frac{2}{3} \pi(1)^3+\frac{1}{3} \pi(1)^2(1)\)

⇒ \(\frac{2}{3} \pi+\frac{1}{3} \pi\)

= π cm³

Question 10. A granary is in the shape of a Cuboid of a size 8m x 6m x 3m. If a bag of grain occupies a space of 0.65 m³, how many bags Can be stored in the granary?
Solution:

The Size of the granary is 8m x 6m x 3m.

∴ volume of granary =8×6×3 = 144m³

the volume of one bag of grain = 0.65m³

∴ The number of bags that can be stored in the granary

= \(\frac{\text { volume of granary }}{\text { volume of each bag }}\)

= \(\frac{144}{0.65}\)

= 221.54 or 221 bags.

Question 11. A cylindrical bucket 28cm in diameter and 72cm high is full of water. the water is emptied into a rectangular tank 66 cm long and 28cm wide. Find the height of the water level in the tank.
solution:

Let the height of the water level in the tank = xm, then according to problem

πr²h = l×b×x

Or \(\frac{22}{7} \times 14 \times 14 \times 72=66 \times 28 \times x\)

Or \(x=\frac{\frac{22}{7} \times 14 \times 14 \times 72}{66 \times 28}\)

x = 24 Cm

Question 12. A Solid Spherical ball of Iron with a radius of 6cm is melted and recast into three Solid Spherical balls. The radii of the two balls are 3cm and 4cm respectively, determining the diameter of the third ball.
Solution:

Let the radius of the third ball = r cm

∴ The volume of three balls formed = volume of the ball melted

⇒ \(\frac{4}{3} \pi(3)^3+\frac{4}{3} \pi(4)^3+\frac{4}{3} \pi(r)^3=\frac{4}{3} \pi(6)^3\)

⇒ 27 +64 +r³ = 216

⇒ r³ = 125, i.e., r = 5cm

The diameter of the third ball = 2×5cm = 10cm

Question 13. A Semicircle of radius 17.5cm is rotated about its diameter. Find the Curved Surface of So generated Solid.
Solution:

The Solid generated by a circle rotated about its diameter is a Sphere

Now, a radius of Sphere r = 17.5 cm

and its Curved Surface = 4π²

= \(4 \times \frac{22}{7} \times 17.5 \times 17.5\)

= 3850 cm²

Question 14. A sphere of radius 6cm is melted and recast into a Cone of height 6cm. Find the radius of the Cone.
Solution:

Radius of Sphere = 6cm

∴ Volume of Sphere = \(\frac{4}{3} \pi(6)^3\) = 288π cm³

Let radius of Cone = r

Height of Cone = 6cm

∴ Volume of Cone = \(\frac{1}{3} \pi r^2 h=\frac{1}{3} \pi r^2 \times 6=2 \pi r^2\)

Given that, Volume of Cone = volume of a sphere

2πr² = 288π

r² = 144

r = 12

Therefore, a radius of Cone = 12cm

Question 15. A metallic Cylinder of diameter 16 cm and height 9cm is melted and recast Into Sphere of diameter 6cm. How many such Spheres can be formed?
Solution:

For the Cylinder,

Radius = \(\frac{16}{2}\) = 8cm

Height = 9cm

∴ Volume of Cylinder = π (8)²(9) = 576 π cm³

Diameter of Sphere = 6cm

∴ Radius of Sphere = \(\frac{6}{2}\) = 3 cm

Now, Volume of one Sphere = \(\frac{4}{3} \pi(3)^3\) = 36π cm³

∴ Number of Spheres formed = \(=\frac{\text { volume of Cylinder }}{\text { volume of one Sphere }}\)

⇒ \(\frac{576 \pi}{36 \pi}\)

= 16

Question 16. The volume of a sphere is 288π Cm³, 27 Small Spheres Can be formed with this Sphere. Find the radius of the Small Sphere.
Solution:

Volume of 27 Small Spheres = Volume of One big Sphere = 288π

⇒ Volume of 1 Small Sphere = \(\frac{288}{27} \pi\)

⇒ \(\frac{4}{3} \pi r^3=\frac{22}{3} \pi\)

⇒ r³ = 8

⇒ r = 2cm

Question 17. A metallic Sphere of radius 4.2cm is melted and recast into the Shape of a Cylinder of radius 6cm, Find the height of the cylinder.
Solution:

Radius of Sphere R = 4.2cm

Radius of Cylinder r = 6cm

Let the height of the Cylinder = h

Now, the volume of the cylinder = Volume of the Sphere

⇒ \(\pi r^2 h=\frac{4}{3} \pi R^3\)

⇒ h = \(\frac{4 R^3}{3 r^2}\)

⇒ \(\frac{4 \times 4.2 \times 4.2 \times 4.2}{3 \times 6 \times 6} \mathrm{~cm}\)

= 2.744 сm

∴ Height of Cylinder = 2.744cm

Question 18. Metallic Spheres of radii 6cm, 8cm, and 10 cm, respectively, are melted to form a Single Solid Sphere. Find the radius of the resulting Sphere.
Solution:

Let r₁ = 6cm r₂ =8cm and r₃ = 10cm

let the radius of a bigger Solid Sphere = R

The volume of bigger Solid Volume = Sum of volumes of three given Spheres

⇒ \(\frac{4}{3} \pi R^3=\frac{4}{3} \pi r_1^3+\frac{4}{3} \pi r_2^3+\frac{4}{3} \pi r_3^3\)

⇒ \(R^3=r_1^3+r_2^3+r_3^3\)

⇒ \(R^3=6^3+8^3+10^3\)

⇒ R³ = 216 +512 +1000

⇒ R³ = 1728

⇒ R² = 12³

⇒ R = 12cm

∴ Radius of new Solid Sphere = 12 cm

Question 19. A 20m deep well with a diameter 7m is dug and the earth from digging is evenly Spread out to form a platform 22m of the platform.
Solution:

Radius of well, r = \(\frac{7}{2} m\)

and depth h = 20m

Let the height of the platform be H meter.

∴ The volume of platform = Volume of well

⇒ 22 x 14 x H = πr²h

⇒ \(22 \times 14 \times H=\frac{22}{7} \times \frac{7}{2} \times \frac{7}{2} \times 20\)

⇒ H = \(\frac{7 \times 5}{14}\)

⇒ H = \(\frac{35}{14}\)

⇒ H = 2.5mn

Height of platform = 2.5m

Question 20. A Cone is divided into two parts by drawing a plane through the mid-point of its axis, parallel to its base, Compare the volume of the two parts.
Solution:

We Can Solve this using Similarity

Let r and h be the radius and height of a Cone OAB

Let OE = \(\frac{h}{2}\)

As OED and OFB are Similar

∴ \(\frac{OE}{O F}=\frac{ED}{F B}\)

⇒ \(\frac{h / 2}{h}=\frac{ED}{r}\)

⇒ ED = \(\frac{r}{2}\)

Now volume of Cone OCD = \(\frac{1}{3} \pi r^2 h=\frac{1}{3} \times \pi \times\left(\frac{r}{2}\right)^2 \times \frac{h}{2}\)

⇒ \(\frac{\pi r^2 h}{24}\)

and volume of Cone DAB = \(\frac{1}{3} \times \pi x r^2 \times h\) = \(\frac{\pi r^2 h}{3}\)

∴ \(\frac{\text { volume of Part } O C D}{\text { volume of Part } C D A B}\)

⇒ \(\frac{\frac{\pi r^2 h}{24}}{\frac{\pi r^2 h}{3}-\frac{\pi r^2 h}{24}}\)

⇒ \(\frac{\frac{1}{24}}{\frac{1}{3}-\frac{1}{24}}\)

⇒ \(\frac{\frac{1}{24}}{\frac{1}{3}-\frac{1}{24}}\)

⇒ \(\frac{\frac{1}{24}}{\frac{8-1}{24}}\)

⇒ \(\frac{1}{7}\)

Question 21. A well of diameter 3m is dug lum deep. The earth taken out of it has been Spread evenly all around it in the Shape of a Circular ring of width um to form an embankment. Find the height of the embankment.
Solution:

Diameter of well 2r = 3m

r = \(\frac{2}{3}\) = 1.5m

and depth h = 14m

∴ Volume of earth taken out from well = πr²h

⇒ \(\frac{22}{7} \times 1.5 \times 1.5 \times 14=99 \mathrm{~m}^3\)

Now, the outer radius of the well, R = 1.5+4 = 5.5m

∴ Area of the ring of platform = π(R²– r²)

⇒ \(\frac{22}{7}\left[(5.5)^2-(1.5)^2\right]\)

⇒ \(\frac{22}{7}[30.25-2.25]\)

⇒ \(\frac{22}{7} \times 7 \times 4=88 \mathrm{~m}^2\)

let the height of the embankment = H

∴ 88 x H=99

⇒ \(H=\frac{99}{88}=\frac{9}{8}\)

= 1.125m

∴ Height of embankment = 1.125m

CBSE Solutions For Class 10 Mathematics Chapter 12 Area Related To Circles

Question 1. Find the area of a circle whose Circumference is 440m. 
Solution: 

Circumference of a circle = 440m

2πr = 440

⇒ \(r=\frac{440 \times 7}{2 \times 22}\)

r = 70m

Area of a circle = πr² = \(\frac{22}{77} \times 70 \times 70\)

= 22 ×10×70

= 15400 m²

Question 2. Find the radius of a Circular sheet whose area is 55442″. 
Solution: 

Area of Circular sheet

= 5544 m²

πr² = 5544

πr²= \(\frac{5544 \times 7}{22}\)

r² = \(\frac{38808}{22}\)

r² = 1764

r = 42m

Read and Learn More Class 10 Maths

Question 3. The area of a Circular plot is 346.5m. Calculate the Cost of fencing the plot at the rate of ± 6 Per metre. 
Solution:

Area of plot = 346.5m²

⇒ πr² = 346.5m²

⇒ r² = \(\frac{3465 \times 7}{22}\)

⇒ r²= 110.25

⇒ r = 10.5m

Circumference of plot = 2πY = 2x \(\frac{22}{7}\) x 10.5 =  66m

Cost of fencing = Circumference X Cost of fencing per metre.

= 66 × 6 =7396

Question 4. The radii of the two Circles are 19 cm and 9cm respectively. Find the radius of the Circle which has a Circumference equal to the Sum of the circumferences of two circles. 
Solution: 

Here, r₁ =19 cm and r₂ = 9 cm

Let the radius of the new circle =R Cm

Given that,

Circumference of given two new circles = Sum of the Circumferences of given two Circles

⇒ 2πR = 2πr₁ + 2πr₂

⇒ R = r₁+r₂ = 19+9 = 28cm

Question 5. The distance of a Cycle wheel is 28cm How many revolutions will it make in moving 13.2km? 
Solution: 

Distance travelled by the wheel in one revolution = 271 = 22 = x 28 = 88m Total distance travelled by the wheel = 13.2 x 1000 x 100 cm

Number of revolution made by the wheel = \(\frac{\text { total distance }}{\text { Circumference }}\)

⇒ \(\frac{13.2 \times 1000 \times 100}{88}\)

= 15000 revolutions.

Question 6. The Circumference of a Circle exceeds the diameter by 16-8 cm. Find the radius of the Circle. 

Solution: 

let the radius of the Circle be r.

Diameter = 2r

Circumference of Circle =2πr

using the given Information, we have

2πr = 2r+ 16.8

⇒ \(2 \times \frac{22}{7} \times r\) = 2r+16·8

⇒ 44r = l4r+ 16.8×7

⇒ 30r = 117.6

⇒ \(r=\frac{117.6}{30}=3.92 \mathrm{~cm}\)

Question 7. Find the area of 15cm. ring whose outer and Inner radii are respectively 20cm and 

Solution: 

Outer radius R = 200m

Immer radius r = 15 Cm

Area of ring = πT (Rr² – r²)

Area of ring = \(\frac{22}{7}\) [(20)² = (15)²]

⇒ \(\frac{22}{7}\) (400-225)

⇒ \(\frac{22}{7}\)  x 175

⇒ 22×25

⇒ 550cm²

Question 8. A race track is in the form of a · ring with an inner circumference of 352m and an outer Circumference of 396m. Find the width of the track. 

Solution: 

Let R and r be the outer and inner radii of the Circle.

The width of the track = (R-r) (m

Now,  2πr = 352

⇒ 2 x  \(\frac{22}{7}\) x r  = 352

⇒ r = \(\frac{352 \times 7}{2 \times 22}\)

r = 7 x 8= 56m

Again, 2πR=396

⇒ \(2 x \frac{352 \times 7}{2 \times 22}\) x R = 396

⇒ R= \(\frac{396 \times 7}{2 \times 22}\) = 7×9= 63m

R= 63m, r=56m

width of the track = (R-r)m = (63-56)m = 7m

Question 9. Two Circles touch internally. The Sum of their areas is 116π (m² and the distance between their Centres is 6cm. Find the radii of the circles. 

Solution: 

let two circles with Centers ‘o’ and o  having radial R and r respectively touch each other at P.

It is given that θ = 6

⇒ R-r=6

⇒ R= 6+r²

Also, πR²+ πr² = 116π

⇒ π(R² + r²) = 116π

⇒ R² + r² = 116 → 2

From equations (1) and (2), we get (6+r)² + r² = 116

⇒ 36+r²+12r+r²=116

⇒ 27 2 +(20-80=0

⇒ r² 768-40=0

⇒ (1+(0)(x-4)=0

So r=-10 and r=4

But the radius Cannot be negative. So, we reject r=-10

r = 4cm

R=6+4=10cm

Hence, the radii of the two circles are 4cm and 10 Cm.

Question 10. The radius of a wheel of a bus is 5 cm. Determine its Speed in kilometers per hour, when its wheel makes 315 revolutions per minute. 

Solution: 

The radius of the wheel of the bus = 45 Cm

Circumference of the wheel = 2πr

⇒ \(=2 \times \frac{22}{7} \times 45=\frac{1980}{7} \mathrm{~cm}\)

Distance Covered by the wheel in One revolution = \(=\frac{1980}{7} \mathrm{~cm}\)

Distance Covered by the wheel in 315 revolution = \(\frac{1980}{7}\)

= 45 x 1980 = 89100 cm

⇒ \(\frac{89100}{1000 \times 100} \mathrm{~km}=\frac{891}{1000} \mathrm{~km}\)

Distance Covered in 60 minutes to Ihr  = \(\frac{891}{1000} \times 60=\frac{5346}{100}=\) 53.46km

Hence, Speed of bus  = 53.46 km/hr.

Question 11. A Square of the largest circle is lost as trimmings? area is cut out of a circle. What /% of the area of 

Solution: 

Let the radius of the Circle be v units,

Area of Circle = πr² Sq. units

Let ABCD be the largest Square.

Length of diagonal = 2r= Side√2

Side = \(\frac{2 r}{\sqrt{2}}\) =√2r

Area (Square ABCD) = (Side)2 = (2x)2 = 2r²

Area of Circle lost by cutting out of a Square of largest area = 2r²

Required percentage of the area of c\(=\frac{2 r^2}{\pi r^2} \times 100=\frac{200}{\pi} \%\)

Question 12. The perimeter of a Semi Circular protractor is 32.4cm Calculate: 

1. The radius of the protractor in Cm, 
2.  The are of protractors in m². 

Solution:

1 . let the radius of the protractor be 5cm.

Perimeter of semicircle protractor = (πr+21) Cm

r(π+2)=324

⇒ r \(\left(\frac{22}{7}+2\right)\) = 32.4

⇒ \(r \times \frac{36}{7}=32.4\)

⇒ \(\frac{324 \times 7}{36}\)

⇒ r=6.3 cm

Hence, the radius of the protractor = 6.3 cm

2) Area of Semi Circular protractor =\(\frac{1}{2} \pi r^2\)

⇒ \(\frac{1}{2} \times \frac{22}{7} \times 6.3 \times 6.3\)

⇒ 62.37 Cm²

Hence, the area of the protractor = 62.37 cm²

Question 13. The minute hand of a clock Is √21 cm long. Find the area described as the minute hand on the face of the clock between 6 am. and 605 am. 

Solution: 

In 60 minutes, the minute hand of a clock move through an angle of 360°:

In 5 minutes hand will move through an angle = 360° x 5 = 30°,

Now, r=√21 Cm and 0=30°

Area of Sector described by the minute hand between 6 am and 6.05 am.

⇒ \(\frac{\pi r^2 \theta}{360^{\circ}} \)

⇒ \(\frac{22}{7} \times(\sqrt{21})^2 \times 30^{\circ} \times \frac{1}{360^{\circ}}\)

⇒ \(\frac{22}{7} \times 21 \times \frac{1}{12}\)

Question 14.  In the adjoining figure, Calculate: 

1. The length of minor arc ACB 
2. Area of shaded Sector.

Solution: 

Here, θ =150°, r=14cm

1) Length of minor are = \(\frac{\pi r \theta}{180^{\circ}}\)

\(\frac{22}{7} \times 14 \times 150^{\circ} \times \frac{1}{360^{\circ}}\)

= 36.67 Cm

2) Area of shaded sector= \(\frac{\pi r^2 \theta}{360^{\circ}}\)

⇒ \(\frac{22}{7} \times(14)^2 \times 150^{\circ} \times \frac{1}{360^{\circ}}\)

⇒ \(\frac{22}{7} \times 4^2 \times 14 \times 150^{\circ} \times \frac{1}{360^{\circ}}\)

⇒ \(44 \times 14 \times 150^{\circ} \times \frac{1}{360^{\circ}}\)

= 256.67 cm²

Question 15. Find the area of a sector of a circle with a radius of 6 cm if the angle of the Sector is 60°. 

Solution: 

Here, the radius of the circle, r=6cm

The angle of Sector, θ = 60°

Area of Sector =\(\frac{\theta}{360^{\circ}} \times \pi r^2\)

⇒ \(\frac{60^{\circ}}{360^{\circ}} \times \frac{22}{7} \times 6 \times 6 \mathrm{~cm}^2\)

⇒ \(\frac{1}{6} \times \frac{22}{7} \times 6 \times 6 \mathrm{~cm}^2\)

⇒ \(=\frac{132}{7} \mathrm{~cm}^2\)

= 18.86cm²

Question 16. Find the area of a quadrant of a circle whose Circumference is 22cm.

Solution: 

Circumference of Circle, 2πr=22

⇒ \(2 \times \frac{22}{7} \times r=22\)

⇒ \(r=\frac{7}{2} \mathrm{~cm}\)

Now, the area of the quadrant of the Circle,

⇒ \(\frac{1}{4} \pi r^2\)

⇒ \(\frac{1}{4} \times \frac{2 \pi}{7} \times \frac{7}{2} \times \frac{7}{2}\)

⇒ \(\frac{1}{2} \times 11 \times \frac{1}{2} \times \frac{7}{2}\)

⇒ \(\frac{11}{4} \times \frac{7}{2}\)

⇒ \(\frac{77}{8} \mathrm{~cm}^2\)

Question 17. The length of the minute hand of a clock is 14cm. Find the area an Sq ft take Swept by the Minute hand in 5 minutes. 

Solution: 

Length of a minute hand of clock = 14cm

Radius of Circle = 14cm

Angle Subtended by minute hand in 60 min  = 360°

Angle subtended by minute hand in Iminute =\(\frac{360^{\circ}}{60^{\circ}}=6^{\circ}\)

The angle subtended by minute hand in 5 minutes = 30°

From the formula,

Area of Sector of Circle = \(\frac{\theta \pi r^2}{360^{\circ}}\)

⇒ \(=30^{\circ} \times \frac{22 \times(14)^2}{7 \times 360^{\circ}}\)

⇒ \(\frac{22 \times 14 \times 2}{12}\)

⇒ \(\frac{616}{12}\)

⇒ \(\frac{154}{3} \mathrm{~cm}^2\)

Question 18. A chord of a circle of radius 10 cm Subtends a right angle at the  Centre. Find the area of the Corresponding: 

1. minor Segment 
2. major segment 

Solution: 

Given the radius of the Circle, A0-10cm.

The perpendicular is drawn from the Centre of the circle to the chord of the Circle to the chord of the circle which bisects this chord.

AD=DC

and LAOD = <COD  = 45°

LAOC = LAOD + LCOD

= 45° +45° =90°

In the right AAOD,

⇒ Sin45\(=\frac{A D}{A O} \Rightarrow \frac{1}{\sqrt{2}}=\frac{A D}{10}\)

⇒ AD=5√2 Cm

and cos 45° = \(\frac{O D}{A O} \Rightarrow \frac{1}{\sqrt{2}}=\frac{O D}{10}\)

⇒ OD = 5√2 Cm

Now, AC=2AD

= 2X5√2 = 10√2 cm

Now, the area of AAOC

⇒ \(\frac{1}{2}\) X AC X OD

⇒ \(\frac{1}{2}\) x 10√2 × 5√52

= 50cm²

Now, area of Sector

⇒ \(\frac{\theta \pi r^2}{360^{\circ}}=\frac{90^{\circ}}{360^{\circ}} \times 3.14 \times(10)^2\)

⇒ \(\frac{314}{4}\)

=78.5cm²

(1)Area of minor Segment AEC

= area of sector OAEC – area of Aoc

= 78.5-50 =  28,50m²

(2)Area of major SegSector OAFGCO

= area of a circle – an area of sector OAEC = πr²-78.5

= 3-14x(10) 278.5

= 314-785

= 235.5cm²

Question 19. A chord of a circle of radius 12CM Subtends an angle of 120° at the Centre Find the area of the Corresponding Segment of the Circle. (use πT = 3.14 and √3 = 1-73) 

Solution: 

Here, the radius of the circle, r = 12 Cm

Angle Subfended by the chord at the Centre, 0=120°

Area of Corresponding minor segment

⇒ \(\frac{\pi r^2 \theta}{360^{\circ}}-\frac{1}{2} r^2 \sin \theta\)

⇒ \(r^2\left(\frac{\pi \theta}{360^{\circ}}-\frac{1}{2} \sin \theta\right)\)

⇒ \(12 \times 12 \times\left(\frac{3.14 \times 120^{\circ}}{360^{\circ}}-\frac{1}{2} \times \sin 120^{\circ}\right)\)

⇒ \(144\left(\frac{3.14}{3}-\frac{1}{2} \times \frac{\sqrt{3}}{2}\right)\)

⇒ \(144\left(\frac{3.14}{3}-\frac{1.73}{4}\right)\)

= 88,44 cm²

Question 20.  A Car has two wipers which do not overlap. Each wiper has a blade of length 25 cm Sweeping through each Sweep of the blades. an angle of 115 find the total area cleaned at 

Solution:

Given, length of wiper blade  = 25 cm = r (Say)

The angle formed by this blade, 0=115°

Area cleaned by a blade = area of Sector formed  by blade

⇒ \(\frac{\theta \pi r^2}{360^{\circ}}\)

⇒ \(115^{\circ} \times \frac{22}{7 \times 360^{\circ}} \times(25)^2\)

⇒ \(\frac{23 \times 22}{7 \times 72} \times 625\)

⇒ \(\frac{23 \times 11 \times 625}{7 \times 36}\)

Total area cleaned by two blades =  2x area cleaned by a blade

⇒ \(\frac{2 \times 158125}{252}\)

⇒ \(\frac{158125}{126} \mathrm{~cm}^2\)

CBSE Solutions For Class 10 Mathematics Chapter 10 Circles

Question 1. The radius of a Circle and 8 Cm. Calculate the length of a tangent down to this Circle from a point at a distance of 10 Cm from its Centre.
Solution:

Since the tangent is perpendicular to the radius through the paint of Contact

∠OTP = 90

In the right triangle OTP, we have

⇒  Op² = OT²+ PT²

⇒  (10)² = (8)² + PT²

⇒ 100-64=PT²

⇒  PT² = 36

⇒ PT = 6 Cm

Hence, the length of the tangent is 6cm

Read and Learn More Class 10 Maths

Question 2. Prove that the tangents drawn at the ends of the diameter of a Circle are Parallel.
Solution:

Let AB be the diameter of a circle with Centre O. PA and PB are the tangents to the Circle at pants A and B respectively.

Now ∠PAB=90°

and∠QBA=90°

⇒  ∠PAB + ∠QBA = 90° +90° = 180°

PA ll QB

Question 3. prove that the perpendicular at the point of contact to the tangent to a Circler passes through the Centre.
Solution:

Given: A Circle with Centre 0 and a PQ tangent AQB and a Perpordicits is a dragon from point of contact Q to AB.

To prove: The perpendicular pa Passes through the Centre of the Circle.

Proof: AQ is the tangent of the Circle at point Q.

AQ will be the perpendicular to the radius of the circle.

⇒  PQ⊥AQ

⇒  The Centre of the Circle will lie on the line PQ.

Perpendicular PQ passes through the Centre of the Circle.

Question 4. A quadrilateral ABCD is drawn to Circumscribe a circle, and prove that AB+CD = AD+BC.
Solution:

As shown, the sides of a quadrilateral ABCD touch P a the Circle at P, Q, R and s. We know the tangents drawn from an external point to the Clucle are equal.

AP=AS, BP = BQ, CR = CQ, DR = DS

On adding, AP+BP + CR+DR

⇒  AB + BQ + CQ + DS

⇒ AB+CD= (AS + DS) + (BQ+CQ)

⇒  AB + CD = AP+BC

Hence proved.

Question 5. Ap is tangent to Circle 0 at point P. What is the length of OP?
solution:

Let the radius of the given Circle is r.

OP = OB = r

OA=2+r, OP=r, AP=4

∠OPA = 90°

In the right ∠OPA,

⇒  OA²= op² +Ap²

⇒  (2+r)² = r²+(4)²

⇒  4+r²+4r= r²+16

⇒  4r = 12 =) r=3

Op=3cm.

Question 6. If the angle between two tangents drawn from an external point p to a Clicle of radius ‘a’ and Centre 0, is 60°, then find the length of op .
Solution:

PA and PB are two tangents from an external point p such that

∠APB = 60°

∠OPA = ∠OPB = 30°

(tangents are equally inclined at the centre)

Also, ∠OAP=90°

Now, in right ∠OAP,

Sin 30° =\(=\frac{O A}{O P}\)

⇒ \(\frac{1}{2}=\frac{a}{o p}\)OP=2a units.

Question 7. In the given figure, if AB = AC, prove that BE = EC.
Solution:

We know that lengths of tangents from an external Point are equal.

AD=AF

DB = BE

EC = FC

Now, it is given that

AB = AC

⇒  AD+DB = AF + EC

⇒  AD+DB = A8+EC

⇒  DB = EC

BE = EC

Question 8. In the given figure, AT is tangent to the Chicle with Centre 0 Such that Oto 4cm and LOTA = 30° Find the length of Segment AT.
Solution:

In the right ∠OAT,

Cos 30°\(=\frac{A T}{O T}\)

⇒ \( \frac{\sqrt{3}}{2}=\frac{A T}{4}\)

⇒  AT = 2√3 Cm

Question 9. The length of a tangent from point A at a distance of 5 cm from the Centre P 5cm of the Circle is ucm. Find the radius of the Circle.
Solution:

Let o be the Centre of the Circle and PQ is a tangent to the Circle from point P.

Given that, PQ=4cm and op=5cm

Now,∠OOP = 90°

In ∠OQP,

⇒  OQ² = Op²= PQ²

= 52-42

=25-16=9

OQ = 3cm

Radius of Circle = 3cm

Question 10. Prove that the angle between the two tangents drawn from an external point to a circle is Supplementary to the angle Subtended by the line segment joining the points of contact at the Centre.
Solution:

PA and PB are the tangents of the Circle.

∠OAP = ∠OBP = 90°

In □ OAPB,

In □ OAPB,

∠OAP + ∠APB +∠OBP + ∠AOB = 360°

⇒ 90°+ ∠APB +90° +∠AOB = 360°

⇒  ∠APB + ∠AOB = 180°

⇒  ∠APB and ∠ADB are Supplementary

CBSE Solutions For Class 10 Mathematics Chapter 7 Co-ordinate Geometry

Question 1. Find the distance between the following points.

1.  A(-6,4) and B(2,-2)

Solution:

Distance between the points (-6,4) and (2,-2)

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2} \)

⇒  \(\sqrt{(2+6)^2+(-2-4)^2}\)

⇒  \(\sqrt{(8)^2+(-6)^2}\)

⇒  \(\sqrt{64+36}\)

⇒  \(\sqrt{100}\)

10 Units

2.A(-5,-1) and B (0,4)

Solution:

Distance between the points (-5,-1) and (0,4)

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

⇒  \(\sqrt{(0+5)^2+(4+1)^2}\)

⇒  \(\sqrt{(5)^2+(5)^2}\)

⇒  \(\sqrt{25+25}\)

⇒  \(\sqrt{50}\)

⇒  \(\sqrt{25 \times 2} \Rightarrow 5 \sqrt{2} \text { units }\)

Read and Learn More Class 10 Maths

 

3. A(-4,-1) and B(7,3)

Solution:

Distance between the points (-4,-1) and (7,3)

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

⇒  \(\sqrt{(7-4)^2+(3+1)^2}\)

⇒  \(\sqrt{(3)^2+(4)^2}\)

⇒  \(\sqrt{9+16}\)

⇒  \(\sqrt{25}\)

5 Units

4. A(3,4) And B(5,2)

Solution:

Distance between the points (3,4) And (5,2)

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

⇒  \(\sqrt{(5-3)^2+(2-4)^2}\)

⇒  \(\sqrt{(2)^2+(-2)^2}\)

⇒  \(\sqrt{4+4}\)

⇒  \(\sqrt{8}\)

⇒  \(\sqrt{4 \times 2} \Rightarrow 2 \sqrt{2} \text { units }\)

Question 2. Find the Distance of the following points from the origin:

1. (3,-4)

Solution:

Distance between the points (3,-4) And (0,0)

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

⇒  \(\sqrt{(0-3)^2+(0+4)^2}\)

⇒  \(\sqrt{(-3)^2+(4)^2}\)

⇒  \(\sqrt{9+16}\)

⇒  \(\sqrt{25}\)

5 Units

2. (-8,6)

Solution:

Distance between the points (-8,6) And (0,0)

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

⇒  \(\sqrt{(0+8)^2+(0+6)^2}\)

⇒  \(\sqrt{(8)^2+(6)^2}\)

⇒  \(\sqrt{64+36}\)

⇒  \(\sqrt{100}\)

10 Units

Question 3. Find the Distance Between the points (a,b) and (-b, a)

Solution:

Distance between the points (-b, a) and (a,b)

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

⇒  \(\sqrt{(a+b)^2+(b-a)^2}\)

⇒  \(\sqrt{a^2+b^2+2 a b+b^2+a^2-2 a b}\)

⇒  \(\sqrt{2 a^2+2 b^2}\)

⇒  \(\sqrt{2\left(a^2+b^2\right)} \text { units }\)

Question 4. Find the Distance Between the points (2a,3a) and (6a,6a)

Solution:

Distance Between the points (2a,3a) and (6a,6a)

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

⇒  \(\sqrt{(6 a-2 a)^2+(6 a-3 a)^2}\)

⇒  \(\sqrt{(4 a)^2+(3 a)^2}\)

⇒  \(\sqrt{16 a^2+9 a^2}\)

⇒  \(\sqrt{25 a^2}\)

5a Units

Question 5. Find the Distance Between the points (a,-b)

Solution:

Distance Between the points (a,-b) and (0,0)

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

⇒  \(\sqrt{(0-a)^2+(0+b)^2}\)

⇒  \(\sqrt{a^2+b^2} \text { units }\)

Question 6. Find the Distance Between the points (6,0) and (0,y) is 10 units, and find the value of y.

Solution:

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

⇒  \(\sqrt{(0-6)^2+(y-0)^2}\)

⇒  \(\sqrt{(6)^2+(y)^2}\)

⇒  \(\sqrt{36+y^2}\)

Given that,

⇒  \(\sqrt{36+y^2}\)

y²+36 = 100

y²= 100-36

y² = 64

y = √64

⇒  \(y= \pm 8\)

Question 7. Find the Distance Between the points (3,x) and (-2,-6) is 13 units, and find the value of x.

Solution:

Distance Between the points (-2,-6) and (3,x)

⇒  \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2}\)

⇒  \(\sqrt{(3+2)^2+(x+6)^2} \Rightarrow \sqrt{(5)^2+x^2+36+12 x}\)

⇒  \(\sqrt{25+x^2+36+12 x}\)

⇒  \(\sqrt{x^2+|2 x+61}\)

Given that,

⇒  \(\sqrt{x^2+|2 x+61}\) = 13

⇒  \({x^2+|2 x+61}\)= 169

x²+12x+61-169=0

x²+12x+108=0

x²-6x+18x-108=0

x(x-6)(x+18)

(x-6)(x+18)=0

x-6=0 or x+18=0

x=6 or x= -18

Question 8. Prove that the following points are the vertices of a right-angled triangle:

1. A(-2,2) B(13,11) and C(10,14)

Solution:

Let the points are A(-2,2) B(13,11) and C(10,14)

AB² = (13+2)²+(11-2)²

= (15)²+(9)²

= 225+81=306

BC² = (10-13)² + (14-11)²

= (3)² +(3)²

= 9+9=18

CA² = (10+2)²+(14-2)²

=(12)²+(12)²

=144+144=283

Therefore, AB = BC2+CA2

306=18+288

306=306

and AB² = BC²+ CA²

ΔABC is a right-angled triangle.

2. A(-1,-6), B(-9,10), ((-7, ()

Solution:

let the points are A(-1,-6), B(-9-10) and C(-7,6)

AB² = (9+1)² + (-10+6)²

= (-8)² + (-4) 2

= 64+16= 80

Bc²= (-7+9)² + (6+10)²

= (2) 4 (16) 2

= 4+256=260

AC²= (-7+1)² + (6+6)²

= (-6)²+(12)²

=36+144 = 180

Therefore 260=80+180

and, BC²= AB² +AC²

ΔABC is a right-angled triangle.

Question 9. Prove that the following points are the Vertices of an Isosceles right-angled triangle:

1. A(-8-9), B(0-3) and C(-6,5)

Solution: Let the points are A(-8-9), B(0,-3) and c(-6,5)

AB2 = (0+8) + (-3+9) 2

= (8)²+(6)²

= 64+36=100

BC² = (6=0)² + (5+3)²

= (-6)² +(8)²

= 36 +64 = 100

(A2= (6+8)² + (5+9)²

= (2)² + (14)²

= 4+196=200

Therefore, AB = BC = √100

and AC² = AB²+BC²

ΔABC is an isosceles right-angled triangle.

2. A (9-3), B(2,-1) and c(-2,-1)

Solution:

Let the points are A(9-3), B(2,-1) and c(-2,-1)

AB² = (2-0)² + (-1+3)²

=(2)²+(2)²

=4+4=8

BC² = (-2-2)² +(-1+1)²

(-4)²=16

Ac² = (2-0)² + (-1+3)²

= (-2)² + (2)²

=4+4=8

Therefore, AB=AC=√5

and BC² = AB² + AC²

AABC is an isosceles right-angled triangle.

Question 10. Prove that the points A(1,1), B(-1,-1) and C(√3,-√3) are the vertices of an equilateral triangle.

Solution:

Let the points are A(1, 1), B(-1,-1) and C(√3-√3)

⇒  \(AB=\sqrt{(-1-1)^2+(-1-1)^2}\)

⇒  \(AB=\sqrt{(-2)^2+(-2)^2}\)

\(AB=\sqrt{4+4}=\sqrt{8}=2 \sqrt{2}\)

⇒  \(BC=\sqrt{(\sqrt{3}+1)^2+(-\sqrt{3}+1)^2}\)

⇒  \(BC=\sqrt{3+1+2 \sqrt{3}+3+3-2 \sqrt{3}}\)

⇒  \(CA=\sqrt{(\sqrt{3}-1)^2+(-\sqrt{3}-1)^2}\)

⇒  \(CA=\sqrt{3+1-2 \sqrt{3}+3+1+2 \sqrt{3}}\)

⇒  \(CA=\sqrt{8}=2 \sqrt{2}\)

AB=BC=CA

Question 11. Prove that the points (-1,-2), (-2,-5), (-4-6), and (-3,-3) are the vertices of a Parallelogram.

Solution:

Let the points are A(-1,-2), B(-2,-5), c(-4,-6) and D(-3,-3)

⇒  \(AB=\sqrt{(-2+1)^2+(-5+2)^2}\)

⇒  \(AB=\sqrt{(-1)^2+(-3)^2}\)

⇒  \(AB=\sqrt{1+9}=\sqrt{10}\)

⇒  \(BC=\sqrt{(-4+2)^2+(-6+5)^2}\)

⇒  \(BC=\sqrt{(-2)^2+(-1)^2}\)

⇒  \(BC=\sqrt{4+1}=\sqrt{5} \)

⇒  \(CD=\sqrt{(-3+4)^2+(-3+6)^2}\)

⇒  \(CD=\sqrt{(1)^2+(3)^2}\)

⇒  \(CD=\sqrt{1+9}\)

⇒  \(CD=\sqrt{10}\)

⇒  \(AD=\sqrt{(-3+1)^2+(-3+2)^2}\)

⇒  \(AD=\sqrt{(-2)^2+(-1)^2}\)

⇒  \(AD=\sqrt{4+1}=\sqrt{5}\)

Therefore, AB = CD = √TO

BC = AD = √5

☐ ABCD is a parallelogram

Question 12. prove that the points (-4,-3), (3,2), (2,3) and (1,-2) are the vertices of a rhombus.

Solution:

let the points are ·A(-4,-3), B(-3,2), C(2,3) and (1,-2)

⇒  \(A B=\sqrt{(-3+4)^2+(2+3)^2}\)

⇒  \(A B=\sqrt{(1)^2+(5)^2}=\sqrt{1+25}=\sqrt{26}\)

⇒  \(B C=\sqrt{(2+3)^2+(3-2)^2}\)

⇒  \(B C=\sqrt{(5)^2+(1)^2}=\sqrt{25+1}=\sqrt{26}\)

⇒  \(C D=\sqrt{(1-2)^2+(-2-3)^2}\)

⇒  \(C D=\sqrt{(-1)^2+(-5)^2}=\sqrt{1+25}=\sqrt{26}\)

⇒  \(D A=\sqrt{(1+4)^2+(-2+3)^2}\)

⇒  \(D A=\sqrt{(5)^2+(1)^2}=\sqrt{25+1}=\sqrt{26}\)

⇒  \(A C=\sqrt{(2+4)^2+(3+3)^2}\)

⇒  \(A C=\sqrt{(6)^2+(9)^2}=\sqrt{36+81}=\sqrt{117}\)

⇒  \(B D=\sqrt{(1+3)^2+(-2-2)^2}\)

⇒  \(B D=\sqrt{(4)^2+(-4)^2}=\sqrt{16+16}=\sqrt{32}\)

Therefore, AB = BC= CD = DA = √26

AC and BD

〈〉 ABCD is a rhombus.

Question 13. Show that the following points are the vertices of a rectangle:

1. A(4,2), B(0,-4), c(-3,-2), D(14)

Solution.

Let the points are A (4,2), B(0,-4), C(-3,-2) and D(1,4)

⇒  \(A B=\sqrt{(0-4)^2+(-4-2)^2} \)

⇒  \(A B=\sqrt{(-4)^2+(-6)^2}=\sqrt{16+36}=\sqrt{52}\)

⇒  \(B C=\sqrt{(-3-0)^2+(-2+4)^2}\)

⇒  \(B C=\sqrt{(-3)^2+(-2)^2}=\sqrt{9+36} \sqrt{9+4}=\sqrt{13}\)

⇒  \(C D=\sqrt{(-3+1)^2+(4+2)^2}\)

⇒  \(C D=\sqrt{(-4)^2+(6)^2}=\sqrt{18+36}=\sqrt{52}\)

⇒  \(A D=\sqrt{(1-4)^2+(4-2)^2}\)

⇒  \(A D=\sqrt{(-3)^2+(2)^2}=\sqrt{9+4}=\sqrt{13}\)

Therefore, AB = CD=√52

BC=AD = √13

☐ ABCD is a rectangle

2. A(1,-1), B(2, 2), C(4,8), D(7,5)

Solution:

Let the points are A(1,-1), B(-2,2), c(4,8) and D(7,5)

⇒  \(A B=\sqrt{(-2-1)^2+(2+1)^2}\)

⇒  \(A B=\sqrt{(-3)^2+(3)^2}=\sqrt{9+9}=\sqrt{18}\)

⇒  \(B C=\sqrt{(4+2)^2+(8-2)^2}\)

⇒  \(B C=\sqrt{(6)^2+(6)^2}=\sqrt{36+36}=\sqrt{72}\)

⇒  \(C D=\sqrt{(7-4)^2+(5-8)^2}\)

⇒  \(C D=\sqrt{(3)^2+(3)^2}=\sqrt{9+9}=\sqrt{18}\)

⇒  \(A D=\sqrt{(7-1)^2+(5+1)^2}\)

⇒  \(A D=\sqrt{(6)^2+(6)^2}=\sqrt{36+36}=\sqrt{72}\)

Therefore, AB = CD= √18

BC=AD = √72

☐ ABCD is a rectangle

Question 14. Show that the points A(2,1),B(0,3), C(-2,1) and D(0,-1) are the Vertices of a Square.

Solution:

Let the points are A(2,1), B(0,3), C(-2, 1) and D(0,-1)

⇒  \(A B=\sqrt{(3-2)^2+(3-1)^2}\)

⇒  \(A B=\sqrt{(-2)^2+(2)^2}=\sqrt{4+4}=\sqrt{8}\)

⇒  \(B C=\sqrt{(-2-0)^2+(1-3)^2}\)

⇒  \(B C=\sqrt{(-2)^2+(-2)^2}=\sqrt{4+4}=\sqrt{8}\)

⇒  \(C D=\sqrt{(2)^2+(-2)^2}=\sqrt{4+4}=\sqrt{8}\)

⇒  \(A D=\sqrt{(0-2)^2+(-1-1)^2}\)

⇒  \(A D=\sqrt{(-2)^2+(-2)^2}=\sqrt{4+4}=\sqrt{8}\)

⇒  \(A C=\sqrt{(-2-2)^2+(1-1)^2}\)

⇒  \(A C=\sqrt{(-4)^2}=\sqrt{16}=4\)

⇒  \(B D=\sqrt{(0-0)^2+(-1-3)^2}\)

⇒  \(B D=\sqrt{(-4)^2}=\sqrt{16}=4\)

Therefore, AB = BC= CD=AD=√8

AC=BD=4

☐ ABCD is a Square

Question 15. Show that the points (1,1), (2,3) and (5,9) are collinear.

Solution:

⇒  \(A B=\sqrt{(2-1)^2+(3-1)^2}\)

⇒  \(A B=\sqrt{(1)^2+(2)^2}=\sqrt{1+4}=\sqrt{5}\)

⇒  \(B C=\sqrt{(9-3)^2+(5-2)^2}\)

⇒  \(B C=\sqrt{(6)^2+(3)^2}=\sqrt{36+9}=\sqrt{45}=\sqrt{9 \times 5}=3 \sqrt{5}\)

⇒  \(A C=\sqrt{(5-1)^2+(9-1)^2}\)

⇒  \(A C=\sqrt{(4)^2+(8)^2}=\sqrt{16+64}=\sqrt{80}\)

⇒  \(\sqrt{16 \times 5}=4 \sqrt{5}\)

Now, AB+BC = √5 +3√5 = 4√5 = AC

Points A, B, and care collinear.

Question 16. Show that the points (0,0), (5, 3), and (10,6) are Collinear.

Solution:

let the points are A(0,0), B(5, 3) and c(10,6)

⇒  \(A B=\sqrt{(5-0)^2+(3-0)^2}\)

⇒  \(A B=\sqrt{(5)^2+(3)^2}=\sqrt{25+9}=\sqrt{34} \)

⇒  \(B C=\sqrt{(10-5)^2+(6-3)^2}\)

⇒  \(B C=\sqrt{(5)^2+(3)^2}=\sqrt{25+9}=\sqrt{34}\)

⇒  \(A C=\sqrt{(10-0)^2+(6-0)^2} [/atex][/atex]\)

⇒  \(A C=\sqrt{(16)^2+(6)^2}=\sqrt{100+36}=\sqrt{136}=2 \sqrt{34}\)

Therefore, AB+BC= √34+ √34 = 2√34 = AC

Points A, B, and Care Collinear.

Question 17. Find the coordinates of a point that divides the line joining the points (5,3) and (10,8) in the ratio 2:3 internally.

Solution:

Let the Co-ordinates of the required point be (1,9).

Here, (X, Y1) = (5, 3) and (X2 Y2) = (10,8)

m : n = 2 : 3

⇒  \(x=\frac{m x_2+n x_1}{m+n}=\frac{2(10)+3(5)}{2+3}=\frac{20+15}{5}=\frac{35}{5}=7\)

⇒  \(y=\frac{m y_2+n y_1}{m+n}=\frac{2(8)+3(3)}{2+3}=\frac{16+9}{5}=\frac{25}{5}=45\)

Coordinates of required point = (7,5)

Question 18. Find the Coordinates of a point that divides the line joining the points (-1,2) and (3,5) in the ratio 3:5 internally.

Solution:

Let the Co-ordinates of the required point be (x, y)

Here, (X₁, y₁) = (-1,2) and (x₂,y₂)=(3,5)

m:n = 3:5

⇒  \(x=\frac{m x_2+n x_1}{m+n}=\frac{3(3)+5(-1)}{3+5}=\frac{9-5}{8}=\frac{4}{8}=\frac{1}{2}\)

⇒  \(y=\frac{m y_2+n y_1}{m+n}=\frac{3(5)+5(2)}{3+5}=\frac{15+10}{8}=\frac{25}{8}\)

Co-ordinates of required point \(=\left(\frac{1}{2}, \frac{25}{8}\right)\)

Question 19. Find the Co-ordinates of a point that divides the line. Segment joining the points (1,3) and (4,6) in the ratio 2:1 internally.

Solution:

Let the Co-ordinates required point be (x,y)

Here, (X1,Y1) = (1,3) and (X₂, y₂) = (-4,6)

m:n = 2:1

⇒  \(x=\frac{m x_2+n x_1}{m+n}=\frac{2(-4)+1(1)}{2+1}=\frac{-8+1}{3}=\frac{-7}{3}\)

⇒  \(y=\frac{m y_2+n y_1}{m+n}=\frac{2(6)+1(3)}{2+1}=\frac{12+3}{3}=\frac{15}{3}=5\)

Coordinates of required point \( =\left(-\frac{7}{3}, 5\right)\)

Question 20. If point A lies on the line segment joining the points P(6,0) and $(0,0) Such that AP: AQ = 2:3, find the coordinates of point A.

Solution:

Let the Co-ordinates require point A (1,4)

Here, (X₁,Y₁,) = (6,0) and (x₂,y₂) = (0,8)

m:n=2:3

⇒  \(x=\frac{m x_2+n x_1}{m+n}=\frac{2(0)+3(6)}{2+3}=\frac{18}{5}\)

⇒  \(y=\frac{m y_2+n y_1}{m+n}=\frac{2(8)+3(0)}{2+3}=\frac{16}{5}\)

Co-ordinates of required point A\(\left(\frac{18}{5}, \frac{16}{5}\right)\)

Question 21. Find the ratio in which the Y-axis divides the line segment joining the points (3,4) and (-2,5).

Solution:

Let Y-axis divide the join of pants (3,4) and (-2,1) in the ratio k:1.

⇒  \(\frac{k \cdot x_2-1 \cdot x_1}{k+1}=0 \)

⇒  \(\frac{k(-2)+1 \cdot(3)}{k+1}=0\)

⇒  \(\frac{-2 k+3}{k+1}=0 \)

⇒  \(-2 k+3=0 \)

⇒  \(-2 k=-3 \)

⇒  \(k=\frac{3}{2}\)

required ratio = 3:2

Question 22. Find the Co-ordinates of the mid-point of the line joining the following points:

1. (2,4) and (6,2)

Solution: Co-ordinates of the mid-point of AB = \(\left(\frac{2+6}{2}, \frac{4+2}{2}\right)=\left(\frac{8}{2}, \frac{6}{2}\right)=(4,3)\)

2. (0,2) and (2,-4)

Solution: Co-ordinates of mid-point of AB = \(\left(\frac{0+2}{2}, \frac{-4+2}{2}\right)=\left(\frac{2}{2}, \frac{-2}{2}\right)=(1,-1)\)

3. (a+b, a-b) and (b-a, a+b)

Solution: Co-ordinates of mid-point of AB= \(\left(\frac{a+b+b-a}{2}, \frac{a-b+a+b b}{2}\right)=\left(\frac{2 b}{2}, \frac{2 a}{2}\right)\) = (b,a)

4. (3,-5) and (-1,3)

Solution: Co-ordinates of mid-point of AB= \(=\left(\frac{3-1}{2}, \frac{-5+3}{2}\right)=\left(\frac{2}{2}, \frac{-2}{2}\right)=(1,-1)\)

Question 23. The coordinates of the endpoints of the diameter of a Circle are (3,-2) and (-3,6). Find the Co-ordinates of the Centre and radius.

Solution: Let the points be (3,-21) (-3,6)

⇒  \(\left(\frac{3-3}{2}, \frac{-2+6}{2}\right)\)

⇒  \(\left(0, \frac{4}{2}\right)=\left(0, \frac{4}{2}\right)\)

Center = (0,2)

Distance d = \(d=\sqrt{(-3-3)^2+(6+2)^2}\)

⇒  \(d=\sqrt{(-6)^2+(8)^2} \)

⇒  \(d=\sqrt{36+64}\)

⇒  \(d=\sqrt{100}=10\)

radius=\(\frac{\text { diameter }}{2}=\frac{10}{2}=5\)

Co-ordinates of the Centre (0,2) and radius = 5.

Question 24. The Co-ordinates of the vertices of a 4ABC are A(1, 0), B(3,6) and ((3,2). Find the length of its medians.

Solution:

let the points are A(1,0), B(3,6) and ((3,2)

let Ap be the median drawn from Vertex A.

The midpoint of BC is p.

Now, the Co-ordinates of P

⇒  \(\left(\frac{3+3}{2}, \frac{6+2}{2}\right)=\left(\frac{6}{2}, \frac{8}{2}\right)=(3,4)\)

⇒  \(AP =\sqrt{(3-1)^2+(4-0)^2}\)

⇒  \(\sqrt{(2)^2+(4)^2}\)

⇒  \(\sqrt{4+16} \)

⇒  \(\sqrt{20}\)

⇒  \(\sqrt{5 \times 4}=2 \sqrt{5}\)

let Bp be the median drawn from vertex Vertex B.

The mid-point Ac is p \(\left(\frac{1+3}{2}, \frac{0+2}{2}\right)=\left(\frac{4}{2}, \frac{2}{2}\right)=(2,1)\)

⇒  \(\text { and } B p=\sqrt{(2-3)^2+(1-6)^2}\)

⇒  \(\sqrt{(-1)^2+(-5)^2}\)

⇒  \(\sqrt{1+25}=\sqrt{26}\)

Let Cp be the median drawn from Vertex C.

The mid-point AB is P

⇒  \(\left(\frac{1+3}{2}, \frac{0+6}{2}\right)=\left(\frac{4}{2}, \frac{6}{2}\right)=(2,3)\)

and \(C p=\sqrt{(2-3)^2+(3-2)^2}\)

⇒  \(\sqrt{(-1)^2+(1)^2} \)

⇒  \(\sqrt{2}\)

Question 25. The coordinates of three consecutive vertices of a Parallelogram are (2,0), (4,1) and (6,4). Find the Coordinates of its 4th vertex.

Solution: Let A(2,0), B(4,1), c(6,4), and D(X, Y) be the vertex of a parallelogram ABCD.

We know that the diagonals of a parallelogram bisect each other.

Co-ordinates of the mid-point of AC = Co-ordinates of the mid-point of BD

⇒  \(\left.\left(\frac{2+6}{2}\right), \frac{0+4}{2}\right)=\left(\frac{4+x}{2}, \frac{1+4}{2}\right)\)

⇒  \(\left(\frac{8}{2}, \frac{4}{2}\right)=\left(\frac{4+x}{2}, \frac{1+y}{2}\right)\)

⇒  \((4,2)=\left(\frac{4+x}{2}, \frac{1+y}{2}\right)\)

⇒  \(4=\frac{4+x}{2} \text { and } 2=\frac{1+y}{2}\)

8=4+x and 4=1+y

x=4 and y=3

Co-ordinates of fourth vertex = (4,3)

Question 26. Find the Coordinates of the points of trisection of the line segment joining the points (2,5) and (6-2).
Solution:

Let P(a,b) and Q(c,d) trisect the line joining the points A(2,5) and B(6,-2).

Now, Point Pla,b) divides the line AB in the ratio 1:2.

⇒  \(a=\frac{1(2)+2(6)}{1+2}=\frac{2+12}{3}=\frac{14}{3}\)

⇒  \(b=\frac{1(5)+2(-2)}{1+2}=\frac{5-4}{3}=\frac{1}{3}\)

Therefore, co-ordinate of point p= \(\left(\frac{14}{3}, \frac{1}{3}\right)\)

Q(c,d) divides the line AB in the ratio 2:1

⇒  \(c=\frac{2(2)+1(6)}{2+3}=\frac{4+6}{3}=\frac{10}{3}\)

⇒  \(d=\frac{2(5)+1(-2)}{2+1}=\frac{10-2}{3}=\frac{8}{3}\)

Therefore, Co-ordinates of Q =\(\left(\frac{10}{3}, \frac{8}{3}\right)\)

Co-ordinates of points of trisection of AB \(=\left(\frac{14}{3}, \frac{1}{3}\right) \text { and }\left(\frac{10}{3}, \frac{8}{3}\right) \text {. }\)

Question 27. Find the Coordinates of the points of trisection of the line segment joining the points (-2,0) and (4,0).

Solution:

Let P(a,b) and Q(Cd) trisect the line joining the points A(-2,0) and B(4,0).

Now, Point (a,d) divides the line AB in the ratio 1:2.

⇒  \(a=\frac{1(-2)+2(4)}{1+2}=\frac{-2+8}{3}=\frac{6}{3}=2\)

⇒  \(b=\frac{1(0)+2(0)}{1+3}=0\)

Therefore, the Co-ordinate of point P = (2,0)

Q(c,d) divides the line AB in the ratio 2:1

⇒  \(c=\frac{2(-2)+1(4)}{1+2}=\frac{-4+4}{3}=0\)

⇒  \( d=\frac{2(0)+1(0)}{1+2}=0\)

Therefore, Co-ordinates of Q = (0,0)

Co-ordinates of points of trisection of AB =(2,0) and (0,0)

Question 28. Find the ratio in which the join of points (3,-1) and (8,9) is divided by the line y-x+2=0.

Solution:

let the line y-x+2=0 divide the line segment joining the points (3-1) and (8,9) in the ratio k:1.

Co-ordinates of p= \(\left(\frac{8 k+3}{k+1}, \frac{9 k-1}{k+1}\right)\)

but this point p lies on the line y-x+2=0

⇒  \(\frac{9 k-1}{k+1}-\frac{8 k+3}{k+1}+2=0\)

9 k-1-8 k+3+2 k+2=0

3k=2

⇒  \(k=\frac{2}{3}\)

Required ratio \(=\frac{2}{3}\):1 – 2:3

Question 29. Find the area of that triangle whose vertices are (2,3), (-3,4), and (7,5).

Solution:

Area of triangle =\(\frac{1}{2}\left[x_1\left(y_2-y_3\right)+x_2\left(y_3-y_1\right)+x_3\left(y_1-y_2\right)\right]\)

⇒  \(\frac{1}{2}[2(4-5)-3(5-3)+7(3-4)]\)

⇒  \(\frac{1}{2}[2(-1)-3(2)+7(-1)]\)

⇒  \(\frac{1}{2}[-2-6-7]\)

⇒  \(\frac{-15}{2}\)

But the area of the triangle Cannot be negative

Area of triangle = \(\frac{-15}{2}\) Square units

Question 30. Find the area of that triangle whose vertices are (1,1), (-1,4) and (3,2).

Solution:

Area of triangle=\( \frac{1}{2}\left[x_1\left(y_2-y_3\right)+x_2\left(y_3-y_1\right)+x_3\left(y_1-y_2\right)\right]\)

⇒  \(\frac{1}{2}[1(4-2)-1(2-1)+3(1-4)]\)

⇒  \(\frac{1}{2}(1(2)-1(1)+3(-3)]\)

⇒  \(\frac{1}{2}[2-1-9]\)

⇒  \(\frac{1}{2}[-8]\)

= -4

But the area of the triangle Cannot be negative.

Area of triangle = 4 Square units.

Question 31. Find the area of that triangle whose vertices are (5,2), (-4,3), and (-2,1)

Solution:

Area of triangle = \( \frac{1}{2}\left[x_1\left(y_2-y_3\right)+x_2\left(y_3-y_1\right)+y_3\left(y_1-y_2\right)\right] \)

⇒  \(\frac{1}{2}[5(3-1)-4(1-2)-2(2-3)] \)

⇒  \(\frac{1}{2}[5(2)-4(-1)-2(-1)]\)

⇒  \(\frac{1}{2}[10+4+2]\)

⇒  \(\frac{16}{2}=8\)

Question 32. Find the area of that triangle whose vertices are (b+c, a), (b-ca), and (9, -a),

Solution:

Area of triangle =\( \frac{1}{2}\left[x_1\left(y_2-y_3\right)+x_2\left(y_3-y_1\right)+x_3\left(y_1-y_2\right)\right]\)

⇒  \(\frac{1}{2}[b+c(a+a)+b-c(-a-a)+a(a-a)]\)

⇒  \( \frac{1}{2}\left[b a+b b+c a+c a-b b-b a+c a+c a+a^2-\alpha^2\right]\)

⇒  \( \frac{1}{2}[4 a c]\)

= 2ac

Area of triangle = 2ac Square units

Question 33. Prove that the following points are Collinear:

1. (2,1), (4,3), and (3,2)

Solution: Area of triangle = \( \frac{1}{2}\left[x_1\left(y_2-y_3\right)+x_2\left(y_3-y_1\right)+x_3\left(y_1-y_2\right)\right]\)

⇒  \( \frac{1}{2}[2(3-2)+4(2-1)+3(1-3)]\)

⇒  \( \frac{1}{2}[2(1)+4(1)+3(-2)]\)

⇒  \( \frac{1}{2}[2+4-6]\)

⇒  \( \frac{1}{2}[6-6]\)

⇒  \( \frac{1}{2}(0)=0\)

Therefore, the given points are collinear.

2. (9,6), (1,6) and (-7,-6)

Solution: Area of triangle =\( \frac{1}{2}\left[x_1\left(y_2-y_3\right)+x_2\left(y_3-y_1\right)+x_3\left(y_1-y_2\right)\right]\)

⇒  \(\frac{1}{2}[9(0+6)+1(-6-6)-7(6-0)]\)

⇒  \(\frac{1}{2}[9(6)+12-7(6)]\)

⇒  \(\frac{1}{2}[54-12-42]\)

⇒  \(\frac{1}{2}[54-54]\)

⇒  \(\frac{1}{2}(0)=0\)

Therefore, the given points are collinear

3. (b+c, 2), (c+a, b), and (a+b, c)

Solution: Area of triangle = \( \frac{1}{2}\left[x_1\left(y_2-y_3\right)+x_2\left(y_3-y_1\right)+x_3\left(y_1-y_2\right)\right]\)

⇒  \( \frac{1}{2}[b+c(b-c)+c+a(c-a)+a+b(a-b)]\)

⇒  \( \frac{1}{2}\left[b^2-b c+c b-c^2+c^2-ca+ac-a^2+ a^2-ab+ba-b^2\right]\)

⇒  \( \frac{1}{2}\)

These fore, the given points are collinear.

4. (5,6), (-1,4) and (2,5)

Solution:

Area of triangle =\( \frac{1}{2}\left[x_1\left(y_2-y_3\right)+x_2\left(y_3-y_1\right)+x_3\left(y_1-y_2\right)\right]\)

⇒  \(\frac{1}{2}[5(4-5)-1(5-6)+2(6-4)]\)

⇒  \(\frac{1}{2}[5(-1)-1(-1)+2(2)]\)

⇒  \(\frac{1}{2}[-5+1+4]\)

⇒  \(\frac{1}{2}[-5+5]\)

⇒  \(\frac{1}{2}(0)=0\)

Therefore given points are Collinear.

Question 34.1. If the points (2,3), (5,k), and (6,7) are Collinear, find the value of k.

Solution: Given points are Collinear

Area of triangle = 0

⇒  \(\frac{1}{2}[2(k-7)+5(7-3)+6(3-7)\)

⇒  \(\frac{2}{2}[2 k-14+20-24]=0\)

⇒  \(\frac{1}{2}[2 k-13]=0\)

⇒  2 k-18=0

⇒  2 k=17

⇒  \(k=\frac{18}{2}\)

⇒  K = 9

2. If the points A(k+1,2k), B(3k, 2k+3) and C(SK-1,5k) are collinear, find the Value of k.

Solution: Given points are Collinear

Area of Triangle = 0

⇒  \(\frac{1}{2}[k+1(2 k+3-5 k)+3 k(5 k-2 k)+5 k-1(2 k-2 k-3)]=0\)

⇒  \(\frac{1}{2}[k+1(-3 k+3)+3 k(3 k)+5 k-1(-3)]=0\)

⇒  \(\frac{1}{2}\left[-3 k^2-3 k+3 k+3+9 k^2-15 k+3\right]=0\)

⇒  \(\frac{1}{2}\left[6 k^2-15 k+6\right]=0 \)

⇒  \(\frac{3}{2}\left[2 k^2-5 k+2\right]=0\)

⇒  2x²=5k+2=0

⇒  2k²-4k-k+2=0

⇒  2k(k-2)-(K-2)=0

⇒ (2k-1) (k-2)=0

⇒  2k-1=0 and K-2=0

⇒  2k = 1

⇒  k= \(\frac{1}{2}\)

Question 35. If the points (x, y), (-1,3) and (5,-3) are Collinear, then Show that x+y=2.

Solution:

Given points are Collinear

Area of triangle = 0

⇒  \(\frac{1}{2}[x(3+3)-1(-3-y)+5(y-3)]=0 \)

⇒  \(\frac{1}{2}[x(6)+3+y+5 y-3(5)=0\)

⇒  \(\frac{1}{2}[6 x+6 y-12]=0\)

⇒  \(\frac{6(x+y-2)}{2}=0\)

⇒  x+y=2

Question 36. Find the Values of y for which the distance between the points A(3,-1) and B(11,y) is 10 units.

Solution:

Distance between the points A(3,-1) B(11,y)

⇒  \(AB =\sqrt{(11-3)^2+(y+1)^2}=10\)

⇒  \((8)^2+y^2+1+2 y=100\)

⇒  \( 64+y^2+1+2 y-100=0 \)

⇒  \( y^2+2 y-35=0\)

⇒  \(y^2-5 y+7 y-35=0\)

⇒  y(y-5)+7(9-5)=0

⇒   (y+7) (4-5)=0

⇒  4+7=0 or 4-5=0

⇒  y=-7 or y=5

Question 37. Find the relation between x and y Such that the point p(x,y) is equidistant from the points A(1,4) and B (-1,2).

Solution:

Given that P(x,y) A(1,4) and B(-12)

⇒  PA= PB ⇒ PA²+ PB²

⇒  \( (x-1)^2+(y-4)^2=(y+1)^2+(y-2)^2 \)

⇒  \(x^2+\left(-2 x+y^2+16-8 y=x^2+1+2 x+y^2+4-4 y\right.\)

⇒  -2x-8y+17-2x+4y-5=0

⇒  -4x-4y+12=0 -4(x+y-3)=0

⇒  x+4=3

Question 38. Find the point on the y-axis which is equidistant from the points (-512) and (9,-2).

Solution:

let the required point on the y-axis be p(0,4) and the given points be A(-5,2) and B(9,-2).

Now, given that

PA=PB ⇒ PA² = PB²

⇒  (0+5)² + (9-2)² = (6-9) + (Y+2)²

⇒  25+4 + 4-4y= 81+ y²+4+499

⇒  -44+29-44-85= 0

⇒  -8y-16=0

⇒  -8y=16

⇒  \(y=\frac{-16}{8}\)

y= -2

Question 39. Show that the pants (1,1), (1,5), (7,9) and (9,5) taken in that order, are the Vertices of a rectangle

Solution:

Given points are A(1, 1), B(-1,5), ((7,9) and D(9,5)

⇒  \(A B=\sqrt{(-1-1)^2+(5-1)^2}\)

⇒  \(\sqrt{(-2)^2+(4)^2}=\sqrt{4+16}=\sqrt{20}\)

⇒  \(B C=\sqrt{(7+1)^2+(9-5)^2}\)

⇒  \(\sqrt{(8)^2+(4)^2}=\sqrt{64+16}=\sqrt{80}\)

⇒  \(C D=\sqrt{(9-7)^2+(5-9)^2}\)

⇒  \(\sqrt{(2)^2+(-4)^2}=\sqrt{4+16}=\sqrt{20}\)

⇒  \(A D=\sqrt{(9-1)^2+(5-1)^2}\)

⇒  \(\sqrt{(8)^2+(4)^2}=\sqrt{64+(6}=\sqrt{80}\)

⇒  \(A B=C D=\sqrt{20}\)

⇒  \(B C=A D=\sqrt{80}\)

☐ ABCD is a rectangle.

Question 40. Show that the points ‘A (3,5), B( 6,01, C(,-3), and D(-2,2) are the Vertices of a Square ABCD.

Solution:

Given that

⇒  \(A B=\sqrt{(6-3)^2+(0-5)^2}=\sqrt{(3)^2+(-5)^2}=\sqrt{9+25}=\sqrt{34}\)

⇒  \(B C=\sqrt{(1-6)^2+(-3-0)^2}=\sqrt{(-5)^2+(-3)^2}=\sqrt{25+9}=\sqrt{34}\)

⇒  \(C D=\sqrt{(-2-1)^2+(2+3)^2}=\sqrt{(-3)^2+(5)^2}=\sqrt{9+25}=\sqrt{34}\)

⇒  \(A D=\sqrt{(-2-3)^2+(2-5)^2}=\sqrt{(-5)^2+(-3)^2}=\sqrt{25+9}=\sqrt{34}\)

⇒  \(A C=\sqrt{(1-3)^2+(-3-5)^2}=\sqrt{(-2)^2+(-8)^2}=\sqrt{4+64}=\sqrt{68}\)

⇒  \(B D=\sqrt{(-2-6)^2+(2-0)^2}=\sqrt{(-8)^2+(2)^2}=\sqrt{64+4}=\sqrt{68}\)

⇒  \(A B=B C=C D=A B=\sqrt{34}\)

⇒  \(A C=B D=\sqrt{63}\)

Arithmetic Progression

Question 1. The nth term of a Sequence In defined as follows. Find the first four terms:

1. a_n=3n+1

Solution:

Given: a_n=3n+1

3n=3nt

Put n= 1,2,3,4, we get

a₁ = 3×1+1=4

a₂ = 3×2+1=7

a₃ = 3×3 +1 = 10

a₄=3×4+1= 13

The first four terms of the Sequence are 4,7, 10,13.

2. a_n= n²+3

Solution:

Given:

a_n=n²+3

Put n=1,2,3,4 we get

a1= (1)2+3 = 4

a₂=(2)2 +3= 7

a₃ = (3)2+3 = 12

Read and Learn More Class 10 Maths

a₄=(4)2 +3= 19

The first four terms of the Sequence are 4, 7, 12, and 19.

3. a_n = n(n+1)

Solution:

a_n=n (n+1)

Put n= 1,2,3,4 we get

a₁ = 1(1+1)=2

a₂ = 2(2+1)=6

a₃=3(3+1)= (2

a₄ = 4(4+1)=20

The first four terms of the Sequence are 2,6,12,20

4.\(a_n=n+\frac{1}{n}\)

Solution:

Given:

⇒ \(a_n=n+\frac{1}{n}\)

Put n=1,2,3,4 we get

⇒ \(a_1=1+\frac{1}{1}=2 \)

⇒ \(a_2=2+\frac{1}{2}=\frac{4+1}{2}=\frac{5}{2} \)

⇒ \(a_3=3+\frac{1}{3}=\frac{9+1}{3}=\frac{10}{3} \)

⇒ \(a_4=4+\frac{1}{4}=\frac{16+1}{3}=\frac{17}{3}\)

First four terms of the Sequence are 2,\( \frac{5}{2}, \frac{10}{3}, \frac{17}{3}\)

5. an= 3_n

Solution:

Given

a_n=3_n

Put n=1,2,3,4 we get

a₁=31=3

a₂=32 = 9

a₃=33=27

a₄=34=81

The first four terms of the Sequence are 3, 9, 27, 81

Question 2. The nth term of a Sequence is (3n-7). Find its 20th term.

Solution:

3n-7

n=20

3(20)-7

60-7

57

The 20^th term of a Sequence is 57

Question 3. Which of the following are A.p.’s? If they form an A.P., find the Common difference ‘d’ and write three more terms:

1. -10, -6, -2, 2,…….

Solution:

Here a=-10,

d=-6-10=4

-10, -6, -2, 2,4,6,10,14

Yes d= 4, next three items = 6, 10, 14

2. 3,3+ √2, 3+252, 3+3√2,…..

Solution:

Here a=3

d=3+√2-3 =) √2

3, 3+ √2, 3+2√2, 3+3√2, 3+4√2,3+5√2

Yes d=52, next three terms = 3+3√2, 3+4√2,3+5√2.

3. 0, -4, -8,-12,

Solution:

Here a=0

d=0-4=-4

0,-4,-8,-12-16,-20,-24

Yes do, next three terms = -16,-20,-24

Question 4. For the following A.-P. ‘S write the first terms and Common differences:

1. 2,5, 8, 11,..

Solution:

2, 5, 8, 11,

Her first term a=2

Common difference = 5-2 = 3

2.  -5,-1, 3, 7,

Solution: -5,-1, 3, 7

Her first term a=-5

Common difference = -541 = 4

Question 5. write the first four terms of the Ap., when the first term ‘a’ and the Common difference ‘d’ are given as follows:

1.  a=5, d=3

Solution:

a=5, d= 3

a1 =5

a2=5+3=8

a3=8+3=11

a4=11+3= 14

The first four terms are 5, 8, 11, 14

2.  a=-2,d=4

Solution:

a=-2, d=4

a₁ =-2

a₂=-2+4=2

a₃=2+4=6

a₄=6+4= 10

The first four terms are -2,2,6,10

Question 6. Find the 10^th term of the AP: 1,3,5,7,..

Solution:

Here, a=1

d=3-1=2,

n = 10

a_n = a+ (n-1)

90= 1+ (10-1)2

⇒96=1+18

⇒ 210=19

10^th term of the given Ap=19

Question 7. Find the 7^th term of the AP 80, 77,74,71,

Solution:

Here a=80

d=77-80=-3,

n=7

a₇ = a + (n-1)d

a₇=80+(7-1)-3

a₇=80-18

⇒ a₇ = 62

7^th term of the given A.p. =62

Question 8. Find the n^th term of the A⋅p: -5, -3, -1, 1, —-

Solution:

Here a = -5

d=-3-5=2

n = n

a_n = a+ (n-1) d

a_n=-5+ (n-1)2

⇒ a_n=-5+2n-2

⇒ a_n= 2n-7

n^th term of the given A.p. = (2n-7)

Question 9. Which term of the A-P. 4, 8, 12, is 76?

Solution:

Here, a = 4,

d=8-4=4

Let an=76

=) 4+ (n-1)4=76

= 4+4n-4=76

4n=76

⇒ \(n=\frac{76}{4} \Rightarrow n=19\)

19^thterm of the given A.P. is 76

Question 10. which term of the Ap. 36, 33, 30, is Zero?

Solution:

Here a=36

d=33-36=-3

let an = 0

36+ (n-1)-3=0

36-3n+3=0

-3n=-39

\(n=\frac{39}{3} \Rightarrow n=13\)

The 13^th term of the given A.P. is zero

Question 11. which term of the\(\frac{3}{4}, 1, \frac{5}{4}, \ldots \text {. is } 12 ?\)

Solution:

⇒ \(\text { Here } a=\frac{3}{4} \)

⇒ \(d=1-\frac{3}{4}=\frac{1}{4} \)

⇒ \(\text { let } a_n=12 \)

⇒ \(\frac{3}{4}+(n-1) \frac{1}{4}=12 \)

⇒ \(\frac{3+(n-1)}{4}=12\)

3+n-1=48

⇒ n+2=48

⇒n=46

46^th term of the given AP is 12.

Question 12. Find the number of terms in the Ap. 8, 12, 16,

Solution:

Here a=8

d=12-8=) 4

let an=124

=) 8+ (n-1)4=124

=) 8+40-4=124

=) 4n=124-4

4n = 120

⇒ n = \(n=\frac{120}{4} \Rightarrow 30\)

30^th term of the given A.P. is 124.

Question 13. Find the number of terms in the A.p. 75, 70, 65, 15

Solution:

Here a=75

d=70-75=-5

let an=15

75+ (n-1)-5=15

75-5n+5=15

70-5n=15

-517=15-70

-5n=-55 ⇒ n=11

11^th term of the given A. p. is 15.

Question 14. Find the 10th term from the end of the A.P. 82, 79, 76, —-,4.

Solution:

Here aa 1=4

d=79-82=-3

n=10

(-(10-1)defined

– 4-(10-1)-3

=4727

=31

10^th term from the end = 31

Question 15. Find the 16th term from the end of the A.P. 3,6,9,99

Solution:

Here, 1=99

d= 6-3 = 3,

n=16

⇒ 99-(16-1) 3

⇒ 99-45

⇒ 54

16^th  term from the end = 54

Question 16. Find the Sum of the following A.p.: 3,8, 13, to 20 terms

Solution:

S₁ =3+8+13,

a₁=3

d=8-3=5

n=20

⇒ \(S_n=\frac{n}{2}[2 a+(n-1) d]] \)

⇒ \(S_{20}=\frac{20}{2}[2(3)+(20-1) 5]\)

⇒ \(S_{20}=10[6+95]\)

⇒ \(S_{20}=10[101]\)

⇒ \(S_{20}=1010 \)

Question 17. Find the sum of the following A.p. 5: 1,4,7,– to 50 terms

Solution:

S = 1+4+7+—–50

a=1

d=4-1= 3

n=50

⇒ \(S_n=\frac{n}{2}[2 a+(n-1) d] \)

⇒ \(S_{50}=\frac{50}{2}[2(1)+(50-1) 3] \)

⇒ \(S_{50}=25[2+147] \)

⇒ \(S_{50}=25[(49]\)

⇒ \(S_{50}=3,725\)

Question 18. Find the Sum given below: 3+6+9+ …..+96

Solution:

S=3+6+9+…… +96

a₁ =3, d= 6-3 =) 3

an=96

a₁+ (n-1) d=96

3+(n-1)3=96

3+3n-3=96

3n=96

⇒ \(n=\frac{96}{3} \Rightarrow n=32\)

S1 = Sum of 32 terms with first 3 terms and last term 96

⇒ \(S_1=\frac{32}{2}[3+96]\)

⇒ \(S_1=\frac{32}{2}[99]\)

⇒ \(S_1=16[99]\)

⇒ \(S_1=1584\)

Question 19. Find the Sum given below! 2 + 4+ 6+.

Solution:

S=2+4+6+—–+50

a₁ =2,

d=4-2 =) 2 Q1=2,

an=50

a₁+ (n-1)d=50

2+(n-1)2=50

2+2n=2=50

⇒ \(n=\frac{50}{2} \Rightarrow n=25\)

S₁ = Sum of 25 terms with first 2 terms and last terms so

⇒ \(S_1=\frac{50}{2}[2+50] \)

⇒ \(S_1=\frac{50}{2}[526] \)

⇒ \(S_1=50[26] \)

⇒ \(S_1=650\)

Question 20. In an A.p.: given a=2, d= 3, Qn = 50, find n and Sn.

Solution:

Given a=2, d=3, an =50

a+(n-1)d=an

2+(n-1)3=50

2+3n-3=50

3n=5041

⇒ \(n=\frac{51}{3} \Rightarrow n=17 \)

⇒ \(S_n=\frac{n}{2}[2 a+(n-1) d] \)

⇒ \(5_n=\frac{17}{2}[2(2)+(17-1) 3] \)

⇒ \(S_n=\frac{17}{2}[2(2)+(17-1) 3] \)

⇒ \(S_n=\frac{17}{2}[4+48] \)

⇒ \(S_n=\frac{17}{2}\left[S_2\right] \)

⇒ \(S_n=17[26] \)

⇒ \(S_n=442\)

Question 21. Find the Value of x for which (x+2), 2x, (2x+3) are three consecutive terms of A.P.

Solution:

(x+2), 2x, (2x+3) are three consecutive terms of A.p.

\(2 x=\frac{(x+2)+(2 x+3)}{2}\)

4x= x+2+2x+3

4x=3x+5

4x-3x=5

x=5