Haritha

Probability

Question 1. Given that E and F are events such that P(E)=0.6, P(F)=0.3 and \(\mathrm{P}(\mathrm{E} \cap \mathrm{F})=0.2\), find \(\mathrm{P}(\mathrm{E} \mid \mathrm{F})\) and \(P(F \mid E)\).
Solution:

It is given that P(E)=0.6, P(F)=0.3, and \(P(E \cap F)=0.2\)

⇒ \(P(E \mid F)=\frac{P(E \cap F)}{P(F)}=\frac{0.2}{0.3}=\frac{2}{3}\)

⇒ \(P(F \mid E)=\frac{P(F \cap E)}{P(E)}=\frac{0.2}{0.6}=\frac{1}{3}\)

Question 2. Compute \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})\), if \(\mathrm{P}(\mathrm{B})=0.5\) and \(\mathrm{P}(\mathrm{A} \cap \mathrm{B})=0.32\)
Solution:

It is given that P(B)=0.5 and \(P(A \cap B)=0.32 \Rightarrow P\left(\frac{A}{B}\right)=\frac{P(A \cap B)}{P(B)}=\frac{0.32}{0.5}=\frac{16}{25}\)

Question 3. If P(A)=0.8, P(B)=0.5 and \(P(B \mid A)=0.4\), find

1. \(\mathrm{P}(\mathrm{A} \cap \mathrm{B})\)
2. \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})\)
3. \(\mathrm{P}(\mathrm{A} \cup \mathrm{B})\)

Solution:

It is given that P(A)=0.8, P(B)=0.5, and \(P(B \mid A)=0.4\)

1. \(\mathrm{P}(\mathrm{B} \mid \mathrm{A})=0.4\)

∴ \(\frac{P(B \cap A)}{P(A)}=0.4\)

(because \(\mathrm{P}\left(\frac{\mathrm{B}}{\mathrm{A}}\right)=\frac{\mathrm{P}(\mathrm{B} \cap \mathrm{A})}{\mathrm{P}(\mathrm{A})}\))

∴ \(\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{0.8}=0.4 \Rightarrow \mathrm{P}(\mathrm{A} \cap \mathrm{B})=0.32\)

(because \(B \cap A=A \cap B\))

2. \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})=\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})}\)

(because \(\mathrm{P}\left(\frac{\mathrm{A}}{\mathrm{B}}\right)=\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})}\))

⇒ \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})=\frac{0.32}{0.5}=0.64\)

3. \(\mathrm{P}(\mathrm{A} \cup \mathrm{B})=\mathrm{P}(\mathrm{A})+\mathrm{P}(\mathrm{B})-\mathrm{P}(\mathrm{A} \cap \mathrm{B}) \Rightarrow \mathrm{P}(\mathrm{A} \cup \mathrm{B})=0.8+0.5-0.32=0.98\)

Read and Learn More Class 12 Maths Chapter Wise with Solutions

Question 4. Evaluate \(\mathrm{P}(\mathrm{A} \cup \mathrm{B})\), if 2P(A)=P(B)=\(\frac{5}{13}\) and \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})=\frac{2}{5}\)
Solution:

It is given that, \(2 P(A)=P(B)=\frac{5}{13} \Rightarrow P(A)=\frac{5}{26}\) and \(P(B)=\frac{5}{13}\)

Now, \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})=\frac{2}{5} \Rightarrow \frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})}=\frac{2}{5}\)

(because \(\mathrm{P}\left(\frac{\mathrm{A}}{\mathrm{B}}\right)=\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})}\))

⇒ \(\mathrm{P}(\mathrm{A} \cap \mathrm{B})=\frac{2}{5} \times \mathrm{P}(\mathrm{B})=\frac{2}{5} \times \frac{5}{13}=\frac{2}{13}\)

It is known that, \(\mathrm{P}(\mathrm{A} \cup \mathrm{B})=\mathrm{P}(\mathrm{A})+\mathrm{P}(\mathrm{B})-\mathrm{P}(\mathrm{A} \cap \mathrm{B})\)

⇒ \(\mathrm{P}(\mathrm{A} \cup \mathrm{B})=\frac{5}{26}+\frac{5}{13}-\frac{2}{13} \Rightarrow \mathrm{P}(\mathrm{A} \cup \mathrm{B})=\frac{5+10-4}{26} \Rightarrow \mathrm{P}(\mathrm{A} \cup \mathrm{B})=\frac{11}{26}\)

Question 5. If \(P(A)=\frac{6}{11}, P(B)=\frac{5}{11}\) and \(P(A \cup B)=\frac{7}{11}\), find

1. \(\mathrm{P}(\mathrm{A} \cap \mathrm{B})\)
2. \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})\)
3. \(\mathrm{P}(\mathrm{B} \mid \mathrm{A})\)

Solution:

It is given that \(P(A)=\frac{6}{11}, P(B)=\frac{5}{11}\) and \(P(A \cup B)=\frac{7}{11}\)

1. P(A ∪ B)=\(\frac{7}{11}\) (because \(P(A \cup B)=P(A)+P(B)-P(A \cap B)\))

∴ \(P(A)+P(B)-P(A \cap B)=\frac{7}{11}\)

⇒ \(\frac{6}{11}+\frac{5}{11}-P(A \cap B)=\frac{7}{11}\)

⇒ \(P(A \cap B)=\frac{11}{11}-\frac{7}{11}=\frac{4}{11}\)

2. It is known that, \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})=\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})} \Rightarrow \mathrm{P}(\mathrm{A} \mid \mathrm{B})=\frac{\frac{4}{11}}{\frac{5}{11}}=\frac{4}{5}\)

3. It is known that, \(\mathrm{P}(\mathrm{B} \mid \mathrm{A})=\frac{\mathrm{P}(\mathrm{B} \cap \mathrm{A})}{\mathrm{P}(\mathrm{A})} \Rightarrow \mathrm{P}(\mathrm{B} \mid \mathrm{A})=\frac{\frac{4}{11}}{\frac{6}{11}}=\frac{4}{6}=\frac{2}{3}\)

Determine P(E | F)

Question 6. A coin is tossed three times, where

1. E: head on third toss, F: heads on first two tosses
2. E: at least two heads, F: at most two heads
3. E: at most two tails, F: at least one tail

Solution:

If a coin is tossed three times, then the sample space S is S = {HHH, HHT, HTH, HTT, THH, THT, TTH, TTT}

⇒ n(S) = 8

1. E = {HHH, HTH, THH, TTH}

F = {HHH, HHT}

∴ E ∩ F = {HHH}

∴ P(F) = \(\frac{2}{8}=\frac{1}{4} \text { and } P(E \cap F)=\frac{1}{8}\)

∴ \(P(E \mid F)=\frac{P(E \cap F)}{P(F)}=\frac{\frac{1}{8}}{\frac{1}{4}}=\frac{4}{8}=\frac{1}{2}\)

2. E = {HHH, HHT, HTH, THH}

F = {HHT, HTH, HTT, THH, THT, TTH, TTT}

∴ E ∩ F = {HHT, HTH, THH}

Clearly, \(\mathrm{P}(\mathrm{E} \cap \mathrm{F})=\frac{3}{8}\) and \(\mathrm{P}(\mathrm{F})=\frac{7}{8}\)

∴ \(\mathrm{P}(\mathrm{E} \mid \mathrm{F})=\frac{\mathrm{P}(\mathrm{E} \cap \mathrm{F})}{\mathrm{P}(\mathrm{F})}=\frac{\frac{3}{8}}{\frac{7}{8}}=\frac{3}{7}\)

3. E = {HHH, HHT, HTT, HTH, THH, THT, TTH}

F = {HHT, HTT, HTH, THH, THT, TTH, TTT}

∴ E ∩ F = {HHT, HTT, HTH, THH, THT, TTH}

⇒ \(\mathrm{P}(\mathrm{F})=\frac{7}{8}\) and \(\mathrm{P}(\mathrm{E} \cap \mathrm{F})=\frac{6}{8}\)

Therefore, \(\mathrm{P}(\mathrm{E} \mid \mathrm{F})=\frac{\mathrm{P}(\mathrm{E} \cap \mathrm{F})}{\mathrm{P}(\mathrm{F})}=\frac{\frac{6}{8}}{\frac{8}{8}}=\frac{6}{7}\)

Question 7. Two coins are tossed once, where:

1. E: tail appears on one coin, F: one coin shows the head
2. E: no tail appears, F: no head appears

Solution:

If two coins are tossed once, then the sample space S is S = {HH, HT, TH, TT}

1. E = {HT, TH}, F = {HT, TH}

∴ E ∩ F = {HT, TH}

⇒ \(\mathrm{P}(\mathrm{F})=\frac{2}{4}=\frac{1}{2}\)

∴ \(\mathrm{P}(\mathrm{E} \cap \mathrm{F})=\frac{2}{4}=\frac{1}{2} \Rightarrow \mathrm{P}(\mathrm{E} \mid \mathrm{F})=\frac{\mathrm{P}(\mathrm{E} \cap \mathrm{F})}{\mathrm{P}(\mathrm{F})}=\frac{\frac{1}{2}}{\frac{1}{2}}=1\)

2. E = {HH} [Set of events having no tail]

F = {TT} [Set of events having no head]

∴ \(E \cap F=\phi\)

P(E) = \(\frac{1}{4}, P(F)=\frac{1}{4}\) and \(P(E \cap F)=0\)

∴ \(P(E \mid F)=\frac{P(E \cap F)}{P(F)}=\frac{0}{1 / 4}=0\)

Question 8. A die is thrown three times, E: 4 appears on the third toss, F: 6 and 5 appear respectively on the first two tosses
Solution:

If a die is thrown three times, then the number of elements in the sample space will be 6x6x6 = 216 The sample space is S = {(x, y, z): x, y, z ∈ 1,2, 3, 4, 5, 6}

E = \(\left\{\begin{array}{l}
(1,1,4),(1,2,4), \ldots . .(1,6,4) \\
(2,1,4),(2,2,4), \ldots \ldots(2,6,4) \\
(3,1,4),(3,2,4), \ldots \ldots(3,6,4) \\
(4,1,4),(4,2,4), \ldots \ldots(4,6,4) \\
(5,1,4),(5,2,4), \ldots \ldots(5,6,4) \\
(6,1,4),(6,2,4), \ldots \ldots . .(6,6,4)
\end{array}\right\} \Rightarrow n(E)=36\)

F = {(6, 5, 1), (6, 5, 2), (6, 5, 3), (6, 5, 4), (6, 5, 5), (6, 5, 6)}

∴ \(E \cap F=\{(6,5,4)\} \Rightarrow n(E \cap F)=1\)

P(F) = \(\frac{6}{216} \text { and } P(E \cap F)=\frac{1}{216}\)

⇒ \(P(E \mid F)=\frac{P(E \cap F)}{P(F)}=\frac{\frac{1}{6}}{\frac{216}{216}}=\frac{1}{6}\)

Question 9. Mother, father, and son line up at random for a family picture, E: son on one end, F: father in middle.
Solution:

If the mother (M), father (F), and son (S) line up for the family picture, then the sample space will be S = {MFS, MSF, FMS, FSM, SMF, SFM}

⇒ E = (MFS, FMS, SMF, SFM}, F = (MFS, SFM} => E n F = (MFS, SFM} = 2

⇒ \(P(E \cap F)=\frac{2}{6}=\frac{1}{3} \text { and } P(F)=\frac{2}{6}=\frac{1}{3} \Rightarrow P(E \mid F)=\frac{P(E \cap F)}{P(F)}=\frac{\frac{1}{3}}{\frac{1}{3}}=1\)

 

Question 10. A black and a red dice are rolled.

1. Find the conditional probability of obtaining a sum greater than 9, given that the black die resulted in a 5.
2. Find the conditional probability of obtaining the sum 8, given that the red die resulted in a number less than 4.

Solution:

Let the first observation be from the black die and the second from the red die. When two dice (one black and another red) are rolled, the sample space S = 6 x 6 = 36 number of elements.

1. Let A: Obtaining a sum greater than 9 = {(4, 6), (5. 5), (5, 6), (6, 4), (6, 5), (6, 6)}

B: Black die results in a 5 = {(5, 1), (5,2), (5, 3), (5,4), (5, 5), (5, 6)}

∴ A ∩ B= {(5, 5), (5, 6)}

The conditional probability of obtaining a sum greater than 9, given that the black die resulted in a 5, is given by P (A|B).

∴ P(A|B) = \(\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})}=\frac{\frac{2}{36}}{\frac{6}{36}}=\frac{2}{6}=\frac{1}{3}\)

2. E: Sum of the observations is 8 = {(2, 6), (3, 5), (4, 4), (5, 3), (6, 2)}

F: Red die resulted in a number less than 4 = \(\left\{\begin{array}{l}
(1,1),(1,2),(1,3),(2,1),(2,2),(2,3), \\
(3,1),(3,2),(3,3),(4,1),(4,2),(4,3), \\
(5,1),(5,2),(5,3),(6,1),(6,2),(6,3)
\end{array}\right\}\)

∴ E ∩ F = {(5, 3),(6, 2)}

P(F) = 18/36 and P(E n F) = 2/36

The conditional probability of obtaining the sum equal to 8, given that the red die resulted in a number less than 4, is given by P (E | F).

Therefore, \(P(E \mid F)=\frac{P(E \cap F)}{P(F)}=\frac{\frac{2}{36}}{\frac{18}{36}}=\frac{2}{18}=\frac{1}{9}\)

Question 11. A fair die is rolled. Consider events E = {1, 3, 5}, F = {2, 3} and G = (2, 3,4, 5}. Find

1. P(E | F) and P(F | E)
2. P(E | G) and P(G | E)
3. P((E ∪ F) | G) and P ((E ∩ F) | G)

Solution:

When a fair die is rolled, the sample space S will be S = {1,2,3, 4, 5, 6)

It is given that E = {1, 3, 5}, F = z[2, 3}, and G = {2, 3, 4, 5}

∴ P(E) = \(\frac{3}{6}=\frac{1}{2}, P(F)=\frac{2}{6}=\frac{1}{3}, P(G)=\frac{4}{6}=\frac{2}{3}\)

1. \(\mathrm{E} \cap \mathrm{F}=\{3\} \Rightarrow \mathrm{P}(\mathrm{E} \cap \mathrm{F})=\frac{1}{6}\)

∴ \(\mathrm{P}(\mathrm{E} \mid \mathrm{F})=\frac{\mathrm{P}(\mathrm{E} \cap \mathrm{F})}{\mathrm{P}(\mathrm{F})}=\frac{\frac{1}{6}}{\frac{1}{3}}=\frac{1}{2} ; \mathrm{P}(\mathrm{F} \mid \mathrm{E})=\frac{\mathrm{P}(\mathrm{F} \cap \mathrm{E})}{\mathrm{P}(\mathrm{E})}=\frac{\frac{1}{6}}{\frac{1}{2}}=\frac{1}{3}\)

2. \(\mathrm{E} \cap \mathrm{G}=\{3,5\}\)

∴ \(\mathrm{P}(\mathrm{E} \cap \mathrm{G})=\frac{2}{6}=\frac{1}{3}\)

∴ \(P(E \mid G)=\frac{P(E \cap G)}{P(G)}=\frac{\frac{1}{3}}{\frac{2}{3}}=\frac{1}{2} ; P(G \mid E)=\frac{P(G \cap E)}{P(E)}=\frac{\frac{1}{3}}{\frac{1}{2}}=\frac{2}{3}\)

3. \(\mathrm{P} \cup \mathrm{F}=\{1,2,3,5\}\)

⇒ \((\mathrm{E} \cup \mathrm{F}) \cap \mathrm{G}=\{1,2,3,5\} \cap\{2,3,4,5\}=\{2,3,5\}\)

⇒ \(\mathrm{E} \cap \mathrm{F}=\{3\}\)

⇒ \((\mathrm{E} \cap \mathrm{F}) \cap \mathrm{G}=\{3\} \cap\{2,3,4,5\}=\{3\}\)

⇒ \(P((\mathrm{E} \cup \mathrm{F}) \cap \mathrm{G})=\frac{3}{6}=\frac{1}{2}, \mathrm{P}((\mathrm{E} \cap \mathrm{F}) \cap \mathrm{G})=\frac{1}{6}\)

∴ \(\mathrm{P}((\mathrm{E} \cap \mathrm{F}) \mid \mathrm{G})=\frac{\mathrm{P}((\mathrm{E} \cap \mathrm{F}) \cap \mathrm{G})}{\mathrm{P}(\mathrm{G})}=\frac{\frac{1}{6}}{\frac{2}{3}}=\frac{1}{6} \times \frac{3}{2}=\frac{1}{4}\)

Question 12. Assume that each bom child is equally likely to be a boy or a girl. If a family has two children, what is the conditional probability that both are girls given that

1. The youngest is a girl,
2. At least one is a girl?

Solution:

Let b and g represent the boy and the girl child respectively. If a family has two children, the sample space will be

S = {(b, b), (b, g), (g, b), (g, g)}

Let A be the event that both children are girls.

∴ A={(g,g)}

1. Let B be the event that the youngest child is a girl.

∴ B = [(b, g), (g, g)] ⇒ A ∩ B = {(g, g)}

∴ \(P(B)=\frac{2}{4}=\frac{1}{2}, \quad P(A \cap B)=\frac{1}{4}\)

The conditional probability that both are girls, given that the youngest child is a girl, is given by P (A | B).

⇒ \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})=\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})}=\frac{\frac{1}{4}}{\frac{1}{2}}=\frac{1}{2}\)

Therefore, the required probability is 1/2.

2. Let C be the event that at least one child is a girl.

∴ C = {(b, g), (g,b), (g, g)} ⇒ A ∩ C = {g, g} ⇒ P(C) = 3/4

⇒ P(A ∩ C) = 1/4

The conditional probability that both are girls, given that at least one child is a girl, is given by P(A|C).

Therefore. P(A C) = \(P(A \mid C)=\frac{P(A \cap C)}{P(C)}=\frac{\frac{1}{4}}{\frac{3}{4}}=\frac{1}{3}\)

Question 13. An instructor has a question bank consisting of 300 easy True/False questions, 200 difficult True/False questions, 500 easy multiple choice questions, and 400 difficult multiple choice questions. If a question is selected at random from the question bank, what is the probability that it will be an easy question given that it is a multiple-choice question?
Solution:

The given data can be tabulated as

Let us denote E = easy questions, M = multiple choice questions, D = difficult questions, and T = True/False questions

Total number of questions = 1400

Total number of multiple choice questions = 900

Therefore, the probability of selecting an easy multiple choice question is \(P(E \cap M)=\frac{500}{1400}=\frac{5}{14}\)

Probability of selecting a multiple choice question, \(P(M)=\frac{900}{1400}=\frac{9}{14}\)

P (E | M) represents the probability that a randomly selected question will be an easy question, given that it is a multiple-choice question.

∴ \(\mathrm{P}(\mathrm{E} \mid \mathrm{M})=\frac{\mathrm{P}(\mathrm{E} \cap \mathrm{M})}{\mathrm{P}(\mathrm{M})}=\frac{\frac{-5}{14}}{\frac{9}{14}}=\frac{5}{9}\)

Therefore, the required probability is 5/9

Question 14. Given that the two numbers appearing on throwing the two dice are different. Find the probability of the event ‘the sum of numbers on the dice is 4.
Solution:

When two dice are thrown, the number of observations in the sample space = 6 x 6 = 36

Let A be the event that the sum of the numbers on both dice is 4 and B be the event that the two numbers appearing on throwing the two dice are different.

∴ A ={(1,3), (2, 2), (3,1)}

⇒ n(A) = 3

B = \(\left\{\begin{array}{l}
(1,2),(1,3),(1,4),(1,5),(1,6) \\
(2,1),(2,3),(2,4),(2,5),(2,6) \\
(3,1),(3,2),(3,4),(3,5),(3,6) \\
(4,1),(4,2),(4,3),(4,5),(4,6) \\
(5,1),(5,2),(5,3),(5,4),(5,6) \\
(6,1),(6,2),(6,3),(6,4),(6,5)
\end{array}\right\}=n(B)=30\)

∴ \(A \cap B=\{(1,3),(3,1)\}\)

⇒ P(B) = \(\frac{30}{36}=\frac{5}{6} \text { and } P(A \cap B)=\frac{2}{36}=\frac{1}{18}\)

Let \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})\) represent the probability that the sum of the numbers on both dice is 4, given that the two numbers appearing on throwing the two dice are different.

∴ \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})=\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})}=\frac{\frac{1}{18}}{\frac{5}{6}}=\frac{1}{15}\)

Therefore, the required probability is \(\frac{1}{15}\).

Question 15. Consider the experiment of throwing a die, if a multiple of 3 comes up, throw the die again and if any other number comes, toss a coin. Find the conditional probability of the event ‘the coin shows a tail’, given that at least one die shows a 3.
Solution:

The sample space of the experiment is,

S = \(\left\{\begin{array}{l}
(1, H),(1, T),(2, H),(2, T),(3,1),(3,2),(3,3),(3,4),(3,5),(3,6) \\
(4, H),(4, T),(5, H),(5, T),(6,1),(6,2),(6,3),(6,4),(6,5),(6,6)
\end{array}\right\}\)

Let A be the event that the coin shows a tail and B be the event that at least one die shows 3.

∴ A = {(1, T), (2, T), (4, T), (5, T)}

B = {(3,1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6), (6, 3)}

⇒ A ∩ B = ø

∴ P(A ∩ B) = 0

because there are no common elements.

Then, P(B) =P({3,1}) + P({3,2}) + P({3,3}) + P({3,4}) + P({3,5}) + P({3,6}) + P({6,3})

= \(\frac{1}{36}+\frac{1}{36}+\frac{1}{36}+\frac{1}{36}+\frac{1}{36}+\frac{1}{36}+\frac{1}{36}=\frac{7}{36}\)

The probability of the event that the coin shows a tail, given that at least one die shows 3, is given by P(A | B).

Therefore. \(P(A \mid B)=\frac{P(A \cap B)}{P(B)}=\frac{0}{\frac{7}{36}}=0\)

Choose The Correct Answer

Question 16. If P(A) = \(\frac{1}{2}\), P(B) = 0, then P(A | B) is

1. 0
2. \(\frac{1}{2}\)
3. Not Defined
4. 1

Solution: 3. Not defined

It is given that P(A) = \(\frac{1}{2}\) and P(B) = 0

P\((A \mid B)=\frac{P(A \cap B)}{P(B)}=\frac{P(A \cap B)}{0}\)

Therefore, P (A | B) is not defined.

Thus, the correct Answer is 3.

Question 17. If A and B are events such that P (A | B) = P(B | A), then

1. A ⊂ B but A ≠ B
2. A = B
3. A ∩ B = ø
4. P(A) = P(B)

Solution: 4. P(A) = P(B)

It is given that, P(A | B) = P(B | A)

⇒ \(\frac{P(A \cap B)}{P(B)}=\frac{P(B \cap A)}{P(A)}\)

⇒ P(A)=P(B)

Thus, the correct Answer is 4.

Probability Exercise 13.2

Question 1. If P(A) = \(\frac{3}{5}\) and P(B) = \(\frac{1}{5}\), find P (A ∩ B) if A and B are independent events.
Solution:

It is given that P(A) = \(\frac{3}{5}\) and P(B) = \(\frac{1}{5}\)

A and B are independent events. Therefore, P(A ∩ B) = P(A)P(B) = \(\frac{3}{5}\) \(\frac{1}{5}\) = \(\frac{3}{25}\)

Question 2. Two cards are drawn at random and without replacement from a pack of 52 playing cards. Find the probability that both the cards are black.
Solution:

There are 26 black cards in a deck of 52 cards.

Let A: event that 1st card is black, B: event that 2nd card is black.

P(getting a black card in the first draw) = P(A) = \(\frac{26}{52}\) = \(\frac{1}{2}\)

P (getting a black card on the second draw) – P(B/A) = \(\frac{25}{51}\) (v card is not replaced)

Thus, P(getting both the cards black) = P(A ∩ B) = P(A).P(B/A) = \(\frac{1}{2}\) x \(\frac{25}{51}\) = \(\frac{25}{102}\)

Question 3. A box of oranges is inspected by examining three randomly selected oranges drawn without replacement. If all three oranges are good, the box is approved for sale, otherwise, it is rejected. Find the probability that a box containing 15 oranges out of which 12 are good and 3 are bad ones will be approved for sale.
Solution:

Let A, B, and C be the events respectively that the first, second, and third drawn orange is good.

Therefore, probability that lust drawn orange is good, P(A) = \(\frac{12}{15}\)

Since the second orange is drawn without replacement (now the total number of good oranges will be 11 and the total oranges will be 14

∴ The conditional probability of B, given that A has already occurred is P(B/A) ⇒ P(B/A) = \(\frac{11}{14}\)

Again, the third orange is drawn without replacement (now the total number of good oranges will be 10, and the total number of oranges will be 13).

∴ The conditional probability of C, given that A and B have already occurred is P(C/AB)

⇒ P(C/AB)= \(\frac{10}{13}\)

The box is approved for sale if all three oranges are good.

Thus, the probability of getting all the oranges good

= P(A ∩ B ∩ C) = P(A). P(B/A). P(C/AB) = \(\frac{12}{15}\) x \(\frac{11}{14}\) x \(\frac{10}{3}\) = \(\frac{44}{91}\)

Therefore, the probability that the box is approved for sale is \(\frac{44}{91}\)

Question 4. A fair coin and an unbiased die are tossed. Let A be the event ‘head appears on the coin’ and B be the event ‘3 on the die’. Check whether A and B are independent events or not.
Solution:

If a fair coin and an unbiased die are tossed, then the sample space S is given by,

S = \(\left\{\begin{array}{l}
(\mathrm{H}, 1),(\mathrm{H}, 2),(\mathrm{H}, 3),(\mathrm{H}, 4),(\mathrm{H}, 5),(\mathrm{H}, 6) \\
(\mathrm{T}, 1),(\mathrm{T}, 2),(\mathrm{T}, 3),(\mathrm{T}, 4),(\mathrm{T}, 5),(\mathrm{T}, 6)
\end{array}\right\} \Rightarrow \mathrm{n}(\mathrm{S})=12\)

Let A: Head appears on the coin

A = {(H, 1), (H, 2), (H, 3), (H, 4), (H, 5), (H, 6)}

⇒ P(A) = \(\frac{6}{12}\) = \(\frac{1}{2}\)

B: 3 on die = {(H, 3), (T, 3)}

P(B) = \(\frac{2}{12}\) = \(\frac{1}{6}\)

∴ A∩B = {(H, 3)}

P(A∩B) = \(\frac{1}{12}\)

Also, P(A)·P(B) =\(\frac{1}{2}\) x \(\frac{1}{6}\) =\(\frac{1}{12}\) = P(A∩B)

Therefore, A and B are independent events.

Question 5. A die marked 1, 2, 3 in red and 4, 5, 6 in green is tossed. Let A be the event, ‘the number is even,’ and B be the event, ‘the number is red’. Are A and B independent?
Solution:

When a die is thrown, the sample space (S) is S = {1, 2, 3, 4, 5, 6}

Let A: the number is even = {2, 4, 6} ⇒ P(A) = \(\frac{3}{6}\) = \(\frac{1}{2}\)

B: the number is red = {1,2, 3} ⇒ P(B) = \(\frac{3}{6}\) = \(\frac{1}{2}\)

∴ A∩B = {2} ⇒ P(A∩B) = \(\frac{1}{6}\)

Also, P(A)·P(B) = \(\frac{1}{2}\) x \(\frac{1}{2}\) = \(\frac{1}{4}\)≠\(\frac{1}{6}\)≠P(A∩B)

⇒ P(A) · P(B) ≠ P(A∩B)

Therefore, A and B are not independent.

Question 6. Let E and F be events withP(E) = \(\frac{3}{5}\), P(F) = \(\frac{3}{10}\) and P(E∩F) = \(\frac{1}{5}\). Are E and F independent?
Solution:

It is given that P(E) = \(\frac{3}{5}\), P(F) = \(\frac{3}{10}\) and P(E∩F) = \(\frac{1}{5}\)

Now, P(E) · P(F) = \(\frac{3}{5} \cdot \frac{3}{10}=\frac{9}{50} \neq \frac{1}{5} \Rightarrow \mathrm{P}(\mathrm{E} \cap \mathrm{F}) \Rightarrow \mathrm{P}(\mathrm{E}) \cdot \mathrm{P}(\mathrm{F}) \neq \mathrm{P}(\mathrm{E} \cap \mathrm{F})\)

Therefore, E and F are not independent.

Question 7. Given that the events A and B are such that P(A) = \(\frac{1}{2}\), P(A∪B) = \(\frac{3}{5}\) and P(B) = p. Find p if they are

1. Mutually exclusive
2. Independent

Solution:

It is given that P(A) = \(\frac{1}{2}\), P(A∪B) = \(\frac{3}{5}\) and P(B) = p

1. When A and B are mutually exclusive, A∩B = ø

∴ P(A∩B) = 0

It is known that, P(A∪B) = P(A) + P(B) – P(A∩B)

2. When A and B are independent, events then, P(A∩B) = P(A)·P(B) = \(\frac{1}{2}\) p

It is known that, P(A∩B) = P(A) + P(B) – P(A∩B)

⇒ \(\frac{3}{5}=\frac{1}{2}+\mathrm{p}-\frac{1}{2} \mathrm{p} \Rightarrow \frac{3}{5}=\frac{1}{2}+\frac{\mathrm{p}}{2}\)

⇒ \(\frac{\mathrm{p}}{2}=\frac{3}{5}-\frac{1}{2}=\frac{1}{10} \Rightarrow \mathrm{p}=\frac{2}{10}=\frac{1}{5}\)

Question 8. Let A and B be independent events with P(A) = 0.3 and P(B) = 0.4. Find

1. (P(A∩B),
2. P(A∪B),
3. P(A|B),
4. P(B|A)

Solution:

It is given that P(A) = 0.3 and P(B) = 0.4

1. If A and B are independent events, then P(A∩B) = P(A)·P(B) = 0.3 x 0.4 = 0.12
2. P(A∪B) = P(A) + P(B)-P(A∩B) ⇒ P(A∪B) = 0.3 + 0.4 – 0.12 = 0.58
3. It is known that, P(A | B) = \(\frac{P(A \cap B)}{P(B)} \Rightarrow P(A \mid B)=\frac{0.12}{0.4}=0.3\)
4. It is known that, P(B | A) = \(\frac{\mathrm{P}(\mathrm{B} \cap \mathrm{A})}{\mathrm{P}(\mathrm{A})} \Rightarrow \mathrm{P}(\mathrm{B} \mid \mathrm{A})=\frac{0.12}{0.3}=0.4\)

Question 9. If A and B are two events such that P(A) = \(\frac{1}{2}\), P(B) = \(\frac{1}{2}\) and P(A∩B) = \(\frac{1}{8}\), find P(not A and not B).
Solution:

It is given that P(A) = \(\frac{1}{4}\), P(B) = \(\frac{1}{2}\) and P(A∩B) = \(\frac{1}{8}\)

P(not A and not B) = P(A’∩B’)

P(not A and not B) = p((A∪B)’) [A’∩B’ = (A∪B)’]

= \(1-P(A \cup B)=1-[P(A)+P(B)-P(A \cap B)]\)

= \(1-\left[\frac{1}{4}+\frac{1}{2}-\frac{1}{8}\right]=1-\frac{5}{8}=\frac{3}{8}\)

Question 10. Events A and B are such that P(A) = \(\frac{1}{2}\), P(B) = \(\frac{7}{12}\) and P(not A or not B) = \(\frac{1}{4}\). State whether A and B are independent.
solution:

It is given that \(P(A)=\frac{1}{2}, P(B)=\frac{7}{12}\) and P(not A or not B)= \(\frac{1}{4}\).

⇒ \(\mathrm{P}\left(\mathrm{A}^{\prime} \cup \mathrm{B}^{\prime}\right)=\frac{1}{4}\)

⇒ \(\mathrm{P}\left((\mathrm{A} \cap \mathrm{B})^{\prime}\right)=\frac{1}{4}\)

(because \(\mathrm{A}^{\prime} \cup \mathrm{B}^{\prime}=(\mathrm{A} \cap \mathrm{B})^{\prime}\))

⇒ 1-\(\mathrm{P}(\mathrm{A} \cap \mathrm{B})=\frac{1}{4} \Rightarrow \mathrm{P}(\mathrm{A} \cap \mathrm{B})=\frac{3}{4}\)….(1)

However, \(\mathrm{P}(\mathrm{A}) \cdot \mathrm{P}(\mathrm{B})=\frac{1}{2} \cdot \frac{7}{12}=\frac{7}{24}\)

Here, \(\frac{3}{4} \neq \frac{7}{24}\)…..(2)

∴ \(P(A \cap B) \neq P(A) \cdot P(B)\)

Therefore, A and B are not independent events.

Question 11. Given two independent events A and B such that P (A) = 0.3, P (B) = 0.6. Find

1. P(A and B)
2. P(A and not B)
3. P(A or B)
4. P(neither A nor B)

Solution:

It is given that P (A) = 0.3 and P (B) = 0.6

Also, A and B are independent events.

1. P(A and B) = P(A)·P(B) ⇒ P(A∩B) = 0.3 x 0.6 = 0.18
2. P(A and not B) – P(A∩B’) = P(A) – P(A∩B) = 0.3 – 0.18 = 0.12
3. P(A or B) = P(A∪B) = P(A) + P(B) – P(A∩B) = 0.3 + 0.6 – 0.18 = 0.72
4. P(neither A nor B) = P(A’∩B’) = P((A∪B)’) = 1 – P(A∪B) = 1- 0.72 = 0.28

Question 12. A die is tossed thrice. Find the probability of getting an odd number at least once.
Solution:

Probability of getting an odd number in a single throw of a die = \(\frac{3}{6}\) = \(\frac{1}{2}\)

Similarly, probability of getting an even number = \(\frac{3}{6}\) = \(\frac{1}{2}\)

Probability of getting an even number three times = \(\frac{1}{2}\) x \(\frac{1}{2}\) x \(\frac{1}{2}\) = \(\frac{1}{8}\)

Therefore, the probability of getting an odd number at least once

= 1 – Probability of getting an odd number in none of the throws

= 1 – Probability of getting an even number thrice

= 1- \(\frac{1}{8}\) = \(\frac{7}{8}\)

Question 13. Two balls are drawn at random with replacement from a box containing 10 black and 8 red balls. Find the probability that

1. Both balls are red.
2. The first ball is black and the second is red.
3. One of them is black and the other is red.

Solution:

Let R: event that drawn ball is red

B: event that drawn ball is black

∴ P(R) = \(\frac{8}{18}\) = \(\frac{4}{9}\) and P(B) = \(\frac{10}{18}\) = \(\frac{5}{9}\)

1. P(Both balls are red)

= \(P(R \cap R)=P(R) \cdot P(R)\) (Ball is replaced)

= \(\frac{4}{9} \cdot \frac{4}{9}=\frac{16}{81}\)

2. P(first ball is black and second is red) = P(B∩R)

= P(B). P(R) (Ball is replaced)

= \(\frac{5}{9} \cdot \frac{4}{9}=\frac{20}{81}\)

3. P(one of them is black and other red)

= P [(R∩B)∪(B∩R)] = P(R∩B) + P(B∩R)

= P(R). P(B) + P(B). P(R) (Ball is replaced)

= \(\frac{4}{9} \cdot \frac{5}{9}+\frac{5}{9} \cdot \frac{4}{9}=\frac{20}{81}+\frac{20}{81}=\frac{40}{81}\)

Question 14. The probability of solving specific problems independently by A and B are \(\frac{1}{2}\) and \(\frac{1}{3}\) respectively. If both try to solve the problem independently, find the probability that

1. The problem is solved
2. Exactly one of them solves the problem.

Solution:

Let E₁: an event that A solves the problem

E₂: an event that B solves the problem

Then \(P\left(E_1\right)=\frac{1}{2}\) and \(P\left(E_1\right)=\frac{1}{3} \Rightarrow P\left(\bar{E}_1\right)=1-\frac{1}{2}=\frac{1}{2}\) and \(P\left(\bar{E}_2\right)=1-\frac{1}{3}=\frac{2}{3}\)

Clearly, \(\mathrm{E}_1\) and \(\mathrm{E}_2\) are independent events :

1. P (the problem is solved)

= \(P(\text { at least one of } A \text { and } B \text { solves the problem })\)

= \(P\left(E_1 \cup E_2\right)=P\left(E_1\right)+P\left(E_2\right)-P\left(E_1 \cap E_2\right)=P\left(E_1\right)+P\left(E_2\right)-P\left(E_1\right), P\left(E_2\right)\)

= \(\frac{1}{2}+\frac{1}{3}-\frac{1}{2} \cdot \frac{1}{3}=\frac{2}{3}\)

2. P(exactly one of them solves the problem)

= \(P\left[\left(E_1 \cap \bar{E}_2\right) \cup\left(\bar{E}_1 \cap E_2\right)\right]\)

= \(P\left(E_1 \cap \bar{E}_2\right)+P\left(\bar{E}_1 \cap E_2\right)\)

= \(P\left(E_1\right) \cdot P\left(\bar{E}_2\right)+P\left(\bar{E}_1\right) \cdot P\left(E_2\right)\)

= \(\frac{1}{2} \times \frac{2}{3}+\frac{1}{3} \times \frac{1}{2}=\frac{1}{2}\)

Question 15. One card is drawn at random from a well-shuffled deck of 52 cards. In which of the following cases are the events E and F independent?

1. E: ‘The card drawn is a spade’, F: ‘The card drawn is an ace’
2. E: ‘The card drawn is black’, F: ‘The card drawn is a king’
3. E: ‘The card drawn is a king or queen’, F: ‘The card drawn is a queen or jack’

Solution:

1. In a deck of 52 cards, 13 cards are spades and 4 cards are aces.

∴ P(E) = P(the card drawn is a spade) = \(\frac{13}{52}\) = \(\frac{1}{4}\)

∴ P(F) = P(the card drawn is an ace) = \(\frac{4}{52}\) = \(\frac{1}{3}\)

In the deck of cards, only 1 card is an ace of spades.

P(E∩F) = P(the card drawn is spade and an ace) = \(\frac{1}{52}\)

⇒ \(\mathrm{P}(\mathrm{E}) \cdot \mathrm{P}(\mathrm{F})=\frac{1}{4} \cdot \frac{1}{13}=\frac{1}{52}=\mathrm{P}(\mathrm{E} \cap \mathrm{F})\)

⇒ \(\mathrm{P}(\mathrm{E}) \cdot \mathrm{P}(\mathrm{F})=\mathrm{P}(\mathrm{E} \cap \mathrm{F})\)

Therefore, the events E and F are independent.

2. In a deck of 52 cards, 26 cards are black and 4 cards are kings.

∴ P(E) = P(the card drawn is black) = \(\frac{26}{52}\) = \(\frac{1}{2}\)

∴ P(F) = P(the card drawn is black) = \(\frac{4}{52}\) = \(\frac{1}{13}\)

In the pack of 52 cards, 2 cards are black as well as kings.

∴ P(E∩F) = P(the card drawn is a black king) = \(\frac{2}{52}\) = \(\frac{1}{26}\)

Also, \(P(E) \times P(F)=\frac{1}{2} \cdot \frac{1}{13}=\frac{1}{26}=P(E \cap F)\)

Therefore, the given events E and F are independent.

3. In a deck of 52 cards, 4 cards are kings, 4 cards are queens, and 4 cards are jacks.

∴ P(E) = P(the card drawn is a king or a queen) = \(\frac{8}{52}\) = \(\frac{2}{13}\)

∴ P(F) = P(the card drawn is a queen or a jack) = \(\frac{8}{52}\) = \(\frac{2}{13}\)

There are 4 cards which are either king or queen and either queen or jack.

∴P(E∩F) = P(the card drawn is either king or queen and either queen or jack) = \(\frac{4}{52}\) = \(\frac{1}{13}\)

Now, \(\mathrm{P}(\mathrm{E}) \times \mathrm{P}(\mathrm{F})=\frac{2}{13} \cdot \frac{2}{13}=\frac{4}{169} \neq \frac{1}{13}\)

⇒ P(E)·P(F) ≠ P(E∩ F)

Therefore, the given events E and F are not independent.

Question 16. In a hostel, 60% of the students read Hindi newspapers, 40% read English newspapers and 20% read both Hindi and English newspapers. A student is selected at random.

1. Find the probability that she reads neither Hindi nor English newspapers.
2. If she reads a Hindi newspaper, find the probability that she reads an English newspaper.
3. If she reads an English newspaper, find the probability that she reads a Hindi newspaper.

Solution:

Let H denote the students who read Hindi newspapers and E denote the students who read English newspapers.

It is given that, P(H)=60 \(\%=\frac{6}{10}=\frac{3}{5} ; \mathrm{P}(\mathrm{E})=40 \%=\frac{40}{100}=\frac{2}{5} ; \mathrm{P}(\mathrm{H} \cap \mathrm{E})=20 \%=\frac{20}{100}=\frac{1}{5}\)

1. The probability that a student reads neither Hindi nor English newspapers is,

⇒ \(\mathrm{P}_{(}\left(\mathrm{H}^{\prime} \cap \mathrm{E}^{\prime}\right)=\mathrm{P}\left(\mathrm{H} \cup \mathrm{E}^{\prime}\right.=1-\mathrm{P}(\mathrm{H} \cup \mathrm{E})=1-\{\mathrm{P}(\mathrm{H})+\mathrm{P}(\mathrm{E})-\mathrm{P}(\mathrm{H} \cap \mathrm{E})\}\)

= \(1-\left(\frac{3}{5}+\frac{2}{5}-\frac{1}{5}\right)=1-\frac{4}{5}=\frac{1}{5}\)

2. The probability that a randomly chosen student reads an English newspaper if she reads a Hindi newspaper, is given by \(\mathrm{P}(\mathrm{E} \mid \mathrm{H})\).

P(E | H) = \(\frac{P(E \cap H)}{P(H)}=\frac{\frac{1}{5}}{\frac{3}{5}}=\frac{1}{3}\)

3. The probability that a randomly chosen student reads a Hindi newspaper if she reads an English newspaper, is given by \(\mathrm{P}(\mathrm{H} \mid \mathrm{E})\).

P(H | E) = \(\frac{P(H \cap E)}{P(E)}=\frac{\frac{1}{5}}{\frac{2}{5}}=\frac{1}{2}\)

Choose The Correct Answer

Question 17. The probability of obtaining an even prime number on each die. when a pair of dice is rolled is

1. 0
2. 1/3
3. 1/12
4. 1/36

Solution: 4. 1/36

When two dice are rolled, the number of outcomes is 36. i.e. ⇒ n(S) = 36

The only even prime number is 2.

i.e. ⇒ n(E) = 1 Let E be the event of getting an even prime number on each die.

∴ E = {(2, 2)} ⇒ P(E) = 1/36

Therefore, the correct Answer is 4.

Question 18. Two events A and B will be independent, if

1. A and B are mutually exclusive
2. P(A)=P(B)
3. P(A’B’) = [1 – P(A)] [1 – P(B)]
4. P(A) = P(B) (D) P(A) + P(B) – 1

Solution: 2. P(A)=P(B)

Two events A and B are said to be independent if P(A∩B) = P(A) x P(B)

Consider the result given in alternative B.

P(A’B’) = [1 – P(A)] [1 – P(B)]

⇒ P(A’∩B’) = l-P(A)-P(B) + P(A)P(B)

⇒ 1-P(A∪B)=l- P(A) – P(B) + P(A)P(B)

⇒ P(A∪B) = P(A) + P(B)-P(A)P(B)

⇒ P(A) + P(B) – P(AB) = P(A) + P(B) – P(A)P(B)

⇒ P(AB) = P(A)P(B)

This implies that A and B are independent, if P(A’B’) = [1 – P(A)] [1 – P(B)]

Probability Exercise 13.3

Question 1. An urn contains 5 red and 5 black balls. A ball is drawn at random, its color is noted and is returned to the urn. Moreover, 2 additional balls of the color drawn are put in the urn and then a ball is drawn at random. What is the probability that the second ball is red?
Solution:

The urn contains 5 red and 5 black balls.

Let a red ball be drawn in the first attempt.

∴ P (drawing a red ball) = \(\frac{5}{10}\) = \(\frac{1}{2}\)

If two red balls are added to the urn, then the urn contains 7 red and 5 black balls.

P(drawing a red ball) = \(\frac{7}{12}\)

Let a black ball be drawn in the first attempt.

∴ P (drawing a black ball in the first attempt) = \(\frac{5}{10}\) = \(\frac{1}{2}\)

If two black balls are added to the urn, then the urn contains 5 red and 7 black balls.

P(drawing a red all) = \(\frac{5}{12}\)

Therefore, probability of drawing second ball as red is = \(\frac{1}{2} \times \frac{7}{12}+\frac{1}{2} \times \frac{5}{12}=\frac{1}{2}\left(\frac{7}{12}+\frac{5}{12}\right)=\frac{1}{2} \times 1=\frac{1}{2}\)

Question 2. A bag contains 4 red and 4 black balls, another bag contains 2 red and 6 black balls. One of the two bags is selected at random and a ball is drawn from the bag which is found to be red. Find the probability that the ball is drawn from the first bag.
Solution:

Let E₁ and E₂ be the events of selecting the first bag and second bag respectively.

∴ \(\mathrm{P}\left(\mathrm{E}_1\right)=\mathrm{P}\left(\mathrm{E}_2\right)=\frac{1}{2}\)

Let A be the event of getting a red bail.

⇒ P(A | E₁) = P(drawing a red ball from first bag) = \(\frac{4}{8}\) = \(\frac{1}{2}\)

⇒ P(A | E₂) = P(drawing a red ball from second bag) = \(\frac{2}{8}\) = \(\frac{1}{4}\)

The probability of drawing a ball from the first bag, given that it is red, is given by P (E₁/A). By using Bayes’ theorem, we obtain

⇒ \(P\left(E_1 \mid A\right)=\frac{P\left(E_1\right) \cdot P\left(A \mid E_1\right)}{P\left(E_1\right) \cdot P\left(A \mid E_1\right)+P\left(E_2\right) \cdot P\left(A \mid E_2\right)}\)

= \(\frac{\frac{1}{2} \cdot \frac{1}{2}}{\frac{1}{2} \cdot \frac{1}{2}+\frac{1}{2} \cdot \frac{1}{4}}=\frac{\frac{1}{4}}{\frac{1}{4}+\frac{1}{8}}=\frac{\frac{1}{4}}{\frac{3}{8}}=\frac{2}{3}\)

Question 3. Of the students in a college, it is known that 60% reside in a hostel and 40% are day scholars (not residing in the hostel). Previous year results report that 30% of all students who reside in hostel attain A grades and 20% of day scholars attain A grades in their annual examination. At the end of the year, one student is chosen at random from the college and he has an A grade, what is the probability that the student is hostlier?
Solution:

Let E₁ and E₂ be the events in which the student is a hostlier and a day scholar respectively and A be the event in which the chosen student gets a grade A.

Then, E₁ and E₂ are naturally exclusive and exhaustive.

∴ \(\mathrm{P}\left(\mathrm{E}_1\right)=60 \%=\frac{60}{100}=0.6 ; \quad \mathrm{P}\left(\mathrm{E}_2\right)=40 \%=\frac{40}{100}=0.4\)

P(A|E₁) = P(student getting an A grade is a costlier) = 30% = 0.3

P(A|E₂) = P(student getting an A grade is a day scholar) = 20% = 0.2

The probability that a randomly chosen student is a hostlier, given that he has an A grade, is given by P(E₁|A).

By using Bayes’ theorem, we obtained

⇒ \(P\left(E_1 \mid A\right)=\frac{P\left(E_1\right) \cdot P\left(A \mid E_1\right)}{P\left(E_1\right) \cdot P\left(A \mid E_1\right)+P\left(E_2\right) \cdot P\left(A \mid E_2\right)}=\frac{0.6 \times 0.3}{0.6 \times 0.3+0.4 \times 0.2}=\frac{0.18}{0.26}=\frac{18}{26}=\frac{9}{13}\)

Question 4. In answering a question on a multiple-choice test, a student either knows the answer or guesses. Let \(\frac{3}{4}\) be the probability that he knows the answer and \(\frac{1}{4}\)  be the probability that he guesses.

Assuming that a student who guesses the answer will be correct with probability \(\frac{1}{4}\), What is the probability that the student knows the answer given that he answered it correctly?

Solution:

Let E₁ and E₂ be the events which the student knows the answer and guesses the answer respectively.

Let A be the event that the answer is correct.

∴ \(\mathrm{P}\left(\mathrm{E}_1\right)=\frac{3}{4} ; \quad \mathrm{P}\left(\mathrm{E}_2\right)=\frac{1}{4}\)

The probability that the student answered correctly, given that he knows the answer, is 1.

∴ \(\mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_1\right)=1\)

The probability that the student answered correctly, given that he guessed, is

∴ \(\mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_2\right)=1\)

The probability that the student knows the answer, given that he answered it correctly, is given by P(E₁|A).

By using Bayes’ theorem, we obtain

⇒ \(P\left(E_1 \mid A\right)=\frac{P\left(E_1\right) \cdot P\left(A \mid E_1\right)}{P\left(E_1\right) \cdot P\left(A \mid E_1\right)+P\left(E_2\right) \cdot P\left(A \mid E_2\right)}=\frac{\frac{3}{4} \cdot 1}{\frac{3}{4} \cdot 1+\frac{1}{4} \cdot \frac{1}{4}}=\frac{\frac{3}{4}}{\frac{3}{4}+\frac{1}{16}}=\frac{\frac{3}{4}}{\frac{13}{16}}=\frac{12}{13}\)

Question 5. A laboratory blood test is 99% effective in detecting a certain disease when it is in fact, present. However, the test also yields a false positive result for 0.5% of the healthy person tested (i.e., if a healthy person is tested, then, with a probability of 0.005, the test will imply he has the disease). If 0.1 percent of the population actually has the disease, what is the probability that a person has the disease given that his test result is positive?
Solution:

Let E₁ and E₂ be the events that a person has a disease and a person is healthy respectively.

Since E₁ and E₂ are pairwise disjoint and exhaustive events.

P(E₁) + P(E₂) = 1 ⇒ P(E₂) = 1 – P(E₁) = 1 – 0.001 = 0.999 [P(E₁) = 0.1% = 0.001]

Let A be the event that the blood test result is positive.

P(A|E₁) = P(result is positive given the person has disease) = 99% = 0.99

P(A|E₂) = Pfresult is positive given that the person is healthy) = 0.5% = 0.005

The probability that a person has a disease, given that his test result is positive, is given by P (E₁|A).

By using Bayes’ theorem, we obtain

⇒ \(\mathrm{P}\left(\mathrm{E}_1 \mid \mathrm{A}\right) =\frac{\mathrm{P}\left(\mathrm{E}_1\right) \cdot \mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_1\right)}{\mathrm{P}\left(\mathrm{E}_1\right) \cdot \mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_1\right)+\mathrm{P}\left(\mathrm{E}_2\right) \cdot \mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_2\right)}\)

= \(\frac{0.001 \times 0.99}{0.001 \times 0.99+0.999 \times 0.005}=\frac{0.00099}{0.00099+0.004995}\)

= \(\frac{0.00099}{0.005985}=\frac{990}{5985}=\frac{110}{665}=\frac{22}{133}\)

Question 6. There are three coins. One is a two-headed coin (having heads on both faces), another is a biased coin that comes up heads 75% of the time and the third is an unbiased coin. One of the three coins is chosen at random and tossed, it shows heads, what is the probability that it was the two-headed coin?
Solution:

Let E₁, E_2,  and E₃ be the respective events of choosing a two-headed coin, a biased coin, and an unbiased coin.

Then, E₁, E₂, and E₃ are mutually exclusive and exhaustive events.

∴ P(E₁) = P(E₂) = P(E₃) = 1/3

Let A be the event that the coin shows heads.

A two-headed coin will always show heads.

∴ P(A|E₁) = P(coin showing heads, given that it is a two-headed coin) = 1

Probability of heads coming up, given that it is a biased coin= 75%

∴ P(A|E₂) = P(coin showing heads, given that it is a biased coin) = \(\frac{75}{1000}\) = \(\frac{3}{4}\)

Since the third coin is unbiased, the probability that it shows heads is always  \(\frac{1}{2}\)

∴ P(A|E₃) = P(coin showing heads, given that it is a biased coin) = \(\frac{1}{2}\)

The probability that the coin is two-headed. given that it shows heads, is given by P (E₁lA)

By using Bayes’ theorem, we obtain

⇒ \(P\left(E_1 \mid A\right)=\frac{P\left(E_1\right) \cdot P\left(A \mid E_1\right)}{P\left(E_1\right) \cdot P\left(A \mid E_1\right)+P\left(E_2\right) \cdot P\left(A \mid E_2\right)+P\left(E_3\right) \cdot P\left(A \mid E_3\right)}\)

= \(\frac{\frac{1}{3} \cdot 1}{\frac{1}{3} \cdot 1+\frac{1}{3} \cdot \frac{3}{4}+\frac{1}{3} \cdot \frac{1}{2}}=\frac{\frac{1}{3}}{\frac{1}{3}\left(1+\frac{3}{4}+\frac{1}{2}\right)}=\frac{1}{\frac{9}{4}}=\frac{4}{9}\)

Question 7. An insurance company insured 2000 scooter drivers, 4000 car drivers, and 6000 truck drivers. The probability of accidents is 0.01,0.03 and 0.15 respectively. One of the insured persons meets with an accident. What is the probability that he is a scooter driver?
Solution:

Let E₁, E_2, and E₃ be the events in which the driver is a scooter driver, a car driver, and a truck driver respectively.

Let A be the event that the person meets with an accident.

There are 2000 scooter drivers, 4000 car drivers, and 6000 truck drivers.

Total number of drivers = 2000 + 4000 + 6000 = 12000

P\(\left(E_1\right)\)=P(driver is a scooter driver)=\(\frac{2000}{12000}=\frac{1}{6}\)

P\(\left(\mathrm{E}_2\right)=\mathrm{P}\)(driver is a car driver) = \(\frac{4000}{12000}=\frac{1}{3}\)

P\(\left(\mathrm{E}_3\right)=\mathrm{P}\)(driver is a truck driver )=\(\frac{6000}{12000}=\frac{1}{2}\)

P\(\left(\mathrm{A} \mid \mathrm{E}_1\right)=\mathrm{P}\)(scooter driver met with an accident) =0.01 = \(\frac{1}{100}\)

P\(\left(\mathrm{A} \mid \mathrm{E}_2\right)=\mathrm{P}\)(car driver met with an accident)[/latex] = \(0.03=\frac{3}{100}\)

P\(\left(\mathrm{A} \mid \mathrm{E}_3\right)=\mathrm{P}\)(truck driver met with an accident) = \(0.15=\frac{15}{100}\)

The probability that the driver is a scooter driver, given that he met with an accident, is given by \(\mathrm{P}\left(\mathrm{E}_1 \mid \mathrm{A}\right)\).

By using Bayes’ theorem, we obtain

⇒ \(P\left(E_1 \mid A\right)=\frac{P\left(E_1\right) \cdot P\left(A \mid E_1\right)}{P\left(E_1\right) \cdot P\left(A \mid E_1\right)+P\left(E_2\right) \cdot P\left(A \mid E_2\right)+P\left(E_3\right) \cdot P\left(A \mid E_3\right)}\)

= \(\frac{\frac{1}{6} \cdot \frac{1}{100}}{\frac{1}{6} \cdot \frac{1}{100}+\frac{1}{3} \cdot \frac{3}{100}+\frac{1}{2} \cdot \frac{15}{100}}=\frac{\frac{1}{6} \cdot \frac{1}{100}}{\frac{1}{100}\left(\frac{1}{6}+1+\frac{15}{2}\right)}=\frac{\frac{1}{6}}{\frac{104}{12}}=\frac{1}{6} \times \frac{12}{104}=\frac{1}{52}\)

Question 8. A factory has two machines A and B. Past records show that machine A produced 60% of the items of output and machine B produced 40% of the items. Further, 2% of the items produced by machine A and 1% produced by machine B were defective. All the items are put into one stockpile and then one item is chosen at random from this and is found to be defective. What is the probability that was produced by machine B?
Solution:

Let E₁ and E₂ be the events of items produced by machines A and B respectively. Let X be the event that the produced item was found to be defective.

∴ Probability of items produced by machine A, P (E₁) = 60% = \(\frac{3}{4}\)

Probability of items produced by machine B, P (E₂) = 40% = \(\frac{2}{5}\)

Probability that machine A produced defective items, P(X|E₁)= 2% = \(\frac{2}{100}\)

Probability that machine B produced defective items, P(X|E₂) = 1% = \(\frac{1}{100}\)

The probability that the randomly selected item was from machine B, given that it is defective, is given by P (E₂|X).

By using Bayes’ theorem, we obtain

⇒ \(P\left(E_2 \mid X\right)=\frac{P\left(E_2\right) \cdot P\left(X \mid E_2\right)}{P\left(E_1\right) \cdot P\left(X \mid E_1\right)+P\left(E_2\right) \cdot P\left(X \mid E_2\right)}=\frac{\frac{2}{5} \cdot \frac{1}{100}}{\frac{3}{5} \cdot \frac{2}{100}+\frac{2}{5} \cdot \frac{1}{100}}=\frac{\frac{2}{500}}{\frac{6}{500}+\frac{2}{500}}=\frac{2}{8}=\frac{1}{4}\)

Question 9. Two groups are competing for the position on the board of directors of a corporation. The probabilities that the first and the second groups will win are 0.6 and 0.4 respectively. Further, if the first group wins, the probability of introducing a new product is 0.7 and the corresponding probability is 0.3 if the second group wins. Find the probability that the new product introduced was by the second group.
Solution:

Let E₁ and E₂ be the events that the first group and the second group win the competition respectively. Let A be the event of introducing a new product.

P (E₁) = Probability that the first group wins the competition = 0.6

P (E₂) = Probability that the second group wins the competition = 0.4

P (A|E₁) = Probability of introducing a new product if the first group wins = 0.7

P (A|E₂) = Probability of introducing a new product if the second group wins = 0.3

The probability that the new product is introduced by the second group is given by P (E₂|A).

By using Bayes’ theorem, we obtain

⇒ \(P\left(E_2 \mid A\right)=\frac{P\left(E_2\right) \cdot P\left(A \mid E_2\right)}{P\left(E_1\right) \cdot P\left(A \mid E_1\right)+P\left(E_2\right) \cdot P\left(A \mid E_2\right)}=\frac{0.4 \times 0.3}{0.6 \times 0.7+0.4 \times 0.3}=\frac{0.12}{0.42+0.12}=\frac{0.12}{0.54}=\frac{12}{54}=\frac{2}{9}\)

Question 10. Suppose a girl throws a die. If she gets a 5 or 6, she tosses a coin three times and notes the number of heads. If she gets 1, 2, 3, or 4, she tosses a coin once and notes whether a head or tail is obtained. If she obtained exactly one head, what is the probability that she threw 1, 2, 3, or 4 with the die?
Solution:

Let E₁ be the event that the outcome on the die is 5 or 6 and E2 be the event that the outcome on the die is 1,2, 3, or 4.

∴ \(P\left(E_1\right)=\frac{2}{6}=\frac{1}{3} \text { and }\left(E_2\right)=\frac{4}{6}=\frac{2}{3}\)

Let A be the event of getting exactly one head.

P(A|E₁) = Probability of getting exactly one head by tossing the coin three times if she gets 5 or 6 = \(\frac{3}{8}\)

P(A|E₂) = Probability of getting exactly one head in a single throw of the coin if she gets 1, 2, 3, or 4 = \(\frac{1}{2}\)

The probability that the girl took 1,2, 3, or 4 with the die, if she obtained exactly one head, is given by P(E₂|A). By using Bayes’ theorem, we obtain

∴ \(P\left(E_2 \mid A\right)=\frac{P\left(E_2\right) \cdot P\left(A \mid E_2\right)}{P\left(E_1\right) \cdot P\left(A \mid E_1\right)+P\left(E_2\right) \cdot P\left(A \mid E_2\right)}=\frac{\frac{2}{3} \cdot \frac{1}{2}}{\frac{1}{3} \cdot \frac{3}{8}+\frac{2}{3} \cdot \frac{1}{2}}=\frac{\frac{1}{3}}{\frac{1}{3}\left(\frac{3}{8}+1\right)}=\frac{1}{\frac{11}{8}}=\frac{8}{11}\)

Question 11. A manufacturer has three machine operators A, B, and C. The first operator A produces 1% defective items, whereas the other two operators B and C produce 5% and 7% defective items respectively. A is on the job for 50% of the time, B is on the job for 30% of the time and C is on the job for 20% of the time. A defective item is produced, what is the probability that was produced by A?
Solution:

Let E₁, E₂, and E₃ be the events of the time consumed by machine operators A, B, and C for the job respectively.

⇒ \(\mathrm{P}\left(\mathrm{E}_1\right)=50 \%=\frac{50}{100}=\frac{1}{2} ; \mathrm{P}\left(\mathrm{E}_2\right)=30 \%=\frac{30}{100}=\frac{3}{10} ; \mathrm{P}\left(\mathrm{E}_3\right)=20 \%=\frac{20}{100}=\frac{1}{5}\)

Let X be the event of producing defective items.

⇒ \(\mathrm{P}\left(\mathrm{X} \mid \mathrm{E}_1\right)=1 \%=\frac{1}{100} ; \mathrm{P}\left(\mathrm{X} \mid \mathrm{E}_2\right)=5 \%=\frac{5}{100} ; \mathrm{P}\left(\mathrm{X} \mid \mathrm{E}_3\right)=7 \%=\frac{7}{100}\)

The probability that the defective item was produced by A is given by \(\mathrm{P}(\mathrm{E} \mid \mathrm{A})\).

By using Bayes’ theorem, we obtain

⇒ \(P\left(E_1 \mid X\right)=\frac{P\left(E_1\right) \cdot P\left(X \mid E_1\right)}{P\left(E_1\right) \cdot P\left(X \mid E_1\right)+P\left(E_2\right) \cdot P\left(X \mid E_2\right)+P\left(E_3\right) \cdot P\left(X \mid E_3\right)}\)

= \(\frac{\frac{1}{2} \cdot \frac{1}{100}}{\frac{1}{2} \cdot \frac{1}{100}+\frac{3}{10} \cdot \frac{5}{100}+\frac{1}{5} \cdot \frac{7}{100}}=\frac{\frac{1}{100} \cdot \frac{1}{2}}{\frac{1}{100}\left(\frac{1}{2}+\frac{3}{2}+\frac{7}{5}\right)}=\frac{\frac{1}{17}}{\frac{17}{5}}=\frac{5}{34}\)

Question 12. A card from a pack of 52 cards is lost. From the remaining cards of the pack, two cards are drawn and are found to be both diamonds. Find the probability of the lost card being a diamond.
Solution:

Let E₁ and E₂ be the events that the lost card is a diamond card and a card that is not a diamond respectively.

Let A be the event that two diamond cards are drawn.

Out of 52 cards, 13 cards are diamond and 39 cards are not diamond.

∴ \(\mathrm{P}\left(\mathrm{E}_1\right)=\frac{13}{52}=\frac{1}{4} ; \quad \mathrm{P}\left(\mathrm{E}_2\right)=\frac{39}{52}=\frac{3}{4}\)

When one diamond card is lost, there are 12 diamond cards out of 51 cards.

Two diamond cards can be drawn out of 12 diamond cards in ¹²C₂ ways and 2 cards can be drawn out of 51 cards in ⁵¹C₂, ways. The probability of getting two diamond cards, when one diamond card is lost, is given by P (A|E₁).

⇒ \(\mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_1\right)=\frac{{ }^{12} \mathrm{C}_2}{{ }^{51} \mathrm{C}_2}=\frac{12 !}{2 ! \times 10 !} \times \frac{2 ! \times 49 !}{51 !}=\frac{11 \times 12}{50 \times 51}=\frac{22}{425}\)

When the lost card is not a diamond, there are 13 diamond cards out of 51 cards.

Two diamond cards can be drawn out of 13 diamond cards in ¹³C₂ ways whereas 2 cards can be drawn out of 51 cards in ⁵¹C₂, ways.

The probability of getting two diamond cards, when one card is lost which is not a diamond, is given by P (A|E₂).

∴ \(P\left(A \mid E_2\right)=\frac{{ }^{13} C_2}{{ }^{51} C_2}=\frac{13 !}{2 ! \times 11 !} \times \frac{2 ! \times 49 !}{51 !}=\frac{12 \times 13}{50 \times 51}=\frac{26}{425}\)

The probability of getting two diamond cards, when one card is lost which is not a diamond, is given by P (A|E₂)

∴ \(P\left(A \mid E_2\right)=\frac{{ }^{13} C_2}{{ }^{51} C_2}=\frac{13 !}{2 ! \times 11 !} \times \frac{2 ! \times 49 !}{51 !}=\frac{12 \times 13}{50 \times 51}=\frac{26}{425}\)

The probability that the lost card is a diamond if drawn cards are found to be both diamonds is given by P(E₁lA),

By using Bayes’ theorem, we obtain \(P\left(E_1 \mid A\right)=\frac{P\left(E_1\right) \cdot P\left(A \mid E_1\right)}{P\left(E_1\right) \cdot P\left(A \mid E_1\right)+P\left(E_2\right) \cdot P\left(A \mid E_2\right)}\)

= \(\frac{\frac{1}{4} \cdot \frac{22}{425}}{\frac{1}{4} \cdot \frac{22}{425}+\frac{3}{4} \cdot \frac{26}{425}}=\frac{\frac{1}{425}\left(\frac{22}{4}\right)}{\frac{1}{425}\left(\frac{22}{4}+\frac{26 \times 3}{4}\right)}\)

= \(\frac{\frac{11}{25}}{50}\)

Question 13. The probability that A speaks truth is \(\frac{4}{5}\). A coin is tossed. Reports that a head appears. The probability that actually there was a head is

1. \(\frac{4}{5}\)
2. \(\frac{1}{2}\)
3. \(\frac{1}{5}\)
4. \(\frac{2}{5}\)

Solution: 1. \(\frac{4}{5}\)

Let E₁ and E₂ be the events such that

E₁: A speaks truth, E₂: A speaks lie

Let X be the event that a head appears.

⇒ \(P\left(E_1\right)=\frac{4}{5}\)

∴ \(P\left(E_2\right)=1-P\left(E_1\right)=1-\frac{4}{5}=\frac{1}{5}\)

If a coin is tossed, then it may result in either head (H) or tail (T).

The probability of getting a head is \(\frac{1}{2}\) and the probability of not getting a head is also \(\frac{1}{2}\)

∴ \(P\left(X \mid E_1\right)=P\left(X \mid E_2\right)=\frac{1}{2}\)

The probability that there is actually a head is given by \(\mathrm{P}\left(\mathrm{E}_{\mid} \mathrm{X}\right)\).

⇒ \(P\left(E_1 \mid X\right)=\frac{P\left(E_1\right) \cdot P\left(X \mid E_1\right)}{P\left(E_1\right) \cdot P\left(X \mid E_1\right)+P\left(E_2\right) \cdot P\left(X \mid E_2\right)}=\frac{\frac{4}{5} \cdot \frac{1}{2}}{\frac{4}{5} \cdot \frac{1}{2}+\frac{1}{5}+\frac{1}{2}}=\frac{\frac{1}{2} \cdot \frac{4}{5}}{\frac{1}{2}\left(\frac{4}{5}+\frac{1}{5}\right)}=\frac{\frac{4}{5}}{1}=\frac{4}{5}\)

Therefore, the correct answer is 1.

Question 14. If A and B are two events such that \(A \subset B\) and \(P(B) \neq 0\), then which of the following is correct?

1. \(P(A \mid B)=\frac{P(B)}{P(A)}\)
2. \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})<\mathrm{P}(\mathrm{A})\)
3. \(\mathrm{P}(\mathrm{A} \mid \mathrm{B}) \geq \mathrm{P}(\mathrm{A})\)
4. None of these

Solution: 3. \(\mathrm{P}(\mathrm{A} \mid \mathrm{B}) \geq \mathrm{P}(\mathrm{A})\)

If \(A \subset B\), then \(A \cap B=A\)

⇒ \(\mathrm{P}(\mathrm{A} \cap \mathrm{B})=\mathrm{P}(\mathrm{A})\)

Also, \(\mathrm{P}(\mathrm{A})<\mathrm{P}(\mathrm{B})\)

Consider \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})=\frac{\mathrm{P}(\mathrm{A} \cap \mathrm{B})}{\mathrm{P}(\mathrm{B})}=\frac{\mathrm{P}(\mathrm{A})}{\mathrm{P}(\mathrm{B})} \neq \frac{\mathrm{P}(\mathrm{B})}{\mathrm{P}(\mathrm{A})}\)…..(1)

Again, consider \(P(A \mid B)=\frac{P(A \cap B)}{P(B)}=\frac{P(A)}{P(B)}\)….(2)

It is known that, \(\mathrm{P}(\mathrm{B}) \leq 1 \Rightarrow \frac{1}{\mathrm{P}(\mathrm{B})} \geq 1 \Rightarrow \frac{\mathrm{P}(\mathrm{A})}{\mathrm{P}(\mathrm{B})} \geq \mathrm{P}(\mathrm{A})\)

From (2), we obtain

∴ \(\mathrm{P}(\mathrm{A} \mid \mathrm{B}) \geq \mathrm{P}(\mathrm{A})\)…….(3)

∴ \(P(A \mid B)\) is not less than P(A).

Thus, from (3), it can be concluded that the relation given in alternative 3 is correct.

Probability Exercise 13.4

Random Variable And Its Probability Distribution, Mean Of Random Variable

Question 1. Slate which of the following are not the probability distributions of a random variable? Give reasons for your answer:

Solution:

It is known that the sum of all the probabilities in a probability distribution is one.

1. Sum of the probabilities = 0.4 + 0.4 + 0.2 = 1

Therefore, the given table is a probability distribution of random variables.

2. It can be seen that for X = 3, P (X) = -0.1

It is known that the probability of any observation is not negative. Therefore, the given table is not a probability distribution of random variables.

3. Sum of the probabilities .= 0,6 + 0.1 + 0.2 = 0.9 ≠ 1

Therefore, the given table is not a probability distribution of random variables,

4. Sum of the probabilities = 0.3 + 0,2 + 0.4 + 0.1 + 0.05 = 1.05 ≠ 1

Therefore, the given table is not a probability distribution of random variables.

Question 2. An urn contains 5 red and 2 black balls. Two balls are randomly drawn. Let X represent the number of black balls. What are the possible values of X? Is X a random variable?
Solution:

The two balls selected can be represented as BB, BR, RB, and RR, where B represents a black ball and R represents a red ball.

X represents the number of black balls.

∴ X(BB) = 2; X (BR) = 1; X (RB) = 1; X (RR) = 0

Therefore, the possible values of X are 0, 1, or 2.

Yes, X is a random variable.

Question 3. Let X represent the difference between the number of heads and the number of tails obtained when a coin is tossed 6 times. What are possible values of X?
Solution:

A coin is tossed six times and X represents the difference between the number of heads and the number of tails.

∴ X (6H, 0T) = |6-0| = 6; X (5H, IT) = |5 – 1| = 4

X (4H, 2T) = |4 – 2| = 2; X (3H, 3T) = |3 – 3| = 0

X (2H, 4T) = |2 – 4| = 2; X (1H, 5T) = |1 – 5| = 4

X (0H, 6T) = |0 – 6| = 6

Thus, the possible values of X are 6,4, 2 or 0.

Question 4. Find the probability distribution of

1. Number of heads in two tosses of a coin
2. Number of tails in the simultaneous tosses of three coins
3. Number of heads in four tosses of a coin

Solution:

1. When one coin is tossed twice, the sample space is {HH, HT, TH, TT}

Let X represent the number of heads.

∴ X (HH) = 2, X (HT) = 1, X (TH) = 1, X (TT) = 0

Therefore, X can take the value of 0, 1, or 2.

It is known that,

P(HH) = P(HT) = P(TH) = P(TT} = \(\frac{1}{4}\)

P(X = 0) = P(TT) = \(\frac{1}{4}\)

P(X = 1) = P (HT) + P(TH) = \(\frac{1}{4}\) + \(\frac{1}{4}\) = \(\frac{1}{2}\)

P(X = 2) = P (HH) = \(\frac{1}{4}\)

Thus, the required probability distribution is as follows,

2. When three coins are tossed simultaneously, the sample space is {HHH, HHT, HTH HTT, THH, THT, TTH, TTT}

Let X represent the number of tails.

It can be seen that X can take the value of 0, 1, 2, or 3.

P (X = 0) = P (HHH) = \(\frac{1}{8}\)

P (X = 1) = P (HHT) + P (HTH) + P (THH) = \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) = \(\frac{3}{8}\)

P (X = 2) = P (HTT) + P (THT) + P (TTH) = \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) = \(\frac{3}{8}\)

P (X = 3) = P (TTT) = \(\frac{1}{8}\)

Thus, the probability distribution is as follows.

3. When a coin is tossed four times, the sample space is

S = {HHHH, HHHT, HHTH, HHTT, HTHT, HTHH, HTTH, HTTT, THHH, THHT, THTH, THTT, TTHH, TTHT, TTTH, TTTT}

Let X be the random variable, which represents the number of heads.

It can be seen that X can take the value of 0,1,2, 3, or 4,

P(X = 0) = P(TTTT) = \(\frac{1}{16}\)

p (X = 1) = p (TTTH) + p (TTHT) + P (THTT) + P (HTTT) = \(\frac{1}{16}\) + \(\frac{1}{16}\) + \(\frac{1}{16}\) + \(\frac{1}{16}\) = \(\frac{1}{4}\)

P(X = 2) = P (HHTT) + P (THHT) + P (TTHH) + p (IITTH) + p (HTHT) + P (THTH)

= \(\frac{1}{16}\) + \(\frac{1}{16}\) + \(\frac{1}{16}\) + \(\frac{1}{16}\) + \(\frac{1}{16}\) = \(\frac{1}{16}\) = \(\frac{6}{16}\) = \(\frac{3}{8}\)

P (X = 3) = P (HHHT) + P (HHTH) + P (HTHH) P (THHH)

= \(\frac{1}{16}\) + \(\frac{1}{16}\) + \(\frac{1}{16}\) = \(\frac{4}{16}\) = \(\frac{1}{4}\)

P (X = 4) = P(HHHH)’ = \(\frac{1}{16}\)

Thus, the probability distribution is as follows.

Question 5. Find the probability distribution of the number of successes in two tosses of a die, where success is defined as

1. Number greater than 4
2. Six appear on at least one die

Solution:

When a die is tossed two times, we obtain (6×6) = 36 number of observations.

Let X be the random variable, which represents the number of successes,

1. Here, success refers to the number greater than 4, X can take the value 0, 1 or 2.

P (X = 0) = P (a number less than or equal to 4 on both the tosses) = \(\frac{4}{6}\) x \(\frac{4}{6}\) = \(\frac{4}{9}\)

P (X = 1) = P (number less than or equal to 4 on first toss and greater than 4 on second toss) + P (number greater than 4 on first toss and less than or equal to 4 on second toss)

= \(\frac{4}{6}\) x \(\frac{2}{6}\) + \(\frac{4}{6}\) x \(\frac{2}{6}\) = \(\frac{4}{9}\)

P (X = 2) = P (number greater than 4 on both the tosses) = \(\frac{2}{6}\) x \(\frac{2}{6}\) = \(\frac{1}{9}\)

Thus, the probability distribution is as follows.

2. Here, success means six appears on at least one die, X can take the value 0 or 1.

P (X = 0) = P (six does not appear on any of the dice) = \(\frac{5}{6}\) x \(\frac{5}{6}\) = \(\frac{25}{36}\)

P (X = 1) = P (six appears on at least one of the dice).

Thus, the required probability distribution is as follows.

Question 6. From a lot of 30 bulbs which include 6 defectives, a sample of 4 bulbs is drawn at random with replacement. Find the probability distribution of the number of defective bulbs.
Solution:

It is given that out of 30 bulbs, 6 are defective.

⇒ Number of non-defective bulbs = 30 – 6 = 24

4 bulbs are drawn from the lot with replacement.

Let X be the random variable that denotes the number of defective bulbs In the selected bulbs, X can take the value 0, 1, 2, 3 or 4.

∴ P (X = 0) = P (4 non-defective and 0 defective) = \({ }^4 \mathrm{C}_0 \cdot \frac{4}{5} \cdot \frac{4}{5} \cdot \frac{4}{5} \frac{4}{5}=\frac{256}{625}\)

P (X = 1) = P (3 non-defective and 1 defective) = \({ }^4 \mathrm{C}_1 \cdot\left(\frac{1}{5}\right) \cdot\left(\frac{4}{5}\right)^3=\frac{256}{625}\)

P (x = 2) = P (2 non-defective and 2 detective) = \({ }^4 C_2 \cdot\left(\frac{1}{5}\right)^2 \cdot\left(\frac{4}{5}\right)^2=\frac{96}{625}\)

p (X = 3) = P(1 non-defective and 3 defective) = \({ }^4 \mathrm{C}_3 \cdot\left(\frac{1}{5}\right)^3 \cdot\left(\frac{4}{5}\right)=\frac{16}{625}\)

P (x = 4) = P (0 non-defective and 4 defective)= \({ }^4 \mathrm{C}_4 \cdot\left(\frac{1}{5}\right)^4 \cdot\left(\frac{4}{5}\right)^0=\frac{1}{625}\)

Therefore, the required probability distribution is as follows

Question 7. A coin is biased so that the head is 3 times as likely to occur as the tail. If the coin is tossed twice, find the probability distribution of a number of tails.
Solution:

Let the probability of getting a tail in tossing one biased coin be x.

P (T) = x

⇒ P(H) = 3x

Now, P (T) + P (H) = 1

⇒ x + 3x = 1

⇒ 4x = l

x = \(\frac{1}{4}\)

∴ P (T) = \(\frac{1}{4}\) and P (H) = \(\frac{3}{4}\)

When the coin is tossed twice, the sample space is {HH, TT, HT, TH}.

Let X be the random variable representing the number of tails, Here ‘X’ can be 0, 1 or 2

∴ P(X = 0) – P (no tail) = P(H) x P(H) = \(\frac{3}{4} \times \frac{3}{4}=\frac{9}{16}\)

p (X= 1) = P (one tail) = P (HT) + P (TH) = \(\frac{3}{4} \cdot \frac{1}{4}+\frac{1}{4} \cdot \frac{3}{4}=\frac{3}{8}\)

P (X = 2) = P (two tails) = P (TT) = \(\frac{1}{4} \times \frac{1}{4}=\frac{1}{16}\)

Therefore, the required probability distribution is as follows.

Question 8. A random variable X has the following probability distribution. Determine

1. k
2. P (X < 3)
3. P (X > 6)
4. P (0 < X < 3)

Solution:

1. It is known that the sum of probabilities of a probability distribution of random variables is one.

∴ 0 + k + 2k + 2k + 3k + k² + 2k² + (7k² + k) = 1

⇒ 10k² + 9k – 1 = 0 ⇒(l0k-1)(k + 1) = 0

⇒ k = -1, \(\frac{1}{10}\)

k = -1 is not possible as the probability of an event is never negative.

∴ k = \(\frac{1}{10}\)

2. P (X < 3) = P (X – 0) + P (X = 1) + P (X = 2)

= \(0+\mathrm{k}+2 \mathrm{k}=3 \mathrm{k}=3 \times \frac{1}{10}=\frac{3}{10}\) (because \(\mathrm{k}=\frac{1}{10}\))

3. \(\mathrm{P}(\mathrm{X}>6)=\mathrm{P}(\mathrm{X}=7)=7 \mathrm{k}^2+\mathrm{k}=7 \times\left(\frac{1}{10}\right)^2+\frac{1}{10}=\frac{7}{100}+\frac{1}{10}=\frac{17}{100}\)

4. \(\mathrm{P}(0<\mathrm{X}<3)=\mathrm{P}(\mathrm{X}=1)+\mathrm{P}(\mathrm{X}=2)=\mathrm{k}+2 \mathrm{k}=3 \mathrm{k}=3 \times \frac{1}{10}=\frac{3}{10}\) (because \(\mathrm{k}=\frac{1}{10})\)

Question 9. The random variable X has probability distribution P(X) of the following form, where k is some number:

P(X) = \(=\left\{\begin{array}{cc}
k, & \text { if } x=0 \\
2 k, & \text { if } x=1 \\
3 k & \text { if } x=2 \\
0 & \text { otherwise }
\end{array}\right.\)

1. Determine the value of k.
2. Find P(X < 2), P(X ≤ 2), P(X ≥ 2).

Solution:

1. It is known that the sum of probabilities of a probability distribution of random variables is one.

∴ k + 2k + 3k + 0 = 1 6k = 1 ⇒ k = \(\frac{1}{6}\)

2. P(X < 2) = P(X = 0) + P(X = l) = k + 2k = 3k = \(\frac{3}{6}\) = \(\frac{1}{2}\)

P(X ≤ 2) = P(X = 0) + P(X = 1) + P (X = 2) = k + 2k + 3k + 6k = \(\frac{6}{6}\) = 1

P(X ≥ 2) = P(X = 2) + P (X > 2) = 3k + 0 = 3k = \(\frac{3}{6}\) = \(\frac{1}{2}\)

Question 10. Find the mean number of heads in three tosses of a fair coin.
Solution:

Let X denote the success of getting heads.

Therefore, the sample space is S = {HHH, HHT, HTH, HTT, THH, THT, TTH, TTT} It can be seen that X can take the value of 0, 1, 2 or 3.

P(X = 0) = P (TTT) = \(\frac{1}{8}\)

P(X = 1) = P(getting one head and two tails)

= P(TTH} + P{THT} + P{HTT} = \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) = \(\frac{3}{8}\)

P(X = 2) = P (getting 2 head and 1 tail)

= P(HHT) + P (HTH) + P(THH) = \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) = \(\frac{3}{8}\)

P(X = 3) = P(HHH) = \(\frac{1}{8}\)

Therefore, the required probability distribution is as follows.

Mean \(\mu=\Sigma X_i P\left(X_i\right)=0 \times \frac{1}{8}+1 \times \frac{3}{8}+2 \times \frac{3}{8}+3 \times \frac{1}{8}=\frac{3}{8}+\frac{3}{4}+\frac{3}{8}=\frac{3}{2}=1.5\)

Question 11. Two dice are thrown simultaneously. If X denotes the number of sixes, find the expectation of X.
Solution:

Here, X represents the number of sixes obtained when two dice are thrown simultaneously.

Therefore, X can take the value of 0, 1 or 2.

∴ P(X = 0) = P (not getting six on any of the dice) = \(\frac{25}{36}\)

P(X = 1) = P (six on first die and no six on second die) + P (no six on first die and six on second die) \(\frac{1}{6} \times \frac{5}{6}+\frac{1}{6} \times \frac{5}{6}=2\left(\frac{1}{6} \times \frac{5}{6}\right)=\frac{10}{36}\)

P (X = 2) = P (six on both the dice) = \(\frac{1}{36}\)

Therefore, the required probability distribution is as follows.

Then, expectation of X = \(E(X)=\sum X_i P\left(X_i\right)=0 \times \frac{25}{36}+1 \times \frac{10}{36}+2 \times \frac{1}{36}=\frac{1}{3}\)

Question 12. Two numbers are selected at random (without replacement) from the first six positive integers. Let X denote the larger of the two numbers obtained. Find E(X).
Solution:

The two positive integers can be selected from the first six positive integers without replacement in 6 x 5 = 30 ways.

X represents the larger of the two numbers obtained. Therefore, X can hike the value of 2,3,4, 5 or 6.

For X = 2, the possible observations are (1, 2) and (2, 1).

∴ P(X = 2) = \(\frac{2}{30}\) = \(\frac{1}{15}\)

For X = 3, the possible observations are (1,3), (2, 3), (3,1) and (3,2).

∴ P(X = 3) = \(\frac{4}{30}\) = \(\frac{2}{15}\)

For X = 4, the possible observations are (1, 4), (2, 4), (3, 4), (4, 3), (4, 2) and (4, 1).

∴ P(X = 4) = \(\frac{6}{30}\) = \(\frac{3}{15}\)

For X = 5, the possible observations are (1, 5), (2, 5), (3, 5), (4, 5), (5,4), (5, 3), (5,2) and (5,1).

∴ P(X = 5) = \(\frac{8}{30}\) = \(\frac{4}{15}\)

For X = 6, the possible observations are (1, 6), (2, 6), (3, 6), (4, 6), (5, 6), (6, 5), (6, 4), (6, 3), (6, 2) and (6. 1).

∴ P(X = 6) = \(\frac{10}{30}\) = \(\frac{1}{3}\)

Therefore, the required probability distribution is as follows.

Then, \(E(X)=\Sigma X_i P\left(X_i\right)=2 \cdot \frac{1}{15}+3 \cdot \frac{2}{15}+4 \cdot \frac{1}{5}+5 \cdot \frac{4}{15}+6 \cdot \frac{1}{3}\)

= \(\frac{2}{15}+\frac{2}{5}+\frac{4}{5}+\frac{4}{3}+2=\frac{70}{15}=\frac{14}{3}\)

Choose The Correct Answer

Question 13. The mean of the numbers obtained on throwing a die having written 1 on three faces, 2 on two faces and 5 on one face is

1. 1
2. 2
3. 5
4. 8/3

Solution:

Let X be the random variable representing a number on the die. Here X can be 1,2 or 5

The total number of observations is six.

∴ P(X=1) = \(\frac{3}{6}=\frac{1}{2}\)

P(X=2) = \(\frac{2}{6}=\frac{1}{3}\)

P(X=5) = \(\frac{1}{6}\)

Therefore, the probability distribution is as follows:

Mean = \(E(X)=\Sigma X_i P\left(X_i\right)=1 \times \frac{1}{2}+2 \times \frac{1}{3}+5 \times \frac{1}{6}=\frac{1}{2}+\frac{2}{3}+\frac{5}{6}=\frac{3+4+5}{6}=\frac{12}{6}=2\)

Question 14. Suppose that two cards are drawn at random from a deck of cards. Let X be the number of aces obtained. Then the value of E(X) is

1. \(\frac{37}{221}\)
2. \(\frac{5}{13}\)
3. \(\frac{1}{13}\)
4. \(\frac{2}{13}\)

Solution:

Let X denote the number of aces obtained. Therefore, X can take any of the values of 0, 1, or 2.

In a deck of 52 cards, 4 cards are aces. Therefore, there are 48 non-ace cards.

∴ P (X = 0) = P (0 ace and 2 non-ace cards)

= \(\frac{{ }^4 \mathrm{C}_0 \times{ }^{48} \mathrm{C}_2}{{ }^{52} \mathrm{C}_2}=\frac{1128}{1326}\)

P(X=1) = P(1 ace and 1 non-ace cards)

= \(\frac{{ }^4 \mathrm{C}_1 \times{ }^{48} \mathrm{C}_1}{{ }^{52} \mathrm{C}_2}=\frac{192}{1326}\)

P(X=2)=P(2 ace and 0 non- ace cards)

= \(\frac{{ }^4 \mathrm{C}_2 \times{ }^{48} \mathrm{C}_6}{{ }^{57} \mathrm{C}_2}=\frac{6}{1326}\)

Thus, the probability distribution is as follows.

Then, \(E(X)=\Sigma P\left(X_i\right) \cdot X_i=0 \times \frac{1128}{1326}+1 \times \frac{192}{1326}+2 \times \frac{6}{1326}=\frac{204}{1326}=\frac{2}{13}\)

Therefore, the correct answer is (4).

Probability Miscellaneous Exercise

Question 1. A and B are two events such that P(A)≠0.Find P(B | A), If

1. A is a subset of B
2. A∩B = ø

Solution:

It is given that. P (A) ≠ 0

1. Given A is a subset of B

⇒ \(A \cap B=A \text { i.e. }(A \subset B)\)

∴ \(P(A \cap B)=P(B \cap A)=P(A)\)

∴ \(P(B \mid A)=\frac{P(B \cap A)}{P(A)}=\frac{P(A)}{P(A)}=1\)

2. \(\mathrm{A} \cap \mathrm{B}=\phi \Rightarrow \mathrm{P}(\mathrm{A} \cap \mathrm{B})=0\)

∴ \(P(B \mid A)=\frac{P(A \cap B)}{P(A)}=0\)

Question 2. A couple has two children,

1. Find the probability that both children are males, if it is known that at least one of the children is male,
2. Find the probability that both children are females if it is known that the elder child is a female.

Solution:

If a couple has two children, then the sample space is S = |(b,b),(b,g),(g.b),(g,g)|

1. Let E and F respectively denote the events that both children are males and at least one of the children is a male.

∴ \(\mathrm{E} \cap \mathrm{F}=\{(\mathrm{b}, \mathrm{b})\} \Rightarrow \mathrm{P}(\mathrm{E} \cap \mathrm{F})=\frac{1}{4}\)

∴ \(\mathrm{P}(\mathrm{E})=\frac{1}{4} ; \mathrm{P}(\mathrm{F})=\frac{3}{4} \Rightarrow \mathrm{P}(\mathrm{E} \mid \mathrm{F})=\frac{\mathrm{P}(\mathrm{E} \cap \mathrm{F})}{\mathrm{P}(\mathrm{F})}=\frac{\frac{1}{4}}{\frac{3}{4}}=\frac{1}{3}\)

2. Let A and B respectively denote the events that both children are females and the elder child is a female.

A = \(\{(g, g)\} \Rightarrow P(A)=\frac{1}{4}, B=\{(g, b),(g, g)\} \Rightarrow P(B)=\frac{2}{4}\)

A \(\cap B=\{(g, g)\} \Rightarrow P(A \cap B)=\frac{1}{4}\)

P\((A \mid B)=\frac{P(A \cap B)}{P(B)}=\frac{\frac{1}{4}}{\frac{2}{4}}=\frac{1}{2}\)

Question 3. Suppose that 5% of men and 0.25% of women have grey hair. A grey-haired person is selected at random. What is the probability of this person being male? Assume that there are equal numbers of males and females.
Solution:

Let E₁ and E₂ be the events of male and female persons respectively.

∴ P(E₁) = 0.5 and P(E₂) = 0.5

Let A be the event of a grey-hair person

⇒ P(A | E₁) = Probability of selected a grey-haired male = 5% = 0.05

⇒ P(A | E₂) = Probability of selected a grey-haired female = 0.25% = 0.0025

The probability of the person selected is male if the person is grey-haired

i.e. \(P\left(E_1 | A\right)=\frac{P\left(E_1\right) \cdot P\left(A | E_1\right)}{P\left(E_1\right) \cdot P\left(A \mid E_1\right)+P\left(E_2\right) \cdot P\left(A | E_2\right)}\)

= \(\frac{0.5 \times 0.05}{0.5 \times 0.0025+0.5 \times 0.05}\)

= \(\frac{0.5 \times 0.05}{0.5[0.0025+0.05]}\)

= \(\frac{0.05}{0.0525}=\frac{500}{525}=\frac{20}{21}\)

Question 4. Suppose that 90% of people are right-handed. What is the probability that at most 6 of a random sample of 10 people are right-handed?
Solution:

A person can be either right-handed or left-handed.

It is given that 90% of the people are right-handed.

∴ p = P (Right-handed) = \(\frac{9}{10}\)

q = P (Left-handed) = 1 – \(\frac{9}{10}\) = \(\frac{1}{10}\)

Using the binomial distribution, the probability that more than 6 people are right-handed is given by,

⇒ \(\sum_{\mathrm{r}=7}^{10}{ }^{10} \mathrm{C}_{\mathrm{r}} \mathrm{p}^{\mathrm{T}} \mathrm{q}^{\mathrm{n}-\mathrm{r}}=\sum_{\mathrm{r}=7}^{10}{ }^{10} \mathrm{C}_{\mathrm{r}}\left(\frac{9}{10}\right)^{\mathrm{r}}\left(\frac{1}{10}\right)^{10-\mathrm{r}}\)

Therefore, the probability that at most 6 people are right-handed

= 1-P More than 6 are right-handed

= \(1-\sum_{r=7}^{10}{ }^{10} \mathrm{C}_r(0.9)^r(0.1)^{10-r}\)

Question 5. If a leap year is selected at random, what is the chance that it will contain 53 Tuesdays?
Solution:

In a leap year, there are 366 days, i.e., 52 weeks and 2 days.

In 52 weeks, there are 52 Tuesdays.

Therefore, the probability that the leap year will contain 53

Tuesdays is equal to the probability that one of the remaining 2 days will be Tuesdays.

The remaining 2 days can be S = {Monday Tuesday, Tuesday Wednesday, Wednesday Thursday,

Thursday Friday, Friday Saturday, Saturday Sunday, Sunday Monday}

⇒ n(S) = 7

Favourable cases F = {(Monday, Tuesday), (Tuesday, Wednesday)}

⇒ n(F) = 2

Probability that a leap year will have 53 Tuesdays = \(\frac{2}{7}\)

Question 6. Suppose we have four boxes. A, B, C and D containing coloured marbles as given below:

One of the boxes has been selected at random and a single marble is drawn from it. If the marble is red, what is the probability that it was drawn from box A?, box B?, or box C?

Solution:

Let R be the event of drawing the red marble

Let E_A, E_B and E_C respectively denote the events of selecting the boxes A, B and C.

Total number of marbles = 40

Number of red marbles = 15

∴ P(R) = \(\frac{15}{40}\) = \(\frac{3}{8}\)

The probability of drawing the red marble from box A is given by P(E_A|R).

∴ \(P\left(E_A \mid R\right)=\frac{P\left(E_A \cap R\right)}{P(R)}=\frac{\frac{1}{40}}{\frac{3}{8}}=\frac{1}{15}\)

The probability that the red marble is from box B is P(E_B|R)

∴ \(P\left(E_B \mid R\right)=\frac{P\left(E_B \cap R\right)}{P(R)}=\frac{\frac{6}{40}}{\frac{3}{8}}=\frac{2}{5}\)

The probability that the red marble is from box C is P(E_C|R)

∴ \(P\left(E_C \mid R\right)=\frac{P\left(E_C \cap R\right)}{P(R)}=\frac{\frac{8}{40}}{\frac{3}{8}}=\frac{8}{15}\)

Question 7. Assume that the chances of the patient having a heart attack are 40%. It is also assumed that a meditation and yoga course reduces the risk of heart attack by 30% and prescription of certain drugs reduces its chances by 25%. At a time a patient can choose any one of the two options with equal probabilities. It is given that after going through one of the two options the patient selected at random suffers a heart attack. Find the probability that the patient followed a course of meditation and yoga.
Solution:

Let E₁ and E₂ denote the events that the selected person followed the course of yoga and meditation, and the person adopted the drug prescription, respectively

∴ P(E₁) = P(E₂) = \(\frac{1}{2}\)

Let A be the event that person has a heart attack

∴ P(A) = 0.40

P(A|E₁) = 0.40×0.70 = 0.28

P(A|E₂) = 0.40X0.75 = 0.30

The probability that the patient suffering a heart attack followed a course of meditation and yoga is given by P(E₁|A).

⇒ \(\mathrm{P}\left(\mathrm{E}_1 \mid \mathrm{A}\right)=\frac{\mathrm{P}\left(\mathrm{E}_1\right) \mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_1\right)}{\mathrm{P}\left(\mathrm{E}_1\right) \mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_1\right)+\mathrm{P}\left(\mathrm{E}_2\right) \mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_2\right)}\)

= \(\frac{\frac{1}{2} \times 0.28}{\frac{1}{2} \times 0.28+\frac{1}{2} \times 0.30}=\frac{14}{29}\)

Question 8. If each element of a second-order determinant is either zero or one, what is the probability that the value of the determinant is positive? (Assume that the individual entries of the determinant are chosen independently, each value being assumed with probability \(\frac{1}{2}\)).
Solution:

The total number of determinants of second order with each element being 0 or 1 is (2)⁴ =16

The value of the determinant is positive in the following cases, \(\left\{\left|\begin{array}{ll}
1 & 0 \\
0 & 1
\end{array}\right|,\left|\begin{array}{ll}
1 & 1 \\
0 & 1
\end{array}\right|,\left|\begin{array}{ll}
1 & 0 \\
1 & 1
\end{array}\right|\right\}\)

Required probability = \(\frac{3}{16}\)

Question 9. An electronic assembly consists of two subsystems, say A and B. From previous testing procedures, the following probabilities are assumed to be known:

1. P (A fails) = 0.2
2. P (B fails alone) = 0,15
3. P (A and B fail) = 0.15

Evaluate the following probabilities

1. P (A fails | B has failed)
2. P (A fails alone)

Solution:

Let the event in which A fails and B fails to be denoted by E_A and E_B.

P(E_A)=0.2

P(E_A∩E_B) = 0.15

P (B fails alone) = P (E_B) – P(E_A∩E_B)

∴ 0.15 = P(E_B)-0.15

⇒ P(E_B) = 0.3

1. \(P\left(E_A \mid E_B\right)=\frac{P\left(E_A \cap E_B\right)}{P\left(E_B\right)}\) = \(\frac{0.15}{0.3}=0.5\)
2. \(\mathrm{P}(\mathrm{A} \text { fails alone })=\mathrm{P}\left(\mathrm{E}_{\mathrm{A}}\right)-\mathrm{P}\left(\mathrm{E}_{\mathrm{A}} \cap \mathrm{E}_{\mathrm{B}}\right)\) =0.2-0.15=0.05

Question 10. Bag 1 contains 3 red and 4 black balls and Bag 2 contains 4 red and 5 black balls. One ball is transferred from Bag 1 to Bag 2 and then a ball is drawn from Bag 2. The ball so drawn is found to be red in colour. Find the probability that the transferred ball is black.
Solution:

Let E₁ and E₂ respectively denote the events that a red ball is transferred from bag 1 to 2 and a black ball is transferred from bag 1 to 2.

⇒ \(\mathrm{P}\left(\mathrm{E}_1\right)=\frac{3}{7} \text { and } \mathrm{P}\left(\mathrm{E}_2\right)=\frac{4}{7}\)

Let A be the event that the ball drawn is red.

When a red ball is transferred from bag 1 to 2, \(\mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_1\right)=\frac{5}{10}=\frac{1}{2}\)

When a black ball Is transferred from bag 1 to 2, \(\mathrm{P}\left(\mathrm{A} \mid \mathrm{E}_2\right)=\frac{4}{10}=\frac{2}{5}\)

The probability that the transferred ball is black in colour when the ball is drawn is red in colour is given by P(E₂|A)

By using Baye’s theorem, we obtain

∴ \(P\left(E_2 \mid A\right)=\frac{P\left(E_2\right) P\left(A \mid E_2\right)}{P\left(E_1\right) P\left(A \mid E_1\right)+P\left(E_2\right) P\left(A \mid E_2\right)}\)

= \(\frac{\frac{4}{7} \times \frac{2}{5}}{\frac{3}{7} \times \frac{1}{2}+\frac{4}{7} \times \frac{2}{5}}=\frac{16}{31}\)

Choose The Correct Answer

Question 11. If A and B are two events such that \(P(A) \neq 0\) and \(P(B \mid A)=1\), then.

1. \(\mathrm{A} \subset \mathrm{B}\)
2. \(\mathrm{B} \subset \mathrm{A}\)
3. \(\mathrm{B}=\phi\)
4. \(\mathrm{A}=\phi\)

Solution: 1. \(\mathrm{A} \subset \mathrm{B}\)

P\((\mathrm{A}) \neq 0\) and \(\mathrm{P}(\mathrm{B} \mid \mathrm{A})=1\)

Now, \(\mathrm{P}(\mathrm{B} \mid \mathrm{A})=\frac{\mathrm{P}(\mathrm{B} \cap \mathrm{A})}{\mathrm{P}(\mathrm{A})}\)

1 = \(\frac{P(B \cap A)}{P(A)}\)

⇒ \(P(A)=P(B \cap A) \Rightarrow A \subset B\)

Thus, the correct answer is 1.

Question 12. If P\((\mathrm{A} \mid \mathrm{B})>\mathrm{P}(\mathrm{A})\), then which of the following is correct:

1. \(\mathrm{P}(\mathrm{B} \mid \mathrm{A})<\mathrm{P}(\mathrm{B})\)
2. \(\mathrm{P}(\mathrm{A} \cap \mathrm{B})<\mathrm{P}(\mathrm{A}) \cdot \mathrm{P}\)
3. \(\mathrm{P}(\mathrm{B} \mid \mathrm{A})>\mathrm{P}(\mathrm{B})\)
4. \(\mathrm{P}(\mathrm{B} \mid \mathrm{A})=\mathrm{P}(\mathrm{B})\)

Solution: 3. \(\mathrm{P}(\mathrm{B} \mid \mathrm{A})>\mathrm{P}(\mathrm{B})\)

⇒ \(\mathrm{P}(\mathrm{A} \mid \mathrm{B})>\mathrm{P}(\mathrm{A})\)

⇒ \(\frac{P(A \cap B)}{P(B)}>P(A)\)

⇒ \(P(A \cap B)>P(A) \cdot P(B)\)

⇒ \(\frac{P(A \cap B)}{P(A)}>P(B)\)

⇒ \(P(B \mid A)>P(B)\)

Thus, the correct answer is (3).

Question 13. If A and B are any two events such that P (A) + P (B) – P (A and B) = P (A), then

1. P (B|A) = 1
2. P (A]B) = 1
3. P (B|A) = 0
4. P (A|B) = 0

Solution:

P (A) + P (B) – P (A and B) = P(A)

⇒ P(A) + P(B)-P(A∩B)=P(A)

⇒ P(B)-P(A∩B) = 0

⇒ P(A∩B)=P(B)

Thus, the correct answer is (2).

 

 

Linear Programming Exercise 12.1

Solve the following Linear Programming problems graphically.

Question 1. Maximize Z = 3x+4y,

Subject to the constraints x+y≤4, x≥0 and y≥0.

Solution:

We have to

Maximize Z = 3x + 4y

Subject to constraints x + y≤4, x≥0, y≥0

Firstly, draw the graph of the line x+y = 4

Then, putting (0, 0) in the inequality x+y≤4 we have 0 + 0≤4

⇒ ≤4 (Which is true)

So, the half-plane is towards the origin.

Since, x,y≥0

So, the feasible region lies in the first quadrant.

∴ The feasible region is OABO.

The comer points of the feasible region are 0(0,0), A(4,0), and B(0,4), The values of Z at these points are as follows:

Therefore, the maximum value of Z is 16 at the point B(0,4).

Question 2. Minimize Z = -3x + Ay, subject to constraints x + 2y≤8,3x + 2y≤1 2,x≥0 and y≥0.
Solution:

We have to

Minimize Z = -3x+4y

Subject to constraints x+2y≤8, 3x + 2y≤12, x≥0, y≥0

Firstly, draw the graph of the line, x + 2y = 8

Putting (0, 0) in the inequality x + 2y≤8, we have 0 + 0≤8

⇒ 0≤8 (Which is true)

Read and Learn More Class 12 Maths Chapter Wise with Solutions

So, the half-plane is towards the origin.

Since, x,y≥0

So, the feasible region lies in the first quadrant.

Secondly, draw the graph of the line, 3x + 2y = 12

Putting (0, 0) in the inequality 3x + 2y≤12 we have 3 x 0 + 2 x 0 ≤ 12

⇒ 0 ≤12 (Which is true)

So, the half-plane is towards the origin.

∴ Feasible region is OABCO.

On solving equations x + 2y = 8 and 3x+2y = 12, we get x = 2 and y = 3

∴ Intersection point B is (2,3)

The corner points of the feasible region are 0(0,0), A(4,0), B(2,3)and C(0,4).

The values of Z at these points are as follows.

Therefore, the minimum value of Z is -12 at the point A(4,0).

Question 3. Maximize Z = 5x + 3y, subject to constraints 3x + 5y≤ 15. 5x + 2y≤10. x≥0 and y≥0.
Solution:

We have to

Maximize Z = 5x + 3y

Subject to constraints 3x + 5y≤15, 5x + 2y≤10, x≥0, y≥0

Firstly, draw the graph of the line. 3x + 5y = 15

Putting (0, 0) in the inequality 3x + 5y≤15. we have 3 x 0 + 5 x 0≤15

⇒ 0≤15 (Which is true)

So, the half-plane is towards the origin.

Since, x,y ≥ 0 So, the feasible region lies in the first quadrant.

Secondly, draw the graph of the line, 5x + 2y≤10

Putting (0, 0) in the inequality 5x + 2y≤10 we have 5 x 0 + 2 x 0 ≤ 10

⇒ 0≤10 (Which is true)

So, the half-plane is towards the origin.

On solving equations, 3x + 5y = 15 and 5x + 2y = 10, we get x = 20/19 and y = 45/19

Coordinates of point B is \(\left(\frac{20}{19}, \frac{45}{19}\right)\)

∴ The feasible region is OABCO

The corner points of the feasible region are 0(0,0), A(2,0), B\(\left(\frac{20}{19}, \frac{45}{19}\right)\) and C(0,3)

The values of Z at these points are as follows:

Therefore, the maximum value of Z is \(\frac{235}{19}\) at the point B\(\left(\frac{20}{19}, \frac{45}{19}\right)\)

Question 4. Minimize Z = 3x + 5y subject to constraints x + 3y≥3, x+y≥2 and x,y≥0.
Solution:

We have to

Minimize Z = 3x + 5y

Subject to constraints x + 3y≥3, x+y≥2, x≥0, y≥0

Firstly, draw the graph of the line, x + 3y = 3

Putting (0, 0) in the inequality x + 3y≥3, we have 0 + 3 x 0 ≥ 3

⇒ 0≥3 (Which is false)

So, the half-plane is away from the origin. Since, x,y≥0 So, the feasible region lies in the first quadrant.

Secondly, draw the graph of the line, x+y = 2

Putting (0, 0) in the inequality x + y≥2 we have 0 + 0≥2

⇒ G≥2 (Which is false)

So, the half-plane is away from the origin. It can be seen that the feasible region is unbounded.

On solving equations x+y = 2 and x + 3y = 3, we get x = 3/2 and y = 1/2

∴ Intersection point is B\(\left(\frac{3}{2}, \frac{1}{2}\right)\)

The corner points of the feasible region axe A(3,0), B\(\left(\frac{3}{2}, \frac{1}{2}\right)\) and C(0,2).

The values of Z at these points are as follows:

As the feasible region is unbounded, therefore, 7 may or may not be the minimum value of Z.

For this, we draw the graph of the inequality, 3x + 5y < 7, and check whether the resulting half-plane has points in common with the feasible region or not.

It can be seen that the feasible region has no common point with 3x + 5y < 7.

Therefore, the maximum value of Z is 7 at point B\(\left(\frac{3}{2}, \frac{1}{2}\right)\)

Question 5. Maximize Z = 3x + 2y, subject to constraints x + 2y≤10, 3x + y≤15and x,y≥0.
Solution:

We have to

Maximize Z = 3x + 2y

Subject to constraints x + 2y≤10, 3x + y≤15, x≥0, y≥0

Firstly, draw the graph of the line, x + 2y = 10

Putting (0, 0) in the inequality x + 2y≤10, we have 0 + 2 x 0≤10

⇒ 0 ≤10 (Which is true)

So, the half-plane is towards the origin.

Since, x,y≥0

So, the feasible region lies in the first quadrant.

Secondly, draw the graph of the line, 3x+y = 15

Putting (0, 0) in the inequality 3x+y≤15 we have 3 x 0 + 0 ≤ 15

⇒ 0 ≤ 15 (Which is true)

So, the half-plane is towards the origin.

On solving equations x + 2y = 10 and 3x + y = 15, we get x = 4 and y = 3

∴ Intersection point B is (4,3)

∴ The feasible region is OABCO.

The corner points of the feasible region are 0(0,0), A(5,0), B(4,3)and C(0,5). The values of Z at these points are as follows:

Therefore, the maximum value of Z is 18 at the point B(4,3).

Question 6. Minimize Z = x + 2y, subject to constraints are 2x+y≥3, x + 2y≥6 and x,y≥0. Show that the minimum of Z occurs at more than two points.
Solution:

We have to

Minimize Z = x + 2y

Subject to constraints 2x+y≥3, x + 2y≥6 , x≥0, y≥ 0

Firstly, draw the graph of the line, 2x+y = 3

Putting (0, 0) in the inequality 2x+y≥3, we have 2 x 0 + 0≥3

⇒ 0≥3 (Which is false)

So, the half-plane is away from the origin.

Since, x, y≥0 So, the feasible region lies in the first quadrant.

Secondly, draw the graph of the line, x + 2y = 6

Putting (0, 0) in the inequality x+2y≥6 we have 0 + 2 x 0 ≥ 6

⇒ 0≥6 (Which is false) So. the half plane is away from the origin.

The intersection point of the lines x + 2y = 6 and 2x + y = 3 is B (0,3)

The corner points of the feasible region are A(6,0), and B(0,3). The values of Z at these points are as follows:

As the feasible region is unbounded, therefore, Z = 6 may or may not be the minimum value. For this, we draw the inequality, x+2y<6, and check whether the resulting half-plane has points in common with the feasible region or not.

Here there is no common point between the unbounded feasible region and the open half plane Therefore, the value of Z is minimum at every point on the line, x+2y = 6.

Question 7. Minimize and maximize Z = 5x + 10y subject to constraints are x+2y≤120, x+y≥60, x-2y≥0and x,y≥0.
Solution:

We have to

Minimize and maximize Z = 5x +10y

Subject to constraints x+2y≤120, x+y≥60, x,y≥0, x-2y≥0

Firstly, draw the graph of the line, x+2y = 120

Putting (0, 0) in the inequality x + 2y≤120, we have 0 + 2 x 0≤120 ⇒ 0≤120 (Which is true) So, the half-plane is towards the origin.

Secondly, draw the graph of the line, x+y = 60

Putting (0, 0) in the inequality x+y≥60, we have 0 + 0≥60

⇒ 0≥60 (Which is false)

So, the half-plane is away from the origin.

Thirdly, draw the graph of the line x- 2y = 0

Putting (5, 0) in the inequality x-2y≥0 we have 5 – 2 x 0 ≥ 0

⇒ 5≥0 (Which is true)

So, the half-plane is towards the A-axis. Since, x,y≥0

So, the feasible region lies in the first quadrant.

∴ The feasible region is ABCDA.

On solving equations x – 2y = 0 and x + y = 60, we get D(40,20)

And on solving equations x-2v = 0 and x+2y = 120 , we get C(60,30)

The corner points of the feasible region are, A(60,0), B(120,0), C(60,30), and D(40,20).

The values of Z at these points are as follows:

The minimum value of Z is 300 at A (60,0) and the maximum value of Z is 600 at all the points on the line segment joining the points B (120, 0) and C (60, 30).

Question 8. Minimize and maximize Z = x + 2y subject to constraints are x + 2y≥100, 2x -y≤ 0,
2x + y≤200 and x,y≥0.
Solution:

We have to

Minimize and maximize Z = x+2y

Subject to constraints x+2y≥100, 2x-y≤ 0, 2x + y≤200, x≥0, y≥ 0

Firstly, draw the graph of the line, x + 2y = 100

Putting (0, 0) in the inequality x + 2y≥100, we have 0 + 2×0≥100

⇒ 0≥100 (Which is false)

So, the half-plane is away from the origin.

Secondly, draw the graph of the line, 2x -y = 0

Putting (5, 0) in the inequality 2x -y≤0

we have 2 x 5 – 0 ≤ 0

⇒ 10≤0 (Which is false)

So, the half-plane is towards the Y-axis.

Thirdly, draw the graph of the line 2x+y = 200

Putting (0, 0) in the inequality 2x+y≤200 we have 2 x 0 + 0 ≤ 200 ⇒ 0 < 200 (Which is true)

So, the half-plane is towards the origin. Since, x,y ≥ 0 So, the feasible region lies in the first quadrant.

On solving equations 2x-y = 0 and x + 2y = 100 , we get B(20,40)

And on solving equations2x-y = 0and 2x+y = 200 , we get C(50, l00)

∴ The feasible region is ABCDA.

The corner points of the feasible region are, A (0,50), B(20,40), C(50,100), and (0,200).

The values of Z at these points are as follows:

The maximum value of Z is 400 at D(0,200)and the minimum value of Z is 100 at all the points on the line segment joining A(0,50)and B(20,40).

Question 9. Maximize Z = -x + 2y, subject to the constraints x≥3, x + y≥5,x + 2y≥6 and y≥0.
Solution:

We have to

Maximize Z = -x+2y

Subject to constraints x≥3, x + y≥5, x+2y≥6, x≥0, y≥0

Firstly, draw the graph of the line, x+y = 5

Putting (0, 0) in the inequality x+y≥5, we have 0 + 0≥5

⇒ 0≥5 (Which is false)

So, the half-plane is away from the origin.

Secondly, draw the graph of the line, x+2y = 6

Putting (0, 0) in the inequality x + 2y≥6, we have 0 + 2 x 0≥6

⇒ 0≥6 (Which is false)

So, the half-plane is away from the origin.

It can be seen that the feasible region is unbounded.

The corner points of the feasible region are A(6,0), B(4,1) and C(3,2).

The values of Z at these points are as follows;

As the feasible region is unbounded, therefore, Z = 1 may or may not be the maximum value.

For this, we draw the inequality, -x + 2y > 1, and check whether the resulting half-plane has points in common with the feasible region or not.

The resulting feasible region has points in common with the feasible region.

Therefore, Z = 1, is not the maximum value.

Hence, Z has no maximum value.

Question 10. Maximize Z = x+y, subject to constraints are x-y≤-1, -x + y≤0 and x, y≥0.
Solution:

We have to

Maximize Z = x+y

Subject to constraints x-y≤-1, -x + y≤0, x ≥0, y ≥ 0

Firstly, draw the graph of the line, x-y = -1

Putting (0, 0) in the inequality x-y ≤ -1, we have 0-0≤-1

⇒ 0≤-1 (Which is false)

So, the half-plane is away from the origin.

Secondly, draw the graph of the line, -x + y = 0

Putting (2, 0) in the inequality-x+y ≤ 0 we have -2 + 0≤0

⇒ -2≤0 (Which is true)

So, the half-plane is towards the X-axis.

Since, x,y≥0

So, the feasible region lies in the first quadrant.

From the above graph, it is clearly shown that there is no common region. Hence, there is no feasible region and thus Z has no maximum value.

 

 

 

Three-Dimensional Geometry Exercise 11.1

Question 1. If a line makes angles 90°, 135°, and 45° with x, y, and z-axes respectively, find its direction cosines. Sol. Let the direction cosines of the line be l, m, and n.
Solution:

Let the direction cosines of the line be l, m, and n.

l = \(\cos 90^{\circ}=0 ; m=\cos \left(180^{\circ}-45^{\circ}\right)=-\cos 45^{\circ}=-\frac{1}{\sqrt{2}} ; \mathrm{n}=\cos 45^{\circ}=\frac{1}{\sqrt{2}}\)

Therefore, the direction cosines of the line are \(0,-\frac{1}{\sqrt{2}}\) and \(\frac{1}{\sqrt{2}}\)

Therefore, the direction cosines of the line are 0,\(-\frac{1}{\sqrt{2}}\) and \(\frac{1}{\sqrt{2}}\)

Question 2. Find the direction cosines of a line which makes equal angles with the coordinate axes.
Solution:

Let the line make an angle ‘α’ with each of the coordinate axes.

∴ l = \(\cos \alpha, \mathrm{m}=\cos \alpha, \mathrm{n}=\cos \alpha\)

∴ \(l^2+\mathrm{m}^2+\mathrm{n}^2=1\)

⇒ \(\cos ^2 \alpha+\cos ^2 \alpha+\cos ^2 \alpha=1 \Rightarrow 3 \cos ^2 \alpha=1 \Rightarrow \cos ^2 \alpha=\frac{1}{3} \Rightarrow \cos \alpha= \pm \frac{1}{\sqrt{3}}\)

Thus, the direction cosines of the line, which is equally inclined to the coordinate axes, are \(\pm \frac{1}{\sqrt{3}}, \pm \frac{1}{\sqrt{3}}\), and \(\pm \frac{1}{\sqrt{3}}\)

Question 3. If a line has the direction ratios -18, 12, – 4, then what are its direction cosines?
Solution:

If a line has direction ratios of -18, 12, and -4, then its direction cosines are \(\frac{-18}{\sqrt{(-18)^2+(12)^2+(-4)^2}}, \frac{12}{\sqrt{(-18)^2+(12)^2+(-4)^2}}, \frac{-4}{\sqrt{(-18)^2+(12)^2+(-4)^2}}\)

i.e., \(\frac{-18}{22}, \frac{12}{22}, \frac{-4}{22} \Rightarrow \frac{-9}{11}, \frac{6}{11}, \frac{-2}{11}\)

Thus, the direction cosines are \(\frac{-9}{11}, \frac{6}{11}\), and \(\frac{-2}{11}\)

Read and Learn More Class 12 Maths Chapter Wise with Solutions

Question 4. Show that the points (2, 3, 4), (-1, -2, 1), (5, 8, 7) are collinear.
Solution:

The given points are A (2, 3, 4), B (- 1, – 2, 1), and C (5, 8, 7).

It is known that the direction ratios of lines joining the points, (x₁, y₁, z₁) and (x₂, y₂, z₂), are given by, (x₂-x₁, y₂-y₁, z₂-z₁).

The direction ratios of AB are a₁= (-1 – 2), b₁= (-2 – 3), and c₁= (1 -4) i.e., a₁ = -3, b₁= -5, and c₁= – 3.

The direction ratios of BC are a₂= (5 – (- 1)), b₂ = (8 – (- 2)), and c₂= (7-1) i.e., a₂ = 6, b₂= 10, and c₂= 6.

∴ \(\frac{\mathrm{a}_1}{\mathrm{a}_2}=\frac{\mathrm{b}_1}{\mathrm{~b}_2}=\frac{\mathrm{c}_1}{\mathrm{c}_2}=-\frac{1}{2}\) i.e. they are proportional.

Therefore, AB is parallel to BC. Since point B is common to both AB and BC, points A, B, and C are collinear.

Question 5. Find the direction cosines of the sides of the triangle whose vertices are (3, 5, – 4), (-1,1,2), and (-5,-5,-2)
Solution:

The vertices of ΔABC are A (3, 5, -4), B (-1, 1,2), and C (-5, -5, -2)

The direction ratios of side AB are (-1 -3), (1 -5), and (2 -(-4)) i.e., (- 4, -4, 6).

Therefore, the direction cosines of AB are \(\frac{-4}{\sqrt{(-4)^2+(-4)^2+(6)^2}}, \frac{-4}{\sqrt{(-4)^2+(-4)^2+(6)^2}}, \frac{6}{\sqrt{(-4)^2+(-4)^2+(6)^2}}\)

⇒ \(\frac{-4}{2 \sqrt{17}}, \frac{-4}{2 \sqrt{17}}, \frac{6}{2 \sqrt{17}}=\frac{-2}{\sqrt{17}}, \frac{-2}{\sqrt{17}}, \frac{3}{\sqrt{17}}\)

The direction ratios of BC are (-5-(-1)), (-5-1), and (-2-2) i.e., (-4,-6,-4). Therefore, the direction cosines of BC are \(\frac{-4}{\sqrt{(-4)^2+(-6)^2+(-4)^2}}, \frac{-6}{\sqrt{(-4)^2+(-6)^2+(-4)^2}}, \frac{-4}{\sqrt{(-4)^2+(-6)^2+(-4)^2}}\)

= \(\frac{-4}{2 \sqrt{17}}, \frac{-6}{2 \sqrt{17}}, \frac{-4}{2 \sqrt{17}}=\frac{-2}{\sqrt{17}}, \frac{-3}{\sqrt{17}}, \frac{-2}{\sqrt{17}}\)

The direction ratios of AC are (-5-3),(-5-5), and (-2-(-4)) i.e., (-8,-10 and 2). Therefore, the direction cosines of AC are \(\frac{-8}{\sqrt{(-8)^2+(-10)^2+(2)^2}}, \frac{-10}{\sqrt{(-8)^2+(-10)^2+(2)^2}}, \frac{2}{\sqrt{(-8)^2+(-10)^2+(2)^2}}\)

= \(\frac{-8}{2 \sqrt{42}}, \frac{-10}{2 \sqrt{42}}, \frac{2}{2 \sqrt{42}}=\frac{-4}{\sqrt{42}}, \frac{-5}{\sqrt{42}}, \frac{1}{\sqrt{42}}\)

Three-Dimensional Geometry Exercise 11.2

Question 1. Show that the three lines with direction cosines \(\frac{12}{13}, \frac{-3}{13}, \frac{-4}{13}; \frac{4}{13}, \frac{12}{13}, \frac{3}{13}; \frac{3}{13}, \frac{-4}{13}, \frac{12}{13}\) are mutually perpendicular.
Solution:

Two lines with direction cosines, l₁, m₁, n₁, and l₂, m₂, n₂, are perpendicular to each other, if l₁l₂ + m₁m₂ + n₁n₂ = 0

1. For the lines with direction cosines, \(\frac{12}{13}, \frac{-3}{13}, \frac{-4}{13}\) and \(\frac{4}{13}, \frac{12}{13}, \frac{3}{13}\), we obtain

∴ \(l_1 l_2+m_1 m_2+n_1 n_2=\left(\frac{12}{13}\right) \times\left(\frac{4}{13}\right)+\left(\frac{-3}{13}\right) \times\left(\frac{12}{13}\right)+\left(\frac{-4}{13}\right) \times\left(\frac{3}{13}\right)\)

= \(\frac{48}{169}-\frac{36}{169}-\frac{12}{169}=0\)

Therefore, the lines are perpendicular

2. For the lines with direction cosines, \(\frac{4}{13}, \frac{12}{13}, \frac{3}{13}\) and \(\frac{3}{13}=\frac{-4}{13}, \frac{12}{13}\), we obtain

∴ \(l_1 l_2+m_1 m_2+n_1 n_2=\left(\frac{4}{13}\right) \times\left(\frac{3}{13}\right)+\left(\frac{12}{13}\right) \times\left(\frac{-4}{13}\right)+\left(\frac{3}{13}\right) \times\left(\frac{12}{13}\right)\)

= \(\frac{12}{169}-\frac{48}{169}+\frac{36}{169}=0\)

Therefore, the lines are perpendicular,

3. For the lines with direction cosines, \(\frac{3}{13}, \frac{-4}{13}, \frac{12}{13}\) and \(\frac{12}{13}, \frac{-3}{13}, \frac{-4}{13}\), we obtain

∴ \(l_1 l_2+\mathrm{m}_1 \mathrm{~m}_2+\mathrm{n}_1 \mathrm{n}_2=\left(\frac{3}{13}\right) \times\left(\frac{12}{13}\right)+\left(\frac{-4}{13}\right) \times\left(\frac{-3}{13}\right)+\left(\frac{12}{13}\right) \times\left(\frac{-4}{13}\right)\)

= \(\frac{36}{169}+\frac{12}{169}-\frac{48}{169}=0\)

Therefore, the lines are perpendicular.

Thus, all lines are mutually perpendicular.

Question 2. Show that the line through the points (1, -1,2) and (3, 4, -2) is perpendicular to the line through the points (0, 3, 2) and (3, 5, 6).
Solution:

Let AB be the line joining the points, (1, -1, 2) and (3, 4, -2), and CD be the line joining the points, (0, 3, 2) and (3, 5, 6).

The direction ratios, a₁, b∴ ₁, c₁, of AB are (3 -1), (4 – (-1)), and (-2 -2) i.e., (2, 5, – 4).

The direction ratios, a₂, b₂, c₂, of CD are (3 – 0), (5 -3), and (6 -2) i.e., (3, 2, 4).

AB and CD will be perpendicular to each other if a₁a₂ + b₁b₂ + c₁c₂ = 0 ⇒ a₁a₂ + b₁b₂ + c₁c₂ = 2 x 3 + 5 x 2 + (-4) x 4= 6 + 10 -16 = 0

Therefore, AB and CD are perpendicular to each other.

Question 3. Show that the line through the points (4, 7, 8), (2, 3, 4) is parallel to the line through the points (-1,-2, 1), (1,2, 5).
Solution:

Let AB be the line through the points, (4, 7, 8) and (2, 3, 4), and CD be the line through the points, (-1, -2, 1) and (1, 2, 5).

The directions ratios, a₁, b₁, c₁ of AB are (2 – 4), (3 -7), and (4 -8) i.e., (- 2, – 4, – 4).

The direction ratios, a₂, b₂, c₂ of CD are (1 – (-1)), (2 – (-2)), and (5 – 1) i.e., (2, 4, 4).

AB will be parallel to CD, if \(\frac{a_1}{a_2}=\frac{b_1}{b_2}=\frac{c_1}{c_2}\)

⇒ \(\frac{\mathrm{a}_1}{\mathrm{a}_2}=\frac{-2}{2}=-1, \frac{\mathrm{b}_1}{\mathrm{~b}_2}=\frac{-4}{4}=-1\)

and \(\frac{\mathrm{c}_1}{\mathrm{c}_2}=\frac{-4}{4}=-1\)

∴ \(\frac{\mathrm{a}_1}{\mathrm{a}_2}=\frac{\mathrm{b}_1}{\mathrm{~b}_2}=\frac{\mathrm{c}_1}{\mathrm{c}_2}=-1\)

Thus, AB is parallel to CD.

Question 4. Find the equation of the line that passes through the point (1, 2, 3) and is parallel to the vector \(3 \hat{i}+2 \hat{j}-2 \hat{k}\)
Solution:

It is given that the line passes through the point A(1,2,3). Therefore, the position vector point A is \(\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}+2 \hat{\mathrm{j}}+3 \hat{\mathrm{k}}\).

Let, \(\overrightarrow{\mathrm{b}}=3 \hat{\mathrm{i}}+2 \hat{\mathrm{j}}-2 \hat{\mathrm{k}}\)

It is known that the line which passes through point A and parallel to \(\vec{b}\) is given by \(\vec{r}=\vec{a}+\lambda \vec{b}\), where \(\lambda\) is a constant \(\vec{r}=\hat{i}+2 \hat{j}+3 \hat{k}+\lambda(3 \hat{i}+2 \hat{j}-2 \hat{k})\).

This is the required equation of the line.

Question 5. Find the equation of the line in vector and in Cartesian form’ that passes through the point with position vector \(2 \hat{\mathrm{i}}-\hat{\mathrm{j}}+4 \hat{\mathrm{k}}\) and is in the direction \(\hat{\mathrm{i}}+2 \hat{\mathrm{j}}-\hat{\mathrm{k}}\).
Solution:

⇒ \(\vec{a}=2 \hat{i}-\hat{j}+4 \hat{k}\) and \(\vec{b}=\hat{i}+2 \hat{j}-\hat{k}\)

It is known that a line through a point with position vector \(\vec{a}\) and parallel to \(\vec{b}\) is given by the equation \(\overrightarrow{\mathrm{r}}=\overrightarrow{\mathrm{a}}+\lambda \overrightarrow{\mathrm{b}} \Rightarrow \overrightarrow{\mathrm{i}}=2 \hat{\mathrm{i}}-\hat{\mathrm{j}}+4 \hat{\mathrm{k}}+\lambda(\hat{\mathrm{i}}+2 \hat{\mathrm{j}}-\hat{\mathrm{k}})\)

This is the required equation of the line in vector form.

⇒ \(\vec{r}=x \hat{i}+y \hat{j}+z \hat{k} \Rightarrow x \hat{i}+y \hat{j}+z \hat{k}=(\lambda+2) \hat{i}+(2 \lambda-1) \hat{j}+(-\lambda+4) \hat{k}\)

Eliminating \(\lambda\), we obtain the Cartesian form equation as \(\frac{x-2}{1}=\frac{y+1}{2}=\frac{z-4}{-1}\)

This is the required equation of the given time in Cartesian form.

Question 6. Find the Cartesian equation of the line which passes through the point (-2, 4, -5) and parallel to \(\frac{x+3}{3}=\frac{y-4}{5}=\frac{z+8}{6}\)
Solution:

It is given that the line passes through the point (-2, 4, -5) and is parallel to \(\frac{x+3}{3}=\frac{y-4}{5}=\frac{z+8}{6}\)

The direction rations of the line, \(\frac{x+3}{3}=\frac{y-4}{5}=\frac{z+8}{6}\) are (3, 5, 6).

The required line is parallel to \(\frac{x+3}{3}=\frac{y-4}{5}=\frac{z+8}{6}\)

Therefore, its direction ratios are 3k, 5k, and 6k, where k ≠ 0

It is known that the equation of the line through the point (x₁, y₁, z₁) and with direction ratios, a, b, c is given by \(\frac{x-x_1}{a}=\frac{y-y_1}{b}=\frac{z-z_1}{c}\)

Therefore the equation of the required line is \(\frac{x+2}{3 k}=\frac{y-4}{5 k}=\frac{z+5}{6 k} \Rightarrow \frac{x+2}{3}=\frac{y-4}{5}=\frac{z+5}{6}=k\)

Question 7. The Cartesian equation of a line is \(\frac{x-5}{3}=\frac{y+4}{7}=\frac{z-6}{2}\). Write its Vector form.
Solution:

The Cartesian equation of the line is \(\frac{x-5}{3}=\frac{y+4}{7}=\frac{z-6}{2}\)

The given line passes through the point (5, -4, 6), The position vector of this point is \(\overrightarrow{\mathrm{a}}=5 \hat{\mathrm{i}}-4 \hat{\mathrm{j}}+6 \hat{\mathrm{k}}\)

Also, the direction ratios of the given line are 3, 7, and 2.

This means that the line is in the direction of the vector, \(\overrightarrow{\mathrm{b}}=3 \hat{\mathrm{i}}+7 \hat{\mathrm{j}}+2 \hat{\mathrm{k}}\)

It is known that the line through position vector \(\vec{a}\) and in the direction of the vector \(\vec{b}\) is given by the equation, \(\overrightarrow{\mathrm{r}}=\overrightarrow{\mathrm{a}}+\lambda \overrightarrow{\mathrm{b}}, \lambda \in \mathrm{R} \Rightarrow \overrightarrow{\mathrm{r}}=(5 \hat{\mathrm{i}}-4 \hat{\mathrm{j}}+6 \hat{\mathrm{k}})+\lambda(3 \hat{\mathrm{i}}+7 \hat{\mathrm{j}}+2 \hat{\mathrm{k}})\)

This is the required equation of the given line in vector form.

Question 8. Find the angle between the following pairs of lines:

1. \(\overrightarrow{\mathrm{r}}=2 \hat{\mathrm{i}}-5 \hat{\mathrm{j}}+\hat{\mathrm{k}}+\lambda(3 \hat{\mathrm{i}}+2 \hat{\mathrm{j}}+6 \hat{\mathrm{k}})\) and \({\overrightarrow{\mathrm{r}}}=7 \hat{\mathrm{i}}-6 \hat{\mathrm{k}}+\mu(\hat{\mathrm{i}}+2 \hat{\mathrm{j}}+2 \hat{\mathrm{k}})\)
2. \(\overrightarrow{\mathrm{r}}=3 \hat{\mathrm{i}}+\hat{\mathrm{j}}-2 \hat{\mathrm{k}}+\lambda(\hat{\mathrm{i}}-\hat{\mathrm{j}}-2 \hat{\mathrm{k}})\) and \(\overrightarrow{\mathrm{r}}=2 \hat{\mathrm{i}}-\hat{\mathrm{j}}-56 \hat{\mathrm{k}}+\mu(3 \hat{\mathrm{i}}-5 \hat{\mathrm{j}}-4 \hat{\mathrm{k}})\)

Solution:

1. Let θ be the angle between the given lines.

The angle between the given pairs of lines is given by, \(\cos \theta=\left|\frac{\vec{b}_1 \cdot \vec{b}_2}{\left|\vec{b}_1\right|\left|\vec{b}_2\right|}\right|\)

The given lines are parallel to the vectors, \(\vec{b}_1=3 \hat{i}+2 \hat{j}+6 \hat{k}\) and \(\vec{b}_2=\hat{i}+2 \hat{j}+2 \hat{k}\) respectively.

∴ \(\left|\vec{b}_1\right|=\sqrt{3^2+2^2+6^2}=7\)

∴ \(\left|\vec{b}_2\right|=\sqrt{(1)^2+(2)^2+(2)^2}=3\)

⇒ \(\vec{b}_1 \cdot \vec{b}_2=(3 \hat{i}+2 \hat{j}+6 \hat{k})(\hat{i}+2 \hat{j}+2 \hat{k})=3 \times 1+2 \times 2+6 \times 2=3+4+12=19\)

⇒ \(\cos \theta=\frac{19}{7 \times 3} \Rightarrow \theta=\cos ^{-1}\left(\frac{19}{21}\right)\)

2. The given lines are parallel to the vectors, \(\vec{b}_1=\hat{i}-\hat{j}-2 \hat{k}\) and \(\vec{b}_2=3 \hat{i}-5 \hat{j}-4 \hat{k}\) respectively

∴ \(\left|\vec{b}_1\right|=\sqrt{(1)^2+(-1)^2+(-2)^2}=\sqrt{6}\)

∴ \(\left|\vec{b}_2\right|=\sqrt{(3)^2+(-5)^2+(-4)^2}=\sqrt{50}=5 \sqrt{2}\)

∴ \(\vec{b}_1 \vec{b}_2=(\hat{i}-\hat{j}-2 \hat{k})(3 \hat{i}-5 \hat{j}-4 \hat{k})=1.3-1(-5)-2(-4)=3+5+8=16\)

⇒ \(\cos \theta=\left|\frac{\vec{b}_1, \vec{b}_2}{\left|\vec{b}_1\right|\left|\vec{b}_2\right|}\right|\)

⇒ \(\cos \theta=\frac{16}{\sqrt{6} \cdot 5 \sqrt{2}}=\frac{16}{\sqrt{2} \cdot \sqrt{3} \cdot 5 \sqrt{2}}=\frac{16}{10 \sqrt{3}} \Rightarrow \cos \theta=\frac{8}{5 \sqrt{3}} \Rightarrow \theta=\cos ^{-1}\left(\frac{8}{5 \sqrt{3}}\right)\)

Question 9. Find the angle between the following pairs of lines :

1. \(\frac{x-2}{2}=\frac{y-1}{5}=\frac{z+3}{-3}\) and \(\frac{x+2}{-1}=\frac{y-4}{8}=\frac{z-5}{4}\)
2. \(\frac{x}{2}=\frac{y}{2}=\frac{z}{1}\) and \(\frac{x-5}{4}=\frac{y-2}{1}=\frac{z-3}{8}\)

Solution:

1. Let \(\vec{b}_1\) and \(\vec{b}_2\) be the vectors parallel to the pair of lines, \(\frac{x-2}{2}=\frac{y-1}{5}=\frac{z+3}{-3}\) and \(\frac{x+2}{-1}=\frac{y-4}{8}=\frac{z-5}{4}\), respectively

⇒ \(\vec{b}_1=2 \hat{i}+5 \hat{j}-3 \hat{k}\) and \(\vec{b}_2=-\hat{i}+8 \hat{j}+4 \hat{k}\)

∴ \(\left|\vec{b}_1\right|=\sqrt{(2)^2+(5)^2+(-3)^2}=\sqrt{38}\)

∴ \(\left|\vec{b}_2\right|=\sqrt{(-1)^2+(8)^2+(4)^2}=\sqrt{81}=9\)

∴ \(\vec{b}_1 \vec{b}_2=(2 \hat{i}+5 \hat{j}-3 \hat{k}) \cdot(-\hat{i}+8 \hat{j}+4 \hat{k})=2(-1)+5 \times 8+(-3) \cdot 4=-2+40-12=26\)

The angle \(\theta\) between the given pair of lines is given by the relation,

⇒ \(\cos \theta=\left|\frac{\vec{b}_1, \vec{b}_2}{\left|\overrightarrow{\mathrm{b}}_1\right|\left|\overrightarrow{\mathrm{b}}_2\right|}\right| \Rightarrow \cos \theta=\frac{26}{9 \sqrt{38}} \Rightarrow \theta=\cos ^{-1}\left(\frac{26}{9 \sqrt{38}}\right)\)

2. Let \(\vec{b}_1\) and \(\vec{b}_2\) be the vectors parallel to the given pair of lines, \(\frac{x}{2}=\frac{y}{2}=\frac{z}{1}\) and \(\frac{x-5}{4}=\frac{y-2}{1}=\frac{z-3}{8}\), respectively.

⇒ \(\vec{b}_1=2 \hat{i}+2 \hat{j}+\hat{k} \text { and } \vec{b}_2=4 \hat{i}+\hat{j}+8 \hat{k}\)

⇒ \(\left|\vec{b}_1\right|=\sqrt{(2)^2+(2)^2+(1)^2}=\sqrt{9}=3\)

⇒ \(\left|\vec{b}_2\right|=\sqrt{4^2+1^2+8^2}=\sqrt{81}=9\)

⇒ \(\vec{b}_1, \vec{b}_2=(2 \hat{i}+2 \hat{j}+\hat{k}) \cdot(4 \hat{i}+\hat{j}+8 \hat{k})=2 \times 4+2 \times 1+1 \times 8=8+2+8=18\)

If \(\theta\) is the angle between the given pair of lines, then

∴ \(\cos \theta=\left|\frac{\vec{b}_1 \cdot \vec{b}_2}{\left|\vec{b}_1\right| \mid \vec{b}_2}\right| \Rightarrow \cos \theta=\frac{18}{3 \times 9}=\frac{2}{3} \Rightarrow \theta=\cos ^{-1}\left(\frac{2}{3}\right)\)

Question 10. Find the values of p so that the lines \(\frac{1-x}{3}=\frac{7 y-14}{2 p}=\frac{z-3}{2} \text { and } \frac{7-7 x}{3 p}=\frac{y-5}{1}=\frac{6-z}{5}\) are at right angles.
Solution:

The given equations can be written in the standard form as \(\frac{x-1}{-3}=\frac{y-2}{\frac{2 p}{7}}=\frac{z-3}{2} \text { and } \frac{x-1}{\frac{-3 p}{7}}=\frac{y-5}{1}=\frac{z-6}{-5}\)

The direction ratios of the lines are – 3, 2p/7, 2, and -3p/7,1,-5 respectively.

Two lines with direction ratios, a₁, b₁, c₁ and a₂, b₂, c₂ are perpendicular to each other, if a₁a₂ + b₁b₂ + c₁c₂ = 0

∴ (-3) \(\cdot\left(\frac{-3 p}{7}\right)+\left(\frac{2 p}{7}\right) \cdot(1)+2(-5)=0\)

⇒ \(\frac{9 p}{7}+\frac{2 p}{7}=10 \quad \Rightarrow 11 p=70 \quad \Rightarrow p=\frac{70}{11}\)

Thus, the value of p is \(\frac{70}{11}\)

Question 11. Show that the lines \(\frac{x-5}{7}=\frac{y+2}{-5}=\frac{z}{1} \text { and } \frac{x}{1}=\frac{y}{2}=\frac{z}{3}\) are perpendicular to each other.
Solution:

The equations of the given lines are \(\frac{x-5}{7}=\frac{y+2}{-5}=\frac{z}{1} \text { and } \frac{x}{1}=\frac{y}{2}=\frac{z}{3}\)

The direction ratios of the given lines are 7, -5, 1, and 1, 2, and 3 respectively.

Two lines with direction ratios, a₁, b₁, c₁ and a₂, b₂, c₂ are perpendicular to each other, if a₁a₂+ b₁b₂+ c₁c₂= 0

∴ 7 x 1 + (-5) x 2+1 x 3 = 7- 10 + 3 = 0

Therefore, the given lines are perpendicular to each other.

Question 12. Find the shortest distance between the lines: \(\overrightarrow{\mathrm{r}}=(\hat{\mathrm{i}}+2 \hat{\mathrm{j}}+\hat{\mathrm{k}})+\lambda(\hat{\mathrm{i}}-\hat{\mathrm{j}}+\hat{\mathrm{k}}) \text { and } \overrightarrow{\mathrm{r}}=(2 \hat{\mathrm{i}}-\hat{\mathrm{j}}-\hat{\mathrm{k}})+\mu(2 \hat{\mathrm{i}}+\hat{\mathrm{j}}+2 \hat{\mathrm{k}})\)
Solution:

The equations of the given lines are \(\overrightarrow{\mathrm{r}}=(\hat{\mathrm{i}}+2 \hat{\mathrm{j}}+\hat{\mathrm{k}})+\lambda(\hat{\mathrm{i}}-\hat{\mathrm{j}}+\hat{\mathrm{k}}) \text { and } \overrightarrow{\mathrm{r}}=(2 \hat{\mathrm{i}}-\hat{\mathrm{j}}-\hat{\mathrm{k}})+\mu(2 \hat{\mathrm{i}}+\hat{\mathrm{j}}+2 \hat{\mathrm{k}})\)

It is known that the shortest distance between the lines, \(\vec{r}=\vec{a}_1+\lambda \vec{b}_1\) and \(\vec{r}=\vec{a}_2+\mu \vec{b}_2\) is given by

d = \(\left|\frac{\left(\vec{b}_1 \times \vec{b}_2\right) \cdot\left(\vec{a}_2-\vec{a}_1\right)}{\left|\vec{b}_1 \times \vec{b}_2\right|}\right|\)……(1)

Comparing the given equations, we obtain

∴ \(\vec{a}_1=\hat{i}+2 \hat{j}+\hat{k}, \vec{b}_1=\hat{i}-\hat{j}+\hat{k}, \vec{a}_2=2 \hat{i}-\hat{j}-\hat{k}, \vec{b}_2=2 \hat{i}+\hat{j}+2 \hat{k}\)

⇒ \(\vec{a}_2-\vec{a}_1=(2 \hat{i}-\hat{j}-\hat{k})-(\hat{i}+2 \hat{j}+\hat{k})=\hat{i}-3 \hat{j}-2 \hat{k}\)

⇒ \(\overrightarrow{\mathrm{b}}_1 \times \overrightarrow{\mathrm{b}}_2\)

= \(\left|\begin{array}{ccc}
\hat{\mathrm{i}} & \hat{\mathrm{j}} & \hat{\mathrm{k}} \\
1 & -1 & 1 \\
2 & 1 & 2
\end{array}\right|\)

⇒ \(\overrightarrow{\mathrm{b}}_1 \times \overrightarrow{\mathrm{b}}_2=(-2-1) \hat{\mathrm{i}}-(2-2) \hat{\mathrm{j}}+(1+2) \hat{\mathrm{k}}=-3 \hat{\mathrm{i}}+3 \hat{\mathrm{k}}\)

⇒ \(\left|\overrightarrow{\mathrm{b}}_1 \times \overrightarrow{\mathrm{b}}_2\right|=\sqrt{(-3)^2+(3)^2}=\sqrt{9+9}=\sqrt{18}=3 \sqrt{2}\)

Substituting all the values in equation (1), we obtain

d = \(\left|\frac{(-3 \hat{i}+3 \hat{k}) \cdot(\hat{i}-3 \hat{j}-2 \hat{k})}{3 \sqrt{2}}\right| \Rightarrow d=\left|\frac{-3 \cdot 1+3(-2)}{3 \sqrt{2}}\right| \Rightarrow d=\left|\frac{-9}{3 \sqrt{2}}\right|\)

⇒ d = \(\frac{3}{\sqrt{2}}=\frac{3 \times \sqrt{2}}{\sqrt{2} \times \sqrt{2}}=\frac{3 \sqrt{2}}{2}\)

Therefore, the shortest distance between the two lines is \(\frac{3 \sqrt{2}_2^{-}}{2}\) units.

Question 13. Find the shortest distance between the lines \(\frac{x+1}{7}=\frac{y+1}{-6}=\frac{z+1}{1}\) and \(\frac{x-3}{1}=\frac{y-5}{-2}=\frac{z-7}{1}\)
Solution:

The given lines are \(\frac{x+1}{7}=\frac{y+1}{-6}=\frac{z+1}{1}\) and \(\frac{x-3}{1}=\frac{y-5}{-2}=\frac{z-7}{1}\)

It is known that the shortest distance between the two lines,
\(\frac{x-x_1}{a_1}=\frac{y-y_1}{b_1}=\frac{z-z_1}{c_1}\) and \(\frac{x-x_2}{a_2}=\frac{y-y_2}{b_2}=\frac{z-z_2}{c_2}\) is given as :

d = \(\frac{\left|\begin{array}{ccc}
x_2-x_1 & y_2-y_1 & z_2-z_1 \\
a_1 & b_1 & c_1 \\
a_2 & b_2 & c_2
\end{array}\right|}{\sqrt{\left(b_1 c_2-b_2 c_1\right)^2+\left(c_1 a_2-c_2 a_1\right)^2+\left(a_1 b_2-a_2 b_1\right)^2}}\)…..(1)

Comparing the given equations, we obtain

⇒ \(x_1=-1, y_1=-1, z_1=-1 ; x_2=3, y_2=5, z_2=7\)

⇒ \(a_1=7, b_1=-6, c_1=1 ; a_2=1, b_2=-2, c_2=1\)

Then, \(\left|\begin{array}{ccc}x_2-x_1 & y_2-y_1 & z_2-z_1 \\ a_1 & b_1 & c_1 \\ a_2 & b_2 & c_2\end{array}\right|=\left|\begin{array}{ccc}4 & 6 & 8 \\ 7 & -6 & 1 \\ 1 & -2 & 1\end{array}\right|\)

= \(4(-6+2)-6(7-1)+8(-14+6)=-16 -36-64=-116\)

⇒ \(\sqrt{\left(b_1 c_2-b_2 c_1\right)^2+\left(c_1 a_2-c_2 a_1\right)^2+\left(a_1 b_2-a_2 b_1\right)^2}=\sqrt{(-6+2)^2+(1-7)^2+(-14+6)^2}\)

= \(\sqrt{16+36+64}=\sqrt{116}=2 \sqrt{29}\)

Substituting all the values in equation (1), we obtain

d = \(\left|\frac{-116}{\sqrt{116}}\right|=\frac{116}{\sqrt{116}}=2 \sqrt{29}\)

Since distance is always non-negative, the distance between the given lines is \(2 \sqrt{29}\) units.

Question 14. Find the shortest distance between the lines whose vector equations are: \(\vec{r}=\hat{i}+2 \hat{j}+3 \hat{k}+\lambda(\hat{i}-3 \hat{j}+2 \hat{k})\) and \(\vec{r}=4 \hat{i}+5 \hat{j}+6 \hat{k}+\mu(2 \hat{i}+3 \hat{j}+\hat{k})\)
Solution:

The given lines are \(\vec{r}=\hat{i}+2 \hat{j}+3 \hat{k}+\lambda(\hat{i}-3 \hat{j}+2 \hat{k})\) and \(\vec{r}=4 \hat{i}+5 \hat{j}+6 \hat{k}+\mu(2 \hat{i}+3 \hat{j}+\hat{k})\)

It is known that the shortest distance between the lines, \(\vec{r}=\vec{a}_1+\lambda \vec{b}_1\) and \(\vec{r}=\vec{a}_2+\mu \vec{b}_2\) is given by,

d = \(\left|\frac{\left.\overrightarrow{\mathrm{b}}_1 \times \overrightarrow{\mathrm{b}}_2\right) \cdot\left(\overrightarrow{\mathrm{a}}_2-\overrightarrow{\mathrm{a}}_1\right)}{\left|\overrightarrow{\mathrm{b}}_1 \times \overrightarrow{\mathrm{b}}_2\right|}\right|\)…….(1)

Comparing the given equations with \(\overrightarrow{\mathrm{r}}=\overrightarrow{\mathrm{a}}_1+\lambda \overrightarrow{\mathrm{b}}_1\) and \(\overrightarrow{\mathrm{r}}=\overrightarrow{\mathrm{a}}_2+\mu \overrightarrow{\mathrm{b}}_2\); we have:

⇒ \(\vec{a}_1=\hat{i}+2 \hat{j}+3 \hat{k} ; \vec{a}_2=4 \hat{i}+5 \hat{j}+6 \hat{k}\)

⇒ \(\vec{b}_1=\hat{i}-3 \hat{j}+2 \hat{k} ; \vec{b}_2=2 \hat{i}+3 \hat{j}+\hat{k}\)

⇒ \(\vec{a}_2-\vec{a}_1=(4 \hat{i}+5 \hat{j}+6 \hat{k})-(\hat{i}+2 \hat{j}+3 \hat{k})=3 \hat{i}+3 \hat{j}+3 \hat{k}\)

⇒ \(\vec{b}_1 \times \vec{b}_2\)

= \(\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
1 & -3 & 2 \\
2 & 3 & 1
\end{array}\right|\)

= \((-3-6 \hat{i}-(1-4) \hat{j}+(3+6) \hat{k}=-9 \hat{i}+3 \hat{j}+9 \hat{k}\)

⇒ \(\left|\vec{b}_1 \times \vec{b}_2\right|=\sqrt{(-9)^2+(3)^2+(9)^2}=\sqrt{81+9+81}=\sqrt{171}=3 \sqrt{19}\)

⇒ \(\left(\vec{b}_1 \times \vec{b}_2\right) \cdot\left(\vec{a}_2-\vec{a}_1\right)=(-9 \hat{i}+3 \hat{j}+9 \hat{k}) \cdot(3 \hat{i}+3 \hat{j}+3 \hat{k})=-9 \times 3+3 \times 3+9 \times 3=9\)

Substituting all the values in equation (1), we obtain

d = \(\left|\frac{9}{3 \sqrt{19}}\right|=\frac{3}{\sqrt{19}}\)

Therefore, the shortest distance between the two given lines is \(\frac{3}{\sqrt{19}}\) units.

Question 15. Find the shortest distance between the lines whose vector equations are \(\vec{r}=(1-t) \hat{i}+(t-2) \hat{j}+(3-2 t) \hat{k}\) and \(\vec{r}=(s+1) \hat{i}+(2 s-1) \hat{j}-(2 s+1) \hat{k}\)
Solution:

The given lines are

⇒ \(\vec{r}=(1-t) \hat{i}+(t-2) \hat{j}+(3-2 t) \hat{k}\)

⇒ \(\vec{r}=(\hat{i}-2 \hat{j}+3 \hat{k})+t(-\hat{i}+\hat{j}-2 \hat{k})\)….(1)

⇒ \(\vec{r}=(s+1) \hat{i}+(2 s-1) \hat{j}-(2 s+1) \hat{k}\)

⇒ \(\vec{r}=(\hat{i}-\hat{j}-\hat{k})+s(\hat{i}+2 \hat{j}-2 \hat{k})\)…..(2)

It is known that the shortest distance between the lines, \(\vec{r}=\vec{a}_1+\lambda \vec{b}_1\) and \(\vec{r}=\vec{a}_2+\mu \vec{b}_2\) is given by

d = \(\left|\frac{\left(\vec{b}_1 \times \vec{b}_2\right) \cdot\left(\vec{a}_2-\vec{a}_1\right)}{\left|\vec{b}_1 \times \vec{b}_2\right|}\right|\)……..(3)

For the given equations,

⇒ \(\vec{a}_1=\hat{i}-2 \hat{j}+3 \hat{k} ; \vec{a}_2=\hat{i}-\hat{j}-\hat{k}\)

⇒ \(\vec{b}_1=-\hat{i}+\hat{j}-2 \hat{k} ; \vec{b}_2=\hat{i}+2 \hat{j}-2 \hat{k}\)

⇒ \(\vec{a}_2-\vec{a}_1=(\hat{i}-\hat{j}-\hat{k})-(\hat{i}-2 \hat{j}+3 \hat{k})=\hat{j}-4 \hat{k}\)

⇒ \(\vec{b}_1 \times \vec{b}_2\)

= \(\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
-1 & 1 & -2 \\
1 & 2 & -2
\end{array}\right|\)

= \((-2+4) \hat{i}-(2+2) \hat{j}+(-2-1) \hat{k}=2 \hat{i}-4 \hat{j}-3 \hat{k}\)

⇒ \(\left|\vec{b}_1 \times \vec{b}_2\right|=\sqrt{(2)^2+(-4)^2+(-3)^2}=\sqrt{4+16+9}=\sqrt{29}\)

∴ \(\left(\vec{b}_1 \times \vec{b}_2\right) \cdot\left(\vec{a}_2-\vec{a}_1\right)=(2 \hat{i}-4 \hat{j}-3 \hat{k}) \cdot(\hat{j}-4 \hat{k})=-4+12=8\)

Substituting all the values in equation (3), we, obtain \(\mathrm{d}=\left|\frac{8}{\sqrt{29}}\right|=\frac{8}{\sqrt{29}}\)

Therefore, the shortest distance between the lines is \(\frac{8}{\sqrt{29}}\) units.

Three-Dimensional Geometry Miscellaneous Exercise

Question 1. Find the angle between the lines whose direction ratios are (a, b, c) and (b – c, c – a, a -b).
Solution:

The angle θ between the lines with direction cosines, (a, b, c) and (b – c, c – a, a -b), is given by,

cos \(\theta=\left|\frac{a_1 a_2+b_1 b_2+c_1 c_2}{\sqrt{a_1^2+b_1^2+c_1^2}+\sqrt{a_2^2+b_2^2+c_2^2}}\right| \Rightarrow \cos \theta=\left|\frac{a(b-c)+b(c-a)+c(a-b)}{\sqrt{a^2+b^2+c^2}+\sqrt{(b-c)^2+(c-a)^2+(a-b)^2}}\right|\)

⇒cos \(\theta=0 \Rightarrow \theta=\cos ^{-1}(0) \Rightarrow \theta=90^{\circ}\)

Thus, the angle between the lines is 90°.

Question 2. Find the equation of a line parallel to the x-axis and passing through the origin.
Solution:

The line parallel to the x-axis and passing through the origin is the x-axis itself.

Let A be a point on the x-axis. Therefore, the coordinates of A are given by (a, 0, 0), where a ∈ R.

The direction ratios of OA are (a – 0), (0 – 0), (0 – 0) i.e. a, 0, 0

The equation of OA is given by \(\frac{x-0}{a}=\frac{y-0}{0}=\frac{z-0}{0} \Rightarrow \frac{x}{1}=\frac{y}{0}=\frac{z}{0}=a\)

Thus, the equation of the line parallel to the x-axis and passing through the origin is \(\frac{x}{1}=\frac{y}{0}=\frac{z}{0}\)

Question 3. If the lines \(\frac{x-1}{-3}=\frac{y-2}{2 k}=\frac{z-3}{2}\) and \(\frac{x-1}{3 k}=\frac{y-1}{1}=\frac{z-6}{-5}\) are perpendicular, find the value of k.
Solution:

The direction ratios of the lines, \(\frac{x-1}{-3}=\frac{y-2}{2 k}=\frac{z-3}{2}\) and \(\frac{x-1}{3 k}=\frac{y-1}{1}=\frac{z-6}{-5}\), are -3,2 k, 2 and 3 k, 1,-5 respectively.

It is known that two lines with direction ratios, \(\mathrm{a}_1, \mathrm{~b}_1, \mathrm{c}_1\) and \(\mathrm{a}_2, \mathrm{~b}_2, \mathrm{c}_2\), are perpendicular if \(a_1 a_2+b_1 b_2+c_1 c_2=0\)

∴ -3(3k) + 2kx 1 + 2(-5) = 0 => ~9k + 2k-10 = 0 => 7k=-10 => k = -10/7

Question 4. Find the shortest distance between lines \(\overrightarrow{\mathrm{r}}=6 \hat{i}+2 \hat{j}+2 \hat{k}+\lambda(\hat{i}-2 \hat{j}+2 \hat{k})\) and \(\overrightarrow{\mathrm{r}}=-4 \hat{\mathrm{i}}-\hat{\mathrm{k}}+\mu(3 \hat{\mathrm{i}}-2 \hat{\mathrm{j}}-2 \hat{\mathrm{k}})\)
Solution:

The given lines are \(\vec{r}=6 \hat{i}+2 \hat{j}+2 \hat{k}+\lambda(\hat{i}-2 \hat{j}+2 \hat{k})\)…..(1)

⇒ \(\vec{r}=-4 \hat{i}-\hat{k}+\mu(3 \hat{i}-2 \hat{j}-2 \hat{k})\)….(2)

It is known that the shortest distance between two lines, \(\vec{r}=\vec{a}_1+\lambda \vec{b}_1\), and \(\vec{r}=\vec{a}_2+\mu \vec{b}_2\) is given by

d = \(\left|\frac{\left(\overrightarrow{\mathrm{b}}_1 \times \overrightarrow{\mathrm{b}}_2\right) \cdot\left(\overrightarrow{\mathrm{a}}_2-\overrightarrow{\mathrm{a}}_1\right)}{\left|\overrightarrow{\mathrm{b}}_1 \times \overrightarrow{\mathrm{b}}_2\right|}\right|\)……(3)

Comparing \(\overrightarrow{\mathrm{r}}=\overrightarrow{\mathrm{a}}_1+\lambda \overrightarrow{\mathrm{b}}_1\) and \(\overrightarrow{\mathrm{r}}=\overrightarrow{\mathrm{a}}_2+\mu \overrightarrow{\mathrm{b}}_2\) to equations (1) and (2), we obtain

⇒ \(\vec{a}_1=6 \hat{i}+2 \hat{j}+2 \hat{k}, \vec{b}_1=\hat{i}-2 \hat{j}+2 \hat{k}, \vec{a}_2=-4 \hat{i}-\hat{k}, \vec{b}_2=3 \hat{i}-2 \hat{j}-2 \hat{k}\)

⇒ \(\vec{a}_2-\vec{a}_1=(-4 \hat{i}-\hat{k})-(6 \hat{i}+2 \hat{j}+2 \hat{k})=-10 \hat{i}-2 \hat{j}-3 \hat{k}\)

⇒ \(\vec{b}_1 \times \vec{b}_2=\left|\begin{array}{ccc}
i & \hat{j} & \hat{k} \\
1 & -2 & 2 \\
3 & -2 & -2
\end{array}\right|\)

= \((4+4) \hat{i}-(-2-6) \hat{j}+(-2+6) \hat{k}=8 \hat{i}+8 \hat{j}+4 \hat{k}\)

⇒ \(\left|\vec{b}_1 \times \vec{b}_2\right|=\sqrt{(8)^2+(8)^2+(4)^2}=12\)

⇒ \(\left(\vec{b}_1 \times \vec{b}_2\right) \cdot\left(\vec{a}_2-\vec{a}_1\right)=(8 \hat{i}+8 \hat{j}+4 \hat{k}) \cdot(-10 \hat{i}-2 \hat{j}-3 \hat{k})=-80-16-12=-108\)

Substituting all the values in equation (3), we obtain \(\mathrm{d}=\left|\frac{-108}{12}\right|=9\) units

Therefore, the shortest distance between the two given lines is 9 units.

Question 5. Find the vector equation of the line passing through the point (1, 2, -4) and perpendicular to the two lines: \(\frac{x-8}{3}=\frac{y+19}{-16}=\frac{z-10}{7}\) and \(\frac{x-15}{3}=\frac{y-29}{8}=\frac{z-5}{-5}\)
Solution:

Let the required line be parallel to the vector \(\vec{b}\) given by, \(\vec{b}=b_1 \hat{i}+b_2 \hat{j}+b_3 \hat{k}\)

The position vector of the point (1,2,-4) is \(\vec{a}=\hat{i}+2 \hat{j}-4 \hat{k}\)

The equation of the line passing through (1,2,-4) and parallel to vector \(\vec{b}\) is \(\overrightarrow{\mathrm{r}}=\overrightarrow{\mathrm{a}}+\lambda \overrightarrow{\mathrm{b}} \Rightarrow \overrightarrow{\mathrm{r}}=(\hat{\mathrm{i}}+2 \hat{\mathrm{j}}-4 \hat{\mathrm{k}})+\lambda\left(\mathrm{b}_1 \hat{\mathrm{i}}+\mathrm{b}_2 \hat{\mathrm{j}}+\mathrm{b}_3 \hat{\mathrm{k}}\right)\)……..(1)

The equations of the lines are \(\frac{x-8}{3}=\frac{y+19}{-16}=\frac{z-10}{7}\)…..(2)

and \(\frac{x-15}{3}=\frac{y-29}{8}=\frac{z-5}{-5}\)…..(3)

Lines (1) and line (2) are perpendicular to each other \(3 b_1-16 b_2+7 b_3=0\)…..(4)

Also, lines (1) and line (3) are perpendicular to each other. \(3 b_1+8 b_2-5 b_3=0\)…..(5)

From equations (4) and (5), we obtain

\(\frac{b_1}{(-16)(-5)-8 \times 7}=\frac{b_2}{7 \times 3-3(-5)}=\frac{b_3}{3 \times 8-3(-16)} \Rightarrow \frac{b_1}{24}=\frac{b_2}{36}=\frac{b_3}{72} \Rightarrow \frac{b_1}{2}=\frac{b_2}{3}=\frac{b_3}{6}\)

∴ Direction ratios of \(\overrightarrow{\mathrm{b}}\) are 2,3, and 6.

∴ \(\vec{b}=2 \hat{i}+3 \hat{j}+6 \hat{k}\)

Substituting \(\vec{b}=2 \hat{i}+3 \hat{j}+6 \hat{k}\) in equation (1), we obtain \(\vec{r}=(\hat{i}+2 \hat{j}-4 \hat{k})+\lambda(2 \hat{i}+3 \hat{j}+6 \hat{k})\)

This is the equation of the required line.

 

Vector Algebra Exercise 10.1

Question 1. Represent graphically a displacement of 40 km, 30° east of north.
Solution:

Here, \(\overrightarrow{\mathrm{OP}}\) vector represents the displacement of 40 km, 30° East of North.

Question 2. Classify the following measures as scalars and vectors.

1. 10kg
2. 2 metres north-west
3. 40°
4. 40 watt
5. 10^-19
6.  coulomb 20 m/s²

Solution:

1. 10 kg is a scalar quantity because it involves only magnitude.
2. 2 meters northwest is a vector quantity as it involves both magnitude and direction.
3. 40° is a scalar quantity as it involves only magnitude.
4. 40 watts is a scalar quantity as it involves only magnitude.
5. 10^-19 coulomb is a scalar quantity as it involves only magnitude.
6. 20 m/s² is a vector quantity as it involves magnitude as well as direction.

Question 3. Classify the following as scalar and vector quantities.

1. Time period
2. Distance
3. Force
4. Velocity
5. Work done

Solution:

1. Time period is a scalar quantity as it involves only magnitude.
2. Distance is a scalar quantity as it involves only magnitude.
3. Force is a vector quantity as it involves both magnitude and direction.
4. Velocity is a vector quantity as it involves both magnitude as well as direction.
5. Work done is a scalar quantity as it involves only magnitude.

Question 4. Identify the following vectors,

1. Coinitial
2. Equal
3. Collinear but not equal

Solution:

1. Vectors \(\vec{a}\) and \(\vec{d}\) are coinitial because they have the same initial point.
2. Vectors \(\vec{b}\) and \(\vec{d}\) are equal because they have the same magnitude and direction.
3. Vectors \(\vec{a}\) and \(\vec{c}\) are collinear but not equal. This is because although they are parallel, their directions are not the same.

Read and Learn More Class 12 Maths Chapter Wise with Solutions

Question 5. Answer the following as true or false.

1. \(\vec{\mathrm{a}}\) and –\(\vec{\mathrm{a}}\) are collinear.
2. Two collinear vectors are always equal in magnitude.
3. Two vectors having the same magnitude are collinear.
4. Two collinear vectors having the same magnitude are equal.

Solution:

1. True, Vectors \(\vec{\mathrm{a}}\) and –\(\vec{\mathrm{a}}\) are parallel to the same line.
2. False, Collinear vectors are those vectors that are parallel to the same line.
3. False, Two vectors having the same magnitude need not necessarily be parallel to the same line,
4. False, Only if the magnitude and direction of two vectors are the same regardless of the positions of their initial points, the two vectors are said to be equal.

Vector Algebra Exercise 10.2

Question 1. Compute the magnitude of the following vectors: \(\vec{a}=\hat{i}+\hat{j}+\hat{k} ; \vec{b}=2 \hat{i}-7 \hat{j}-3 \hat{k} ; \vec{c}=\frac{1}{\sqrt{3}} \hat{i}+\frac{1}{\sqrt{3}} \hat{j}-\frac{1}{\sqrt{3}} \hat{k}\)
Solution:

The given vectors are: \(\vec{a}=\hat{i}+\hat{j}+\hat{k} ; \vec{b}=2 \hat{i}-7 \hat{j}-3 \hat{k} ; \vec{c}=\frac{1}{\sqrt{3}} \hat{i}+\frac{1}{\sqrt{3}} \hat{j}-\frac{1}{\sqrt{3}} \hat{k}\)

⇒ \(|\vec{a}|=\sqrt{(1)^2+(1)^2+(1)^2}=\sqrt{3}\)

⇒ \(|\vec{b}|=\sqrt{(2)^2+(-7)^2+(-3)^2}=\sqrt{4+49+9}=\sqrt{62}\)

⇒ \(|\vec{c}|=\sqrt{\left(\frac{1}{\sqrt{3}}\right)^2+\left(\frac{1}{\sqrt{3}}\right)^2+\left(-\frac{1}{\sqrt{3}}\right)^2}=\sqrt{\frac{1}{3}+\frac{1}{3}+\frac{1}{3}}=1\)

Question 2. Write two different vectors having the same magnitude.
Solution:

Consider \(\overrightarrow{\mathrm{a}}=(\hat{\mathrm{i}}-2 \hat{\mathrm{j}}+3 \hat{\mathrm{k}})\) and \(\overrightarrow{\mathrm{b}}=(2 \hat{\mathrm{i}}+\hat{\mathrm{j}}-3 \hat{\mathrm{k}})\).

It can be observed that \(|\overrightarrow{\mathrm{a}}|=\sqrt{1^2+(-2)^2+3^2}=\sqrt{1+4+9}=\sqrt{14}\)

and \(|\vec{b}|=\sqrt{2^2+1^2+(-3)^2}=\sqrt{4+1+9}=\sqrt{14}\)

Hence, \(\vec{a}\) and \(\vec{b}\) are two different vectors having the same magnitude. The vectors are different because they have different directions.

Question 3. Write two different vectors having the same direction.
Solution:

Consider \(\vec{a}=(\hat{i}+\hat{j}+\hat{k})\) and \(\vec{b}=(2 \hat{i}+2 \hat{j}+2 \hat{k})\).

The direction consines of \(\vec{a}\) are given by,

l = \(\frac{1}{\sqrt{1^2+1^2+1^2}}=\frac{1}{\sqrt{3}}, \mathrm{~m}=\frac{1}{\sqrt{1^2+1^2+1^2}}=\frac{1}{\sqrt{3}}, \mathrm{n}=\frac{1}{\sqrt{1^2+1^2+1^2}}=\frac{1}{\sqrt{3}}\).

The direction cosines of \(\vec{b}\) are given by

l = \(\frac{2}{\sqrt{2^2+2^2+2^2}}=\frac{2}{2 \sqrt{3}}=\frac{1}{\sqrt{3}}, \mathrm{~m}=\frac{2}{\sqrt{2^2+2^2+2^2}}=\frac{2}{2 \sqrt{3}}=\frac{1}{\sqrt{3}}, \mathrm{n}=\frac{2}{\sqrt{2^2+2^2+2^2}}=\frac{2}{2 \sqrt{3}}=\frac{1}{\sqrt{3}}\)

The direction cosines of \(\vec{a}\) and \(\vec{b}\) are the same. Hence, the two vectors have the same direction.

Question 4. Find the values of x and y so that the vectors \(2 \hat{\mathrm{i}}+3 \hat{\mathrm{j}} \text { and } x \hat{\mathrm{i}}+y \hat{\mathrm{j}}\) are equal.
Solution:

The two vectors \(2 \hat{\mathrm{i}}+3 \hat{\mathrm{j}} \text { and } x \hat{\mathrm{i}}+y \hat{\mathrm{j}}\) will be equal if their corresponding scalar components are equal. Hence, the required values of x and y are 2 and 3 respectively.

Question 5. Find the scalar and vector components of the vector with initial point (2,1) and terminal point (-5, 7).
Solution:

The vector with the initial point P (2, 1) and terminal point Q (-5, 7) can be given by, \(\overrightarrow{\mathrm{PQ}}=(-5-2) \hat{\mathrm{i}}+(7-1) \hat{\mathrm{j}} \Rightarrow \overrightarrow{\mathrm{PQ}}=-7 \hat{\mathrm{i}}+6 \hat{\mathrm{j}}\)

Hence, the required scalar components are -7 and 6 while the vector components are \(-7 \hat{\mathrm{i}}+6 \hat{\mathrm{j}}\)

Question 6. Find the sum of the vectors \(\vec{a}=\hat{i}-2 \hat{j}+\hat{k}, \hat{b}=-2 \hat{i}+4 \hat{j}+5 \hat{k}\) and \(\vec{c}=\hat{i}-6 \hat{j}-7 \hat{k}\).
Solution:

The given vectors are \(\vec{a}=\hat{i}-2 \hat{j}+\hat{k}, \hat{b}=-2 \hat{i}+4 \hat{j}+5 \hat{k}\) and \(\vec{c}=\hat{i}-6 \hat{j}-7 \hat{k}\).

∴ \(\vec{a}+\vec{b}+\vec{c}=(1-2+1) \hat{i}+(-2+4-6) \hat{j}+(1+5-7)) \hat{k}=0 \hat{i}-4 \hat{j}-1 \hat{k}=-4 \hat{j}-\hat{k}\)

Question 7. Find the unit vector in the direction of the vector \(\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}+\hat{\mathrm{j}}+2 \hat{\mathrm{k}}\).
Solution:

The unit vector in the direction of the vector \(\vec{a}=\hat{i}+\hat{j}+2 \hat{k}\) is given by \(\hat{a}=\frac{\vec{a}}{|\vec{a}|}\).

⇒ \(|\vec{a}|=\sqrt{1^2+1^2+2^2}=\sqrt{1+1+4}=\sqrt{6}\)

∴ \(\hat{a}=\frac{\vec{a}}{|\vec{a}|}=\frac{\hat{i}+\hat{j}+2 \hat{k}}{\sqrt{6}}=\frac{1}{\sqrt{6}} \hat{i}+\frac{1}{\sqrt{6}} \hat{j}+\frac{2}{\sqrt{6}} \hat{k}\)

Question 8. Find the unit vector in the direction of the vector \(\overrightarrow{\mathrm{PQ}}\), where P and Q are the points (1, 2, 3) and (4, 5, 6), respectively.
Solution:

The given points are P(l, 2, 3) and Q (4, 5, 6).

∴ \(\overrightarrow{P Q}=(4-1) \hat{i}+(5-2) \hat{j}+(6-3) \hat{k}=3 \hat{i}+3 \hat{j}+3 \hat{k}\)

∴ Magnitude of given vector, \(|\overrightarrow{\mathrm{PQ}}|=\sqrt{3^2+3^2+3^2}=\sqrt{9+9+9}=\sqrt{27}=3 \sqrt{3}\)

Hence, the unit vector in the direction of \(\overrightarrow{\mathrm{PQ}}\) is \(\frac{\overrightarrow{\mathrm{PQ}}}{|\overrightarrow{\mathrm{PQ}}|}=\frac{3 \hat{\mathrm{i}}+3 \hat{\mathrm{j}}+3 \hat{\mathrm{k}}}{3 \sqrt{3}}=\frac{1}{\sqrt{3}} \hat{\mathrm{i}}+\frac{1}{\sqrt{3}} \hat{\mathrm{j}}+\frac{1}{\sqrt{3}} \hat{\mathrm{k}}\)

Question 9. For given vectors, \(\vec{a}=2 \hat{i}-\hat{j}+2 \hat{k}\) and \(\vec{b}=-\hat{i}+\hat{j}-\hat{k}\), find the unit vector in the direction of the vector \(\vec{a}\) + \(\vec{b}\).
Solution:

The given vectors are \(\vec{a}=2 \hat{i}-\hat{j}+2 \hat{k}\) and \(\vec{b}=-\hat{i}+\hat{j}-\hat{k}\).

∴ \(\vec{a}+\vec{b}=(2-1) \hat{i}+(-1+1) \hat{j}+(2-1) \hat{k}=1 \hat{i}++0 \hat{j}+1 \hat{k}=\hat{i}+\hat{k}\)

⇒ \(|\vec{a}+\vec{b}|=\sqrt{1^2+1^2}=\sqrt{2}\)

Hence, the unit vector in the direction of \((\vec{a}+\vec{b})\) is \(\frac{(\vec{a}+\vec{b})}{|\vec{a}+\vec{b}|}=\frac{\hat{i}+\hat{k}}{\sqrt{2}}=\frac{1}{\sqrt{2}} \hat{i}+\frac{1}{\sqrt{2}} \hat{k}\)

Question 10. Find a vector in the direction of the vector \(5 \hat{\mathrm{i}}-\hat{\mathrm{j}}+2 \hat{\mathrm{k}}\) which has magnitude 8 units.
Solution:

Let \(\vec{a}=5 \hat{i}-\hat{j}+2 \hat{k}\)

∴ \(|\vec{a}|=\sqrt{5^2+(-1)^2+2^2}=\sqrt{25+1+4}=\sqrt{30}\)

⇒ \(\hat{a}=\frac{\vec{a}}{|\vec{a}|}=\frac{5 \hat{i}-\hat{j}+2 \hat{k}}{\sqrt{30}}\)

Hence, the vector in the direction of the vector \(5 \hat{i}-\hat{j}+2 \hat{k}\) which has magnitude of 8 units is given by,

8 \(\hat{\mathrm{a}}=8\left(\frac{5 \hat{\mathrm{i}}-\hat{\mathrm{j}}+2 \hat{\mathrm{k}}}{\sqrt{30}}\right)=\frac{40}{\sqrt{30}} \hat{\mathrm{i}}-\frac{8}{\sqrt{30}} \hat{\mathrm{j}}+\frac{16}{\sqrt{30}} \hat{\mathrm{k}}\)

Question 11. Show that the vectors \(2 \hat{i}-3 \hat{j}+4 \hat{k}\) and \(-4 \hat{i}+6 \hat{j}-8 \hat{k}\) are collinear.
Solution:

Let \(\vec{a}=2 \hat{i}-3 \hat{j}+4 \hat{k}\) and \(\vec{b}=-4 \hat{i}+6 \hat{j}-8 \hat{k}\)

It is observed that \(\vec{b}=-4 \hat{i}+6 \hat{j}-8 \hat{k}=-2(2 \hat{i}-3 \hat{j}+4 \hat{k})=-2 \vec{a}\)

∴ \(\overrightarrow{\mathrm{b}}=\lambda \overrightarrow{\mathrm{a}}\)

where, λ=-2,

Hence, the given vectors are collinear.

Question 12. Find the direction cosines of the vector \(\hat{i}+2 \hat{j}+3 \hat{k}\)
Solution:

Let \(\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}+2 \hat{\mathrm{j}}+3 \hat{\mathrm{k}}\)

∴ \(|\vec{a}|=\sqrt{1^2+2^2+3^2}=\sqrt{1+4+9}=\sqrt{14}\)

∴ \(\hat{a}=\frac{\vec{a}}{|\vec{a}|}\)

Hence, the direction cosines of a are \(\frac{1}{\sqrt{14}}, \frac{2}{\sqrt{14}}, \frac{3}{\sqrt{14}}\).

Question 13. Find the direction cosines of the vector joining the points A (1, 2, -3) and B(-l, -2, 1), directed from A to B.
Solution:

The given points are A (1,2, -3) and B (-1, -2, 1).

∴ \(\overrightarrow{\mathrm{AB}}=(-1-1) \hat{\mathrm{i}}+(-2-2) \hat{\mathrm{j}}+\{1-(-3)\} \hat{\mathrm{k}} \Rightarrow \overrightarrow{\mathrm{AB}}=-2 \hat{\mathrm{i}}-4 \hat{\mathrm{j}}+4 \hat{\mathrm{k}}\)

Direction ratios of \(\overrightarrow{\mathrm{AB}}\) are a = -2, b = -4, c = 4

Now direction cosines of \(\overrightarrow{\mathrm{AB}}\) are :

l \(=\frac{-2}{\sqrt{(-2)^2+(-4)^2+(4)^2}}=\frac{-2}{6}=-\frac{1}{3}, \mathrm{~m}=\frac{-4}{\sqrt{(-2)^2+(-4)^2+(4)^2}}=\frac{-4}{6}=-\frac{2}{3}\)

n = \(\frac{4}{\sqrt{(-2)^2+(-4)^2+(4)^2}}=\frac{4}{6}=\frac{2}{3}\)

Hence, the direction cosines of \(\overrightarrow{\mathrm{AB}}\) are \(-\frac{1}{3},-\frac{2}{3}, \frac{2}{3}\).

Question 14. Show that the vector \(\hat{\mathrm{i}}+\hat{\mathrm{j}}+\hat{\mathrm{k}}\) is equally inclined to the axes, OX, OY, and OZ.
Solution:

Let \(\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}+\hat{\mathrm{j}}+\hat{\mathrm{k}}\). Direction ratios of \(\overrightarrow{\mathrm{a}}\) are \(\vec{a}=\mathrm{b}=\mathrm{c}=1\)

Now, direction cosines are

l = \(\frac{1}{\sqrt{(1)^2+(1)^2+(1)^2}}=\frac{1}{\sqrt{3}}=\mathrm{m}=\mathrm{n}\)

Therefore, the direction cosines of \(\vec{a}\) are \(\frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}\).

Now, let \(\alpha, \beta, and \gamma\) be the angles formed by \(\vec{a}\) with the positive directions of x, y, and z axes.

Then, we have \(\cos \alpha=\frac{1}{\sqrt{3}}, \cos \beta=\frac{1}{\sqrt{3}}, \cos \gamma=\frac{1}{\sqrt{3}}, \alpha=\beta=\gamma=\cos ^{-1}\left(\frac{1}{\sqrt{3}}\right)\)

Hence, the given vector is equally inclined to axes OX, OY, and OZ.

Question 15. Find the position vector of a point R which divides the line joining two points P and Q whose position vectors are \(\hat{i}+2 \hat{j}-\hat{k}\) and \(-\hat{i}+\hat{j}+\hat{k}\) respectively, in the ratio 2:1

1. Internally
2. Externally

Solution:

Here, \(\overrightarrow{\mathrm{OP}}=\hat{\mathrm{i}}+2 \hat{\mathrm{j}}-\hat{\mathrm{k}}=\overrightarrow{\mathrm{a}}\) (let) and \(\overrightarrow{\mathrm{OQ}}=-\hat{\mathrm{i}}+\hat{\mathrm{j}}+\hat{\mathrm{k}}=\overrightarrow{\mathrm{b}}\) (let), also m=2, n=1 when R divides PQ internally in the ratio 2: 1, then

1. P . V. of \(R=\frac{m \vec{b}+n \vec{a}}{m+n}\)

= \(\frac{2(-\hat{i}+\hat{j}+\hat{k})+1(\hat{i}+2 \hat{j}-\hat{k})}{2+1}=\frac{(-2 \hat{i}+2 \hat{j}+2 \hat{k})+(\hat{i}+2 \hat{j}-\hat{k})}{3}\)

= \(\frac{-\hat{i}+4 \hat{j}+\hat{k}}{3}=-\frac{1}{3} \hat{i}+\frac{4}{3} \hat{j}+\frac{1}{3} \hat{k}\)

2. when R divides PQ externally in the ratio 2: 1 then,

P.V. of R = \(\frac{m \vec{b}-n \vec{a}}{m-n}\)

= \(\frac{2(-\hat{i}+\hat{j}+\hat{k})-1(\hat{i}+2 \hat{j}-\hat{k})}{2-1}\)

= \(-3 \hat{i}+3 \hat{k}\)

Question 16. Find the position vector of the midpoint of the vector joining the points P (2,3,4) and Q (4, 1, – 2).
Solution:

The position vector of P and Q are given by \(\vec{p}=2 \hat{i}+3 \hat{j}+4 \hat{k}\) and \(\vec{q}=4 \hat{i}+\hat{j}-2 \hat{k}\) respectively

∴ P.V. of midpoint of PQ = \(\frac{1}{2}(\vec{p}+\vec{q})\)

= \(\frac{(2 \hat{i}+3 \hat{j}+4 \hat{k})+(4 \hat{i}+\hat{j}-2 \hat{k})}{2}=\frac{6 \hat{i}+4 \hat{j}+2 \hat{k}}{2}=3 \hat{i}+2 \hat{j}+\hat{k}\)

Question 17. Show that the points A, B and C with position vectors, \(\vec{a}=3 \hat{i}-4 \hat{j}-4 \hat{k}, \vec{b}=2 \hat{i}-\hat{j}+\hat{k}\) and \(\vec{c}=\hat{i}-3 \hat{j}-5 \hat{k}\), respectively form the vertices of a right-angled triangle.
Solution:

Position vectors of points A, B, and C are respectively given as: \(\vec{a}=3 \hat{i}-4 \hat{j}-4 \hat{k}, \vec{b}=2 \hat{i}-\hat{j}+\hat{k}\) and \(\vec{c}=\vec{i}-3 \hat{j}-5 \hat{k}\)

∴ \(\overrightarrow{A B}=\vec{b}-\vec{a}=(2-3) \hat{i}+(-1+4) \hat{j}+(1+4) \hat{k}=-\hat{i}+3 \hat{j}+5 \hat{k}\)

⇒ \(\overrightarrow{B C}=\vec{c}-\vec{b}=(1-2) \hat{i}+(-3+1) \hat{j}+(-5-1) \hat{k}=-\hat{i}-2 \hat{j}-6 \hat{k}\)

⇒ \(\overrightarrow{C A}=\vec{a}-\vec{c}=(3-1) \hat{i}+(-4+3) \hat{j}+(-4+5) \hat{k}=2 \hat{i}-\hat{j}+\hat{k}\)

Now, \(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}+\overrightarrow{\mathrm{CA}}=(-\hat{\mathrm{i}}+3 \hat{\mathrm{j}}+5 \hat{k})+(-\hat{\mathrm{i}}-2 \hat{\mathrm{j}}-6 \hat{\mathrm{k}})+(2 \hat{\mathrm{i}}-\hat{\mathrm{j}}+\hat{\mathrm{k}})=\overrightarrow{0}\)

∴ A, B, and C are vertices of the triangle

Now, \(|\overrightarrow{\mathrm{AB}}|^2=(-1)^2+3^2+5^2=1+9+25=35\)

⇒ \(|\overrightarrow{B C}|^2=(-1)^2+(-2)^2+(-6)^2=1+4+36=41\)

⇒ \(|\overrightarrow{\mathrm{CA}}|^2=2^2+(-1)^2+1^2=4+1+1=6\)

∴ \(|\overrightarrow{\mathrm{AB}}|^2+|\overrightarrow{\mathrm{CA}}|^2=|\overrightarrow{\mathrm{BC}}|^2=35+6=41\)

Hence, A, B, and C are vertices of a right-angled triangle.

Question 18. In triangle ABC which of the following is not true:

1. \(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}+\overrightarrow{\mathrm{CA}}=\overrightarrow{0}\)
2. \(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}-\overrightarrow{\mathrm{AC}}=\overrightarrow{0}\)
3. \(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}-\overrightarrow{\mathrm{CA}}=\overrightarrow{0}\)
4. \(\overrightarrow{\mathrm{AB}}-\overrightarrow{\mathrm{CB}}+\overrightarrow{\mathrm{CA}}=\overrightarrow{0}\)

Solution:

On applying the triangle law of addition in the given triangle, we have:

⇒ \(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}=\overrightarrow{\mathrm{AC}}\)….(1)

⇒ \(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}=-\overrightarrow{\mathrm{CA}} \Rightarrow \overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}+\overrightarrow{\mathrm{CA}}=\overrightarrow{0}\)…..(2)

∴ The equation given in alternative A is true.

⇒ \(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}=\overrightarrow{\mathrm{AC}} \Rightarrow \overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}-\overrightarrow{\mathrm{AC}}=\overrightarrow{0}\)

∴ The equation given in alternative 2 is true. From equation (2), we have: \(\overrightarrow{\mathrm{AB}}\overrightarrow{\mathrm{CB}}+\overrightarrow{\mathrm{CA}}=\overrightarrow{0}\)

∴ The equation given in alternative 4 is true.

Now, consider the equation given in alternative C: \(\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}-\overrightarrow{\mathrm{CA}}=\overrightarrow{0} \Rightarrow \overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{BC}}=\overrightarrow{\mathrm{CA}}[latex]
\)

From equation (1) and (3), we have: \(\overrightarrow{\mathrm{AC}}=\overrightarrow{\mathrm{CA}}\)

∴ \(\overrightarrow{\mathrm{AC}}=-\overrightarrow{\mathrm{AC}} \Rightarrow \overrightarrow{\mathrm{AC}}+\overrightarrow{\mathrm{AC}}=\overrightarrow{0} \Rightarrow 2 \overrightarrow{\mathrm{AC}}=\overrightarrow{0} \Rightarrow \overrightarrow{\mathrm{AC}}=\overrightarrow{0}\), which is not true.

Hence, the equation given in alternative C is incorrect.

The correct answer is (3).

Question 19. If \(\vec{a}\) and \(\vec{b}\) are two collinear vectors, then which of the following are incorrect:

1. \(\vec{b}=\lambda \vec{a}\), for some scalar \(\lambda\)
2. \(\overrightarrow{\mathrm{a}}= \pm \overrightarrow{\mathrm{b}}\)
3. The respective components of \(\vec{a}\) and \(\vec{b}\) are proportional
4. Both the vectors \(\vec{a}\) and \(\vec{b}\) have same direction, but different magnitudes

Solution:

If \(\vec{a}\) and \(\vec{b}\) are two collinear vectors, then they are parallel.

Therefore, we have: \(\vec{b}=\lambda \vec{a}\) (For some scalar \(\lambda\))

If \(\lambda= \pm 1\), then \(\vec{a}= \pm \vec{b}\).

If \(\vec{a}=a_1 \hat{i}+a_2 \hat{j}+a_3 \hat{k}\) and \(\vec{b}=b_1 \hat{i}+b_2 \hat{j}+b_3 \hat{k}\) and \(\vec{b}=\lambda \vec{a}\).

⇒ \(b_1 \hat{i}+b_2 \hat{j}+b_3 \hat{k}=\lambda\left(a_1 \hat{i}+a_2 \hat{j}+a_3 \hat{k}\right)\)

⇒ \(b_1 \hat{i}+b_2 \hat{j}+b_3 \hat{k}=\left(\lambda a_1\right) \hat{i}+\left(\lambda a_2\right) \hat{j}+\left(\lambda a_3\right) \hat{k}\)

⇒ \(\mathrm{b}_1=\lambda \mathrm{a}_1, \mathrm{~b}_2=\lambda \mathrm{a}_2, \mathrm{~b}_3=\lambda \mathrm{a}_3\)

⇒ \(\frac{b_1}{a_1}=\frac{b_2}{a_2}=\frac{b_3}{a_3}=\lambda\)

Thus, the respective scalar components of \(\vec{a}\) and \(\vec{b}\) are proportional.

However, vectors \(\vec{a}\) and \(\vec{b}\) can have different directions.

Hence, the statement given in 4 is incorrect.

The correct answer is 4.

Vector Algebra Exercise 10.3

Question 1. Find the angle between two vectors \(\vec{a}\) and \(\vec{b}\) with magnitude \(\sqrt{3}\) and 2, respectively having \(\vec{a} \cdot \vec{b}=\sqrt{6}\).
Solution:

It is given that, \(|\vec{a}|=\sqrt{3},|\vec{b}|=2\) and, \(\vec{a} \cdot \vec{b}=\sqrt{6}\)

Now, we know that \(\vec{a} \cdot \vec{b}=|\vec{a}||\vec{b}| \cos \theta\).

∴ \(\sqrt{6}=\sqrt{3} \times 2 \times \cos \theta \Rightarrow \cos \theta=\frac{\sqrt{6}}{\sqrt{3} \times 2} \Rightarrow \cos \theta=\frac{1}{\sqrt{2}} \Rightarrow \theta=\frac{\pi}{4}\)

Hence, the angle between the given vectors \(\vec{a}\) and \(\vec{b}\) is \(\frac{\pi}{4}\).

Question 2. Find the angle between the vectors \(\hat{i}-2 \hat{j}+3 \hat{k}\) and \(3 \hat{i}-2 \hat{j}+\hat{k}\).
Solution:

Let \(\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}-2 \hat{\mathrm{j}}+3 \hat{\mathrm{k}}\) and \(\overrightarrow{\mathrm{b}}=3 \hat{\mathrm{i}}-2 \hat{\mathrm{j}}+\hat{\mathrm{k}}\).

⇒ \(|\vec{a}|=\sqrt{1^2+(-2)^2+3^2}=\sqrt{1+4+9}=\sqrt{14}\)

⇒ \(|\vec{b}|=\sqrt{3^2+(-2)^2+1^2}=\sqrt{9+4+1}=\sqrt{14}\)

Now, \(\vec{a} \cdot \vec{b}=(\hat{i}-2 \hat{j}+3 \hat{k}) \cdot(3 \hat{i}-2 \hat{j}+\hat{k})=1 \cdot 3+(-2)(-2)+3 \cdot 1=3+4+3=10\)

Also, we know that \(\vec{a} \cdot \vec{b}=|\vec{a}| \cdot|\vec{b}| \cos \theta\)

∴ 10 = \(\sqrt{14} \sqrt{14} \cos \theta \Rightarrow \cos \theta=\frac{10}{14} \Rightarrow \theta=\cos ^{-1}\left(\frac{5}{7}\right)\)

Question 3. Find the projection of the vector \(\hat{i}-\hat{j}\) on the vector \(\hat{i}+\hat{j}\).
Solution;

Let \(\vec{a}=\hat{i}-\hat{j}\) and \(\vec{b}=\hat{i}+\hat{j}\)

Now, projection of vector \(\vec{a}\) on \(\vec{b}\) is given by, \(\frac{1}{|\vec{b}|}(\vec{a} \cdot \vec{b})=\frac{1}{\sqrt{1+1}}\{1 \cdot 1+(-1)(1)\}=\frac{1}{\sqrt{2}}(1-1)=0\)

Hence, the projection of vector \(\vec{a}\) on \(\vec{b}\) is 0 .

Question 4. Find the projection of the vector \(\hat{i}+3 \hat{j}+7 \hat{k}\) on the vector \(7 \hat{i}-\hat{j}+8 \hat{k}\).
Solution:

Let \(\vec{a}=\hat{i}+3 \hat{j}+7 \hat{k}\) and \(\hat{b}=7 \hat{i}-\hat{j}+8 \hat{k}\).

Now, projection of vector \(\vec{a}\) on \(\vec{b}\) is given by-

⇒ \(\frac{1}{|\vec{b}|}(\vec{a} \cdot \vec{b})=\frac{1}{\sqrt{7^2+(-1)^2+8^2}}\{1(7)+3(-1)+7(8)\}=\frac{7-3+56}{\sqrt{49+1+64}}=\frac{60}{\sqrt{114}}\)

Hence, the projection of vector \(\vec{a}\) on \(\vec{b}\) is \(\frac{60}{\sqrt{114}}\).

Question 5. Show that each of the given three vectors is a unit vector: \(\frac{1}{7}(2 \hat{i}+3 \hat{\mathrm{j}}+6 \hat{\mathrm{k}}) \cdot \frac{1}{7}(3 \hat{\mathrm{i}}-6 \hat{\mathrm{j}}+2 \hat{\mathrm{k}}), \frac{1}{7}(6 \hat{\mathrm{i}}+2 \hat{\mathrm{j}}-3 \hat{\mathrm{k}})\). Also, show that they are mutually perpendicular to each other.
Solution:

Let,

⇒ \(\overrightarrow{\mathrm{a}}=\frac{1}{7}(2 \hat{\mathrm{i}}+3 \hat{\mathrm{j}}+6 \hat{\mathrm{k}})=\frac{2}{7} \hat{\mathrm{i}}+\frac{3}{7} \hat{\mathrm{j}}+\frac{6}{7} \hat{\mathrm{k}}\)

∴ \(|\overrightarrow{\mathrm{a}}|=\sqrt{\left(\frac{2}{7}\right)^2+\left(\frac{3}{7}\right)^2+\left(\frac{6}{7}\right)^2}=\sqrt{\frac{4}{49}+\frac{9}{49}+\frac{36}{49}}=1\)

⇒ \(\overrightarrow{\mathrm{b}}=\frac{1}{7}(3 \hat{\mathrm{i}}-6 \hat{\mathrm{j}}+2 \hat{\mathrm{k}})=\frac{3}{7} \hat{\mathrm{i}}-\frac{6}{7} \hat{\mathrm{j}}+\frac{2}{7} \hat{\mathrm{k}}\)

∴ \(|\overrightarrow{\mathrm{b}}|=\sqrt{\left(\frac{3}{7}\right)^2+\left(-\frac{6}{7}\right)^2+\left(\frac{2}{7}\right)^2}=\sqrt{\frac{9}{49}+\frac{36}{49}+\frac{4}{49}}=1\)

⇒ \({\mathrm{c}}=\frac{1}{7}(6 \hat{\mathrm{i}}+2 \hat{\mathrm{j}}-3 \hat{\mathrm{k}})=\frac{6}{7} \hat{\mathrm{i}}+\frac{2}{7} \hat{\mathrm{j}}-\frac{3}{7} \hat{\mathrm{k}}\)

∴ \(|\overrightarrow{\mathrm{c}}|=\sqrt{\left(\frac{6}{7}\right)^2+\left(\frac{2}{7}\right)_{-}^2+\left(-\frac{3}{7}\right)^2}=\sqrt{\frac{36}{49}+\frac{4}{49}+\frac{9}{49}}=1\)

Thus, each of the given three vectors is a unit vector.

⇒ \(\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{b}}=\frac{2}{7} \times \frac{3}{7}+\frac{3}{7} \times\left(\frac{-6}{7}\right)+\frac{6}{7} \times \frac{2}{7}=\frac{6}{49}-\frac{18}{49}+\frac{12}{49}=0\)

⇒ \(\overrightarrow{\mathrm{b}} \cdot \overrightarrow{\mathrm{c}}=\frac{3}{7} \times \frac{6}{7}+\left(\frac{-6}{7}\right) \times \frac{2}{7}+\frac{2}{7} \times\left(\frac{-3}{7}\right)=\frac{18}{49}-\frac{12}{49}-\frac{6}{49}=0\)

⇒ \(\overrightarrow{\mathrm{c}} \cdot \overrightarrow{\mathrm{a}}=\frac{6}{7} \times \frac{2}{7}+\frac{2}{7} \times \frac{3}{7}+\left(\frac{-3}{7}\right) \times \frac{6}{7}=\frac{12}{49}+\frac{6}{49}-\frac{18}{49}=0\)

Hence, the given three vectors are mutually perpendicular to each other.

Question 6. Find \(|\vec{a}|\) and \(|\vec{b}|\), if \((\vec{a}+\vec{b}) \cdot(\vec{a}-\vec{b})=8\) and \(|\vec{a}|=8|\vec{b}|\).
Solution:

⇒ \((\vec{a}+\vec{b}) \cdot(\vec{a}-\vec{b})=8 \Rightarrow \vec{a} \cdot \vec{a}-\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{a}-\vec{b} \cdot \vec{b}=8\)

⇒ \(|\vec{a}|^2-|\vec{b}|^2=8\)

because \(\vec{a} \cdot \vec{a}=|\vec{a}|^2\)

⇒ \((8 \mid \vec{b})^2-|\vec{b}|^2=8\)

because \(|\vec{a}|=8|\vec{b}|\)

⇒ \(64|\vec{b}|^2-|\vec{b}|^2=8 \Rightarrow 63|\vec{b}|^2=8 \Rightarrow|\vec{b}|^2=\frac{8}{63}\)

⇒ \(|\vec{b}|=\sqrt{\frac{8}{63}}\) Magnitude of a vector is non-negative

⇒ \(|\vec{b}|=\frac{2 \sqrt{2}}{3 \sqrt{7}}\)

⇒ \(|\vec{a}|=8|\vec{b}|=\frac{8 \times 2 \sqrt{2}}{3 \sqrt{7}}=\frac{16 \sqrt{2}}{3 \sqrt{7}}\)

Question 7. Evaluate the product \((3 \vec{a}-5 \vec{b}) \cdot(2 \vec{a}+7 \vec{b})\).
Solution:

⇒ \((3 \vec{a}-5 \vec{b}) \cdot(2 \vec{a}+7 \vec{b})=6(\vec{a} \cdot \vec{a})+21(\vec{a} \cdot \vec{b})-10(\vec{b} \cdot \vec{a})-35(\vec{b} \cdot \vec{b})\)

= \(6(\vec{a} \cdot \vec{a})+21(\vec{a} \cdot \vec{b})-10(\vec{a} \cdot \vec{b})-35(\vec{b} \cdot \vec{b})\)

= \(6|\vec{a}|^2+11 \vec{a} \cdot \vec{b}-35|\vec{b}|^2\) (because \(\vec{a} \cdot \vec{a}=|\vec{a}|^2 \text { and } \vec{a} \cdot \vec{b}=\vec{b} \cdot \vec{a}\))

Question 8. Find the magnitude of two vectors \(\vec{a}\) and \(\vec{b}\), having the same magnitude and such that the angle between them is \(60^{\circ}\) and their scalar product is \(\frac{1}{2}\).
Solution:

Let \(\theta\) be the angle between the vectors \(\vec{a}\) and \(\vec{b}\).

It is given that \(|\vec{a}|=|\vec{b}|, \vec{a} \cdot \vec{b}=\frac{1}{2}\) and \(\theta=60^{\circ}\)…..(1)

We know that \(\vec{a} \cdot \vec{b}=|\vec{a}||\vec{b}| \cos \theta\).

∴ \(\frac{1}{2}=|\vec{a}||\vec{a}| \cos 60^{\circ}\) Using (1)

⇒ \(\frac{1}{2}=|\vec{a}|^2 \times \frac{1}{2} \Rightarrow|\vec{a}|^2=1 \Rightarrow|\vec{a}|=1 \Rightarrow|\vec{a}|=|\vec{b}|=1\)

Question 9. Find \(|\vec{x}|\), if for a unit vector \(\vec{a},(\vec{x}-\vec{a}) \cdot(\vec{x}+\vec{a})=12\).
Solution:

⇒ \((\vec{x}-\vec{a}) \cdot(\vec{x}+\vec{a})=12 \Rightarrow \vec{x} \cdot \vec{x}+\vec{x} \cdot \vec{a}-\vec{a} \cdot \vec{x}-\vec{a} \cdot \vec{a}=12\)

⇒ \(|\vec{x}|^2-|\vec{a}|^2=12\) (because \(\vec{a} \cdot \vec{a}=|\vec{a}|^2\))

and \(\vec{a} \cdot \vec{b}=\vec{b} \cdot \vec{a})\)

⇒ \(|\vec{x}|^2-1=12 \quad |\vec{a}|=1\) as \(\vec{a}\) is a unit vector

⇒ \(|\overrightarrow{\mathrm{x}}|^2=13\)

∴ \(|\mathrm{x}|=\sqrt{13}\)

Question 10. If \(\overrightarrow{\mathrm{a}}=2 \hat{\mathrm{i}}+2 \hat{\mathrm{j}}+3 \hat{\mathrm{k}}, \overrightarrow{\mathrm{b}}=-\hat{\mathrm{i}}+2 \hat{\mathrm{j}}+\hat{\mathrm{k}}\) and \(\overrightarrow{\mathrm{c}}=3 \hat{\mathrm{i}}+\hat{\mathrm{j}}\) are such that \(\overrightarrow{\mathrm{a}}+\lambda \overrightarrow{\mathrm{b}}\) is perpendicular to \(\overrightarrow{\mathrm{c}}\), then find the value of \(\lambda\).
Solution:

The given vectors are \(\vec{a}=2 \hat{i}+2 \hat{j}+3 \hat{k}, \vec{b}=-\hat{i}+2 \hat{j}+\hat{k}\) and [/latex]\vec{c}=3 \hat{i}+\hat{j}[/latex].

Now, \(\vec{a}+\lambda \vec{b}=(2 \hat{i}+2 \hat{j}+3 \hat{k})+\lambda(-\hat{i}+2 \hat{j}+\hat{k})=(2-\lambda) \hat{i}+(2+2 \lambda) \hat{j}+(3+\lambda) \hat{k}\)

If \((\vec{a}+\lambda \vec{b})\) is perpendicular to \(\vec{c}\), then \((\vec{a}+\lambda \vec{b}) \cdot \vec{c}=0\)

⇒ \([(2-\lambda) \hat{\mathrm{i}}+(2+2 \lambda) \hat{\mathrm{j}}+(3+\lambda) \hat{\mathrm{k}}] \cdot(3 \hat{\mathrm{i}}+\hat{\mathrm{j}})=0 \Rightarrow(2-\lambda) 3+(2+2 \lambda) 1+(3+\lambda) 0=0\)

⇒ 6 – \(3 \lambda+2+2 \lambda \Rightarrow 0 \Rightarrow-\lambda+8=0 \Rightarrow \lambda=8\)

Hence, the required value of \(\lambda\) is 8 .

Question 11. Show that \(|\vec{a}| \vec{b}+|\vec{b}| \vec{a}\) is perpendicular to \(|\vec{a}| \vec{b}-|\vec{b}| \vec{a}\), for any two nonzero vectors \(\vec{a}\) and \(\vec{b}\).
Solution:

⇒ \((|\vec{a}| \vec{b}+|\vec{b}| \vec{a}) \cdot(|\vec{a}| \vec{b}-|\vec{b}| \vec{a})\)

= \(|\vec{a}|^2(\vec{b} \cdot \vec{b})-|\vec{a}||\vec{b}|(\vec{b} \cdot \vec{a})+|\vec{b}||\vec{a}|(\vec{a} \cdot \vec{b})-|\vec{b}|^2(\vec{a} \cdot \vec{a})\)

= \(|\vec{a}|^2|\stackrel{\rightharpoonup}{b}|^2-|\vec{b}|^2|\vec{a}|^2=0\) (because \(\vec{a} \cdot \vec{a}=|\vec{a}|^2\) and \(\vec{a} \cdot \vec{b}=\vec{b} \cdot \vec{a}\)

Hence, \(|\vec{a}| \vec{b}+|\vec{b}| \vec{a}\) and \(|\vec{a}| |\vec{b}|-|\vec{b}| \vec{a}\) are perpendicular to each other for any two non-zero vectors \(\vec{a}\) and \(\vec{b}\).

Question 12. If \(\vec{a} \cdot \vec{a}=0\) and \(\vec{a} \cdot \vec{b}=0\), then what can be concluded about the vector \(\vec{b}\)?
Solution:

It is given that \(\vec{a} \cdot \vec{a}=0\) and \(\vec{a} \cdot \vec{b}=0\).

Now, \(\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{a}}=0 \Rightarrow|\overrightarrow{\mathrm{a}}|^2=0 \Rightarrow|\overrightarrow{\mathrm{a}}|=0\)

⇒ \(\overrightarrow{\mathrm{a}}\) is a zero vector.

Hence, vector \(\vec{b}\) satisfying \(\vec{a} \cdot \vec{b}=0\) can be any vector.

Question 13. If \(\vec{a}, \vec{b}, \vec{c}\) are unit vectors such that \(\vec{a}+\vec{b}+\vec{c}=\overrightarrow{0}\), find the value of \(\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{c}+\vec{c} \cdot \vec{a}\).
Solution;

Given \(\vec{a}, \vec{b}, \vec{c}\) are unit vectors, therefore

⇒ \(|\vec{a}|=1,|\vec{b}|=1 \text { and }|\vec{c}|=1 \text {. }\)…….(1)

Again given \(\overrightarrow{\mathrm{a}}+\overrightarrow{\mathrm{b}}+\overrightarrow{\mathrm{c}}=\overrightarrow{0}\)

⇒ \(|\vec{a}+\vec{b}+\vec{c}|=0 \quad \Rightarrow(\vec{a}+\vec{b}+\vec{c}) \cdot(\vec{a}+\vec{b}+\vec{c})=0\)

⇒ \(\vec{a} \cdot \vec{a}+\vec{b} \cdot \vec{b}+\vec{c} \cdot \vec{c}+2(\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{c}+\vec{c} \cdot \vec{a})=0\)

⇒ \(|\vec{a}|^2+|\vec{b}|^2+|\vec{c}|^2+2(\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{c}+\vec{c} \cdot \vec{a})=0\) (because \(\vec{a} \cdot \vec{a}=|\vec{a}|^2)\))

⇒ \(1+1+1+2(\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{c}+\vec{c} \cdot \vec{a})=0\) [Using equation (1)]

⇒ \(2(\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{c}+\vec{c} \vec{a})=-3 \Rightarrow \vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{c}+\vec{c} \cdot \vec{a}=\frac{-3}{2}\)

Question 14. If either vector \(\vec{a}=\overrightarrow{0}\) or \(\vec{b}=\overrightarrow{0}\), then \(\vec{a} \cdot \vec{b}=0\). But the converse need not be true. Justify your answer with an example.
Solution:

Consider \(\vec{a}=2 \hat{i}+4 \hat{j}+3 \hat{k}\) and \(\vec{b}=3 \hat{i}+3 \hat{j}-6 \hat{k}\).

Then, \(\vec{a} \cdot \vec{b}=2 \cdot 3+4 \cdot 3+3(-6)=6+12-18=0\)

We now observe that: \(|\vec{a}|=\sqrt{2^2+4^2+3^2}=\sqrt{29}\)

⇒ \(\vec{a} \neq \overrightarrow{0}\)

⇒ \(|\overrightarrow{\mathrm{b}}|=\sqrt{3^2+3^2+(-6)^2}=\sqrt{54}\)

⇒ \(\overrightarrow{\mathrm{b}} \neq \overrightarrow{0}\)

Hence, the converse of the given statement need not be true.

Question 15. If the vertices A, B, and C of a triangle ABC are (1,2,3),(-1,0,0),(0,1,2), respectively, then find \(\angle \mathrm{ABC}\). \(\angle \mathrm{ABC}\) is the angle between the vectors \(\overrightarrow{\mathrm{BA}}\) and \(\overrightarrow{\mathrm{BC}}\).
Solution:

The vertices of \(\triangle \mathrm{ABC}\) are given as A(1,2,3), B}(-1,0,0), and C(0,1,2). Also, it is given that \(\angle \mathrm{ABC}\) is the angle between the vectors \(\overrightarrow{\mathrm{BA}}\) and \(\overrightarrow{\mathrm{BC}}\).

⇒ \(\overrightarrow{B A}=(1-(-1)) \hat{i}+(2-0) \hat{j}+(3-0) \hat{k}=2 \hat{i}+2 \hat{j}+3 \hat{k}\)

⇒ \(\overrightarrow{\mathrm{BC}}=(0-(-1)) \hat{\mathrm{i}}+(1-0) \hat{\mathrm{j}}+(2-0) \hat{k}=\hat{\mathrm{i}}+\hat{\mathrm{j}}+2 \hat{\mathrm{k}}\)

∴ \(\overrightarrow{\mathrm{BA}} \cdot \overrightarrow{\mathrm{BC}}=(2 \hat{\mathrm{i}}+2 \hat{\mathrm{j}}+3 \hat{\mathrm{k}}) \cdot(\hat{\mathrm{i}}+\hat{\mathrm{j}}+2 \hat{\mathrm{k}})=2 \times 1+2 \times 1+3 \times 2=2+2+6=10\)

⇒ \(|\overrightarrow{\mathrm{BA}}|=\sqrt{2^2+2^2+3^2}=\sqrt{4+4+9}=\sqrt{17}\)

⇒ \(|\overrightarrow{\mathrm{BC}}|=\sqrt{1+1+2^2}=\sqrt{6}\)

Now, it is known that: \(\overrightarrow{\mathrm{BA}} \cdot \overrightarrow{\mathrm{BC}}=|\overrightarrow{\mathrm{BA}}||\overrightarrow{\mathrm{BC}}| \cos (\angle \mathrm{ABC})\)

⇒ 10 = \(\sqrt{17} \times \sqrt{6} \cos (\angle \mathrm{ABC}) \Rightarrow \cos (\angle \mathrm{ABC})=\frac{10}{\sqrt{17} \times \sqrt{6}} \Rightarrow \angle \mathrm{ABC}=\cos ^{-1}\left(\frac{10}{\sqrt{102}}\right)\)

Question 16. Show that the points A(1,2,7), B(2,6,3) and C(3,10,-1) are collinear.
Solution:

The given points are A(1,2,7), B(2,6,3), and C(3,10,-1).

Position vectors of points A, B, and C are \(\vec{a}=\hat{i}+2 \hat{j}+7 \hat{k}, \vec{b}=2 \hat{i}+6 \hat{j}+3 \hat{k}\) and \(\vec{c}=3 \hat{i}+10 \hat{j}-\hat{k}\) respectively.

∴ \(\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm{b}}-\overrightarrow{\mathrm{a}}=(2-1) \hat{i}+(6-2) \hat{j}+(3-7) \hat{k}=\hat{i}+4 \hat{j}-4 \hat{k}\)

∴ \(\overrightarrow{\mathrm{BC}}=\overrightarrow{\mathrm{c}}-\overrightarrow{\mathrm{b}}=(3-2) \hat{i}+(10-6) \hat{j}+(-1-3) \hat{k}=\hat{i}+4 \hat{j}-4 \hat{k}\)

Since \(\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm{BC}} \Rightarrow \overrightarrow{\mathrm{AB}} \| \overrightarrow{\mathrm{BC}}\)

Here, point B is common in \(\overrightarrow{\mathrm{AB}}\) and \(\overrightarrow{\mathrm{BC}}\)

So, \(\overrightarrow{\mathrm{AB}}\) and \(\overrightarrow{\mathrm{BC}}\) are collinear.

Hence, A, B, and C are collinear

Question 17. Show that the vectors \(2 \hat{i}-\hat{j}+\hat{k}, \hat{i}-3 \hat{j}-5 \hat{k}\) and \(3 \hat{i}-4 \hat{j}-4 \hat{k}\) form the vertices of right angled triangle.
Solution:

Let vectors \(2 \hat{i}-\hat{j}+\hat{k}, \hat{i}-3 \hat{j}-5 \hat{k}\) and \(3 \hat{i}-4 \hat{j}-4 \hat{k}\) be position vectors of points A, B and C respectively.

i.e., \(\overline{O A}=2 \hat{i}-\hat{j}+\hat{k}, \overrightarrow{O B}=\hat{i}-3 \hat{j}-5 \hat{k}\) and \(\overline{O C}=3 \hat{i}-4 \hat{j}-4 \hat{k}\)

∴ \(\overrightarrow{A B}=(1-2) \hat{i}+(-3+1) \hat{j}+(-5-1) \hat{k}=-\hat{i}-2 \hat{j}-6 \hat{k}\)

∴ \(\overrightarrow{\mathrm{BC}}=(3-1) \hat{i}+(-4+3) \hat{j}+(-4+5) \hat{k}=2 \hat{i}-\hat{j}+\hat{k}\)

∴ \(\overrightarrow{\mathrm{AC}}=(3-2) \hat{\mathrm{i}}+(-4+1) \hat{\mathrm{j}}+(-4-1) \hat{k}=\hat{\mathrm{i}}-3 \hat{\mathrm{j}}-5 \hat{\mathrm{k}}\)

Now, \(\overrightarrow{A B}+\overrightarrow{B C}=(-\hat{i}-2 \hat{j}+6 \hat{k})+(2 \hat{i}-\hat{j}+\hat{k})=\hat{i}-3 \hat{j}-5 \hat{k}=\overrightarrow{A C}\)

A, B, and C are vertices of a triangle.

Now, \(|\overrightarrow{\mathrm{AB}}|=\sqrt{(-1)^2+(-2)^2+(-6)^2}=\sqrt{1+4+36}=\sqrt{41}\)

∴ \(|\overrightarrow{\mathrm{BC}}|=\sqrt{2^2+(-1)^2+1^2}=\sqrt{4+1+1}=\sqrt{6}\)

∴ \(|\overrightarrow{\mathrm{AC}}|=\sqrt{(1)^2+(-3)^2+(-5)^2}=\sqrt{1+9+25}=\sqrt{35}\)

∴ \(|\overrightarrow{\mathrm{BC}}|^2+|\overrightarrow{\mathrm{AC}}|^2=6+35=41=|\overrightarrow{\mathrm{AB}}|^2\)

Hence, A, B, and C are vertices of a right-angle triangle.

Question 18. If \(\vec{a}\) is a nonzero vector of magnitude ‘a’ and \(\lambda\) a nonzero scalar, then \(\lambda \vec{a}\) is unit vector if

1. \(\lambda=1\)
2. \(\lambda=-1\)
3. \(a=|\lambda|\)
4. \(a=\frac{1}{|\lambda|}\)

Solution: 4. \(a=\frac{1}{|\lambda|}\)

Given \(\lambda \vec{a}\) is a unit vector.

∴ \(|\lambda \vec{a}|=1 \quad \Rightarrow \quad|\lambda||\vec{a}|=1\)

⇒ \(|\vec{a}|=\frac{1}{|\lambda|} \quad[\lambda \neq 0]\)

⇒ \(a=\frac{1}{|\lambda|}\) (because \(|\vec{a}|\)=a)

Hence, vector \(\lambda \vec{a}\) is a unit vector if \(\mathrm{a}=\frac{1}{|\vec{\lambda}|}, \quad(\lambda \neq 0)\)

The correct answer is (4).

Vector Algebra Exercise 10.4

Question 1. Find \(|\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}|\), if \(\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}-7 \hat{\mathrm{j}}+7 \hat{\mathrm{k}}\) and \(\overrightarrow{\mathrm{b}}=3 \hat{\mathrm{i}}-2 \hat{\mathrm{j}}+2 \hat{\mathrm{k}}\).
Solution:

We have, \(\vec{a}=\hat{i}-7 \hat{j}+7 \hat{k}\) and \(\vec{b}=3 \hat{i}-2 \hat{j}+2 \hat{k}\)

⇒ \(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}\)

= \(\left|\begin{array}{ccc}
\hat{\mathrm{i}} & \hat{\mathrm{j}} & \hat{\mathrm{k}} \\
1 & -7 & 7 \\
3 & -2 & 2
\end{array}\right|\)

= \(\hat{\mathrm{i}}(-14+14)-\hat{\mathrm{j}}(2-21)+\hat{\mathrm{k}}(-2+21)=0 \hat{\mathrm{i}}+19 \hat{\mathrm{j}}+19 \hat{\mathrm{k}}\)

∴ \(|\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}|=\sqrt{(19)^2+(19)^2}=\sqrt{2 \times(19)^2}=19 \sqrt{2}\)

Question 2. Find a unit vector perpendicular to each of the vectors \(\vec{a}+\vec{b}\) and \(\vec{a}-\vec{b}\), where \(\vec{a}=3 \hat{i}+2 \hat{j}+2 \hat{k}\) and \(\vec{b}=\hat{i}+2 \hat{j}-2 \hat{k}\).
Solution:

We have, \(\vec{a}=3 \hat{i}+2 \hat{j}+2 \hat{k}\) and \(\vec{b}=\hat{i}+2 \hat{j}-2 \hat{k}\)

∴ \(\vec{a}+\vec{b}=4 \hat{i}+4 \hat{j}, \vec{a}-\vec{b}=2 \hat{i}+4 \hat{k}\)

∴ \((\vec{a}+\vec{b}) \times(\vec{a}-\vec{b})\)

= \(\left|\begin{array}{lll}
\hat{i} & \hat{j} & \hat{k} \\
4 & 4 & 0 \\
2 & 0 & 4
\end{array}\right|\)

= \(\hat{i}(16)-\hat{j}(16)+\hat{k}(-8)=16 \hat{i}-16 \hat{j}-8 \hat{k}\)

∴ \(|(\vec{a}+\vec{b}) \times(\vec{a}-\vec{b})|=\sqrt{16^2+(-16)^2+(-8)^2}=8 \sqrt{2^2+2^2+1}=8 \sqrt{9}=8 \times 3=24\)

Hence, the unit vector perpendicular to each of the vectors \(\vec{a}+\vec{b}\) and \(\vec{a}-\vec{b}\) is given by the relation,

± \(\frac{(\vec{a}+\vec{b}) \times(\vec{a}-\vec{b})}{|(\vec{a}+\vec{b}) \times(\vec{a}-\vec{b})|}= \pm \frac{(16 \hat{i}-16 \hat{j}-8 \hat{k})}{24}= \pm \frac{(2 \hat{i}-2 \hat{j}-\hat{k})}{3}\)

Required vector is \(\frac{2}{3} \hat{\mathrm{i}}-\frac{2}{3} \hat{\mathrm{j}}-\frac{1}{3} \hat{\mathrm{k}}\) or \(-\frac{2}{3} \hat{\mathrm{i}}+\frac{2}{3} \hat{\mathrm{j}}+\frac{1}{3} \hat{\mathrm{k}}\)

Question 3. If a unit vector a makes an angle \(\frac{\pi}{3}\) with \(\hat{i}, \frac{\pi}{4}\) with \(\hat{j}\) and an acute angle \(\theta\) with \(\hat{k}\), then find \(\theta\) and hence, the components of \(\vec{a}\).
Solution:

Let unit vector \(\vec{a}=a_1 \hat{i}+a_2 \hat{j}+a_3 \hat{k}\)

Since \(\vec{a}\) is a unit vector, \(|\vec{a}|=1\).

Also, it is given that \(\overrightarrow{\mathrm{a}}\) makes angle \(\frac{\pi}{3}\) with \(\hat{\mathrm{i}}, \frac{\pi}{4}\) with \(\hat{\mathrm{j}}\) and an acute angle \(\theta\) with \(\hat{k}\).

Then, we have: \(\cos \frac{\pi}{3}=\frac{a_1}{|\vec{a}|} \Rightarrow \frac{1}{2}=a_1 \quad[|\vec{a}|=1]\)

Also, \(\cos \frac{\pi}{4}=\frac{\mathrm{a}_2}{|\overrightarrow{\mathrm{a}}|} \Rightarrow \frac{1}{\sqrt{2}}=\mathrm{a}_2\) (\(|\vec{a}|=1\))

Also, \(\cos \theta=\frac{\mathrm{a}_3}{|\overrightarrow{\mathrm{a}}|} \Rightarrow \mathrm{a}_3=\cos \theta\)

Now, \(|\overrightarrow{\mathrm{a}}|=1 \Rightarrow \sqrt{\mathrm{a}_1^2+\mathrm{a}_2^2+\mathrm{a}_3^2}=1\)

⇒ \(\left(\frac{1}{2}\right)^2+\left(\frac{1}{\sqrt{2}}\right)^2+\cos ^2 \theta=1 \Rightarrow \frac{1}{4}+\frac{1}{2}+\cos ^2 \theta=1\)

∴ \(\frac{3}{4}+\cos ^2 \theta=1 \Rightarrow \cos ^2 \theta=1-\frac{3}{4}=\frac{1}{4} \Rightarrow \cos \theta=\frac{1}{2} \Rightarrow \theta=\frac{\pi}{3}\) (because \(\theta\) is acute angle)

∴ \(a_3=\cos \frac{\pi}{3}=\frac{1}{2}\)

Hence, \(\theta=\frac{\pi}{3}\) and the components of a are \(\frac{1}{2}, \frac{1}{\sqrt{2}}, \frac{1}{2}\).

Question 4. Show that \((\vec{a}-\vec{b}) \times(\vec{a}+\vec{b})=2(\vec{a} \times \vec{b})\)
Solution:

L.H.S. \((\vec{a}-\vec{b}) \times(\vec{a}+\vec{b})\)

= \((\vec{a}-\vec{b}) \times \vec{a}+(\vec{a}-\vec{b}) \times \vec{b}\) [By distributivity of vector product over addition]

= \(\vec{a} \times \vec{a}-\vec{b} \times \vec{a}+\vec{a} \times \vec{b}-\vec{b} \times \vec{b}\) [Again, by distributivity of vector product over addition]

= \(\overrightarrow{0}+\vec{a} \times \vec{b}+\vec{a} \times \vec{b}-\overrightarrow{0}\)

= \(2(\vec{a} \times \vec{b})\) R.H.S.

Question 5. Find \(\lambda\) and \(\mu\) if \((2 \hat{i}+6 \hat{j}+27 \hat{k}) \times(\hat{i}+\lambda \hat{j}+\mu \hat{k})=\overrightarrow{0}\).
Solution:

⇒ \((2 \hat{i}+6 \hat{j}+27 \hat{k}) \times(\hat{i}+\lambda \hat{j}+\mu \hat{k})=\overrightarrow{0}\)

⇒ \(\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
2 & 6 & 27 \\
1 & \lambda & \mu
\end{array}\right|\)

= \(0 \hat{\mathrm{i}}+0 \hat{\mathrm{j}}+0 \hat{\mathrm{k}} \Rightarrow \hat{\mathrm{i}}(6 \mu-27 \lambda)-\hat{\mathrm{j}}(2 \mu-27)+\hat{\mathrm{k}}(2 \lambda-6)\)

=0 \(\hat{\mathrm{i}}+0 \hat{\mathrm{j}}+0 \hat{\mathrm{k}}\)

On comparing the corresponding components, we have :

6 \(\mu-27 \lambda=0\)….(1)

2 \(\mu-27=0\)….(2)

2 \(\lambda-6=0\)….(3)

Now, from equation (3), \(2 \lambda-6=0 \Rightarrow \lambda=3\)

from equation (2), \(2 \mu-27=0 \Rightarrow \mu=\frac{27}{2}\)

Here, values of \(\lambda\) and \(\mu\) satisfy to equation (1)

Hence, \(\lambda=3\) and \(\mu=\frac{27}{2}\)

Question 6. Given that \(\vec{a} \cdot \vec{b}=0\) and \(\vec{a} \times \vec{b}=\overrightarrow{0}\). What can you conclude about the vectors \(\vec{a}\) and \(\vec{b}\)?
Solution:

⇒ \(\vec{a}, \vec{b}=0\) Either \(|\vec{a}|=0\) or \(|\vec{b}|=0\) or \(\vec{a} \perp \vec{b}\)

⇒ \(\vec{a} \times \vec{b}=0\) Then, either \(|\vec{a}|=0\) or \(|\vec{b}|=0\) or \(\vec{a} \| \vec{b}\)

But, \(\vec{a}\) and \(\vec{b}\) cannot be perpendicular and parallel simultaneously.

Hence, \(|\vec{a}|=0\) or \(|\vec{b}|=0\). i.e. either \(\vec{a}=\overrightarrow{0}\) or \(\vec{b}=\overrightarrow{0}\)

Question 7. Let the vectors \(\vec{a}, \vec{b}, \vec{c}\) are given as \(a_1 \hat{i}+a_2 \hat{j}+a_3 \hat{k}, b_1 \hat{i}+b_2 \hat{j}+b_3 \hat{k}, c_1 \hat{i}+c_2 \hat{j}+c_3 \hat{k}\) respectively.

Then show that \(\vec{a} \times(\vec{b}+\vec{c})=\vec{a} \times \vec{b}+\vec{a} \times \vec{c}\).

Solution:

We have \(\vec{a}=a_1 \hat{i}+a_2 \hat{j}+a_3 \hat{k}, \vec{b}=b_1 \hat{i}+b_2 \hat{j}+b_3 \hat{k}, \vec{c}=c_1 \hat{i}+c_2 \hat{j}+c_3 \hat{k}\)

⇒ \((\vec{b}+\vec{c})=\left(b_1+c_1\right) \hat{i}+\left(b_2+c_2\right) \hat{j}+\left(b_3+c_3\right) \hat{k}\)

Now, \(\vec{a} \times(\vec{b}+\vec{c})\left|\begin{array}{ccc}\hat{i} & \hat{j} & \hat{k} \\ a_1 & a_2 & a_3 \\ b_1+c_1 & b_2+c_2 & b_3+c_3\end{array}\right|\)

= \(\hat{i}\left[a_2\left(b_3+c_3\right)-a_3\left(b_2+c_2\right)\right]-\hat{j}\left[a_1\left(b_3+c_3\right)-a_3\left(b_1+c_1\right)\right]+\hat{k}\left[a_1\left(b_2+c_2\right)-a_2\left(b_1+c_1\right)\right]\)

= \(\hat{i}\left[a_2 b_3+a_2 c_3-a_3 b_2-a_3 c_2\right]+\hat{j}\left[-a_1 b_3-a_1 c_3+a_3 b_1+a_3 c_1\right]\)+\(\hat{k}\left[a_1 b_2+a_1 c_2-a_2 b_1-a_2 c_1\right]\)…..(1)

⇒ \(\vec{a} \times \vec{b}=\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
a_1 & a_2 & a_3 \\
b_1 & b_2 & b_3
\end{array}\right|\)

= \(\hat{i}\left[a_2 b_3-a_3 b_2\right]+\hat{j}\left[a_3 b_1-a_1 b_3\right]+\hat{k}\left[a_1 b_2-a_2 b_1\right]\)….(2)

⇒ \(\vec{a} \times \vec{c}=\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
a_1 & a_2 & a_3 \\
c_1 & c_2 & c_3
\end{array}\right|\)

= \(\hat{i}\left[a_2 c_3-a_3 c_2\right]+\hat{j}\left[a_3 c_1-a_1 c_3\right]+\hat{k}\left[a_1 c_2-a_2 c_1\right]\)….(3)

On adding (2) and (3), we get :

(\(\vec{a} \times \vec{b})+(\vec{a} \times \vec{c})=\hat{i}\left[a_2 b_3+a_2 c_3-a_3 b_2-a_3 c_2\right]+\hat{j}\left[b_1 a_3+a_3 c_1-a_1 b_3-a_1 c_3\right]\)

+ \(\hat{k}\left[a_1 b_2+a_1 c_2-a_2 b_1-a_2 c_1\right]\)….(4)

Now, from (1) and (4), we have: \(\vec{a} \times(\vec{b}+\vec{c})=\vec{a} \times \vec{b}+\vec{a} \times \vec{c}\)

Question 8. If either \(\vec{a}=\overrightarrow{0}\) or \(\vec{b}=\overrightarrow{0}\), then \(\vec{a} \times \vec{b}=\overrightarrow{0}\). Is the converse true? Justify your answer with an example.
Solution:

Take any parallel non-zero vectors so that \(\vec{a} \times \vec{b}=\overrightarrow{0}\).

Let \(\vec{a}=2 \hat{i}+3 \hat{j}+4 \hat{k}, \vec{b}=4 \hat{i}+6 \hat{j}+8 \hat{k}\).

Then, \(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}\)

= \(\left|\begin{array}{lll}\hat{\mathrm{i}} & \hat{\mathrm{j}} & \hat{\mathrm{k}} \\ 2 & 3 & 4 \\ 4 & 6 & 8\end{array}\right|=\hat{\mathrm{i}}(24-24)-\hat{\mathrm{j}}(16-16)+\hat{\mathrm{k}}(12-12)=0 \hat{\mathrm{i}}+0 \hat{\mathrm{j}}+0 \hat{\mathrm{k}}\)

= \(\overrightarrow{0}\)

It can now be observed that : \(|\vec{a}|=\sqrt{2^2+3^2+4^2}=\sqrt{29}\)

⇒ \(\vec{a} \neq \overrightarrow{0}\)

⇒ \(|\vec{b}|=\sqrt{4^2+6^2+8^2}=\sqrt{116}\)

⇒ \(\vec{b} \neq \overrightarrow{0}\)

Hence, the converse of the given statement need not be true.

Question 9. Find the area of the triangle with vertices A(1,1,2), B(2,3,5) and C(1,5,5).
Solution:

The vertices of triangle ABC are given as A(1,1,2), B(2,3,5), and C(1,5,5).

Position vector of points A, B and C are \(\vec{a}=\hat{i}+\hat{j}+2 \hat{k}, \vec{b}=2 \hat{i}+3 \hat{j}+5 \hat{k}\) and \(\vec{c}=\hat{i}+5 \hat{j}+5 \hat{k}\) respectively.

The adjacent sides A, B and \(\overrightarrow{B C}\) of \(\triangle A B C\) are given as:

⇒ \(\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm{b}}-\overrightarrow{\mathrm{a}} \Rightarrow \overrightarrow{\mathrm{AB}}=(2-1) \hat{\mathrm{i}}+(3-1) \hat{\mathrm{j}}+(5-2) \hat{\mathrm{k}}=\hat{\mathrm{i}}+2 \hat{\mathrm{j}}+3 \hat{\mathrm{k}}\)

and \(\overrightarrow{\mathrm{BC}}=\overrightarrow{\mathrm{c}}-\overrightarrow{\mathrm{b}} \Rightarrow \overrightarrow{\mathrm{BC}}=(1-2) \hat{\mathrm{i}}+(5-3) \hat{\mathrm{j}}+(5-5) \hat{k}=-\hat{\mathrm{i}}+2 \hat{\mathrm{j}}\)

∴ \(\overrightarrow{\mathrm{AB}} \times \overrightarrow{\mathrm{BC}}\)

= \(\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
1 & 2 & 3 \\
-1 & 2 & 0
\end{array}\right|\)

= \(\hat{i}(-6)-\hat{j}(3)+\hat{k}(2+2)=-6 \hat{i}-3 \hat{j}+4 \hat{k}\)

∴ \(|\overrightarrow{\mathrm{AB}} \times \overrightarrow{\mathrm{BC}}|=\sqrt{(-6)^2+(-3)^2+4^2}=\sqrt{36+9+16}=\sqrt{61}\)

Area of \(\triangle \mathrm{ABC}\)=\(\frac{1}{2}|\overrightarrow{\mathrm{AB}} \times \overrightarrow{\mathrm{BC}}|=\frac{1}{2} \sqrt{61}\)

Area of \(\triangle \mathrm{ABC}=\frac{1}{2}|\overrightarrow{\mathrm{AB}} \times \overrightarrow{\mathrm{BC}}|=\frac{1}{2} \sqrt{61}\)

Hence, the area of \(\triangle \mathrm{ABC}\) is \(\frac{\sqrt{61}}{2}\) square units.

Question 10. Find the area of the parallelogram whose adjacent sides are determined by the vector \(\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}-\hat{\mathrm{j}}+3 \hat{\mathrm{k}}\) and \(\vec{b}=2 \hat{i}-7 \hat{j}+\hat{k}\).
Solution:

The area of the parallelogram whose adjacent sides are \(\vec{a}\) and \(\vec{b}\) is \(|\vec{a} \times \vec{b}|\).

Adjacent sides are given as: \(\vec{a}=\hat{i}-\hat{j}+3 \hat{k}\) and \(\vec{b}=2 \hat{i}-7 \hat{j}+\hat{k}\)

∴ \(\vec{a} \times \vec{b}\)

= \(\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
1 & -1 & 3 \\
2 & -7 & 1
\end{array}\right|\)

= \(\hat{i}(-1+21)-\hat{j}(1-6)+\hat{k}(-7+2)=20 \hat{i}+5 \hat{j}-5 \hat{k}\)

⇒ \(|\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}|=\sqrt{20^2+5^2+5^2}=\sqrt{400+25+25}=15 \sqrt{2}\)

Hence, the area of the given parallelogram is \(15 \sqrt{2}\) square units.

Choose The Correct Answer

Question 11. Let the vectors \(\vec{a}\) and \(\vec{b}\) be such that \(|\vec{a}|=3\) and \(|\vec{b}|=\frac{\sqrt{2}}{3}\), then \(\vec{a} \times \vec{b}\) is a unit vector, if the angle between \(\vec{a}\) and \(\vec{b}\) is

1. \(\frac{\pi}{6}\)
2. \(\frac{\pi}{4}\)
3. \(\frac{\pi}{3}\)
4. \(\frac{\pi}{2}\)

Solution: 2. \(\frac{\pi}{4}\)

It is given that \(|\vec{a}|=3\) and \(|\vec{b}|=\frac{\sqrt{2}}{3}\).

We know that \(\vec{a} \times \vec{b}=|\vec{a}||\vec{b}| \sin \theta \hat{n}\), where \(\hat{n}\) is a unit vector perpendicular to both \(\vec{a}\) and \(\vec{b}\) and \(\theta\) is the angle between \(\vec{a}\) and \(\vec{b}\).

Now, \(\vec{a} \times \vec{b}\) is a unit vector if \(|\vec{a} \times \vec{b}|=1\)

⇒ \(|\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}|=1 \Rightarrow||\overrightarrow{\mathrm{a}}||\overrightarrow{\mathrm{b}}| \sin \theta|=1 \Rightarrow 3 \times \frac{\sqrt{2}}{3} \times \sin \theta=1 \Rightarrow \sin \theta=\frac{1}{\sqrt{2}} \Rightarrow \theta=\frac{\pi}{4}\)

Hence, \(\vec{a} \times \vec{b}\) is a unit vector if the angle between \(\vec{a}\) and \(\vec{b}\) is \(\frac{\pi}{4}\).

The correct answer is 2.

Question 12. Area of a rectangle having vertices A, B, C, and D with position vectors \(-\hat{i}+\frac{1}{2} \hat{j}+4 \hat{k}\) \(\hat{i}+\frac{1}{2} \hat{j}+4 \hat{k}, \hat{i}-\frac{1}{2} \hat{j}+4 \hat{k},-\hat{i}-\frac{1}{2} \hat{j}+4 \hat{k}\) respectively is-

1. \(\frac{1}{2}\)
2. 1
3. 2
4. 4

Solution: 3. 2

The position vectors of vertices A, B, C, and D of rectangle ABCD are given as:

⇒ \(\overrightarrow{O A}=-\hat{i}+\frac{1}{2} \hat{j}+4 \hat{k}, \overrightarrow{O B}=\hat{i}+\frac{1}{2} \hat{j}+4 \hat{k}, \overrightarrow{O C}=\hat{i}-\frac{1}{2} \hat{j}+4 \hat{k}, \overrightarrow{O D}=-\hat{i}-\frac{1}{2} \hat{j}+4 \hat{k}\)

The adjacent sides \(\overrightarrow{\mathrm{AB}}\) and \(\overrightarrow{\mathrm{BC}}\) of the given rectangle are given as:

⇒ \(\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm{OB}}-\overrightarrow{\mathrm{OA}}=(1+1) \hat{\mathrm{i}}+\left(\frac{1}{2}-\frac{1}{2}\right) \hat{\mathrm{j}}+(4-4) \hat{\mathrm{k}}=2 \hat{\mathrm{i}}\)

⇒ \(\overrightarrow{\mathrm{BC}}=\overrightarrow{\mathrm{OC}}-\overrightarrow{\mathrm{OB}}=(1-1) \hat{\mathrm{i}}+\left(-\frac{1}{2}-\frac{1}{2}\right) \hat{\mathrm{j}}+(4-4) \hat{\mathrm{k}}=-\hat{\mathrm{j}}\)

∴ \(\overrightarrow{\mathrm{AB}} \times \overrightarrow{\mathrm{BC}}\)

= \(\left|\begin{array}{ccc}
\hat{\mathrm{i}} & \hat{\mathrm{j}} & \hat{\mathrm{k}} \\
2 & 0 & 0 \\
0 & -1 & 0
\end{array}\right|\)

= \(\hat{\mathrm{k}}(-2)=-2 \hat{\mathrm{k}} \Rightarrow|\overrightarrow{\mathrm{AB}} \times \overrightarrow{\mathrm{BC}}|=\sqrt{(-2)^2}=2\)

Now, it is known that the area of a rectangle whose adjacent sides are \(\vec{a}\) and \(\vec{b}\) is \(|\vec{a} \times \vec{b}|\)

Hence, the area of the given rectangle is \(|\overrightarrow{A B} \times \overrightarrow{B C}|=2\) square units.

The correct answer is 3.

Vector Algebra Miscellaneous Exercise

Question 1. Write down a unit vector in XY-plane, making an angle of 30n with the positive direction of the x-axis.
Solution:

If \(\overrightarrow{\mathrm{r}}\) is a unit vector in the \(\mathrm{XY}\)-plane, then \(\overrightarrow{\mathrm{r}}=\cos \theta \hat{\mathrm{i}}+\sin \theta \hat{\mathrm{j}}\).

Here, \(\theta\) is the angle made by the unit vector with the positive direction of the x-axis.

Therefore, for \(\theta=30^{\circ}\):

⇒ \(\overrightarrow{\mathrm{r}}=\cos 30^{\circ} \hat{\mathrm{i}}+\sin 30^{\circ} \hat{\mathrm{j}}=\frac{\sqrt{3}}{2} \hat{\mathrm{i}}+\frac{1}{2} \hat{\mathrm{j}}\)

Hence, the required unit vector is \(\frac{\sqrt{3}}{2} \hat{\mathrm{i}}+\frac{1}{2} \hat{\mathrm{j}}\)

Question 2. Find the scalar components and magnitude of the vector joining the points \(P\left(x_1, y_1, z_1\right)\) and \(\mathrm{Q}\left(\mathrm{x}_2, \mathrm{y}_2, \mathrm{z}_2\right)\).
Solution:

The vector joining the points \(\mathrm{P}\left(\mathrm{x}_1, \mathrm{y}_1, \mathrm{z}_1\right)\) and \(\mathrm{Q}\left(\mathrm{x}_2, \mathrm{y}_2, \mathrm{z}_2\right)\) can be obtained by,

⇒ \(\overrightarrow{P Q}\) = P.V. of Q-P.V. of P =\(\left(x_2-x_1\right) \hat{i}+\left(y_2-y_1\right) \hat{j}+\left(z_2-z_1\right) \hat{k}\)

⇒ \(|\overrightarrow{P Q}|=\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2+\left(z_2-z_1\right)^2}\)

Hence, the scalar components and the magnitude of the vector joining the given points are \(\left(x_2-x_1\right),\left(y_2-y_1\right),\left(z_2-z_1\right)\) and \(\sqrt{\left(x_2-x_1\right)^2+\left(y_2-y_1\right)^2+\left(z_2-z_1\right)^2}\) respectively

Question 3. A girl walks \(4 \mathrm{~km}\) towards west, then she walks \(3 \mathrm{~km}\) in a direction \(30^{\circ}\) east of north and stops. Determine the girl’s displacement from her initial point of departure.
Solution:

Let O and B be the initial and final positions of the girl respectively. Then, the girl’s position can be shown as:

OA= \(4 \mathrm{~km}, \mathrm{AB}=3 \mathrm{~km}, \overrightarrow{\mathrm{OA}}=-4 \hat{\mathrm{i}}\) and \(\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm{AC}}+\overrightarrow{\mathrm{CB}} \)

⇒ \(\overrightarrow{\mathrm{AB}}=\left(|\overrightarrow{\mathrm{AB}}| \cos 60^{\circ}\right) \hat{\mathrm{i}}+\left(|\overrightarrow{\mathrm{AB}}| \sin 60^{\circ}\right) \hat{\mathrm{j}}\)

= \(3 \times \frac{1}{2} \hat{\mathrm{i}}+3 \times \frac{\sqrt{3}}{2} \hat{\mathrm{j}}=\frac{3}{2} \hat{\mathrm{i}}+\frac{3 \sqrt{3}}{2} \hat{\mathrm{j}}\)

By the triangle law of vector addition, we have: \(\overrightarrow{\mathrm{OB}} =\overrightarrow{\mathrm{OA}}+\overrightarrow{\mathrm{AB}}=(-4 \hat{\mathrm{i}})+\left(\frac{3}{2} \hat{\mathrm{i}}+\frac{3 \sqrt{3}}{2} \hat{\mathrm{j}}\right)=\left(-4+\frac{3}{2}\right) \hat{\mathrm{i}}+\frac{3 \sqrt{3}}{2} \hat{\mathrm{j}}\)

= \(\left(\frac{-8+3}{2}\right) \hat{\mathrm{i}}+\frac{3 \sqrt{3}}{2} \hat{\mathrm{j}}=\left(\frac{-5}{2}\right) \hat{\mathrm{i}}+\frac{3 \sqrt{3}}{2} \hat{\mathrm{j}}\)

Hence, the girl’s displacement from her initial point of departure is \(\left(\frac{-5}{2}\right) \hat{\mathrm{i}}+\frac{3 \sqrt{3}}{2} \hat{\mathrm{j}}\)

Question 4. If \(\vec{a}=\vec{b}+\vec{c}\), then is it true that \(|\vec{a}|=|\vec{b}|+|\vec{c}|\)? Justify your answer.
Solution:

In \(\triangle \mathrm{ABC}\), let \(\overrightarrow{\mathrm{CB}}=\overrightarrow{\mathrm{a}}, \overrightarrow{\mathrm{CA}}=\overrightarrow{\mathrm{b}}\), and \(\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm{c}}\) (as shown in the following figure).

Now, by the triangle law of vector addition, we have \(\vec{a}=\vec{b}+\vec{c}\).

It is clearly known that \(|\vec{a}|,|\vec{b}|\) and \(|\vec{c}|\) represent the sides of \(\triangle \mathrm{ABC}\).

Also, it is known that the sum of the lengths of any two sides of a triangle is greater than the third side.

∴ \(|\vec{a}|<|\vec{b}|+|\vec{c}|\)

Hence, it is not true that \(|\vec{a}|=|\vec{b}|+|\vec{c}|\).

Question 5. Find the value of x for which \(x(\hat{i}+\hat{j}+\hat{k})\) is a unit vector.
Solution:

If \(x(\hat{i}+\hat{j}+\hat{k})\) is a unit vector, then \(|x(\hat{i}+\hat{j}+\hat{k})|=1\)

⇒ \(\sqrt{\mathrm{x}^2+\mathrm{x}^2+\mathrm{x}^2}=1 \Rightarrow \sqrt{3 \mathrm{x}^2}=1 \Rightarrow \pm \sqrt{3} \mathrm{x}=1 \Rightarrow \mathrm{x}= \pm \frac{1}{\sqrt{3}}\)

Hence, the required value of x is \(\pm \frac{1}{\sqrt{3}}\).

Question 6. Find a vector of magnitude 5 units, and parallel to the resultant of the vectors \(\vec{a}=2 \hat{i}+3 \hat{j}-\hat{k}\) and \(\overrightarrow{\mathrm{b}}=\hat{\mathrm{i}}-2 \hat{\mathrm{j}}+\hat{\mathrm{k}}\).
Solution:

We have, \(\vec{a}=2 \hat{i}+3 \hat{j}-\hat{k}\) and \(\vec{b}=\hat{i}-2 \hat{j}+\hat{k}\)

Let \(\vec{c}\) be the resultant of \(\vec{a}\) and \(\vec{b}\).

Then, \(\vec{c}=\vec{a}+\vec{b}=(2+1) \hat{i}+(3-2) \hat{j}+(-1+1) \hat{k}=3 \hat{i}+\hat{j}\)

⇒ \(|\overrightarrow{\mathrm{c}}|=\sqrt{3^2+1^2}=\sqrt{9+1}=\sqrt{10}\)

⇒ \(\hat{\mathrm{c}}=\frac{\overrightarrow{\mathrm{c}}}{|\overrightarrow{\mathrm{c}}|}=\frac{(3 \hat{\mathrm{i}}+\hat{\mathrm{j}})}{\sqrt{10}}\)

Hence, the vector of magnitude 5 units and parallel to the resultant of vectors \(\vec{a}\) and \(\vec{b}\) is \(\pm 5\). \(\hat{\mathrm{c}}= \pm 5 \cdot \frac{1}{\sqrt{10}}(3 \hat{\mathrm{i}}+\hat{\mathrm{j}})= \pm \frac{3 \sqrt{10}}{2} \hat{\mathrm{i}} \pm \frac{\sqrt{10}}{2} \hat{\mathrm{j}}\).

Question 7. If \(\vec{a}=\hat{i}+\hat{j}+\hat{k}, \vec{b}=2 \hat{i}-\hat{j}+3 \hat{k}\) and \(\vec{c}=\hat{i}-2 \hat{j}+3 \hat{k}\), find a unit vector parallel to the vector \(2 \vec{a}-\vec{b}+3 \vec{c}\).
Solution:

We have, \(\vec{a}=\hat{i}+\hat{j}+\hat{k}, \vec{b}=2 \hat{i}-\hat{j}+3 \hat{k}\) and \(\vec{c}=\hat{i}-2 \hat{j}+3 \hat{k}\)

⇒ \(2 \vec{a}-\vec{b}+3 \vec{c}=2(\hat{i}+\hat{j}+\hat{k})-(2 \hat{i}-\hat{j}+3 \hat{k})+3(\hat{i}-2 \hat{j}+\hat{k})\)

= \(2 \hat{i}+2 \hat{j}+2 \hat{k}-2 \hat{i}+\hat{j}-3 \hat{k}+3 \hat{i}-6 \hat{j}+3 \hat{k}=3 \hat{i}-3 \hat{j}+2 \hat{k}\)

⇒ \(|2 \vec{a}-\vec{b}+3 \vec{c}|=\sqrt{3^2+(-3)^2+2^2}=\sqrt{9+9+4}=\sqrt{22}\)

Hence, the unit vector parallel to \(2 \vec{a}-\vec{b}+3 \vec{c}\) is.

± \(\frac{(2 \vec{a}-\vec{b}+3 \vec{c})}{2 \vec{a}-\vec{b}+3 \vec{c}}= \pm \frac{3 \hat{i}-3 \hat{j}+2 \hat{k}}{\sqrt{22}}= \pm \frac{3}{\sqrt{22}} \hat{i} \mp \frac{3}{\sqrt{22}} \hat{j} \pm \frac{2}{\sqrt{22}} \hat{k}\)

Question 8. Show that the points A(1,-2,-8), B(5,0,-2) and C(11,3,7) are collinear, and find the ratio in which B divides AC.
Solution:

The given points are A(1,-2,-8), B(5,0,-2), and C(11,3,7).

P.V. of point A is \(\vec{a}=\hat{i}-2 \hat{j}-8 \hat{k}\)

P.V. of point B is \(\vec{b}=5 \hat{i}-2 \hat{k}\)

P.V. of point C is \(\overrightarrow{\mathrm{c}}=11 \hat{\mathrm{i}}+3 \hat{\mathrm{j}}+7 \hat{\mathrm{k}}\)

⇒ \(\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm{b}}-\overrightarrow{\mathrm{a}} =(5-1) \hat{\mathrm{i}}+(0+2) \hat{\mathrm{j}}+(-2+8) \hat{k}=4 \hat{\mathrm{i}}+2 \hat{\mathrm{j}}+6 \hat{\mathrm{k}}\)

⇒ \(\overrightarrow{\mathrm{BC}}=\overrightarrow{\mathrm{c}}-\overrightarrow{\mathrm{b}} =(11-5) \hat{\mathrm{i}}+(3-0) \hat{\mathrm{j}}+(7+2) \hat{k}=6 \hat{i}+3 \hat{\mathrm{j}}+9 \hat{\mathrm{k}}\)

= \(\frac{3}{2}(4 \hat{\mathrm{i}}+2 \hat{\mathrm{j}}+6 \hat{\mathrm{k}})=\frac{3}{2} \overrightarrow{\mathrm{AB}}\)

⇒\(\overrightarrow{\mathrm{BC}}=\frac{3}{2} \overrightarrow{\mathrm{AB}} \text { i.e. } \overrightarrow{\mathrm{BC}} \| \overrightarrow{\mathrm{AB}}\)

Here, B is common in \(\overrightarrow{\mathrm{BC}}\) and \(\overrightarrow{\mathrm{AB}}\)

So, \(\overrightarrow{\mathrm{BC}}\) and \(\overrightarrow{\mathrm{AB}}\) are collinear

Hence, A, B, and C are collinear

Now, let point B divide AC in the ratio \(\lambda: 1\). Then, we have:

⇒ \(\overrightarrow{\mathrm{b}}=\frac{\lambda \overrightarrow{\mathrm{c}}+\overrightarrow{\mathrm{a}}}{\lambda+1}\)

⇒ \(5 \hat{\mathrm{i}}-2 \hat{\mathrm{k}}=\frac{\lambda(11 \hat{\mathrm{i}}+3 \hat{\mathrm{j}}+7 \hat{\mathrm{k}})+(\hat{\mathrm{i}}-2 \hat{\mathrm{j}}-8 \hat{\mathrm{k}})}{\lambda+1}\)

⇒ \((\lambda+1)(5 \hat{\mathrm{i}}-2 \hat{\mathrm{k}})=(11 \lambda \hat{\mathrm{i}}+3 \lambda \hat{\mathrm{j}}+7 \lambda \hat{\mathrm{k}})+(\hat{\mathrm{i}}-2 \hat{\mathrm{j}}-8 \hat{\mathrm{k}})\)

⇒ \(5(\lambda+1) \hat{\mathrm{i}}-2(\lambda+1) \hat{\mathrm{k}}=(11 \lambda+1) \hat{\mathrm{i}}+(3 \lambda-2) \hat{\mathrm{j}}+(7 \lambda-8) \hat{\mathrm{k}}\)

On equating the corresponding components, we get: 5\((\lambda+1)=11 \lambda+1\)

⇒ 5\(\lambda+5=11 \lambda+1\)

⇒ 6\(\lambda=4 \Rightarrow \lambda=\frac{4}{6}=\frac{2}{3}\)

Hence, point B divides AC in the ratio 2: 3, internally.

Question 9. Find the position vector of a point R which divides the line joining two points P and Q whose position vectors are \((2 \vec{a}+\vec{b})\) and \((\vec{a}-3 \vec{b})\) externally in the ratio 1: 2. Also, show that P is the midpoint of the line segment RQ.
Solution:

It is given that \(\overrightarrow{O P}=2 \vec{a}+\vec{b}, \overrightarrow{O Q}=\vec{a}-3 \vec{b}\).

It is given that point R divides a line segment joining two points P and Q externally in the ratio 1: 2.

Then, on using the section formula, we get: \(\overrightarrow{\mathrm{OR}}=\frac{2(2 \overrightarrow{\mathrm{a}}+\overrightarrow{\mathrm{b}})-(\overrightarrow{\mathrm{a}}-3 \overrightarrow{\mathrm{b}})}{2-1}=\frac{4 \overrightarrow{\mathrm{a}}+2 \overrightarrow{\mathrm{b}}-\overrightarrow{\mathrm{a}}+3 \overrightarrow{\mathrm{b}}}{1}=3 \overrightarrow{\mathrm{a}}+5 \overrightarrow{\mathrm{b}}\)

Therefore, the position vector of point R is \(3 \vec{a}+5 \vec{b}\).

Position vector of the mid-point of RQ = \(\frac{\overrightarrow{\mathrm{OQ}}+\overrightarrow{\mathrm{OR}}}{2}=\frac{(\overrightarrow{\mathrm{a}}-3 \overrightarrow{\mathrm{b}})+(3 \overrightarrow{\mathrm{a}}+5 \overrightarrow{\mathrm{b}})}{-2}=2 \overrightarrow{\mathrm{a}}+\overrightarrow{\mathrm{b}}=\overrightarrow{\mathrm{OP}}\)

Hence, P is the mid-point of the line segment RQ.

Question 10. The two adjacent sides of a parallelogram are \(2 \hat{i}-4 \hat{j}+5 \hat{k}\) and \(\hat{i}-2 \hat{j}-3 \hat{k}\). Find the unit vector parallel to its diagonal. Also, find its area.
Solution:

Adjacent sides of a parallelogram are given as: \(\vec{a}=2 \hat{i}-4 \hat{j}+5 \hat{k}\) and \(\vec{b}=\hat{i}-2 \hat{j}-3 \hat{k}\).

Then, the diagonal of a parallelogram is given by \(\vec{a}+\vec{b}\).

= \((2+1) \hat{i}+(-4-2) \hat{j}+(5-3) \hat{k}=3 \hat{i}-6 \hat{j}+2 \hat{k}\)

Thus, the unit vector parallel to the diagonal is

± \(\frac{\vec{a}+\vec{b}}{|\vec{a}+\vec{b}|}= \pm \frac{3 \hat{i}-6 \hat{j}+2 \hat{k}}{\sqrt{3^2+(-6)^2+2^2}}= \pm \frac{3 \hat{i}-6 \hat{j}+2 \hat{k}}{\sqrt{9+36+4}}= \pm \frac{3 \hat{i}-6 \hat{j}+2 \hat{k}}{7}\)

= \(\pm\left(\frac{3}{7} \hat{i}-\frac{6}{7} \hat{j}+\frac{2}{7} \hat{k}\right)\).

Now, Area of parallelogram ABCD = \(|\vec{a} \times \vec{b}|\)

⇒ \(\vec{a} \times \vec{b}\)

= \(\left|\begin{array}{ccc}
\hat{i} & \hat{j} & \hat{k} \\
2 & -4 & 5 \\
1 & -2 & -3
\end{array}\right|\)

= \(\hat{i}(12+10)-\hat{j}(-6-5)+\hat{k}(-4+4)=22 \hat{i}+11 \hat{j}\)

⇒ \(\vec{a} \times \vec{b}=11(2 \hat{i}+\hat{j})\)

⇒ \(|\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}|=11 \sqrt{2^2+1^2}=11 \sqrt{5}\)

Hence, the area of the parallelogram is \(11 \sqrt{5}\) square units.

Question 11. Show that the direction cosines of a vector equally inclined to the axes OX, OY, and OZ are \(\frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}\).
Solution:

Let a vector be equally inclined to axes OX, OY, and OZ at angle \(\alpha\).

Then, the direction cosines of the vector are cos\(\alpha\), cos\(\alpha\), and cos\(\alpha\).

Now, \(\cos ^2 \alpha+\cos ^2 \alpha+\cos ^2 \alpha=1 \Rightarrow 3 \cos ^2 \alpha=1 \Rightarrow \cos \alpha= \pm \frac{1}{\sqrt{3}}\)

Hence, the direction cosines of the vector which are equally inclined to the axes are \(\frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}\) or \(-\frac{1}{\sqrt{3}},-\frac{1}{\sqrt{3}},-\frac{1}{\sqrt{3}}\).

Question 12. Let \(\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}+4 \hat{\mathrm{j}}+2 \hat{\mathrm{k}}, \overrightarrow{\mathrm{b}}=3 \hat{\mathrm{i}}-2 \hat{\mathrm{j}}+7 \hat{\mathrm{k}}\) and \(\overrightarrow{\mathrm{c}}=2 \hat{\mathrm{i}}-\hat{\mathrm{j}}+4 \hat{\mathrm{k}}\). Find a vector \(\overrightarrow{\mathrm{d}}\) which is perpendicular to both \(\overrightarrow{\mathrm{a}}\) and \(\overrightarrow{\mathrm{b}}\), and \(\overrightarrow{\mathrm{c}} \cdot \overrightarrow{\mathrm{d}}=15\).
Solution:

Vector \(\vec{d}\) is perpendicular to both \(\vec{a}\) and \(\vec{b} \Rightarrow \vec{d}=\lambda(\vec{a} \times \vec{b})\)

Now, \(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}\)

= \(\left|\begin{array}{ccc}
\hat{\mathrm{i}} & \hat{\mathrm{j}} & \hat{\mathrm{k}} \\
1 & 4 & 2 \\
3 & -2 & 7
\end{array}\right|\)

= \(32 \hat{\mathrm{i}}-\hat{\mathrm{j}}-14 \hat{\mathrm{k}}\)

∴ \(\overrightarrow{\mathrm{d}}=\lambda(32 \hat{\mathrm{i}}-\hat{\mathrm{j}}-14 \hat{\mathrm{k}})\)

Now, \(\overrightarrow{\mathrm{c}} \cdot \overrightarrow{\mathrm{d}}=15 \Rightarrow(2 \hat{\mathrm{i}}-\hat{\mathrm{j}}+4 \hat{\mathrm{k}}) \cdot(32 \lambda \hat{\mathrm{i}}-\lambda \hat{\mathrm{j}}-14 \lambda \hat{\mathrm{k}})=15\)

⇒ 64 \(\lambda+\lambda-56 \lambda=15 \Rightarrow \lambda=\frac{5}{3} \Rightarrow \overrightarrow{\mathrm{d}}=\frac{5}{3}(32 \hat{\mathrm{i}}-\hat{\mathrm{j}}-14 \hat{\mathrm{k}})\)

Question 13. The scalar product of the vector \(\hat{i}+\hat{j}+\hat{k}\) with a unit vector along the sum of the vector \(2 \hat{i}+4 \hat{j}-5 \hat{k}\) and \(\lambda \hat{i}+2 \hat{j}+3 \hat{k}\) is equal to one. Find the value of \(\lambda\).
Solution:

Let, \(\vec{a}=\hat{i}+\hat{j}+\hat{k}, \vec{b}=2 \hat{i}+4 \hat{j}-5 \hat{k}, \vec{c}=\lambda \hat{i}+2 \hat{j}+3 \hat{k}\)

⇒ \(\vec{b}+\vec{c}=(2 \hat{i}+4 \hat{j}-5 \hat{k})+(\lambda \hat{i}+2 \hat{j}+3 \hat{k})=(2+\lambda) \hat{i}+6 \hat{j}-2 \hat{k}\)

Therefore, unit vector along \(\vec{b}+\vec{c}\) is given as:

± \(\frac{(2+\lambda) \hat{i}+6 \hat{j}-2 \hat{k}}{\sqrt{(2+\lambda)^2+6^2+(-2)^2}}= \pm \frac{(2+\lambda) \hat{i}+6 \hat{j}-2 \hat{k}}{\sqrt{4+4 \lambda+\lambda^2+36+4}}= \pm \frac{(2+\lambda) \hat{i}+6 \hat{j}-2 \hat{k}}{\sqrt{\lambda^2+4 \lambda+44}}\)

Scalar product of (\(\vec{a}\)) with this unit vector is 1 .

⇒ \((\hat{i}+\hat{j}+\hat{k}),\left\{ \pm \frac{(2+\lambda) \hat{i}+6 \hat{j}-2 \hat{k}}{\sqrt{\lambda^2+4 \lambda+44}}\right\}=1 \Rightarrow \frac{(2+\lambda)+6-2}{\sqrt{\lambda^2+4 \lambda+44}}= \pm 1\)

⇒ \(\sqrt{\lambda^2+4 \lambda+44}= \pm(\lambda+6) \Rightarrow \lambda^2+4 \lambda+44=(\lambda+6)^2\)

⇒ \(\lambda^2+4 \lambda+44=\lambda^2+12 \lambda+36 \Rightarrow 8 \lambda=8 \Rightarrow \lambda=1\)

Hence, the value of \(\lambda\) is 1 .

Question 14. If \(\vec{a}, \vec{b}, \vec{c}\) are mutually perpendicular vectors of equal magnitudes, show that the vector \(\vec{a}+\vec{b}+\vec{c}\) is equally inclined to \(\vec{a}, \vec{b}\) and \(\vec{c}\).
Solution:

Since \(\vec{a}, \vec{b}\) and \(\vec{c}\) are mutually perpendicular vectors, we have \(\vec{a} \cdot \vec{b}=\vec{b} \cdot \vec{c}=\vec{c} \cdot \vec{a}=0\)

It is given that: \(|\vec{a}|=|\vec{b}|=|\vec{c}|\)

Let vector \(\vec{a}+\vec{b}+\vec{c}\) be inclined to \(\vec{a}, \vec{b}\) and \(\vec{c}\) at angles \(\theta_1, \theta_2\) and \(\theta_3\) respectively.

Then, we have:

cos\(\theta_1=\frac{(\vec{a}+\vec{b}+\vec{c}) \cdot \vec{a}}{|\vec{a}+\vec{b}+\vec{c}||\vec{a}|}=\frac{\vec{a} \cdot \vec{a}+\vec{b} \cdot \vec{a}+\vec{c} \cdot \vec{a}}{|\vec{a}+\vec{b}+\vec{c}||\vec{a}|}\) (\(\vec{b} \cdot \vec{a}=\vec{c} \cdot \vec{a}=0\))

= \(\frac{|\vec{a}|^2}{|\vec{a}+\vec{b}+\vec{c}||\vec{a}|}=\frac{|\vec{a}|}{|\vec{a}+\vec{b}+\vec{c}|}\)

cos\(\theta_2=\frac{(\vec{a}+\vec{b}+\vec{c}) \cdot \vec{b}}{|\vec{a}+\vec{b}+\vec{c}||\vec{b}|}=\frac{\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{ba}+\vec{c} \cdot \vec{b}}{|\vec{a}+\vec{b}+\vec{c}||\vec{b}|}\) (\(\vec{b} \cdot \vec{a}=\vec{c} \cdot \vec{b}=0\))

= \(\frac{|\vec{b}|^2}{|\vec{a}+\vec{b}+\vec{c}||\vec{b}|}=\frac{|\vec{b}|}{|\vec{a}+\vec{b}+\vec{c}|}\)

cos\(\theta_3=\frac{(\vec{a}+\vec{b}+\vec{c}) \cdot \vec{c}}{|\vec{a}+\vec{b}+\vec{c}||\vec{c}|}=\frac{\vec{a} \cdot \vec{c}+\vec{b} \cdot \vec{c}+\vec{c} \cdot \vec{c}}{|\vec{a}+\vec{b}+\vec{c}||\vec{c}|}\)

= \(\frac{|\vec{c}|^2}{\mid \vec{a}+\vec{b}+\vec{c}=0]}=\frac{|\vec{c}|}{|\vec{a}+\vec{b}+\vec{c}|}\)

Now, as \(|\vec{a}|=|\vec{b}|=|\vec{c}|\)

∴ \(\cos \theta_1=\cos \theta_2=\cos \theta_3 \Rightarrow \theta_1=\theta_2=\theta_3\)

Hence, the vector \((\vec{a}+\vec{b}+\vec{c})\) is equally inclined to \(\vec{a}, \vec{b}\) and \(\vec{c}\).

Question 15. Prove that \((\vec{a}+\vec{b}) \cdot(\vec{a}+\vec{b})=|\vec{a}|^2+|\vec{b}|^2\), if and only if \(\vec{a}, \vec{b}\) are perpendicular, given \(\vec{a} \neq \overrightarrow{0}, \vec{b} \neq \overrightarrow{0}\).
Solution:

Let \((\vec{a}+\vec{b})(\vec{a}+\vec{b})=|\vec{a}|^2+|\vec{b}|^2\)

⇒ \(\vec{a} \cdot \vec{a}+\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{a}+\vec{b} \cdot \vec{b}=|\vec{a}|^2+|\vec{b}|^{-2}\) [Distributivity of scalar products over addition]

⇒ \(|\vec{a}|^2+2 \vec{a} \cdot \vec{b}+|\vec{b}|^2=|\vec{a}|^2+|\vec{b}|^2 \quad[\vec{a} \cdot \vec{b}=\vec{b} \cdot \vec{a}\) (Scalar product is commutative)

⇒ \(2 \vec{a} \cdot \vec{b}=0 \quad \Rightarrow \vec{a} \cdot \vec{b}=0\)

∴ \(\vec{a}\) and \(\vec{b}\) are perpendicular. \(\vec{a} \neq \overrightarrow{0}, \vec{b} \neq \overrightarrow{0}\) (Given)

Further, let \(\vec{a} \perp \vec{b} \Rightarrow \vec{a} \cdot \vec{b}=0\)

Now \((\vec{a}+\vec{b}) \cdot(\vec{a}+\vec{b})=\vec{a} \cdot \vec{a}+\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{a}+\vec{b} \cdot \vec{b}=|\vec{a}|^2+|\vec{b}|^2+2(\vec{a} \cdot \vec{b}) \cdot\)

= \(|\vec{a}|^2+|\vec{b}|^2\) (because \(\vec{a} \cdot \vec{b}=0\))

Hence, \((\vec{a}+\vec{b})(\vec{a}+\vec{b})=|\vec{a}|^2+|\vec{b}|^2\)

Choose The Correct Answer

Question 16. If \(\theta\) is the angle between two vectors \(\vec{a}\) and \(\vec{b}\), then \(\vec{a} \cdot \vec{b} \geq 0\) only when:

1. \(0<\theta<\frac{\pi}{2}\)
2. \(0 \leq \theta \leq \frac{\pi}{2}\)
3. \(0<\theta<\pi\)
4. \(0 \leq \theta \leq \pi\)

Solution: 2. \(0 \leq \theta \leq \frac{\pi}{2}\)

Let \(\theta\) be the angle between two vectors \(\vec{a}\) and \(\vec{b}\), if \(\vec{a} \cdot \vec{b} \geq 0\)

⇒ \(|\vec{a}||\vec{b}| \cos \theta \geq 0 \Rightarrow \cos \theta \geq 0\)

⇒ \([|\vec{a}| and |\vec{b}|\) are positive]

⇒ \(0 \leq \theta \leq \frac{\pi}{2}\)

Hence, \(\vec{a} \cdot \vec{b} \geq 0\) when \(0 \leq \theta \leq \frac{\pi}{2}\).

The correct answer is (B).

Question 17. Let \(\vec{a}\) and \(\vec{b}\) be two unit vectors and \(\theta\) is the angle between them. Then \(\vec{a}+\vec{b}\) is a unit vector if :

1. \(\theta=\frac{\pi}{4}\)
2. \(\theta=\frac{\pi}{3}\)
3. \(\theta=\frac{\pi}{2}\)
4. \(\theta=\frac{2 \pi}{3}\)

Solution: \(\theta=\frac{2 \pi}{3}\)

Let \(\vec{a}\) and \(\vec{b}\) be two unit vectors and \(\theta\) be the angle between them. Then, \(|\vec{a}|=|\vec{b}|=1\).

Now, \(\vec{a}+\vec{b}\) is a unit vector then \(|\vec{a}+\vec{b}|=1\)

⇒ \(|\vec{a}+\vec{b}|^2=1 \Rightarrow(\vec{a}+\vec{b}) \cdot(\vec{a}+\vec{b})=1\)

⇒ \(\vec{a} \cdot \vec{a}+\vec{a} \cdot \vec{b}+\vec{b} \cdot \vec{a}+\vec{b} \cdot \vec{b}=1\) (because \(\vec{a} \cdot \vec{a}=|\vec{a}|^2)\))

⇒ \(|\vec{a}|^2+2 \vec{a} \cdot \vec{b}+|\vec{b}|^2=1 \Rightarrow 1+2|\vec{a}||\vec{b}| \cos \theta+1=1 \Rightarrow \cos \theta=-\frac{1}{2} \Rightarrow \theta=\frac{2 \pi}{3}\)

Hence, \(\vec{a}+\vec{b}\) is a unit vector if \(\theta=\frac{2 \pi}{3}\).

The correct answer is (4).

Question 18. The value of \(\hat{i} \cdot(\hat{j} \times \hat{k})+\hat{j} \cdot(\hat{i} \times \hat{k})+\hat{k} \cdot(\hat{i} \times \hat{j})\) is

1. 0
2. -1
3. 1
4. 3

Solution: 3. 1

⇒ \(\hat{i} \cdot(\hat{j} \times \hat{k})+\hat{j} \cdot(\hat{i} \times \hat{k})+\hat{k} \cdot(\hat{i} \times \hat{j})=\hat{i} \cdot \hat{i}+\hat{j} \cdot(-\hat{j})+\hat{k} \cdot \hat{k}=1-1+1=1\)

The correct answer is (3).

Question 19. If \(\theta\) is the angle between any two vectors \(\vec{a}\) and \(\vec{b}\), then \(|\vec{a} \cdot \vec{b}|=|\vec{a} \times \vec{b}|\) when \(\theta\) is equal to

1. 0
2. \(\frac{\pi}{4}\)
3. \(\frac{\pi}{2}\)
4. \(\pi\)

Solution: 2. \(\frac{\pi}{4}\)

Let \(\theta\) be the angle between two vectors \(\vec{a}\) and \(\vec{b}\).

⇒ \(|\vec{a} \cdot \vec{b}|=|\vec{a} \times \vec{b}| \Rightarrow|\vec{a}||\vec{b}| \cos \theta=|\vec{a}||\vec{b}| \sin \theta\)

cos \(\theta=\sin \theta \Rightarrow \tan \theta=1 \Rightarrow \theta=\frac{\pi}{4}\)

Hence, \(|\vec{a} \cdot \vec{b}|=|\vec{a} \times \vec{b}|\) when \(\theta\) is equal to \(\frac{\pi}{4}\).

The correct answer is (2).

 

 

 

 

 

 

 

 

 

 

 

 

 

Differential Equation Exercise 9.1

Determine the Order And Degree (If Defined) Of Differential Equations

Question 1. \(\frac{d^4 y}{d x^4}+\sin \left(y^{\prime \prime \prime}\right)=0\)
Solution:

⇒ \(\frac{\mathrm{d}^4 \mathrm{y}}{\mathrm{dx}}+\sin \left(\mathrm{y}^{\prime \prime \prime}\right)=0 \Rightarrow \mathrm{y}^{\prime \prime \prime \prime}+\sin \left(\mathrm{y}^{\prime \prime \prime}\right)=0\)

The highest order derivative present in the differential equation is y””. Therefore, its order is four.

The given differential equation is not a polynomial equation in its derivatives. Hence, its degree is not defined.

Question 2. y’+5y = 0
Solution:

The given differential equation is: y’ + 5y = 0

The highest-order derivative present in the differential equation is y’. Therefore, its order is one.

It is a polynomial equation in y’. The highest power raised to y’ is 1. Hence, its degree is one.

Question 3. \(\left(\frac{\mathrm{ds}}{\mathrm{dt}}\right)^4+3 \mathrm{~s} \frac{\mathrm{d}^2 \mathrm{~s}}{\mathrm{dt}^2}=0\)
Solution:

The highest order derivative present in the given differential equation is \(\frac{\mathrm{d}^2 \mathrm{~s}}{\mathrm{dt}^2}=0\). Therefore, its order is two.

It is a polynomial equation in \(\frac{\mathrm{d}^2 \mathrm{~s}}{\mathrm{dt}^2}=0\) and \(\frac{ds}{dt}\). The power raised to \(\frac{\mathrm{d}^2 \mathrm{~s}}{\mathrm{dt}^2}=0\) is 1.

Hence, its degree is one.

Read and Learn More Class 12 Maths Chapter Wise with Solutions

Question 4. \(\left(\frac{d^2 y}{d x^2}\right)^2+\cos \left(\frac{d y}{d x}\right)=0\)
Solution:

The highest order derivative present in the given differential equation is \(\frac{d^2 y}{d x^2}\) order is 2.

The given differential equation is not a polynomial equation in its derivatives. Hence, its degree is not defined.

Question 5. \(\frac{d^2 y}{d x^2}=\cos 3 x+\sin 3 x\)
Solution:

⇒ \(\frac{d^2 y}{d x^2}=\cos 3 x+\sin 3 x \Rightarrow \frac{d^2 y}{d x^2}-\cos 3 x-\sin 3 x=0\)

The highest order derivative present in the differential equation is \(\frac{d^2 y}{d x^2}\). Therefore, its order is two.

It is a polynomial equation in \(\frac{d^2 y}{d x^2}\) and the power raised to \(\frac{d^2 y}{d x^2}\) is 1.

Hence, its degree is one.

Question 6. \(\left(y^{\prime \prime \prime}\right)^2+\left(y^{\prime \prime}\right)^3+\left(y^{\prime}\right)^4+y^3=0\)
Solution:

The highest order derivative present in the differential equation is \(\left(y^{\prime \prime \prime}\right)\).

Therefore, its order is three. The given differential equation is a polynomial equation \(iny^{\prime \prime \prime}, y^{\prime \prime} \text { and } y^{\prime} \text {. }\)

The highest power raised to \(y^{\prime \prime \prime}\) is 2. Hence, its degree is 2.

Question 7. \(y^{\prime \prime \prime}+2 y^{\prime \prime}+y^{\prime}=0\)
Solution:

The highest order derivative present in the differential equation is \(y^{\prime \prime \prime}\). Therefore, its order is three.It is a polynomial equation in \(y^{\prime \prime \prime}\), y” and y’. The highest power raised to y is 1. Hence, its degree is 1.

Question 8. y’ + y = e^x
Solution:

y’ + y = e^x ⇒ y’ + y = e^x = 0

The highest-order derivative present in the differential equation is y’. Therefore, its order is one. The given differential equation is a polynomial equation and the highest power raised to y’ is one. Hence, its degree is one.

 

Quetsion 9. y”+(y’)² + 2y = 0
Solution:

The highest order derivative present in the differential equation is Therefore, its order is two.

The given differential equation is a polynomial equation in y” and y’ and the highest power raised to y” is one.

Hence, its degree is one. y”+ 2y’+ sin y = 0

Question 10. y” + 2y’ + sin y = 0
Solution:

The highest order derivative present in the differential equation is y”. Therefore, its order is two. This is a polynomial equation in y” and y’, and the highest power raised to y” is one.

Hence, its degree is one.

Question 11. The degree of the differential equation \(\left(\frac{d^2 y}{d x^2}\right)^3+\left(\frac{d y}{d x}\right)^2+\sin \left(\frac{d y}{d x}\right)+1=0\) is

1. 3
2. 2
3. 1
4. Not Defined

Solution:

The given differential equation is not a polynomial equation in its derivatives. Therefore, its degree is not defined.

Hence, the correct answer is D.

Question 12. The order of the differential equation \(2 x^2 \frac{d^2 y}{d x^2}-3 \frac{d y}{d x}+y=0\)

1. 2
2. 1
3. 0
4. Not Defined

Solution:

The highest order derivative present in the given differential equation is \(\frac{\mathrm{d}^2 \mathrm{y}}{\mathrm{dx}^2}\). Therefore, its order is two.

Hence, the correct answer is A.

Differential Equation Exercise 9.2

Verify That The Given Function (Explicit Or Implicit) Is A Solution Of The Corresponding Differential Equation:

Question 1. y = e^x + 1 : y” – y’ = 0
Solution:

y = e^x + 1

Differentiating both sides of this equation with respect to x, we get: \(\frac{d y}{d x}=\frac{d}{d x}\left(e^x+1\right) \Rightarrow y^{\prime}=e^x\)….(1)

Now, again differentiating equation (1) with respect to x, we get: \(\frac{d}{d x}\left(y^{\prime}\right)=\frac{d}{d x}\left(e^x\right) \Rightarrow y^{\prime \prime}=e^x\)

Substituting the values of y’ and y” in the given differential equation, we get the L.H.S. as y” – y’ = e^x – e^x = 0 = R.H.S.

Thus, the given function is the solution of the corresponding differential equation.

Question 2. y = x² + 2x + C : y’ – 2x – 2 = 0
Solution:

y = x² + 2x + C

Differentiating both sides of this equation with respect to x, we get:

y’ = \(\frac{d}{dx}\)(X² + 2X + C) ⇒ y’ = 2x+2 dx

Substituting the value of y’ in the given differential equation, we get:

L.H.S. = y’ – 2x – 2 =2x + 2-2x-2 = 0 = R.H.S.

Hence, the given function is the solution of the corresponding differential equation.

Question 3. y = cos x + C : y’ + sin x = 0
Solution:

y = cos x + C

Differentiating both sides of this equation with respect to x, we get:

y’ = \(\frac{d}{dx}\)(cos x + C) ⇒ y’ = -sin x

Substituting the value of y’ in the given differential equation, we get:

L.H.S. = y’ + sinx = – sinx + sinx = 0 = R.H.S.

Hence, the given function is the solution of the corresponding differential equation.

Question 4. \(y=\sqrt{1+x^2}: y^{\prime}=\frac{x y}{1+x^2}\)
Solution:

y = \(\sqrt{1+x^2}\)

Differentiating both sides of the equation with respect to x, we get:

⇒ \(y^{\prime}=\frac{d}{d x}\left(\sqrt{1+x^2}\right) \Rightarrow y^{\prime}=\frac{1}{2 \sqrt{1+x^2}} \cdot \frac{d}{d x}\left(1+x^2\right)\)

⇒ \(y^{\prime}=\frac{2 x}{2 \sqrt{1+x^2}}\)

⇒ \(y^{\prime}=\frac{x}{\sqrt{1+x^2}} \Rightarrow y^{\prime}=\frac{x}{1+x^2} \times \sqrt{1+x^2}\)

⇒ \(y^{\prime}=\frac{x}{1+x^2} \cdot y \Rightarrow y^{\prime}=\frac{x y}{1+x^2}\)

∴ L.H.S. = R.H.S.

Hence, the given function is the solution of the corresponding differential solution.

Question 5. y = Ax : xy’ = y (x ≠ 0)
Solution:

y = Ax

Differentiating both sides of the equation with respect to x, we get: \(y^{\prime}=\frac{d}{d x}(A x) \Rightarrow y^{\prime}=A\)

Substituting the value of y’ in the given differential equation, we get:

L.H.S. = xy’ = x-A = Ax = y = R.H.S.

Hence, the given function is the solution of the corresponding differential equation.

Question 6. \(y=x \sin x \quad: \quad x y^{\prime}=y+x \sqrt{x^2-y^2} \quad[x \neq 0 \text { and } x>y \text { or } x<-y]\)
Solution:

y = x sin x…..(1)

Differentiating both sides of this equation with respect to x, we get: \(y^{\prime}=\frac{d}{d x}(x \sin x)\)

⇒ \(y^{\prime}=\sin x \cdot \frac{d}{d x}(x)+x \cdot \frac{d}{d x}(\sin x) \Rightarrow y^{\prime}=\sin x+x \cos x\)

Substituting the value of y’ in the given differential equation, we get:

L.H.S. = x y’ = x(sin x + x cos x) = x sin x + x² cos x

= \(y+x^2 \cdot \sqrt{1-\sin ^2 x}=y+x^2 \sqrt{1-\left(\frac{y}{x}\right)^2}\) [Using Eq.(1)]

= \(y+x \sqrt{x^2-y^2}=\) R.H.S.

Hence, the given function is the solution of the corresponding differential equation

Question 7. \(x y=\log y+C: y^{\prime}=\frac{y^2}{1-x y} \quad(x y \neq 1)\)
Solution:

y – cos y = x….(1)

Differentiating both sides of the equation with respect to x, we get: \(\frac{d y}{d x}-\frac{d}{d x}(\cos y)=\frac{d}{d x}(x) \Rightarrow y^{\prime}+\sin y \cdot y^{\prime}\)=1

⇒ \(y^{\prime}(1+\sin y)=1 \Rightarrow y^{\prime}=\frac{1}{1+\sin y}\)….(2)

Substituting the value of y’ in the given differential equation, we get:

L.H.S. = \((y \sin y+\cos y+x) y^{\prime}=(y \sin y+\cos y+y-\cos y) \times \frac{1}{1+\sin y}\) [Using Eq.(1) and (2)]

= \(y(1+\sin y) \cdot \frac{1}{1+\sin y}=y=\text { R.H.S. }\)

Hence, the given function is the solution of the corresponding differential equation

Question 9. \(x+y=\tan ^{-1} y: y^2 y^{\prime}+y^2+1=0\)
Solution:

x+y = \(\tan ^{-1} y\)

Differentiating both sides of this equation with respect to x, we get:

⇒ \(\frac{d}{d x}(x+y)=\frac{d}{d x}\left(\tan ^{-1} y\right) \Rightarrow 1+y^{\prime}\)

= \(\left[\frac{1}{1+y^2}\right] y^{\prime} \Rightarrow y^{\prime}\left[\frac{1}{1+y^2}-1\right]=1\)

⇒ \(y^{\prime}\left[\frac{1-\left(1+y^2\right)}{1+y^2}\right]=1 \Rightarrow y^{\prime}\left[\frac{-y^2}{1+y^2}\right]\)=1

⇒ \(y^{\prime}=\frac{-\left(1+y^2\right)}{y^2}\)

Substituting the value of \(y^{\prime}\) in the given differential equation, \(y^2 y^1+y^2+1=0\) we get:

L.H.S. = \(y^2y^{\prime}+y^2+1=y^2\left[\frac{-\left(1+y^2\right)}{y^2}\right]+y^2+1=-1-y^2+y^2+1=0\)

Hence, the given function is the solution of the corresponding differential equation.

Question 10. y = \(\sqrt{a^2-x^2}, x \in(-a, a) \quad: x+y \frac{d y}{d x}=0(y \neq 0)\)
Solution:

y = \(\sqrt{a^2-x^2}\)….(1)

Differentiating both sides of this equation with respect to x, we get

⇒ \(\frac{d y}{d x}=\frac{d}{d x}\left(\sqrt{a^2-x^2}\right)\)

⇒ \(\frac{d y}{d x}=\frac{1}{2 \sqrt{a^2-x^2}} \cdot \frac{d}{d x}\left(a^2-x^2\right)=\frac{1}{2 \sqrt{a^2-x^2}}(-2 x)=\frac{-x}{\sqrt{a^2-x^2}}\)…(2)

Substituting the value of \(\frac{dy}{dx}\) in the given differential equation, we get

L.H.S. = \(x+y \frac{d y}{d x}=x+\sqrt{a^2-x^2} \times \frac{-x}{\sqrt{a^2-x^2}}\) (Using eq(1) and (2))

Hence, the given function is the solution of the corresponding differential equation.

Question 11. The number of arbitrary constants in the general solution of a differential equation of fourth order are:

1. 0
2. 2
3. 3
4. 4

Solution: 4. 4

We know that the number of constants in the general solution of a differential equation of order n is equal to its order.

Therefore, the number of constants in the general equation of fourth order differential equation is four.

Hence, the correct answer is (4).

Question 12. The number of arbitrary constants in the particular solution of a differential equation of third order are:

1. 3
2. 2
3. 1
4. 0

Solution: 4. 0

In a particular solution of a differential equation, there are no arbitrary constants.

Hence, the correct answer is (4).

Differential Equation Exercise 9.3

For Each Of The Differential Equations, Find The General Solution

Question 1. \(\frac{d y}{d x}=\frac{1-\cos x}{1+\cos x}\)
Solution:

The given differential equation is

⇒ \(\frac{d y}{d x}=\frac{1-\cos x}{1+\cos x} \Rightarrow \frac{d y}{d x}=\frac{2 \sin ^2 \frac{x}{2}}{2 \cos ^2 \frac{x}{2}}\)

= \(\tan ^2 \frac{x}{2} \quad \Rightarrow \frac{d y}{d x}=\left(\sec ^2 \frac{x}{2}-1\right)\)

Separating the variables, we get: \(\mathrm{dy}=\left(\sec ^2 \frac{\mathrm{x}}{2}-1\right) \mathrm{dx}\)

Now, integrating both sides of this equation, we get:

⇒ \(\int d y=\int\left(\sec ^2 \frac{x}{2}-1\right) d x=\int \sec ^2 \frac{x}{2} d x-\int d x \Rightarrow y=2 \tan \frac{x}{2}-x+C\)

This is the required general solution of the given differential equation.

Question 3. \(\frac{d y}{d x}+y=1 \quad(y \neq 1)\)
Solution:

The given differential equation is: \(\frac{d y}{d x}+y=1 \Rightarrow \frac{d y}{d x}=1-y\)

Separating the variables, we get: \(\frac{d y}{1-y}=d x\)

Now, integrating both sides of this equation, we get: \(\int \frac{d y}{1-y}=\int d x\)

⇒ \(-\log (1-y)=x+\log C \Rightarrow-\log C-\log (1-y)=x \Rightarrow \log C(1-y)=-x\)

⇒ \(C(1-y)=e^{-x} \Rightarrow 1-y=\frac{1}{C} e^{-x} \Rightarrow y=1-\frac{1}{C} e^{-x} \Rightarrow y=1+A e^{-x}\)

(where  A = \(-\frac{1}{C}\))

This is the required general solution of the given differential equation.

Question 4. sec²x tan y dx + sec²y tan x dy =0
Solution:

The given differential equation is: sec²x tan y dx + sec²y tan x dy = 0

⇒ sec²x tan y dx = -sec²y tan x dy

On separating the variables, we get:

⇒ \(\frac{\sec ^2 x}{\tan x} d x=-\frac{\sec ^2 y}{\tan y} d y\)

Integrating both sides of this equation, we get: \(\int \frac{\sec ^2 x}{\tan x} d x=-\int \frac{\sec ^2 y}{\tan y} d y\)

⇒ log |tan x| = -log |tan y| = log C (\(\int \frac{f^{\prime}(x)}{f(x)} d x=\log |f(x)|+C\))

⇒ log |tan x| + log |tan y| = log C

⇒ tan x tan y = C

This is the required general solution of the given differential equation.

Question 5. (e^x + e^-x)dy – (e^x – e^-x)dx = 0
Solution:

The given differential equation is: (e^x + e^-x)dy – (e^x – e^-x)dx = 0 ⇒ (e^x+ e^-x)dy = (e^x – e^-x)dx

Separating the variables, we get: \(d y=\left[\frac{e^x-e^{-x}}{e^x+e^{-x}}\right] d x\)

Integrating both sides of this equation, we get \(\int d y=\int\left[\frac{e^x-e^{-x}}{e^x+e^{-x}}\right] d x+C\)

⇒ y = \(\int\left[\frac{e^x-e^{-x}}{e^x+e^{-x}}\right] d x+C\)

⇒ y = \(\log \left(e^x+e^{-x}\right)+C\)

This is the required general solution of the given differential equation.

Question 6. \(\frac{d y}{d x}=\left(1+x^2\right)\left(1+y^2\right)\)
Solution:

The given differential equation is: \(\frac{d y}{d x}=\left(1+x^2\right)\left(1+y^2\right) \Rightarrow \frac{d y}{1+y^2}=\left(1+x^2\right) d x\)

Integrating both sides of this equation, we get:

⇒ \(\int \frac{d y}{1+y^2}=\int\left(1+x^2\right) d x \Rightarrow \tan ^{-1} y=\int d x+\int x^2 d x \Rightarrow \tan ^{-1} y=x+\frac{x^3}{3}+C\)

This is the required general solution of the given differential equation.

Question 7. y log y dx – x dy = 0
Solution:

The given differential equation is : y log y dx – x dy = 0 ⇒ y log y dx = x dy

Separating the variables, we get: \(\frac{d y}{y \log y}=\frac{d x}{x}\)

Integrating both sides, we get: \(\int \frac{d y}{y \log y}=\int \frac{d x}{x}\)….(1)

Let log y=t ⇒ \(\frac{1}{y} d y=d t\)

Substituting this value in equation (1), we get:

⇒ \(\int \frac{d t}{t}=\int \frac{d x}{x} \Rightarrow \log t=\log x+\log C \Rightarrow \log (\log y)=\log C x\)

⇒ \(\log y=C x \Rightarrow y=e^c\)

This is the required general solution of the given differential equation.

Question 8. \(x^5 \frac{d y}{d x}=-y^5\)
Solution:

The given differential equation is: \(x^5 \frac{d y}{d x}=-y^5\)

Separating the variables, we get: \(\frac{d y}{y^5}=-\frac{d x}{x^5} \Rightarrow \frac{d x}{x^5}+\frac{d y}{y^5}=0\)

Integrating both sides, we get: \(\int \frac{d x}{x^5}+\int \frac{d y}{y^5}=k\) where k is any constant

⇒ \(\int x^{-5} d x+\int y^{-5} d y=k \Rightarrow \frac{x^{-4}}{-4}+\frac{y^{-4}}{-4}=k \Rightarrow x^{-4}+y^{-4}=-4 k\)

⇒ \(x^{-4}+y^{-4}=C \quad(C=-4 k)\)

This is the required general solution of the given differential equation.

Question 9. \(\frac{d y}{d x}=\sin ^{-1} x\)
Solution:

The given differential equation \(\frac{d y}{d x}=\sin ^{-1} x \Rightarrow d y=\sin ^{-1} x d x\)

Integrating both sides, we get Integrating both sides, we get : \(\int d y=\int \sin ^{-1} x d x \Rightarrow y=\int\left(\sin ^{-1} x\right) \cdot 1 d x\)

⇒y = \(\sin ^{-1} x \cdot \int(1) d x-\int\left[\left(\frac{d}{d x}\left(\sin ^{-1} x\right) \cdot \int(1) d x\right)\right] d x\)

⇒ \(y=\sin ^{-1} x \cdot x-\int\left(\frac{1}{\sqrt{1-x^2}} \cdot x\right) d x\)

⇒ y = \(x \sin ^{-1} x+\int \frac{-x}{\sqrt{1-x^2}} d x\)….(1)

Let \(1-x^2=t \Rightarrow-2 x d x=d t\)

Substituting these values in equation (1), we get

y = \(x \sin ^{-1} x+\int \frac{1}{2 \sqrt{t}} d t \Rightarrow y=x \sin ^{-1} x+\frac{1}{2} \cdot \int(t)^{-\frac{1}{2}} d t\)

⇒ \(y=x \sin ^{-1} x+\frac{1}{2} \cdot \frac{t^{\frac{1}{2}}}{\frac{1}{2}}+C\)]

⇒ y = \(x \sin ^{-1} x+\sqrt{t}+C \Rightarrow y=x \sin ^{-1} x+\sqrt{1-x^2}+C\)

This is the required general solution of the given differential equation.

Question 10. e^x tan y dx + (1-e^x) sec²ydy = 0
Solution:

The given differential equation is: \(e^x \tan y d x+\left(1-e^x\right) \sec ^2 y d y=0 \Rightarrow\left(1-e^x\right) \sec ^2 y d y=-e^x \tan y d x\)

Separating the variables, we get \(\frac{\sec ^2 y}{\tan y} d y=\frac{-e^x}{1-e^x} d x\)

Integrating both sides, we get: \(\int \frac{\sec ^2 y}{\tan y} d y=\int \frac{-e^x}{1-e^x} d x\)

⇒ \(\log (\tan y)=\log \left(1-e^x\right)+\log C\) (because \(\int \frac{f^{\prime}(x)}{f(x)} d x=\log |f(x)|+C\))

⇒ \(\log (\tan y)=\log \left[C\left(1-e^x\right)\right]\)

⇒ \(\tan y=C\left(1-e^x\right)\)

This is the required general solution of the given differential equation.

For each of the differential equations, find a particular solution satisfying the given condition

Question 11. \(\left(x^3+x^2+x+1\right) \frac{d y}{d x}=2 x^2+x ; y=1\) when x=0
Solution:

The given differential equation is : \(\left(x^3+x^2+x+1\right) \frac{d y}{d x}=2 x^2+x \Rightarrow \frac{d y}{d x}=\frac{2 x^2+x}{\left(x^3+x^2+x+1\right)}\)

⇒ d y = \(\frac{2 x^2+x}{(x+1)\left(x^2+1\right)} d x\)

Integrating both sides, we get: \(\int d y=\int \frac{2 x^2+x}{(x+1)\left(x^2+1\right)} d x\)…..(1)

Let \(\frac{2 x^2+x}{(x+1)\left(x^2+1\right)}=\frac{A}{x+1}+\frac{B x+C}{x^2+1}\)……(2)

⇒ \(\frac{2 x^2+x}{(x+1)\left(x^2+1\right)}=\frac{A x^2+A+(B x+C)(x+1)}{(x+1)\left(x^2+1\right)}\)

⇒ \(2 x^2+x=A x^2+A+B x^2+B x+C x+C\)

⇒ \(2 x^2+x=(A+B) x^2+(B+C) x+(A+C)\)

Comparing the coefficients of x² and x, we get:

A + B = 2, B + C = 1,A + C = 0

Solving these equations, we get: A = 1/2, B = 3/2 and C = -1/2

Substituting the values of A, B, and C in equation (2), we get:

⇒ \(\frac{2 x^2+x}{(x+1)\left(x^2+1\right)}=\frac{1}{2} \cdot \frac{1}{(x+1)}+\frac{1}{2} \frac{(3 x-1)}{\left(x^2+1\right)}\)

Therefore, equation (1) becomes:

⇒ \(\int d y=\frac{1}{2} \int \frac{1}{x+1} d x+\frac{1}{2} \int \frac{3 x-1}{x^2+1} d x\)

⇒ \(y=\frac{1}{2} \log (x+1)+\frac{3}{2} \int \frac{x}{x^2+1} d x-\frac{1}{2} \int \frac{1}{x^2+1} d x\)

⇒ \(y=\frac{1}{2} \log (x+1)+\frac{3}{4} \cdot \int \frac{2 x}{x^2+1} d x-\frac{1}{2} \tan ^{-1} x+C\)

⇒ \(y=\frac{1}{2} \log (x+1)+\frac{3}{4} \log \left(x^2+1\right)-\frac{1}{2} \tan ^{-1} x+C \)

⇒ \(y=\frac{1}{4}\left[2 \log (x+1)+3 \log \left(x^2+1\right)\right]-\frac{1}{2} \tan ^{-1} x+C\)

⇒ \(y=\frac{1}{4}\left[\log (x+1)^2\left(x^2+1\right)^3\right]-\frac{1}{2} \tan ^{-1} x+C\)…(3)

Now, y=1 when x=0

⇒ \(1=\frac{1}{4} \log (1)-\frac{1}{2} \tan ^{-1} 0+C \Rightarrow 1=\frac{1}{4} \times 0-\frac{1}{2} \times 0+C \Rightarrow C=1\)

Substituting C=1 in equation (3), we get:

y = \(\frac{1}{4}\left[\log (x+1)^2\left(x^2+1\right)^3\right]-\frac{1}{2} \tan ^{-1} x+1\)

which is the required particular solution.

Question 12. \(x\left(x^2-1\right) \frac{d y}{d x}=1 ; y=0\) when x=2
Solution:

⇒ \(x\left(x^2-1\right) \frac{d y}{d x}=1\)

On separating the variables, we get : \(d y=\frac{d x}{x\left(x^2-1\right)} \Rightarrow \int d y=\int \frac{d x}{x^3\left(1-\frac{1}{x^2}\right)}\)

Put \(1-\frac{1}{x^2}=t \Rightarrow \frac{2}{x^3} d x=d t\),

⇒\(\int \mathrm{dy}=\frac{1}{2} \int \frac{\mathrm{dt}}{\mathrm{t}} \Rightarrow \mathrm{y}=\frac{1}{2} \log (\mathrm{t})+\log \mathrm{C}\)

⇒ \(\mathrm{y}=\frac{1}{2} \log \left|\frac{\mathrm{x}^2-1}{\mathrm{x}^2}\right|+\log \mathrm{C}\)…(1)

Now, y = 0 when x = 2

0 = \(\frac{1}{2} \log \left(\frac{3}{4}\right)+\log \mathrm{C}\) (From eq.(1))

(\(\log \mathrm{C}=-\frac{1}{2} \log \left(\frac{3}{4}\right)\))

log \(\mathrm{C}=\frac{1}{2} \log \left(\frac{4}{3}\right)\)

⇒ \(\mathrm{y}=\frac{1}{2} \log \left(\frac{\mathrm{x}^2-1}{\mathrm{x}^2}\right)+\frac{1}{2} \log \left(\frac{4}{3}\right)\)

⇒ \(\mathrm{y}=\frac{1}{2} \log \left[\frac{4\left(\mathrm{x}^2-1\right)}{3 \mathrm{x}^2}\right]\)

which is the required particular solution.

Question 13. \(\cos \left(\frac{d y}{d x}\right)=a(a \in R) ; y=1\) when x=0
Solution:

cos \(\left(\frac{d y}{d x}\right)=a \Rightarrow \frac{d y}{d x}=\cos ^{-1} a\)

On separating the variables, we get, dy = cos^-1 a dx

Integrating both sides, we get: ∫dy = cos^-1 a ∫dx

⇒ y = cos^-1 a x + C ⇒ y = x cos^-1 a + C….(1)

Now, y = 1 when x = 0 ⇒ 1 = 0 · cos^-1 a + C ⇒ C = 1

Substituting C= 1 in equation (1), we get:

y = \(x \cos ^{-1} a+1 \Rightarrow \frac{y-1}{x}=\cos ^{-1} a \Rightarrow \cos \left(\frac{y-1}{x}\right)=a\)

which is the required particular solution.

Question 14. \(\frac{dy}{dx}\)= y tan x ; y = 1 when x = 0
Solution:

⇒ \(\frac{dy}{dx}\)= y tan x

Separating the variables, we get: \(\frac{dy}{dx}\) = tan x dx

Integrating both sides, we get: ∫\(\frac{dy}{dx}\) = ∫tan x dx

⇒ log y = log |sec x| + log C

⇒ log y = log(C sec x) ⇒ y C sec x……(1)

Now, y = 1 when x = 0 ⇒ 1= C x sec0 ⇒ 1 = C x 1 ⇒ C = 1

Substituting C = 1 in equation (1), we get y = sec x which is the required particular solution.

Question 15. Find the equation of a curve passing through the point (0, 0) and whose differential equation is y’ = e^x sin x
Solution:

The differential equation of the curve is: y’ = e^x sin x

⇒ \(\frac{dy}{dx}\) = e^x sin x

Separating the variables, we get: dy = e^x sin x dx

Integrating both sides, we get: J∫dy =∫e^x sin x dx….(1)

Let I = \(\int{e^x} \sin x d x\)

⇒ \(I=\sin x \int e^x d x-\int\left(\frac{d}{d x}(\sin x) \cdot \int e^x d x\right) d x \Rightarrow I=\sin x \cdot e^x-\int \cos x \cdot e^x d x\)

⇒ \(I=\sin x \cdot e^x-\left[\cos x \cdot \int e^x d x-\int\left(\frac{d}{d x}(\cos x) \cdot \int e^x d x\right) d x\right]\)

⇒ \(I=\sin x e^x-\left[\cos x \cdot e^x-\int(-\sin x) \cdot e^x d x\right] \Rightarrow I=e^x \sin x-e^x \cos x-I\)

⇒ \(2 I=e^x(\sin x-\cos x) \Rightarrow I=\frac{e^x(\sin x-\cos x)}{2}\)

Substituting this value in equation (1), we get:

y = \(\frac{e^x(\sin x-\cos x)}{2}+C\)…(2)

Now, the curve passes through point (0,0).

∴ \(0=\frac{\mathrm{e}^0(\sin 0-\cos 0)}{2}+\mathrm{C} \Rightarrow 0=\frac{1(0-1)}{2}+\mathrm{C} \Rightarrow \mathrm{C}=\frac{1}{2}\)

Substituting C = \(\frac{1}{2}\) in equation (2), we get:

y = \(=\frac{\mathrm{e}^{\mathrm{x}}(\sin \mathrm{x}-\cos \mathrm{x})}{2}+\frac{1}{2}\)

⇒ \(2 \mathrm{y}=\mathrm{e}^{\mathrm{x}}(\sin \mathrm{x}-\cos \mathrm{x})+1 \Rightarrow 2 \mathrm{y}-1=\mathrm{e}^{\mathrm{x}}(\sin \mathrm{x}-\cos \mathrm{x})\)

Hence, the required equation of the curve is \(2 y-1=e^x(\sin x-\cos x)\)

Question 16. For the differential equation xy\(\frac{dy}{dx}\) = (x + 2)(y + 2). find the solution curve passing through the point (1,-1).
Solution:

The differential equation of the given curve is: xy \(\frac{dy}{dx}\)= (x + 2)(y + 2)

Separating the variables, we get: \(\left(\frac{y}{y+2}\right) d y=\left(\frac{x+2}{x}\right) d x \Rightarrow\left(1-\frac{2}{y+2}\right) d y=\left(1+\frac{2}{x}\right) d x\)

Integrating both sides, we get:

⇒ \(\int\left(1-\frac{2}{y+2}\right) d y=\int\left(1+\frac{2}{x}\right) d x \Rightarrow \int d y-2 \int \frac{1}{y+2} d y=\int d x+2 \int \frac{1}{x} d x\)

⇒ \( y-2 \log (y+2)=x+2 \log x+C \Rightarrow y-x-C=\log x^2+\log (y+2)^2\)

⇒ \(y-x-C=\log \left[x^2(y+2)^2\right]\)…(1)

Now, the curve passes through point (1,-1).

⇒ \(-1-1-\mathrm{C}=\log \left[(1)^2(-1+2)^2\right] \Rightarrow-2-\mathrm{C}=\log 1=0 \Rightarrow \mathrm{C}=-2\)

Substituting C=-2 in equation (1), we get : \(y-x+2=\log \left[x^2(y+2)^2\right]\)

This is the required solution of the given curve.

Question 17. Find the equation of a curve passing through the point (0, -2) given that at any point (x, y) on the curve, the product of the slope of its tangent and y-coordinate of the point is equal to the x-coordinate of the point.
Solution:

Let x and y be the x-coordinate and y-coordinate of the curve respectively.

We know that the slope of a tangent to the curve in the coordinate axis is given by the relation, \(\frac{dy}{dx}\)

According to the given information, we get:

The product of the slope of a tangent with y-coordinate = x-coordinate

y · \(\frac{dy}{dx}\) = x

Separating the variables, we get; y dy = x dx

Integrating both sides, we get: \(\int y d y=\int x d x \Rightarrow \frac{y^2}{2}=\frac{x^2}{2}+C \Rightarrow y^2-x^2=2 C\)….(1)

Now, the curve passes through the point (0, -2).

∴ (-2)² – 0² = 2C ⇒ 2C = 4

Substituting 2C = 4 in equation (1), we get: y² – x² = 4

This is the required equation of the curve.

Question 18. At any point (x, y) of a curve, the slope of the tangent is twice the slope of the line segment joining the point of contact to the point (-4, -3). Find the equation of the curve given that it passes through (-2, 1).
Solution:

It is given that (x, y) is the point of contact of the curve and its tangent.

The slope (m₁) of the line segment joining (x, y) and (-4, -3) is \(\frac{y-(-3)}{x-(-4)}=\frac{y+3}{x+4}\)

We know that the slope of the tangent to the curve is given by the relation, \(\frac{dy}{dx}\)

∴ Slope (m₂) of the tangent = \(\frac{dy}{dx}\)

According to the given information : \(\mathrm{m}_2=2 \mathrm{~m}_1 \Rightarrow \frac{\mathrm{dy}}{\mathrm{dx}}=\frac{2(\mathrm{y}+3)}{\mathrm{x}+4}\)

Separating the variables, we get: \(\frac{d y}{y+3}=\frac{2 d x}{x+4}\)

Integrating both sides, we get: \(\int \frac{d y}{y+3}=2 \int \frac{d x}{x+4} \Rightarrow \log (y+3)=2 \log (x+4)+\log C\)

⇒ log(y + 3) = log C (x + 4)² ⇒ y + 3 = C(x + 4)²….(1)

This is the general equation of the curve.

It is given that it passes through the point (-2, 1).

⇒ 1 + 3 = C(-2 + 4)² ⇒ 4 = 4C ⇒ C = 1

Substituting C = 1 in equation (1), we get; y + 3 = (x + 4)²

This is the required equation of the curve.

Question 19. The volume of a spherical balloon being inflated changes at a constant rate. If initially its radius is 3 units and after 3 seconds it is 6 units. Find the radius of the balloon after t seconds.
Solution:

Let the rate of change of the volume of the balloon be k (where k is a constant).

⇒ \(\frac{\mathrm{dv}}{\mathrm{dt}}=\mathrm{k} \Rightarrow \frac{\mathrm{d}}{\mathrm{dt}}\left(\frac{4}{3} \pi \mathrm{r}^3\right)=\mathrm{k}\)

(Volume of sphere = \(\frac{4}{3} \pi \mathrm{r}^3\))

⇒ \(\frac{4}{3} \pi \cdot 3 \mathrm{r}^2 \cdot \frac{\mathrm{dr}}{\mathrm{dt}}=\mathrm{k} \Rightarrow 4 \pi \mathrm{r}^2 \mathrm{dr}=\mathrm{kdt}\)

Integrating both sides, we get: \(4 \pi \int r^2 d r=k \int d t \Rightarrow 4 \pi \cdot \frac{r^3}{3}=k t+C \Rightarrow 4 \pi r^3=3(k t+C)\)….(1)

Now, at t=0, r=3

⇒ \(4 \pi \times 3^3=3(\mathrm{k} \times 0+\mathrm{C}) \Rightarrow 108 \pi=3 \mathrm{C} \Rightarrow \mathrm{C}=36 \pi\)

At t=3, r=6

⇒ \(4 \pi \times 6^3=3(\mathrm{k} \times 3+\mathrm{C}) \Rightarrow 864 \pi=3(3 \mathrm{k}+36 \pi) \Rightarrow 3 \mathrm{k}=288 \pi-36 \pi=252 \pi\)

⇒ k=84 \(\pi\)

Substituting the values of k and C in equation (1), we get:

⇒ \(4 \pi r^3=3[84 \pi t+36 \pi] \Rightarrow 4 \pi r^3=4 \pi[63 t+27] \Rightarrow r^3=63 t+27 \Rightarrow r=(63 t+27)^{\frac{1}{3}}\)

Thus, the radius of the balloon after t seconds is \((63 t+27)^{\frac{1}{3}}\).

Question 20. In a bank, the principal increases continuously at the rate of r% per year. Find the value of r if Rs 100 doubles itself in 10 years (log_e2 = 0.6931).
Solution:

Let p, t, and r represent the principal, time, and rate of interest respectively.

It is given that the principal increases continuously at the rate of r% per year.

⇒ \(\frac{d p}{d t}=\left(\frac{r}{100}\right) p \Rightarrow \frac{d p}{p}=\left(\frac{r}{100}\right) d t\)

Integrating both sides, we get:

⇒ \(\int \frac{d p}{p}=\frac{r}{100} \int d t \Rightarrow \log p=\frac{r t}{100}+k \Rightarrow p=e^{\frac{rt}{100}+k}\)….(1)

It is given that when \(\mathrm{t}=0, \mathrm{p}=100. \Rightarrow 100=\mathrm{e}^{\mathrm{k}}\)…..(2)

Now, if t=10, then p=2 x 100=200.

Therefore, equation (1) becomes :

200 = \(e^{\frac{\mathrm{t}}{10}+\mathrm{k}} \Rightarrow 200=\mathrm{e}^{\frac{\mathrm{r}}{10}} \cdot \mathrm{e}^{\mathrm{k}} \Rightarrow 200=\mathrm{e}^{\frac{\mathrm{t}}{11}} \cdot 100\)(From (2)

⇒ \(\mathrm{e}^{\frac{r}{10}}=2 \Rightarrow \frac{r}{10}=\log _4 2 \Rightarrow \frac{r}{10}=0.6931 \Rightarrow r=6.931\)

Hence, the value of r is 6.93%.

Question 21. In a bank, the principal increases continuously at the rate of 5% per year. An amount of Rs 1000 is deposited with this bank, how much will it be worth after 10 years (e^0.5 = 1.648)?
Solution:

Let p and t be the principal and time respectively.

It is given that the principal increases continuously at the rate of 5% per year.

⇒ \(\frac{d p}{d t}=\left(\frac{5}{100}\right) p \Rightarrow \frac{d p}{d t}=\frac{p}{20}\)

Separating the variables, we get: \(\frac{d p}{d t}=\frac{dt}{20}\)

Integrating both sides, we get: \(\int \frac{d p}{p}=\frac{1}{20} \int d t \Rightarrow \log p=\frac{t}{20}+C \Rightarrow p=e^{\frac{t}{20}+C}\)…….(1)

Now, when t = 0, p = 1000.

⇒ 1000 = e^c

At t = 10, equation (1) becomes p=e^{1/2+ C} ⇒ p = e^0.5 x e^C ⇒ p = 1.648 x 1000 (from (2))

⇒ p = 1648

Hence, after 10 years the amount will be worth Rs 1648.

Question 22. In a culture, the bacteria count is 1,00,000. The number is increased by 10% in 2 hours. In how many hours will the count reach 2,00.000, if the rate of growth of bacteria is proportional to the number present?
Solution:

Let y be the number of bacteria at any instant t.

It is given that the rate of growth of the bacteria is proportional to the number present.

∴ \(\frac{d y}{d t} \propto y \Rightarrow \frac{d y}{d t}=k y\) (where k is constant)

Separating the variables, we get: \(\frac{d y}{d t}\) = k dt

Integrating both sides, we get: \(\frac{d y}{d t}\) = k dt ⇒ log y = kt + C……(1)

Let y₀ be the number of bacteria at t = 0. ⇒ log y₀ = C

Substituting the value of C in equation (1), we get:

log y = \(k t+\log y_0 \Rightarrow \log y-\log y_0=k t\)

⇒ \(\log \left(\frac{y}{y_0}\right)=k t\)…..(2)

Also, it is given that the number of bacteria increases by 10 % in 2 hours.

⇒ \(y=\frac{110}{100} y_0 \Rightarrow \frac{y}{y_0}=\frac{11}{10}\)….(3)

Substituting this value from equation (3) in the equation

⇒ \(\mathrm{k} \cdot 2=\log \left(\frac{11}{10}\right) \Rightarrow \mathrm{k}=\frac{1}{2} \log \left(\frac{11}{10}\right)\)

Therefore, equation (2) becomes:

⇒ \(\frac{1}{2} \log \left(\frac{11}{10}\right) \cdot t=\log \left(\frac{y}{y_0}\right) \Rightarrow t=\frac{2 \log \left(\frac{y}{y_0}\right)}{\log \left(\frac{11}{10}\right)}\)…..(4)

Now, let the time when the number of bacteria increases from 100000 to 200000 be t.

⇒ \(\mathrm{y}=2 \mathrm{y}_0\) at \(\mathrm{t}=\mathrm{t}_1\)

From equation (4), we get \(t_1=\frac{2 \log \left(\frac{y}{y_0}\right)}{\log \left(\frac{11}{10}\right)}=\frac{2 \log 2}{\log \left(\frac{11}{10}\right)}\).

Hence, in \(\frac{2 \log 2}{\log \left(\frac{11}{10}\right)}\) hours the number of bacteria increases from 100000 to 200000.

Question 23. The general solution of the differential equation \(\frac{dy}{dt}\) = e^x+y is

1. \(e^x+e^{-y}=C\)
2. \(e^x+e^y=C\)
3. \(\mathrm{e}^{-x}+\mathrm{e}^y=\mathrm{C}\)
4. \(\mathrm{e}^{-x}+\mathrm{e}^{-7}=\mathrm{C}\)

Solution: 1. \(e^x+e^{-y}=C\)

Given, \(\frac{d y}{d x}=e^{x+y}=e^x \cdot e^y\)

Separating the variables, we get : \(\frac{d y}{e^y}=e^x d x \Rightarrow e^{-y} d y=e^x d x\)

Integrating both sides, we get:

⇒ \(\int \mathrm{e}^{-y} \mathrm{dy}=\int \mathrm{e}^{\mathrm{x}} \mathrm{dx} \Rightarrow-\mathrm{e}^{-\mathrm{y}}=\mathrm{e}^{\mathrm{x}}+\mathrm{k} \Rightarrow \mathrm{e}^{\mathrm{x}}+\mathrm{e}^{-\mathrm{y}}=-\mathrm{k} \Rightarrow \mathrm{e}^x+\mathrm{e}^{-\mathrm{y}}=\mathrm{C} \quad(\mathrm{C}=-\mathrm{k})\)

Hence, the correct answer is 1.

Differential Equations Exercise 9.4

Show That The Given Differential Equation Is Homogeneous And Solve Each Of Them.

Question 1. (x² + xy)dy = (x² + y²)dx
Solution:

The given differential equation i.e., (x² + xy)dy = (x² + y²)dx can be written as:

⇒ \(\frac{d y}{d x}=\frac{x^2+y^2}{x^2+x y}\)….(1)

Let F(x, y) = \(\frac{x^2+y^2}{x^2+x y}\)

Now, \(F(\lambda x, \lambda y)=\frac{(\lambda x)^2+\left(\lambda y^2\right)}{(\lambda x)^2+(\lambda x)(\lambda y)}=\frac{\lambda^2\left(x^2+y^2\right)}{\lambda^2\left(x^2+x y\right)}=\lambda^0 \cdot F(x, y)\)

This shows that equation (1) is a homogeneous equation.

To solve it, we make the substitution as y = vx

Differentiating both sides with respect to x. we get: \(\frac{dy}{dx}\) = v + x \(\frac{dv}{dx}\)

Substituting the values of y and \(\frac{dv}{dx}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{x^2+(v x)^2}{x^2+x(v x)} \Rightarrow v+x \frac{d v}{d x}=\frac{1+v^2}{1+v}\)

⇒ \(x \frac{d v}{d x}=\frac{1+v^2}{1+v}-v=\frac{\left(1+v^2\right)-v(1+v)}{1+v} \Rightarrow x \frac{d v}{d x}=\frac{1-v}{1+v}\)

⇒ \(\left(\frac{1+v}{1-v}\right) d v=\frac{d x}{x} \Rightarrow\left(\frac{2-1+v}{1-v}\right) d v=\frac{d x}{x} \Rightarrow\left(\frac{2}{1-v}-1\right) d v=\frac{d x}{x}\)

Integrating both sides, we get:-2 log (1-v)-v = log x-log k

⇒ v = -2 log (1-v)-log x+log k

⇒ v = \(\log \left[\frac{k}{x(1-v)^2}\right] \Rightarrow \frac{y}{x}=\log \left[\frac{k}{x\left(1-\frac{y}{x}\right)^2}\right] \Rightarrow \frac{y}{x}=\log \left[\frac{k x}{(x-y)^2}\right]\)

⇒ \(\frac{k x}{(x-y)^2}=e^{\frac{y}{x}} \Rightarrow(x-y)^2=k x e^{-\frac{x}{x}}\)

This is the required solution of the given differential equation.

Question 2. \(y^{\prime}=\frac{x+y}{x}\)
Solution:

The given differential equation is: \(y^{\prime}=\frac{x+y}{x} \Rightarrow \frac{d y}{d x}=\frac{x+y}{x}\)….(1)

Let F(x, y)= \(\frac{x+y}{x}\)

Now, \(\mathrm{F}(\lambda \mathrm{x}, \lambda \mathrm{y})=\frac{\lambda \mathrm{x}+\lambda \mathrm{y}}{\lambda \mathrm{x}}=\frac{\lambda(\mathrm{x}+\mathrm{y})}{\lambda \mathrm{x}}=\lambda^0 \mathrm{~F}(\mathrm{x}, \mathrm{y})\)

Thus, the given equation is a homogeneous equation.

To solve it, we make the substitution as y = vx

Substituting the values of y and \(\frac{dy}{dx}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{x+v x}{x} \Rightarrow v+x \frac{d v}{d x}=1+v \Rightarrow x \frac{d v}{d x}=1 \Rightarrow \int d v=\int \frac{d x}{x}\)

Integrating both sides, we get:

v = \(\log x+C \Rightarrow \frac{y}{x}=\log x+C \Rightarrow y=x \log x+C x\)

This is the required solution of the given differential equation.

Question 3. (x – y) dy – (x + y)dx = 0
Solution:

The given differential equation is : (x – y) dy – (x + y)dx = 0

⇒ \(\frac{d y}{d x}=\frac{x+y}{x-y}\)…..(1)

Let F(x, y)= \(\frac{x+y}{x-y}\)

∴ \(\mathrm{F}(\lambda \mathrm{x}, \lambda \mathrm{y})=\frac{\lambda \mathrm{x}+\lambda \mathrm{y}}{\lambda \mathrm{x}-\lambda \mathrm{y}}=\frac{\lambda(\mathrm{x}+\mathrm{y})}{\lambda(\mathrm{x}-\mathrm{y})}=\lambda^0 \cdot \mathrm{F}(\mathrm{x}, \mathrm{y})\)

Thus, the given differential equation is a homogeneous equation.

To solve it, we make the substitution as : \(\mathrm{y}=\mathrm{vx}\)

⇒ \(\frac{d}{d x}(y)=\frac{d}{d x}(v x) \Rightarrow \frac{d y}{d x}=v+x \frac{d y}{d x}\)

Substituting the values of y and \(\frac{d y}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{x+v x}{x-v x}=\frac{1+v}{1-v} \Rightarrow x \frac{d v}{d x}=\frac{1+v}{1-v}-v=\frac{1+v-v(1-v)}{1-v}\)

x \(\frac{d v}{d x}=\frac{1+v^2}{1-v} \Rightarrow \int \frac{1-v}{\left(1+v^2\right)} d v=\int \frac{d x}{x} \Rightarrow \int\left(\frac{1}{1+v^2}-\frac{v}{1+v^2}\right) d v\)

= \(\int \frac{d x}{x}\)

⇒ tan \(^{-1} v-\frac{1}{2} \log \left(1+v^2\right)=\log x+C \Rightarrow \tan ^{-1}\left(\frac{y}{x}\right)-\frac{1}{2} \log \left[1+\left(\frac{y}{x}\right)^2\right]=\log x+C\)

⇒ \(\tan ^{-1}\left(\frac{y}{x}\right)-\frac{1}{2} \log \left(\frac{x^2+y^2}{x^2}\right)=\log x+C\)

⇒ \(\tan ^{-1}\left(\frac{y}{x}\right)-\frac{1}{2}\left[\log \left(x^2+y^2\right)-\log x^2\right]=\log x+C\)

⇒ \(\tan ^{-1}\left(\frac{y}{x}\right)=\frac{1}{2} \log \left(x^2+y^2\right)+C\)

This is the required solution of the given differential equation.

Question 4. (x² – y²)dx + 2xy dy = 0
Solution:

The given differential equation is

⇒ \(\left(x^2-y^2\right) d x+2 x y d y=0 \Rightarrow \frac{d y}{d x}=\frac{-\left(x^2-y^2\right)}{2 x y}\)…..(1)

Let F(x, y) = \(\frac{-\left(x^2-y^2\right)}{2 x y}\)

∴ \(F(\lambda x, \lambda y)=-\left[\frac{(\lambda x)^2-(\lambda y)^2}{2(\lambda x)(\lambda y)}\right]=\frac{-\lambda^2\left(x^2-y^2\right)}{\lambda^2(2 x y)}=\lambda^0 \cdot F(x, y)\)

Therefore, the given differential equation is a homogeneous equation.

To solve it, we make the substitution as:

y = \(v x \Rightarrow \frac{d}{d x}(y)=\frac{d}{d x}(v x) \Rightarrow \frac{d y}{d x}=v+x \frac{d v}{d x}\)

Substituting the values of y and \(\frac{d y}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=-\left[\frac{x^2-(v x)^2}{2 x \cdot(v x)}\right] \Rightarrow v+x \frac{d v}{d x}=\frac{v^2-1}{2 v}\)

⇒ \(x \frac{d v}{d x}=\frac{v^2-1}{2 v}-v=\frac{v^2-1-2 v^2}{2 v} \Rightarrow x \frac{d v}{d x}=-\frac{\left(1+v^2\right)}{2 v}\)

⇒ \(\frac{2 v}{1+v^2} d v=-\frac{d x}{x}\)

⇒ \(\int \frac{2 v}{1+v^2} d v=-\int \frac{d x}{x}\)

log \(\left(1+v^2\right)=-\log x+\log C=\log \frac{C}{x} \Rightarrow 1+v^2=\frac{C}{x} \Rightarrow\left[1+\frac{y^2}{x^2}\right]\)

= \(\frac{C}{x} \Rightarrow x^2+y^2=C x\)

This is the required solution of the given differential equation.

Question 5. \(x^2 \frac{d y}{d x}=x^2-2 y^2+x y\)
Solution:

The given differential equation is : \(x^2 \frac{d y}{d x}=x^2-2 y^2+x y\)

⇒ \(\frac{d y}{d x}=\frac{x^2-2 y^2+x y}{x^2}\)….(1)

Let F(x, y) = \(\frac{x^2-2 y^2+x y}{x^2}\)

∴ \(F(\lambda x, \lambda y)=\frac{(\lambda x)^2-2(\lambda y)^2+(\lambda x)(\lambda y)}{(\lambda x)^2}=\frac{\lambda^2\left(x^2-2 y^2+x y\right)}{\lambda^2\left(x^2\right)}=\lambda^0 \cdot F(x, y)\)

Therefore, the given differential equation is a homogeneous equation.

To solve it, we make the substitution as:

y = \(v x \Rightarrow \frac{d y}{d x}=v+x \frac{d v}{d x}\)

Substituting the values of y and \(\frac{d y}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{x^2-2(v x)^2+x \cdot(v x)}{x^2}\)

⇒ \(v+x \frac{d v}{d x}=1-2 v^2+v \Rightarrow x \frac{d v}{d x}=1-2 v^2\)

⇒ \(\frac{d v}{1-2 v^2}=\frac{d x}{x} \Rightarrow \frac{1}{2} \int \frac{d v}{\frac{1}{2}-v^2}=\int \frac{d x}{x}\)

⇒ \(\frac{1}{2} \cdot \int\left[\frac{\mathrm{dv}}{\left(\frac{1}{\sqrt{2}}\right)^2-v^2}\right]=\int \frac{\mathrm{dx}}{\mathrm{x}}\)

⇒ \(\frac{1}{2} \cdot \frac{1}{2 \times \frac{1}{\sqrt{2}}} \log \left|\frac{\frac{1}{\sqrt{2}}+\mathrm{v}}{\frac{1}{\sqrt{2}}-\mathrm{v}}\right|=\log |\mathrm{x}|+\mathrm{C}\)

(because \(\int \frac{d x}{a^2-x^2}=\frac{1}{2 a} \log \left|\frac{a+x}{a-x}\right|+C\))

⇒ \(\frac{1}{2 \sqrt{2}} \log \left|\frac{\frac{1}{\sqrt{2}}+\frac{y}{x}}{\frac{1}{\sqrt{2}}-\frac{y}{x}}\right|=\log |x|+C \Rightarrow \frac{1}{2 \sqrt{2}} \log \left|\frac{x+\sqrt{2} y}{x-\sqrt{2} y}\right|=\log |x|+C \)

This is the required solution for the given differential equation.

Question 6. \(x d y-y d x=\sqrt{x^2+y^2} d x\)
Solution:

⇒ \(x d y-y d x=\sqrt{x^2+y^2} d x \Rightarrow x d y=\left[y+\sqrt{x^2+y^2}\right] d x\)

⇒ \(\frac{d y}{d x}=\frac{y+\sqrt{x^2+y^2}}{x}\)….(1)

Let F(x, y) = \(\frac{y+\sqrt{x^2+y^2}}{x}\)

∴ \(F(\lambda x, \lambda y)=\frac{\lambda x+\sqrt{(\lambda x)^2+(\lambda y)^2}}{\lambda x}=\frac{\lambda\left(y+\sqrt{x^2+y^2}\right)}{\lambda x}=\lambda^0 \cdot F(x, y)\)

Therefore, the given differential equation is a homogeneous equation.

To solve it, we make the substitution as y = vx

⇒ \(\frac{d}{d x}(y)=\frac{d}{d x}(v x) \Rightarrow \frac{d y}{d x}=v+x \frac{d v}{d x}\)

Substituting the values of y and \(\frac{d y}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{v x+\sqrt{x^2+(v x)^2}}{x} \Rightarrow v+x \frac{d v}{d x}=v+\sqrt{1+v^2}\)

⇒ \(\frac{d v}{\sqrt{1+v^2}}=\frac{d x}{x} \Rightarrow \int \frac{d v}{\sqrt{1+v^2}}=\int \frac{d x}{x}\)

⇒ \(\log \left|v+\sqrt{1+v^2}\right|=\log |x|+\log C \Rightarrow \log \left|\frac{y}{x}+\sqrt{1+\frac{y^2}{x^2}}\right|=\log |C x|\)

⇒ \(\log \left|\frac{y+\sqrt{x^2+y^2}}{x}\right|=\log |C x| \Rightarrow y+\sqrt{x^2+y^2}=C x^2\)

This is the required solution of the given differential equation.

Question 7. \(\left\{x \cos \left(\frac{y}{x}\right)+y \sin \left(\frac{y}{x}\right)\right\} y d x=\left\{y \sin \left(\frac{y}{x}\right)-x \cos \left(\frac{y}{x}\right)\right\} x d y\)
Solution:

The given differential equation is:

⇒ \(\left\{x \cos \left(\frac{y}{x}\right)+y \sin \left(\frac{y}{x}\right)\right\} y d x=\left\{y \sin \left(\frac{y}{x}\right)-x \cos \left(\frac{y}{x}\right)\right\} x d y\)

⇒ \(\frac{d y}{d x}=\frac{\left\{x \cos \left(\frac{y}{x}\right)+y \sin \left(\frac{y}{x}\right)\right\} y}{\left\{y \sin \left(\frac{y}{x}\right)-x \cos \left(\frac{y}{x}\right)\right\} x}\)….(1)

Let F(x, y) = \(\frac{\left\{x \cos \left(\frac{y}{x}\right)+y \sin \left(\frac{y}{x}\right)\right\} y}{\left\{y \sin \left(\frac{y}{x}\right)-x \cos \left(\frac{y}{x}\right)\right\} x}\)

⇒ \(\mathrm{F}(\lambda \mathrm{x}, \lambda \mathrm{y})=\frac{\left\{\lambda x \cos \left(\frac{\lambda y}{\lambda x}\right)+\lambda y \sin \left(\frac{\lambda y}{\lambda x}\right)\right\} \lambda y}{\left\{\lambda y \sin \left(\frac{\lambda y}{\lambda x}\right)-\lambda x \cos \left(\frac{\lambda y}{\lambda x}\right)\right\} \lambda x}\)

= \(\frac{\lambda^2\left\{x \cos \left(\frac{y}{x}\right)+y \sin \left(\frac{y}{x}\right)\right\} y}{\lambda^2\left\{y \sin \left(\frac{y}{x}\right)-x \cos \left(\frac{y}{x}\right)\right\} x}=\lambda^0 \cdot F(x, y)\)

Therefore, the given differential equation is a homogeneous equation.

To solve it, we make the substitution as:

y = \(v x \Rightarrow \frac{d y}{d x}=v+x \times \frac{d v}{d x}\)

Substituting the values of y and \(\frac{dy}{dx}\) in equation {1), we get:

v+x \(\frac{d v}{d x}=\frac{(x \cos v+v x \sin v) \cdot v x}{(v x \sin v-x \cos v) \cdot x} \Rightarrow v+x \frac{d v}{d x}=\frac{v \cos v+v^2 \sin v}{v \sin v-\cos v}\)

⇒ \(x \frac{d v}{d x}=\frac{v \cos v+v^2 \sin v}{v \sin v-\cos v}-v\)

⇒ \(x \frac{d v}{d x}=\frac{v \cos v+v^2 \sin v-v^2 \sin v+v \cos v}{v \sin v-\cos v}\)

⇒ \(x \frac{d v}{d x}=\frac{2 v \cos v}{v \sin v-\cos v} \Rightarrow\left[\frac{v \sin v-\cos v}{v \cos v}\right] d v=\frac{2 d x}{x}\)

⇒ \(\left(\tan v-\frac{1}{v}\right) d v=\frac{2 d x}{x}\)

Integrating both sides, we get : \(\int\left(\tan v-\frac{1}{v}\right) d v=2 \int \frac{d x}{x}\)

log (sec v)-log v=2 log x+log C

⇒ \(\log \left(\frac{\sec v}{v}\right)=\log \left(C x^2\right) \Rightarrow\left(\frac{\sec v}{v}\right)=C^2 \Rightarrow \sec v=C^2 v\)

⇒ \(\sec \left(\frac{y}{x}\right)=C \cdot x^2 \cdot \frac{y}{x} \Rightarrow \sec \left(\frac{y}{x}\right)=C x y \Rightarrow \cos \left(\frac{y}{x}\right)=\frac{1}{C x y}=\frac{1}{C} \cdot \frac{1}{x y}\)

⇒ \(x y \cos \left(\frac{y}{x}\right)=k \quad\left(k=\frac{1}{C}\right)\)

This is the required solution of the given differential equation.

Question 8. \(x \frac{d y}{d x}-y+x \sin \left(\frac{y}{x}\right)=0\)
Solution:

x \(\frac{d y}{d x}-y+x \sin \left(\frac{y}{x}\right)=0 \Rightarrow x \frac{d y}{d x}=y-x \sin \left(\frac{y}{x}\right)\)

⇒ \(\frac{d y}{d x}=\frac{y-x \sin \left(\frac{y}{x}\right)}{x}\)…..(1)

Let F(x, y) = \(\frac{y-x \sin \left(\frac{y}{x}\right)}{x}\)

⇒ \(\mathrm{F}(\lambda \mathrm{x}, \lambda \mathrm{y})=\frac{\lambda \mathrm{y}-\lambda \mathrm{x} \sin \left(\frac{\lambda \mathrm{y}}{\lambda \mathrm{x}}\right)}{\lambda \mathrm{x}}=\frac{\lambda\left[\mathrm{y}-\mathrm{x} \sin \left(\frac{\mathrm{y}}{\mathrm{x}}\right)\right]}{\lambda \mathrm{x}}=\lambda^0 \cdot \mathrm{F}(\mathrm{x}, \mathrm{y})\)

Therefore, the given differential equation is a homogeneous equation.

To solve it, we make the substitution as:

y = \(v x \Rightarrow \frac{d}{d x}(y)=\frac{d}{d x}(v x) \Rightarrow \frac{d y}{d x}=v+x \frac{d v}{d x}\)

Substituting the values of x and \(\frac{dy}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{v x-x \sin v}{x} \Rightarrow v+x \frac{d v}{d x}=v-\sin v\)

⇒ \(-\frac{d v}{\sin v}=\frac{d x}{x} \Rightarrow \mathrm{cosec} v d v=-\frac{d x}{x} \Rightarrow \int \mathrm{cosec} v d v=-\int \frac{d x}{x}\)

log \(|\mathrm{cosec} v-\cot v|=-\log x+\log C=\log \frac{C}{x}\)

⇒ cosec \(\left(\frac{y}{x}\right)-\cot \left(\frac{y}{x}\right)=\frac{C}{x}\)

⇒ \(\frac{1}{\sin \left(\frac{y}{x}\right)}-\frac{\cos \left(\frac{y}{x}\right)}{\sin \left(\frac{y}{x}\right)}=\frac{C}{x}\)

⇒ x \(\left[1-\cos \left(\frac{y}{x}\right)\right]=C \sin \left(\frac{y}{x}\right)\)

This is the required solution of the given differential equation.

Question 9. \(y d x+x \log \left(\frac{y}{x}\right) d y-2 x d y=0\)
Solution:

⇒ \(y d x+x \log \left(\frac{y}{x}\right) d y-2 x d y=0 \Rightarrow y d x=\left[2 x-x \log \left(\frac{y}{x}\right)\right] d y\)

⇒ \(\frac{\mathrm{dy}}{\mathrm{dx}}=\frac{\mathrm{y}}{2 \mathrm{x}-\mathrm{x} \log \left(\frac{\mathrm{y}}{\mathrm{x}}\right)}\)….(1)

Let F(x, y) = \(\frac{y}{2 x-x \log \left(\frac{y}{x}\right)}\)

∴ \(F(\lambda x, \lambda y)=\frac{\lambda y}{2(\lambda x)-(\lambda x) \log \left(\frac{\lambda y}{\lambda x}\right)}\)

= \(\frac{\lambda y}{\lambda\left[2 x-x \log \left(\frac{y}{x}\right)\right]}=\lambda^0 F \mathrm{~F}(x, y)\)

Therefore, the given differential equation is a homogeneous equation.

To solve it, we make the substitution as: y = \(v x \Rightarrow \frac{d y}{d x}=\frac{d}{d x}(v x) \Rightarrow \frac{d y}{d x}=v+x \frac{d v}{d x}\)

Substituting the values of y and \(\frac{d y}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{v x}{2 x-x \log v} \Rightarrow v+x \frac{d v}{d x}=\frac{v}{2-\log v} \Rightarrow x \frac{d v}{d x}=\frac{v}{2-\log v}-v\)

⇒ \(x \frac{d v}{d x}=\frac{v-2 v+v \log v}{2-\log v} \Rightarrow x \frac{d v}{d x}=\frac{v \log v-v}{2-\log v} \Rightarrow \frac{2-\log v}{v(\log v-1)} d v=\frac{d x}{x}\)

⇒ \({\left[\frac{1+(1-\log v)}{v(\log v-1)}\right] d v=\frac{d x}{x} \Rightarrow\left[\frac{1}{v(\log v-1)}-\frac{1}{v}\right] d v=\frac{d x}{x} }\)

Integrating both sides, we get: \(\int \frac{1}{v(\log v-1)} d v-\int \frac{1}{v} d v=\int \frac{1}{x} d x\)

⇒ \(\int \frac{d v}{v(\log v-1)}-\log v=\log x+\log C\)…..(2)

Let log v-1=t

⇒ \(\frac{\mathrm{d}}{\mathrm{dv}}(\log \mathrm{v}-1)=\frac{\mathrm{dt}}{\mathrm{dv}} \Rightarrow \frac{1}{\mathrm{v}}=\frac{\mathrm{dt}}{\mathrm{dv}} \Rightarrow \frac{\mathrm{dv}}{\mathrm{v}}=\mathrm{dt}\)

Therefore, equation (2) becomes:

⇒ \(\int \frac{d t}{t}-\log v=\log x+\log C\)

⇒ \(\log t-\log v=\log (C x)=\log (\log v-1)-\log v=\log (C x)\)

⇒ \(\log \left[\log \left(\frac{y}{x}\right)-1\right]-\log \left(\frac{y}{x}\right)=\log (C x)\)

⇒ \(\log \left[\frac{\log \left(\frac{y}{x}\right)-1}{\frac{y}{x}}\right]=\log (C x) \Rightarrow \frac{x}{y}\left[\log \left(\frac{y}{x}\right)-1\right]=C x \Rightarrow \log \left(\frac{y}{x}\right)-1=C y\)

This is the required solution of the given differential equation.

Question 10. \(\left(1+e^{\frac{x}{y}}\right) d x+e^{\frac{x}{y}}\left(1-\frac{x}{y}\right) d y=0\)
Solution:

⇒ \(\left(1+e^{\frac{x}{y}}\right) d x+e^{\frac{x}{y}}\left(1-\frac{x}{y}\right) d y=0 \Rightarrow\left(1+e^{\frac{x}{y}}\right) d x=-e^{\frac{x}{y}}\left(1-\frac{x}{y}\right) d y\)

⇒ \(\frac{\mathrm{dx}}{\mathrm{dy}}=\frac{-\mathrm{e}^{\frac{x}{y}}\left(1-\frac{\mathrm{x}}{\mathrm{y}}\right)}{1+\mathrm{e}^{\frac{\mathrm{x}}{y}}}\)….(1)

Let F(x, y) = \(\frac{-e^{\frac{x}{y}}\left(1-\frac{x}{y}\right)}{1+e^{\frac{x}{y}}}\)

∴ \(F(\lambda x, \lambda y)=\frac{-e^{\frac{\lambda x}{\lambda y}}\left(1-\frac{\lambda x}{\lambda y}\right)}{1+e^{\frac{\lambda x}{\lambda y}}}\)

= \(\frac{-e^{\frac{x}{y}}\left(1-\frac{x}{y}\right)}{1+e^{\frac{x}{y}}}=\lambda^0 \cdot F(x, y)\)

Therefore, the given differential equation is a homogeneous equation.

To solve it, we make the substitution as: \(\mathrm{x}=\mathrm{vy} \Rightarrow \frac{\mathrm{d}}{\mathrm{dy}}(\mathrm{x})\)

= \(\frac{\mathrm{d}}{\mathrm{dy}}(\mathrm{vy}) \Rightarrow \frac{\mathrm{dx}}{\mathrm{dy}}=\mathrm{v}+\mathrm{y} \frac{\mathrm{dv}}{\mathrm{dy}}\)

Substituting the values of x and \(\frac{\mathrm{dx}}{\mathrm{dy}}\) in equation (1), we get:

v+y \(\frac{d v}{d y}=\frac{-e^v(1-v)}{1+e^v} \Rightarrow y \frac{d v}{d y}=\frac{-e^v+v^v}{1+e^v}-v\)

⇒ \(y \frac{d v}{d y}=\frac{-e^v+v^v-v-v e^v}{1+e^v} \Rightarrow y \frac{d v}{d y}=-\left[\frac{v+e^v}{1+e^v}\right]\)

⇒ \(\left[\frac{1+e^v}{v+e^v}\right] d v=-\frac{d y}{y}\)

Integrating both sides, we get:

⇒ \(\log \left(v+e^v\right)=-\log y+\log C=\log \left(\frac{C}{y}\right) \Rightarrow \log \left(v+e^v\right)=\log \left(\frac{C}{y}\right)\)

⇒ \(\frac{x}{y}+e^{\frac{x}{y}}=\frac{C}{y} \Rightarrow x+y e^y=C\)

This is the required solution of the given differential equation.

For Each Of The Differential Equations, Find The Particular Solution Satisfying The Given Condition:

Question 11. (x+y) d y+(x-y) d x=0 ; y=1 when x=1
Solution:

(x+y) d y+(x-y) d x=0 \Rightarrow(x+y) d y=-(x-y) d x

⇒ \(\frac{d y}{d x}=\frac{-(x-y)}{x+y}\)………(1)

The given differential equation is a homogeneous equation.

To solve it, we make the substitution as:

y = \(v x \Rightarrow \frac{d}{d x}(y)=\frac{d}{d x}(v x) \Rightarrow \frac{d y}{d x}=v+x \frac{d v}{d x}\)

Substituting the values of y and \(\frac{d y}{d x}\) in equation (1), we get:

⇒ \(v+x \frac{d v}{d x}=\frac{-(x-v x)}{x+v x} \Rightarrow v+x \frac{d v}{d x}=\frac{v-1}{v+1}\)

⇒ \(x \frac{d v}{d x}=\frac{v-1}{v+1}-v=\frac{v-1-v(v+1)}{v+1} \Rightarrow x \frac{d v}{d x}=\frac{v-1-v^2-v}{v+1}=\frac{-\left(1+v^2\right)}{v+1}\)

⇒ \(\frac{(v+1)}{1+v^2} d v=-\frac{d x}{x} \Rightarrow \int \frac{v+1}{1+v^2} d v=-\int \frac{d x}{x}\)

⇒ \(\int\left[\frac{v}{1+v^2}+\frac{1}{1+v^2}\right] d v=-\int \frac{d x}{x}\)

⇒ \(\frac{1}{2} \log \left(1+v^2\right)+\tan ^{-1} v=-\log x+k \Rightarrow \log \left(1+v^2\right)+2 \tan ^{-1} v=-2 \log x+2 k\)

⇒ \(\log \left(x^2+y^2\right)+2 \tan ^{-1} \frac{y}{x}=2 k\)…..(2)

Now, y=1 at x=1

⇒ \(\log 2+2 \tan ^{-1} 1=2 k \Rightarrow \log 2+2 \times \frac{\pi}{4}=2 k \Rightarrow \frac{\pi}{2}+\log 2=2 k\)

Substituting the value of 2k in equation (2), we get:

⇒ \(\log \left(x^2+y^2\right)+2 \tan ^{-1}\left(\frac{y}{x}\right)=\frac{\pi}{2}+\log 2\)

This is the required solution of the given differential equation.

Question 12. \(x^2 d y+\left(x y+y^2\right) d x=0\) ; y=1 when x=1
Solution:

⇒ \(x^2 d y+\left(x y+y^2\right) d x=0 \Rightarrow x^2 d y=-\left(x y+y^2\right) d x \)

⇒ \(\frac{d y}{d x}=\frac{-\left(x y+y^2\right)}{x^2}\)….(1)

The given differential equation is a homogeneous equation.

To solve it, we make the substitution as:

y = vx \(\frac{d}{d x}(y)=\frac{d}{d x}(v x) \Rightarrow \frac{d y}{d x}=v+x \frac{d v}{d x}\)

Substituting the values of y and \(\frac{d y}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{-\left[x \cdot v x+(v x)^2\right]}{x^2}=-v-v^2\)

⇒ \(x \frac{d v}{d x}=-v^2-2 v=-v(v+2) \Rightarrow \frac{d v}{v(v+2)}=-\frac{d x}{x}\)

⇒ \(\int \frac{d v}{v(v+2)}=-\int \frac{d x}{x} \Rightarrow \int \frac{1}{2}\left[\frac{(v+2)-v}{v(v+2)}\right] d v\)

= \(-\int \frac{d x}{x} \Rightarrow \int \frac{1}{2}\left[\frac{1}{v}-\frac{1}{v+2}\right] d v=-\int \frac{d x}{x}\)

⇒ \(\frac{1}{2}[\log v-\log (v+2)]=-\log x+\log C \Rightarrow \frac{1}{2} \log \left(\frac{v}{v+2}\right)=\log \frac{C}{x}\)

⇒ \(\frac{v}{v+2}=\left(\frac{C}{x}\right)^2 \Rightarrow \frac{\frac{y}{x}}{\frac{y}{x}+2}=\left(\frac{C}{x}\right)^2\)

⇒ \(\frac{y}{y+2 x}=\frac{C^2}{x^2} \Rightarrow \frac{x^2 y}{y+2 x}=C^2\)….(2)

Put, y=1 and x=1 in eq.(2), we get \(\frac{1}{1+2}=C^2 \Rightarrow C^2=\frac{1}{3}\)

Substituting \(C^2=\frac{1}{3}\) in equation (2), we get: \(\frac{x^2 y}{y+2 x}=\frac{1}{3} \Rightarrow y+2 x=3 x^2 y\)

This is the required solution of the given differential equation

Question 13. \(\left[x \sin ^2\left(\frac{y}{x}\right)-y\right] d x+x d y=0 ; y=\frac{\pi}{4}\) when x=1
Solution:

⇒ \(\left[x \sin ^2\left(\frac{y}{x}\right)-y\right] d x+x d y=0 \Rightarrow \frac{d y}{d x}\)

= \(\frac{-\left[x \sin ^2\left(\frac{y}{x}\right)-y\right]}{x}\)…….(1)

The given differential equation is a homogeneous equation.

To solve this differential equation, we make the substitution as: \(\mathrm{y}=\mathrm{vx} \Rightarrow \frac{\mathrm{d}}{\mathrm{dx}}(\mathrm{y})=\frac{\mathrm{d}}{\mathrm{dx}}(\mathrm{vx}) \Rightarrow \frac{\mathrm{dy}}{\mathrm{dx}}=\mathrm{v}+\mathrm{x} \frac{\mathrm{dv}}{\mathrm{dx}}\)

Substituting the values of y and \(\frac{d y}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{-\left[x \sin ^2 v-v x\right]}{x} \Rightarrow v+x \frac{d v}{d x}=-\left[\sin ^2 v-v\right]=v-\sin ^2 v\)

⇒ \(x \frac{d v}{d x}=-\sin ^2 v \Rightarrow \frac{d v}{\sin ^2 v}=-\frac{d x}{x} \)

⇒ \(\mathrm{cosec}^2 v d v=-\frac{d x}{x} \Rightarrow \int \mathrm{cosec}^2 v d v=-\int \frac{d x}{x}\)

⇒ \(\cot \left(\frac{y}{x}\right)=\log |x|-\log C \Rightarrow \cot \left(\frac{y}{x}\right)=\log \left|\frac{x}{C}\right|\)…..(2)

Now, \(y=\frac{\pi}{4}\) at x=1 \(\Rightarrow \cot \left(\frac{\pi}{4}\right)=\log \left|\frac{1}{C}\right| \Rightarrow 1=-\log C \Rightarrow C=e^{-t}\)

Substituting \(\mathrm{C}=\mathrm{e}^{-1}\) in equation (2), we get : \(\cot \left(\frac{\mathrm{y}}{\mathrm{x}}\right)=\log |\mathrm{ex}|\)

This is the required solution of the given differential equation.

Question 14. \(\frac{d y}{d x}-\frac{y}{x}+\mathrm{cosec}\left(\frac{y}{x}\right)=0 ; y=0\) when x=1
Solution:

⇒ \(\frac{d y}{d x}=\frac{y}{x}-\mathrm{cosec}\left(\frac{y}{x}\right)\)…..(1)

The given differential equation is a homogeneous equation.

To solve it, we make the substitution as:

y = \(v x \Rightarrow \frac{d}{d x}(y)=\frac{d}{d x}(v x) \Rightarrow \frac{d y}{d x}=v+x \frac{d v}{d x}\)

Substituting the values of y and \(\frac{d y}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=v-\mathrm{cosec} v \Rightarrow-\frac{d v}{\mathrm{cosec} v}=\frac{d x}{x}\)

⇒ \(-\sin v d v=\frac{d x}{x} \Rightarrow-\int \sin v d v=\int \frac{d x}{x}\)

cos v = \(\log x+\log C=\log |C x| \Rightarrow \cos \left(\frac{y}{x}\right)=\log |C x|\)….(2)

This is the required solution of the given differential equation.

Now, y=0 at x=1

⇒ cos (0) = \(\log \mathrm{C} \Rightarrow 1=\log \mathrm{C} \Rightarrow \mathrm{C}=\mathrm{e}^{\prime}=\mathrm{e}\)

Substituting C=e in equation (2), we get: \(\cos \left(\frac{y}{x}\right)=\log |(e x)|\)

This is the required solution of the given differential equation.

Question 15. \(2 x y+y^2-2 x^2 \frac{d y}{d x}=0 ; y=2\) when x=1
Solution:

2xy + \(y^2-2 x^2 \frac{d y}{d x}=0 \Rightarrow 2 x^2 \frac{d y}{d x}=2 x y+y^2 \Rightarrow \frac{d y}{d x}=\frac{2 x y+y^2}{2 x^2}\)….(1)

The given differential equation is a homogeneous equation.

To solve it, we make the substitution as:

y = \(v x \Rightarrow \frac{d}{d x}(y)=\frac{d}{d x}(v x) \Rightarrow \frac{d y}{d x}=v+x \frac{d v}{d x}\)

Substituting the value of y and \(\frac{d y}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{2 x(v x)+(v x)^2}{2 x^2} \Rightarrow v+x \frac{d v}{d x}=\frac{2 v+v^2}{2} \Rightarrow v+x \frac{d v}{d x}=v+\frac{v^2}{2}\)

⇒ \(\frac{2}{v^2} d v=\frac{d x}{x} \Rightarrow \int \frac{2}{v^2} d v=\int \frac{d x}{x}\)

⇒ \(2 \cdot \frac{v^{-2 d}}{-2+1}=\log |x|+C \Rightarrow-\frac{2}{v}=\log |x|+C\)

⇒ \(-\frac{2}{y}=\log |x|+C \Rightarrow-\frac{2 x}{y}=\log |x|+C\)…..(2)

Now, y = 2 at x = l.

⇒ -l = log(1) + C ⇒ C = -1

Substituting C = -1 in equation (2), we get:

– \(\frac{2 x}{y}=\log |x|-1 \Rightarrow \frac{2 x}{y}=1-\log |x| \Rightarrow y=\frac{2 x}{1-\log |x|},(x \neq 0, x \neq e)\)

This is the required solution of the given differential equation.

Question 16. A homogeneous differential equation of the form \(\frac{dx}{dy}\) = h(\(\frac{x}{y}\)) can be solved by making the substitution

1. y = vx
2. v = yx
3. x = vy
4. x = v

Solution:

For solving the homogeneous equation of the form \(\frac{dx}{dy}\) = h(\(\frac{x}{y}\)), we need to make the substitution as x = vy.

Hence, the correct answer is 3.

Question 17. Which of the following is a homogeneous differential equation?

1. (4x + 6y + 5)dy – (3y + 2x + 4)dx = 0
2. (xy)dx – (x³ + y³)dy = 0
3. (x³ + 2y²)dx + 2xydy = 0
4. y²dx + (x² – xy – y²)dy = 0

Solution:

Out of the given four options; option (4) is the only option in which all coefficients of dx and dy are of the same degree, therefore function F(x, y) is said to be the homogenous function of degree n, if

F(λx, λy) = λ” F(x, y) for any non-zero constant (X).

Consider the equation given in option D: y²dx + (x² – xy – y²)dy = 0

⇒ \(\frac{d y}{d x}=\frac{-y^2}{x^2-x y-y^2}=\frac{y^2}{y^2+x y-x^2}\)

Let F(x, y) = \(\frac{y^2}{y^2+x y-x^2} \Rightarrow F(\lambda x, \lambda y)=\frac{(\lambda y)^2}{(\lambda y)^2+(\lambda x)(\lambda y)-(\lambda x)^2}\)

= \(\frac{\lambda^2 y^2}{\lambda^2\left(y^2+x y-x^2\right)}=\lambda^0\left(\frac{y^2}{y^2+x y-x^2}\right)=\lambda^0 \cdot F(x, y)\)

Hence, the differential equation given in option 4 is a homogenous equation.

Differential Equation Exercise 9.5

For Each Of The Differential Equations, Find The General Solution

Question 1. \(\frac{d y}{d x}+2 y=\sin x\)
Solution:

The given differential equation is \(\frac{\mathrm{dy}}{\mathrm{dx}}+2 \mathrm{y}=\sin \mathrm{x}\)

This is a linear differential equation of the form \(\frac{d y}{d x}+P y=Q\) (where P=2 and Q= sin x)

Now, I.F. = \(\mathrm{e}^{\int \mathrm{Pdtx}}=\mathrm{e}^{\int 2 \mathrm{dx}}=\mathrm{e}^{2 \mathrm{x}}\)

The solution of the given differential equation is given by the relation,

y(I.F) = \(\int(Q \times \text { I.F. }) d x+C \Rightarrow y e^{2 x}=\int \sin x \cdot e^{2 x} d x+C\)…..(1)

Let \(I=\int_I \sin x \cdot e^{2 x} d x\)

⇒ \(I=\sin x \cdot \int e^{2 x} d x-\int\left(\frac{d}{d x}(\sin x) \cdot \int e^{2 x} d x\right) d x\)

⇒ \(I=\sin x \cdot \frac{e^{2 x}}{2}-\int\left(\cos x \cdot \frac{e^{2 x}}{2}\right) d x\)

⇒ I = \(\frac{e^{2 x} \sin x}{2}-\frac{1}{2}\left[\cos x \cdot \int e^{2 x}-\int\left(\frac{d}{d x}(\cos x) \cdot \int e^{2 x} d x\right) d x\right]\)

⇒ \(I=\frac{e^{2 x} \sin x}{2}-\frac{1}{2}\left[\cos x \cdot \frac{e^{2 x}}{2}-\int\left[(-\sin x) \cdot \frac{e^{2 x}}{2}\right] d x\right]\)

⇒ I = \(\frac{e^{2 x} \sin x}{2}-\frac{e^{2 x} \cos x}{4}-\frac{1}{4} \int\left(\sin x \cdot e^{2 x}\right) d x\)

⇒ \(I=\frac{e^{2 x}}{4}(2 \sin x-\cos x)-\frac{1}{4} I \Rightarrow \frac{5}{4} I=\frac{e^{2 x}}{4}(2 \sin x-\cos x)\)

⇒ \(I=\frac{e^{2 x}}{5}(2 \sin x-\cos x)\)

Therefore, equation (1) becomes: \(\mathrm{ye}^{2 \mathrm{x}}=\frac{\mathrm{e}^{2 \mathrm{x}}}{5}(2 \sin \mathrm{x}-\cos \mathrm{x})+\mathrm{C} \Rightarrow \mathrm{y}=\frac{1}{5}(2 \sin \mathrm{x}-\cos \mathrm{x})+\mathrm{Ce}^{-2 \mathrm{x}}\)

This is the required general solution of the given differential equation.

Question 2. \(\frac{d y}{d x}+3 y=e^{-2 x}\)
Solution:

The given differential equation is \(\frac{d y}{d x}+3 y=e^{-2 x}\).

This is a linear differential equation of the form \(\frac{d y}{d x}+P y=Q\) (where P=3 and Q = \(e^{-2 x}\))

Now, I.F. = \(\mathrm{e}^{\int \mathrm{Pdx}}=\mathrm{e}^{\int \frac{3 \mathrm{dx}}{\mathrm{s}}=\mathrm{e}^{3 \mathrm{x}}}\)

The solution of the given differential equation is given by the relation,

y(I.F.)= \(\int(Q \times \text { I.F. }) d x+C \Rightarrow y e^{3 x}=\int\left(e^{-2 x} \times e^{3 x}\right)+C \Rightarrow y e^{3 x}=\int e^x d x+C\)

⇒ \(y e^{3 x}=e^x+C \Rightarrow y=e^{-2 x}+C e^{-5 x}\)

This is the required general solution of the given differential equation.

Question 3. \(\frac{d y}{d x}+\frac{y}{x}=x^2\)
Solution:

The given differential equation is \(\frac{d y}{d x}+\frac{y}{x}=x^2\).

This is a linear differential equation of the form \(\frac{d y}{d x}+P y=Q\) (where \(P=\frac{1}{x}\) and \(Q=x^2\))

Now, I.F. = \(\mathrm{e}^{\int \text { Pdx }}=\mathrm{e}^{\int \frac{1}{x}-\mathrm{dx}}=\mathrm{e}^{\log \mathrm{x}}=\mathrm{x}\)

The solution of the given differential equation is given by,

y(I.F.) = \(\int(Q \times I . F .) d x+C \Rightarrow y(x)=\int\left(x^2 \cdot x\right) d x+C\)

⇒ \(x y=\int x^3 d x+C \Rightarrow x y=\frac{x^4}{4}+C\)

This is the required general solution of the given differential equation.

Question 4. \(\frac{d y}{d x}+(\sec x) y=\tan x\left(0 \leq x<\frac{\pi}{2}\right)\)
Solution:

The given differential equation is \(\frac{\mathrm{dy}}{\mathrm{dx}}+(\sec x) \mathrm{y}=\tan \mathrm{x}\).

This is a linear differential equation of the form \(\frac{d y}{d x}+P y=Q\) (where P=sec x and Q=tan x)

Now, I.F. = \(\mathrm{e}^{\int \mathrm{Prtx}}=\mathrm{e}^{\int \sec x d x}=\mathrm{e}^{\log (\sec x+\tan x)}=\sec \mathrm{x}+\tan \mathrm{x}\)

The general solution of the given differential equation is given by the relation,

y(I.F.) = \(\int(Q \times \text { I.F. }) d x+C\)

⇒ y(sec x+tan x) = \(\int \tan x(\sec x+\tan x) d x+C \Rightarrow y(\sec x+\tan x)=\int \sec x \tan x d x+\int \tan ^2 x d x+C\)

⇒ y(sec x+tan x) = \(\sec x+\int\left(\sec ^2 x-1\right) d x+C \Rightarrow y(\sec x+\tan x)=\sec x+\tan x-x+C\)

Question 5. \(\cos ^2 x \frac{d y}{d x}+y=\tan x\left(0 \leq x<\frac{\pi}{2}\right)\)
Solution:

It is given that \(\cos ^2 x \frac{d y}{d x}+y=\tan x \Rightarrow \frac{d y}{d x}+\sec ^2 x y=\sec ^2 x \tan x\)

This is a differential equation in the form of \(\frac{d y}{d x}+P y=Q\) (where, P= \(sec ^2 x\) and Q = \(\sec ^2 x \tan x\))

Now, I.F. = \(\mathrm{e}^{\int \mathrm{Pdx}}=\mathrm{e}^{\int \sec ^2 \mathrm{x} d \mathrm{x}}=\mathrm{e}^{\tan \mathrm{x}}\)

Thus, the solution of the given differential equation is given by the relation :

y(I.F.) = \(\int(Q \times I . F) d x+C\)

⇒ \(y \cdot e^{\mathrm{tan} x}=\int e^{\mathrm{tan} x} \sec ^2 x \tan x d x+C\)….(1)

Now, Let tan x = \(t \Rightarrow \sec ^2 x d x=d t\)

Thus, the equation (1) becomes

⇒ \(y \cdot e^{\tan x}=\int\left(e^t \cdot t\right) d t+C \Rightarrow y \cdot e^{\tan x}=t \cdot \int e^t d t-\int\left(\frac{d}{d t}(t) \cdot \int e^t d t\right) d t+C\)

⇒ \(y \cdot e^{\tan x}=t \cdot e^t-\int e^t d t+C \Rightarrow y e^{\tan x}=(t-1) e^t+C\)

⇒ \(y e^{\tan x}=(\tan x-1) e^{\tan x}+C \Rightarrow y=(\tan x-1)+C e^{-\tan x}\)

Therefore, the required general solution of the given differential equation \(y=(\tan x-1)+C e^{-\tan x}\)

Question 6. \(x \frac{d y}{d x}+2 y=x^2 \log x\)
Solution:

The given differential equation is : \(x \frac{d y}{d x}+2 y=x^2 \log x \Rightarrow \frac{d y}{d x}+\frac{2}{x} y=x \log x\)

This equation is in the form of a linear differential equation as: \(\frac{d y}{d x}\) +Py=Q (where P = \(\frac{2}{x}\) and Q = x log x)

Now, I.F. = \(\mathrm{e}^{\int \mathrm{Pdx}}=\mathrm{e}^{\int_{\mathrm{x}}^2 \mathrm{dx}}=\mathrm{e}^{2 \log x}=\mathrm{e}^{\log \mathrm{x}^2}=\mathrm{x}^2\)

The general solution of the given differential equation is given by the relation,

y(I.F.) = \(\int(Q \times I . F .) d x+C \Rightarrow y \cdot x^2=\int\left(x \log x \cdot x^2\right) d x+C\)

⇒ \(x^2 y=\int\left(x^3 \log x\right) d x+C \Rightarrow x^2 y=\log x \cdot \int x^3 d x-\int\left[\frac{d}{d x}(\log x) \cdot \int x^3 d x\right] d x+C\)

⇒ \(x^2 y=\log x \cdot \frac{x^4}{4}-\int\left(\frac{1}{x} \frac{x^4}{4}\right) d x+C \Rightarrow x^2 y=\frac{x^4 \log x}{4}-\frac{1}{4} \int x^3 d x+C\)

⇒ \(x^2 y=\frac{x^4 \log x}{4}-\frac{1}{4} \cdot \frac{x^4}{4}+C \Rightarrow x^2 y=\frac{1}{16} x^4(4 \log x-1)+C\)

⇒ \(y=\frac{1}{16} x^2(4 \log x-1)+C x^{-2}\)

Question 7. \(x \log x \frac{d y}{d x}+y=\frac{2}{x} \log x\)
Solution:

The given differential equation is: \(x \log x \frac{d y}{d x}+y=\frac{2}{x} \log x \Rightarrow \frac{d y}{d x}+\frac{y}{x \log x}=\frac{2}{x^2}\)

This equation is the form of a linear differential equation as: \(\frac{d y}{d x}+P y=Q \quad\left(\text { where } P=\frac{1}{x \log x} \text { and } Q=\frac{2}{x^2}\right)\)

Now, I.F. = \(\mathrm{e}^{\int \mathrm{Pdx}}=\mathrm{e}^{\int \frac{1}{x \log x} d x}=\mathrm{e}^{\log (\log x)}=\log \mathrm{x}\)

The general solution of the given differential equation is given by the relation,

y(I.F .) = \(\int(Q \times \text { I.F. }) d x+C \Rightarrow y \log x=\int\left(\frac{2}{x^2} \log x\right) d x+C\)

Now, \(\int\left(\frac{2}{x^2} \log x\right) d x=2 \int\left(\log x \cdot \frac{1}{x^2}\right) d x\)

= \(2\left[\log x \cdot \int \frac{1}{x^2} d x-\int\left\{\frac{d}{d x}(\log x) \cdot \int \frac{1}{x^2} d x\right\} d x\right]\)

= \(2\left[\log x\left(-\frac{1}{x}\right)-\int\left(\frac{1}{x} \cdot\left(-\frac{1}{x}\right)\right) d x\right]=2\left[-\frac{\log x}{x}+\int \frac{1}{x^2} d x\right]\)

= \(2\left[-\frac{\log x}{x}-\frac{1}{x}\right]=-\frac{2}{x}(1+\log x)\)

Substituting the value of \(\int\left(\frac{2}{x^2} \log x\right) d x\) in equation (1), we get : \(y \log x=-\frac{2}{x}(1+\log x)+C\)

This is the required general solution of the given differential equation.

Question 8. \(\left(1+x^2\right) d y+2 x y d x=\cot x d x(x \neq 0)\)
Solution:

(1+ \(x^2\)) dy+2 x y dx =cot x d x

⇒ \(\frac{d y}{d x}+\frac{2 x y}{1+x^2}=\frac{\cot x}{1+x^2}\)

This equation is a linear differential equation of the form:

⇒ \(\frac{d y}{d x}+P y=Q\)(where P = \(\frac{2 x}{1+x^2}\) and Q = \(\frac{\cot x}{1+x^2}\))

Now, I.F. = \(\mathrm{e}^{\int \mathrm{Pdx}}=\mathrm{e}^{\int \frac{2 \mathrm{x}}{1+\mathrm{x}^2} \mathrm{dx}}=\mathrm{e}^{\log \left(1+\mathrm{x}^2\right)}=1+\mathrm{x}^2\)

The general solution of the given differential equation is given by the relation,

y(I.F.) = \(\int(Q \times \text { I.F. }) d x+C \Rightarrow y\left(1+x^2\right)=\int\left[\frac{\cot x}{1+x^2} \times\left(1+x^2\right)\right] d x+C\)

⇒ y\(\left(1+x^2\right)=\int \cot x d x+C \Rightarrow y\left(1+x^2\right)=\log |\sin x|+C\)

Question 9. \(x \frac{d y}{d x}+y-x+x y \cot x=0(x \neq 0)\)
Solution:

⇒ \(x \frac{d y}{d x}+y-x+x y \cot x=0 \Rightarrow x \frac{d y}{d x}+y(1+x \cot x)=x \Rightarrow \frac{d y}{d x}+\left(\frac{1}{x}+\cot x\right) y=1\)

This equation is a linear differential equation of the form:

⇒ \(\frac{d y}{d x}+P y=Q\) (where P = \(\frac{1}{x}+\cot x\) and Q= 1)

Now, I.F. \(=\mathrm{e}^{\int P d x}=\mathrm{e}^{\int\left(\frac{1}{x}+\cot x\right) d x}=e^{\log x+\log (\sin x)}=e^{\log (x \sin x)}=x \sin x\)

The general solution of the given differential equation is given by the relation,

y(I.F.) = \(\int(Q \times \text { I.F. }) d x+C\)

⇒ \(y(x \sin x)=\int(1 \times x \sin x) d x+C \Rightarrow y(x \sin x)=\int(x \sin x) d x+C \)

⇒ \(y(x \sin x)=x \int \sin x d x-\int\left[\frac{d}{d x}(x) \cdot \int \sin x d x\right]+C \)

⇒ \(y(x \sin x)=x(-\cos x)-\int 1 \cdot(-\cos x) d x+C\)

⇒ \(y(x \sin x)=-x \cos x+\sin x+C \Rightarrow y=\frac{-x \cos x}{x \sin x}+\frac{\sin x}{x \sin x}+\frac{C}{x \sin x}\)

⇒ \(y=-\cot \cdot x+\frac{1}{x}+\frac{C}{x \sin x}\) (which is the required solution)

Question 10. \((x+y) \frac{d y}{d x}=1\)
Solution:

⇒ \((x+y) \frac{d y}{d x}=1 \Rightarrow \frac{d y}{d x}=\frac{1}{x+y} \Rightarrow \frac{d x}{d y}=x+y \Rightarrow \frac{d x}{d y}-x=y\)

This is a linear differential equation of the form: \(\frac{\mathrm{dx}}{\mathrm{dy}}+\mathrm{P}_1 \mathrm{x}=\mathrm{Q}_1\) (where \(\mathrm{P}_1=-1\) and \(\mathrm{Q}_1=\mathrm{y}\))

Now, I.F. = \(\mathrm{e}^{\int P_1 d y}=\mathrm{e}^{\int-\mathrm{dy}}=\mathrm{e}^{-\mathrm{y}}\)

The general solution of the given differential equation is given by the relation,

x(I.F.) = \(\int\left(Q_1 \times I . F .\right) d y+C\)

⇒ \(x^{-y}=\int\left(y \cdot e^{-y}\right) d y+C \Rightarrow x e^{-y}=y \cdot \int e^{-y} d y-\int\left[\frac{d}{d y}(y) \int e^{-y} d y\right] d y+C\)

⇒ \(x^{-y}=y\left(-e^{-y}\right)-\int\left(-e^{-y}\right) d y+C \Rightarrow \mathrm{xe}^{-y}=-y e^{-y}+\int e^{-y} d y+C\)

⇒ \(x^{-y}=-y e^{-y}-e^{-y}+C \Rightarrow x=-y-1+C e^z\)

⇒ \(x+y+1=C e^y\) (which is the required solution)

Question 11. \(y d x+\left(x-y^2\right) d y=0\)
Solution:

⇒ \(y d x+\left(x-y^2\right) d y=0 \Rightarrow y d x=\left(y^2-x\right) d y \Rightarrow \frac{d x}{d y}\)

= \(\frac{y^2-x}{y}=y-\frac{x}{y} \Rightarrow \frac{d x}{d y}+\frac{x}{y}=y\)

This is a linear differential equation of the form:

⇒ \(\frac{\mathrm{dx}}{\mathrm{dy}}+\mathrm{P}_1 \mathrm{x}=\mathrm{Q}_1\) (where \( \mathrm{P}_1=\frac{1}{\mathrm{y}}\) and \(\mathrm{Q}_1=\mathrm{y}\))

Now. I.F. = \(\mathrm{e}^{\int {p_1} d y}=\mathrm{e}^{\int \frac{1}{y} d y}=\mathrm{e}^{\log y}=y\)

The general solution of the given differential equation is given by the relation,

x( I.F.) = \(\int\left(Q_1 \times I . F .\right) d y+C \Rightarrow x y=\int(y \cdot y) d y+C \Rightarrow x y=\int y^2 d y+C\)

⇒ xy = \(\frac{y^3}{3}+C \Rightarrow x=\frac{y^2}{3}+\frac{C}{y}\)

Question 12. \(\left(x+3 y^2\right) \frac{d y}{d x}=y(y>0)\)
Solution:

⇒ \(\left(x+3 y^2\right) \frac{d y}{d x}=y \Rightarrow \frac{d y}{d x}=\frac{y}{x+3 y^2} \Rightarrow \frac{d x}{d y}\)

= \(\frac{x+3 y^2}{y}=\frac{x}{y}+3 y \Rightarrow \frac{d x}{d y}-\frac{x}{y}=3 y\)

This is a linear differential equation of the form:

⇒ \(\frac{\mathrm{dx}}{\mathrm{dy}}+\mathrm{P}_1 \mathrm{x}=\mathrm{Q}_1\) (where \(\mathrm{P}_1=-\frac{1}{\mathrm{y}}\) and \(\mathrm{Q}_{\mathbf{l}}=3 \mathrm{y}\))

Now, I.F. = \(\mathrm{e}^{\int P_1 d y}=\mathrm{e}^{-\int \frac{d y}{y}}=\mathrm{e}^{-\log y}=\mathrm{e}^{\log \left(\frac{1}{y}\right)}=\frac{1}{y}\)

The general solution of the given differential equation is given by the relation,

x(I .F.) = \(\int\left(Q_1 \times \text { I.F. }\right) d y+C\)

⇒ \(x \times \frac{1}{y}=\int\left(3 y \times \frac{1}{y}\right) d y+C \Rightarrow \frac{x}{y}=3 y+C \Rightarrow x=3 y^2+C y\)

For Each Of The Differential Equations, Find The Particular Solution Satisfying The Given Conditions:

Question 13. \(\frac{d y}{d x}+2 y \tan x=\sin x ; y=0\) when \(x=\frac{\pi}{3}\)
Solution:

The given differential equation is \(\frac{d y}{d x}+2 y \tan x=\sin x\)

This is a linear equation of the form: \(\frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{Py}=\mathrm{Q}\)(where \(\mathrm{P}=2 \tan \mathrm{x}\) and \(\mathrm{Q}=\sin \mathrm{x}\))

Now, I.F. = \(\mathrm{e}^{\int \mathrm{pdx}}=\mathrm{e}^{\int 2 \mathrm{sin} x d x}=\mathrm{e}^{2 \log |\sec x|}=\mathrm{e}^{\log \left(\sec ^2 \mathrm{x}\right)}=\sec ^2 \mathrm{x}\)

The general solution of the given differential equation is given by the relation,

y(I.F) = \(\int(Q \times \text { I.F. }) d x+C\)

⇒ \(y\left(\sec ^2 x\right)=\int\left(\sin x \cdot \sec ^2 x\right) d x+C \Rightarrow y \sec ^2 x=\int(\sec x \cdot \tan x) d x+C\)

⇒ \(y \sec ^2 x=\sec x+C\)

Put, y=0 and x \(=\frac{\pi}{3}\), in eq.(1)

Therefore, \(0 \times \sec ^2 \frac{\pi}{3}=\sec \frac{\pi}{3}+\mathrm{C} \Rightarrow 0=2+\mathrm{C} \Rightarrow \mathrm{C}=-2\)

Substituting C=-2 in equation (1), we get: \(y \sec ^2 x=\sec x-2 \Rightarrow y=\cos x-2 \cos ^2 x\)

Hence, the required solution of the given differential equation is \(y=\cos x-2 \cos ^2 x\).

Question 14. \(\left(1+x^2\right) \frac{d y}{d x}+2 x y=\frac{1}{1+x^2} ; y=0\) when x=1
Solution:

⇒ \(\left(1+x^2\right) \frac{d y}{d x}+2 x y=\frac{1}{1+x^2} \Rightarrow \frac{d y}{d x}+\frac{2 x y}{1+x^2}=\frac{1}{\left(1+x^2\right)^2}\)

This is a linear differential equation of the form: \(\frac{d y}{d x}+P y=Q\) (where P = \(\frac{2 x}{1+x^2}\) and Q = \(\frac{1}{\left(1+x^2\right)^2}\))

Now, I.F. \(=\mathrm{e}^{\int P \mathrm{Pdx}}=\mathrm{e}^{\int \frac{7 \mathrm{xdx}}{1+\mathrm{x}^2}}=\mathrm{e}^{\log \left(1+\mathrm{x}^2\right)}=1+\mathrm{x}^2\)

The general solution of the given differential equation is given by the relation,

y(I.F.) = \(\int(Q \times I . F .) d x+C\)

⇒ \(y\left(1+x^2\right)=\int\left[\frac{1}{\left(1+x^2\right)^2} \cdot\left(1+x^2\right)\right] d x+C\)

⇒ \(y\left(1+x^2\right)=\int \frac{1}{1+x^2} d x+C \Rightarrow y\left(1+x^2\right)=\tan ^{-1} x+C\)….(1)

Put y=0 and x=1 in eq.(1), we get

0 = \(\tan ^{-1} 1+C \Rightarrow C=-\frac{\pi}{4}\)

Substituting C = \(-\frac{\pi}{4}\) in equation (1), we get : \(y\left(1+x^2\right)=\tan ^{-1} x-\frac{\pi}{4}\)

This is the required general solution of the given differential equation.

Question 15. \(\frac{\mathrm{dy}}{\mathrm{dx}}-3 \mathrm{y} \cot \mathrm{x}=\sin 2 \mathrm{x} ; \mathrm{y}=2\) when \(\mathrm{x}=\frac{\pi}{2}\)
Solution:

The given differential equation is \(\frac{d y}{d x}-3 y \cot x=\sin 2 x\)

This is a linear differential equation of the form: \(\frac{d y}{d x}+P y=Q\) (where P=-3cot x and Q=sin 2 x)

Now, I.F. = \(\mathrm{e}^{\int P \mathrm{Pdx}}=\mathrm{e}^{-3 \int \cot x d x}=\mathrm{e}^{-3 \log |\sin x|}=\mathrm{e}^{\log \left|\frac{1}{\sin } \mathrm{x}\right|}=\frac{1}{\sin ^3 \mathrm{x}}\)

The general solution of the given differential equation is given by the relation,

y(I.F.) = \(\int(Q \times \text { I.F. }) d x+C\)

⇒ \(y \cdot \frac{1}{\sin ^3 x}=\int\left[\sin 2 x \cdot \frac{1}{\sin ^3 x}\right] d x+C \Rightarrow y \mathrm{cosec}^3 x=2 \int(\cot x \cos e c x) d x+C\)

⇒ \(y \mathrm{cosec}^3 x=-2 \mathrm{cosec} x+C \Rightarrow y=-\frac{2}{\mathrm{cosec}^2 x}+\frac{C}{\mathrm{cosec}^3 x}\)

⇒ y = \(-2 \sin ^2 x+C \sin ^3 x\)……(1)

Put y=2 and \(x=\frac{\pi}{2}\) in eq.(1) we get

2 = \(-2 \sin ^2 \frac{\pi}{2}+C \sin ^3 \frac{\pi}{2} \Rightarrow 2=-2+C \Rightarrow C=4\)

Substituting C=4 in equation (1), we get:

y = \(-2 \sin ^2 x+4 \sin ^3 x \Rightarrow y=4 \sin ^3 x-2 \sin ^2 x\)

Question 16. Find the equation of a curve passing through the origin given that the slope of the tangent to the curve at any point (x, y) is equal to the sum of the coordinates of the point.
Solution:

Lel F (x, y) is the curve passing through the origin.

At point (x, y), the scope of the curve will be \(\frac{dy}{dx}\)

According to the given information: \(\frac{d y}{d x}=x+y \Rightarrow \frac{d y}{d x}-y=x\)

This is a linear differential equation of the form: \(\frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{Py}=\mathrm{Q}\) (where P=-1 and Q = x)

Now, I.F. = \(\mathrm{e}^{\int 1 \mathrm{~d} d \mathrm{x}}=\mathrm{e}^{\int(-1) d x}=\mathrm{e}^{-\mathrm{x}}\)

The general solution of the given differential equation is given by the relation,

y(I.F.) = \(\int(Q \times \text { I.F. }) d x+C \Rightarrow \mathrm{ye}^{-x}=\int x e^{-x} d x+C\)……(1)

Now, \(\int x e^{-x} d x=x \int e^{-x} d x-\int\left[\frac{d}{d x}(x) \cdot \int e^{-x} d x\right] d x\)

= \(-x e^{-x}-\int-e^{-x} d x=-x e^{-x}+\left(-e^{-x}\right)=-e^{-x}(x+1)\)

Substituting in equation (1), we get:

⇒ \(\mathrm{ye}^{-x}=-\mathrm{e}^{-3}(\mathrm{x}+1)+\mathrm{C}\)

⇒ \(\mathrm{y}=-(\mathrm{x}+1)+\mathrm{Ce}^x \Rightarrow \mathrm{x}+\mathrm{y}+1=\mathrm{Ce}^{\mathrm{x}}\)….(2)

The curve passes through the origin. So, put x=0, y=0, and equation (2) becomes:

0+0+1= \(\mathrm{Ce}^0\) C=1

Substituting C =1 in equation (2), we get: x+y+1=\(e^x\)

Hence, the required equation of the curve passing through the origin is \(x+y+1=e^x\)

Question 17. Find the equation of a curve passing through the point (0, 2) given that the sum of the coordinates of any point on the curve exceeds the magnitude of ths slope of the tangent to the curve at that point by 5.
Solution:

Let F (x, y) be the curve, and let (x, y) be a point on the curve. The slope of the tangent to the curve at (x, y) is \(\frac{dy}{dx}\)

According to the given information: \(\frac{d y}{d x}+5=x+y \Rightarrow \frac{d y}{d x}-y=x-5\)

This is a linear differential equation of the form: \(\frac{d y}{d x}+P y=Q\) (where P=-1 and Q=x-5)

Now, I.F. = \(\mathrm{e}^{\int P d x}=\mathrm{e}^{\int(-1) d x}=\mathrm{e}^{-\mathrm{x}}\)

The general equation of the curve is given by the relation,

y(I.F.) = \(\int(Q \times I.F.) d x+C \Rightarrow y \cdot e^{-x}=\int(x-5) e^{-x} d x+C\)…..(1)

Now, \(\int(x-5) e^{-x} d x=(x-5) \int e^{-x} d x-\int\left[\frac{d}{d x}(x-5) \cdot \int e^{-x} d x\right] d x\)

= \((x-5)\left(-e^{-x}\right)-\int\left(-e^{-x}\right) d x=(5-x) e^{-x}+\left(-e^{-x}\right)=(4-x) e^{-x}\)

Therefore, equation (1) becomes: \(y^{-x x}=(4-x) e^{-x}+C\)

y = \(4-x+C e^x \Rightarrow x+y-4=C e^x\)…..(2)

The curve passes through the point (0,2)

Therefore, equation (2) becomes : \(0+2-4=\mathrm{Ce}^0 \Rightarrow-2=\mathrm{C} \Rightarrow \mathrm{C}=-2\)

Substituting C=-2 in equation (2), we get: \(\mathrm{x}+\mathrm{y}-4=-2 \mathrm{e}^{\mathrm{x}} \Rightarrow \mathrm{y}=4-\mathrm{x}-2 \mathrm{e}^{\mathrm{x}}\)

This is the required equation of the curve.

Choose The Correct Answer In The Following

Question 18. The integrating factor of the differential equation \(x \frac{d y}{d x}-y=2 x^2\) is

1. \(\mathrm{e}^{x}\)
2. \(\mathrm{e}^{-y}\)
3. \(\frac{1}{\mathrm{x}}\)
4. \(\mathrm{x}\)

Solution: 3. \(\frac{1}{\mathrm{x}}\)

The given differential equation is: \(x \frac{d y}{d x}-y=2 x^2 \Rightarrow \frac{d y}{d x}-\frac{y}{x}=2 x\)

This is a linear differential equation of the form: \(\frac{d y}{d x}+P y=Q\) (where \(P=-\frac{1}{x}\) and Q=2x)

The integrating factor (I.F.) is given by the relation, \(\mathrm{e}^{\int \mathrm{Pdx}}\)

∴ I.F. = \(\mathrm{e}^{\int-\frac{1}{x} d x}=\mathrm{e}^{-\log x}=\mathrm{e}^{\log \left[x^{-1}\right\}}=\mathrm{x}^{-1}=\frac{1}{\mathrm{x}}\)

Hence, the correct answer is 3.

Question 19. The integrating factor of the differential equation \(\left(1-y^2\right) \frac{d x}{d y}+y x=a y(-1<y<1)\) is

1. \(\frac{1}{y^2-1}\)
2. \(\frac{1}{\sqrt{y^2-1}}\)
3. \(\frac{1}{1-y^2}\)
4. \(\frac{1}{\sqrt{1-y^2}}\)

Solution: 4. \(\frac{1}{\sqrt{1-y^2}}\)

The given differential equation is: \(\left(1-y^2\right) \frac{d x}{d y}+y x=a y \Rightarrow \frac{d x}{d y}+\frac{y x}{1-y^2}=\frac{a y}{1-y^2}\)

This is a linear differential equation of the form: \(\frac{\mathrm{dx}}{\mathrm{dy}}+\mathrm{Px}=\mathrm{Q}\) (where \(\mathrm{P}=\frac{\mathrm{y}}{1-\mathrm{y}^2}\) and \(\mathrm{Q}=\frac{\mathrm{ay}}{1-\mathrm{y}^2}\))

The integrating factor (I.F.) is given by the relation,

∴ I.F. = \(\mathrm{e}^{\int \text { P.dy }}=\mathrm{e}^{\int \frac{y}{1-y^2} d y}=\mathrm{e}^{-\frac{1}{2} \log \left(1-y^2\right)}=\mathrm{e}^{\log \left[\frac{1}{\sqrt{1-y^2}}\right]}=\frac{1}{\sqrt{1-y^2}}\)

Hence, the correct answer is 4.

Differential Equations Miscellaneous Exercise

Question 1. For each of the differential equations given below, indicate its order and degree (if defined).

1. \(\frac{d^2 y}{d x^2}+5 x\left(\frac{d y}{d x}\right)^2-6 y=\log x\)
2. \(\left(\frac{d y}{d x}\right)^3-4\left(\frac{d y}{d x}\right)^2+7 y=\sin x\)
3. \(\frac{\mathrm{d}^4 y}{\mathrm{dx}^4}-\sin \left(\frac{\mathrm{d}^3 \mathrm{y}}{\mathrm{dx}^3}\right)=0\)

Solution:

1. The differential equation is given as:

⇒ \(\frac{d^2 y}{d x^2}+5 x\left(\frac{d y}{d x}\right)^2-6 y=\log x \Rightarrow \frac{d^2 y}{d x^2}+5 x\left(\frac{d y}{d x}\right)^2-6 y-\log x=0\)

The highest order derivative present in the differential equation is \(\frac{\mathrm{d}^2 \mathrm{y}}{\mathrm{dx}^2}\).

Thus, its order is two. The highest power raised to \(\frac{\mathrm{d}^2 \mathrm{y}}{\mathrm{dx}^2}\) is one. Hence, its degree is one.

2. The differential equation is given as:

⇒ \(\left(\frac{d y}{d x}\right)^3-4\left(\frac{d y}{d x}\right)^2+7 y=\sin x \Rightarrow\left(\frac{d y}{d x}\right)^3-4\left(\frac{d y}{d x}\right)^2+7 y-\sin x=0\)

The highest order derivative present in the differential equation is \(\frac{d y}{d x}\).

Thus, its order is one. The highest power raised to \(\frac{\mathrm{dy}}{\mathrm{dx}}\) is three. Hence, its degree is three.

3. The differential equation is given as: \(\frac{d^4 y}{d x^4}-\sin \left(\frac{d^3 y}{d x^3}\right)=0\)

The highest order derivative p-=resent in the differential equation, \(\frac{d^4 y}{d x^4}\). Thus, its order is four.

However, the given differential equation is not a polynomial equation in its derivatives,

Hence, its degree is not defined.

Question 2. For each of the exercises given below, verify that the given function (implicit or explicit) is a solution of the corresponding differential equation.

1. \(y=a e^x+b e^{-x}+x^2 \quad: \quad x \frac{d^2 y}{d x^2}+2 \frac{d y}{d x}-x y+x^2-2=0\)
2. \(\mathrm{y}=\mathrm{e}^{\mathrm{x}}(\mathrm{a} \cos \mathrm{x}+\mathrm{b} \sin \mathrm{x}) \quad: \frac{\mathrm{d}^2 \mathrm{y}}{\mathrm{dx}^2}-2 \frac{\mathrm{dy}}{\mathrm{dx}}+2 \mathrm{y}=0\)
3. \(\mathrm{y}=\mathrm{x} \sin 3 \mathrm{x} \quad: \frac{\mathrm{d}^2 \mathrm{y}}{\mathrm{dx}^2}+9 \mathrm{y}-6 \cos 3 \mathrm{x}=0\)
4. \(x^2=2 y^2 \log y \quad: \left(x^2+y^2\right) \frac{d y}{d x}-x y=0\)

Solution:

1. \(\mathrm{y}=a \mathrm{e}^{\mathrm{x}}+b \mathrm{e}^{-\mathrm{x}}+\mathrm{x}^2\)

Differentiating both sides with respect to x, we get:

⇒ \(\frac{d y}{d x}=a \frac{d}{d x}\left(e^x\right)+b \frac{d}{d x}\left(e^{-x}\right)+\frac{d}{d x}\left(x^2\right) \Rightarrow \frac{d y}{d x}=a e^x-b e^{-x}+2 x\)

Again, differentiating both sides with respect to x, we get: \(\frac{d^2 y}{d x^2}=a e^x+b e^{-x}+2\)

Now, on substituting the values of \(\frac{\mathrm{dy}}{\mathrm{dx}}\) and \(\frac{\mathrm{d}^2 \mathrm{y}}{\mathrm{dx}^2}\) in the differential equation, we get:

L.H.S. = \(x \frac{d^2 y}{d x^2}+2 \frac{d y}{d x}-x y+x^2-2\)

= \(x\left(a e^x+b e^{-x}+2\right)+2\left(a e^x-b e^{-x}+2 x\right)-x\left(a e^x+b e^{-x}+x^2\right)+x^2-2\)

= \(\left(a x e^x+b x e^{-x}+2 x\right)+\left(2 a e^x-2 b e^{-x}+4 x\right)-\left(a x e^x+b x e^{-4}+x^3\right)+x^2-2\)

= \(2 \mathrm{ae}^{\mathrm{x}}-2 \mathrm{be}^{-\mathrm{x}}-\mathrm{x}^3+\mathrm{x}^2+6 \mathrm{x}-2 \neq 0\)

⇒ \({ L.H.S. } \neq \text { R.H.S. }\)

Hence, the given function is not a solution to the corresponding differential equation.

2. \(\mathrm{y}=\mathrm{e}^{\mathrm{x}}(\mathrm{a} \cos \mathrm{x}+\mathrm{b} \sin \mathrm{x})=a \mathrm{e}^{\mathrm{x}} \cos \mathrm{x}+b \mathrm{e}^x \sin \mathrm{x}\)

Differentiating both sides with respect to x, we get:

⇒ \(\frac{d y}{d x}=a \cdot \frac{d}{d x}\left(e^x \cos x\right)+b \cdot \frac{d}{d x}\left(e^x \sin x\right)\)

⇒ \(\frac{d y}{d x}=a\left(e^x \cos x-e^x \sin x\right)+b \cdot\left(e^x \sin x+e^x \cos x\right)\)

⇒ \(\frac{d y}{d x}=(a+b) e^x \cos x+(b-a) e^x \sin x\)

Again, differentiating both sides with respect to x, we get:

⇒ \(\frac{d^2 y}{d x^2}=(a+b) \cdot \frac{d}{d x}\left(e^x \cos x\right)+(b-a) \frac{d}{d x}\left(e^x \sin x\right)\)

⇒ \(\frac{d^2 y}{d x^2}=(a+b) \cdot\left[e^x \cos x-e^x \sin x\right]+(b-a)\left[e^x \sin x+e^x \cos x\right]\)

⇒ \(\frac{d^2 y}{d x^2}=e^x[(a+b)(\cos x-\sin x)+(b-a)(\sin x+\cos x]\)

⇒ \(\frac{d^2 y}{d x^2}=e^x[a \cos x-a \sin x+b \cos x-b \sin x+b \sin x+b \cos x-a \sin x-a \cos x]\)

⇒ \(\frac{d^2 y}{d x^2}=\left[2 e^x(b \cos x-a \sin x)\right]\)

Now, on substituting the values of \(\frac{\mathrm{d}^2 y}{\mathrm{dx}^2}\) and \(\frac{\mathrm{dy}}{\mathrm{dx}}\) in the L.H.S. of the given differential equation, we get:

⇒ \(\frac{d^2 y}{d x^2}+ 2 \frac{d y}{d x}+2 y\)

= \(2 e^x(b \cos x-a \sin x)-2 e^x[(a+b) \cos x+(b-a) \sin x]+2 e^x(a \cos x+b \sin x)\)

= \(e^x\left[\begin{array}{l}
(2 b \cos x-2 a \sin x)-(2 a \cos x+2 b \cos x) \\
-(2 b \sin x-2 a \sin x)+(2 a \cos x+2 b \sin x)
\end{array}\right]\)

= \(e^2[(2 b-2 a-2 b+2 a) \cos x]+e^x[(-2 a-2 b+2 a+2 b) \sin x]=0\)

Hence, the given function is a solution of the corresponding differential equation.

3. \(\mathrm{y}=\mathrm{x} \sin 3 \mathrm{x}\)

Differentiating both sides with respect to x, we get: \(\frac{d y}{d x}=\frac{d}{d x}(x \sin 3 x)=\sin 3 x+x \cdot \cos 3 x \cdot 3 \Rightarrow \frac{d y}{d x}=\sin 3 x+3 x \cos 3 x\)

Again, differentiating both sides with respect to x, we get:

⇒ \(\frac{d^2 y}{d x^2}=\frac{d}{d x}(\sin 3 x)+3 \frac{d}{d x}(x \cos 3 x)\)

⇒ \(\frac{d^2 y}{d x^2}=3 \cos 3 x+3[\cos 3 x+x(-\sin 3 x) \cdot 3] \Rightarrow \frac{d^2 y}{d x^2}=6 \cos 3 x-9 x \sin 3 x\)

Substituting the value of \(\frac{\mathrm{d}^2 y}{\mathrm{dx}^2}\) in the L.H.S. of the given differential equation, we get:

⇒ \(\left.\frac{d^2 y}{d x^2}+9 y-6 \cos 3 x=(6 \cos 3 x-9 x \sin 3 x)+9 x \sin 3 x-6 \cos 3 x\right)=0\)

Hence, the given function is a solution of the corresponding differential equation.

4. \(x^2=2 y^2 \log y\)

Differentiating both sides with respect to x, we get:

2x = \(2 \cdot \frac{d}{d x}\left[y^2 \log y\right]\)

⇒ \(x=\left[2 y \cdot \log y \cdot \frac{d y}{d x}+y^2 \cdot \frac{1}{y} \cdot \frac{d y}{d x}\right] \Rightarrow x=\frac{d y}{d x}(2 y \log y+y)\)

⇒ \(\frac{d y}{d x}=\frac{x}{y(1+2 \log y)}\)

Substituting the value of \(\frac{d y}{d x}\) in the L.H.S. of the given differential equation, we get:

L.H.S. = \(\left(x^2+y^2\right) \frac{d y}{d x}-x y=\left(2 y^2 \log y+y^2\right) \cdot \frac{x}{y(1+2 \log y)}-x y\)

= \(y^2(1+2 \log y) \cdot \frac{x}{y(1+2 \log y)}-x y=x y-x y=0\)

Hence, the given function is a solution of the corresponding differential equation.

Question 3. Prove that \(x^2-y^2=c\left(x^2+y^2\right)^2\) is the general solution of differential equation \(\left(x^3-3 x y^2\right) d x=\left(y^3-3 x^2 y\right) d y\), where c is a parameter.
Solution:

⇒ \(\left(x^3-3 x y^2\right) d x=\left(y^3-3 x^2 y\right) d y \Rightarrow \frac{d y}{d x}=\frac{x^3-3 x y^2}{y^3-3 x^2 y}\)

This is a homogeneous differential equation. To simplify it, we need to make the substitution as:

⇒ \(\frac{d}{d x}(y)=\frac{d}{d x}(v x) \Rightarrow \frac{d y}{d x}=v+x \frac{d v}{d x}\)

Substituting the values of y and \(\frac{d y}{d x}\) in equation (1), we get:

v+x \(\frac{d v}{d x}=\frac{x^3-3 x(v x)^2}{(v x)^3-3 x^2(v x)}\)

⇒ v+x \(\frac{d v}{d x}=\frac{1-3 v^2}{v^3-3 v} \Rightarrow x \frac{d v}{d x}=\frac{1-3 v^2}{v^3-3 v}-v\)

⇒ x \(\frac{d v}{d x}=\frac{1-3 v^2-v\left(v^3-3 v\right)}{v^3-3 v}\)

⇒ \(x \frac{d v}{d x}=\frac{1-v^4}{v^3-3 v} \Rightarrow\left(\frac{v^3-3 v}{1-v^4}\right) d v=\frac{d x}{x}\)

⇒ \(\int\left(\frac{v^3-3 v}{1-v^4}\right) d v=\int \frac{d x}{x}\)….(2)

⇒ \(\int\left(\frac{v^3-3 v}{1-v^4}\right) d v=\log x+\log C^{\prime}\)

Now, \(\int\left(\frac{v^3-3 v}{1-v^4}\right) d v=\int \frac{v^3 d v}{1-v^4}-3 \int \frac{v d v}{1-v^4}\)

⇒ \(\int\left(\frac{v^3-3 v}{1-v^4}\right) d v=I_1-3 I_2\), where \(I_1=\int \frac{v^3 d v}{1-v^4}\) and \(I_2=\int \frac{v d v}{1-v^4}\)….(3)

Let \(1-v^4=t\)

∴ \(\frac{d}{d v}\left(1-v^4\right)=\frac{d t}{d v} \Rightarrow-4 v^3=\frac{d t}{d v} \Rightarrow v^3 d v=-\frac{d t}{4}\)

Now, \(I_1=\int \frac{-d t}{4 t}=-\frac{1}{4} \log t=-\frac{1}{4} \log \left(1-v^4\right)\)

And, \(I_2=\int \frac{v d v}{1-v^4}=\int \frac{v d v}{1-\left(v^2\right)^2}\)

Let \(v^2=p\)

⇒ \(\frac{d}{d v}\left(v^2\right)=\frac{d p}{d v} \Rightarrow 2 v=\frac{d p}{d v} \Rightarrow v d v=\frac{d p}{2}\)

⇒ \(I_2=\frac{1}{2} \int \frac{d p}{1-p^2}=\frac{1}{2 \times 2} \log \left|\frac{1+p}{1-p}\right|=\frac{1}{4} \log \left|\frac{1+v^2}{1-v^2}\right|\)

Substituting the values of \(\mathrm{I}_1\) and \(\mathrm{I}_2\) in equation (3), we get:

⇒ \(\int\left(\frac{v^3-3 v}{1-v^4}\right) d v=-\frac{1}{4} \log \left(1-v^4\right)-\frac{3}{4} \log \left|\frac{1+v^2}{1-v^2}\right|\)

Therefore, equation (2) becomes:

⇒ \(-\frac{1}{4} \log \left(1-v^4\right)-\frac{3}{4} \log \left|\frac{1+v^2}{1-v^2}\right|=\log x+\log C^{\prime}\)

⇒ \(-\frac{1}{4} \log \left[\left(1-v^4\right)\left(\frac{1+v^2}{1-v^2}\right)^3\right]=\log C^{\prime} x\)

⇒ \(\frac{\left(1+v^2\right)^4}{\left(1-v^2\right)^2}=\left(C^{\prime} x\right)^{-1}\)

⇒ \(\frac{\left(1+\frac{y^2}{x^2}\right)^4}{\left(1-\frac{y^2}{x^2}\right)^2}=\frac{1}{\left(C^{\prime}\right)^4 x^4}\)

⇒ \(\frac{\left(x^2+y^2\right)^4}{x^4\left(x^2-y^2\right)^2}=\frac{1}{\left(C^{\prime}\right)^4 x^4}\)

⇒ \(\left(x^2-y^2\right)^2=\left(C^{\prime}\right)^4\left(x^2+y^2\right)^4\)

⇒ \(\left(x^2-y^2\right)=\left(C^{\prime}\right)^2\left(x^2+y^2\right)^2\)

⇒ \(x^2-y^2=c\left(x^2+y^2\right)^2\), where c = \(\left(C^{\prime}\right)^2\)

Hence, the given result is proved.

Question 4. Find the general solution of the differential equation \(\frac{d y}{d x}+\sqrt{\frac{1-y^2}{1-x^2}}=0\)
Solution:

⇒ \(\frac{d y}{d x}+\sqrt{\frac{1-y^2}{1-x^2}}=0 \Rightarrow \frac{d y}{d x}=-\sqrt{\frac{1-y^2}{1-x^2}}\)

⇒ \(\frac{d y}{\sqrt{1-y^2}}=\frac{-d x}{\sqrt{1-x^2}} \Rightarrow \int \frac{d y}{\sqrt{1-y^2}}=\int \frac{-d x}{\sqrt{1-x^2}}\)

⇒ \(\sin ^{-1} y=-\sin ^{-1} x+C \Rightarrow \sin ^{-1} x+\sin ^{-1} y=C\)

Question 5. Show that the general solution of the differential equation \(\frac{d y}{d x}+\frac{y^2+y+1}{x^2+x+1}=0\) is given by (x+y+1)=A(1-x-y-2 x y), where A is a parameter
Solution:

⇒ \(\frac{d y}{d x}+\frac{y^2+y+1}{x^2+x+1}=0 \Rightarrow \frac{d y}{d x}=-\frac{\left(y^2+y+1\right)}{x^2+x+1}\)

⇒ \(\frac{d y}{y^2+y+1}=\frac{-d x}{x^2+x+1} \Rightarrow \frac{d y}{y^2+y+1}+\frac{d x}{x^2+x+1}=0\)

⇒ \(\int \frac{d y}{y^2+y+1}+\int \frac{d x}{x^2+x+1}=C\)

⇒ \(\int \frac{d y}{\left(y+\frac{1}{2}\right)^2+\left(\frac{\sqrt{3}}{2}\right)^2}+\int \frac{d x}{\left(x+\frac{1}{2}\right)^2+\left(\frac{\sqrt{3}}{2}\right)^2}=C\)

⇒ \(\frac{2}{\sqrt{3}} \tan ^{-1}\left[\frac{y+\frac{1}{2}}{\frac{\sqrt{3}}{2}}\right]+\frac{2}{\sqrt{3}} \tan ^{-1}\left[\frac{x+\frac{1}{2}}{\frac{\sqrt{3}}{2}}\right]=C\)

⇒ \(\tan ^{-1}\left[\frac{2 y+1}{\sqrt{3}}\right]+\tan ^{-1}\left[\frac{2 x+1}{\sqrt{3}}\right]=\frac{\sqrt{3 C}}{2}\)

⇒ \(\tan ^{-1}\left[\frac{\frac{2 y+1}{\sqrt{3}}+\frac{2 x+1}{\sqrt{3}}}{1-\frac{(2 y+1)}{\sqrt{3}} \frac{(2 x+1)}{\sqrt{3}}}\right]\)

= \(\frac{\sqrt{3} \mathrm{C}}{2}\)

⇒ \(\tan ^{-1}\left[\frac{\frac{2 x+2 y+2}{\sqrt{3}}}{1-\left(\frac{4 x y+2 x+2 y+1}{3}\right)}\right]=\frac{\sqrt{3} C}{2}\)

⇒ \(\tan ^{-1}\left[\frac{2 \sqrt{3}(x+y+1)}{3-4 x y-2 x-2 y-1}\right]=\frac{\sqrt{3} C}{2}\)

⇒ \(\tan ^{-1}\left[\frac{\sqrt{3}(x+y+1)}{2(1-x-y-2 x y)}\right]=\frac{\sqrt{3} C}{2}\)

⇒ \(\frac{\sqrt{3}(x+y+1)}{2(1-x-y-2 x y)}=\tan \left(\frac{\sqrt{3} C}{2}\right)=B\) (where B = \(\tan \frac{\sqrt{3} C}{2}\))

⇒ x+y+1 = \(\frac{2 B}{\sqrt{3}}(1-x-y-2 x y)\)

⇒ \(\mathrm{x}+\mathrm{y}+\mathrm{I}=\mathrm{A}(1-\mathrm{x}-\mathrm{y}-2 \mathrm{xy})\) (where \(\mathrm{A}=\frac{2 \mathrm{~B}}{\sqrt{3}}\))

Hence, the given result is proved.

Question 6. Find the equation of the curve passing through the point \(\left(0, \frac{\pi}{4}\right)\) whose differential equation is, \(\sin x \cos y \mathrm{dx}+\cos \mathrm{x} \sin \mathrm{y} \mathrm{dy}=0\)
Solution:

The differential equation of the given curve is: sin x cos y d x+cos x sin y d y=0

⇒ \(\frac{\sin x \cos y d x+\cos x \sin y d y}{\cos x \cos y}=0 \Rightarrow \tan x d x+\tan y d y=0\)

Integrating both sides, we get \(\int \tan x d x+\int \tan y d y=\log C\)

⇒ log (sec x)+log (sec y)=log C

⇒ log (sec x sec y)=log C

⇒ sec x sec y=C….(1)

The curve passes through point \(\left(0, \frac{\pi}{4}\right)\)

∴ \(1 \times \sqrt{2}=\mathrm{C} \Rightarrow \mathrm{C}=\sqrt{2}\)

On substituting \(\mathrm{C}=\sqrt{2}\) in equation (1), we get:

⇒ \(\sec \mathrm{x} \cdot \sec \mathrm{y}=\sqrt{2}\)

⇒ \(\sec x \cdot \frac{1}{\cos y}=\sqrt{2} \Rightarrow \cos y=\frac{\sec x}{\sqrt{2}}\)

Hence, the required equation of the curve is \(\cos y=\frac{\sec x}{\sqrt{2}}\)

Question 7. Find the particular solution of the differential equation \(\left(1+e^{2 x}\right) d y+\left(1+y^2\right) e^x d x=0\), given that y=1 when x=0
Solution:

⇒ \(\left(1+e^{2 x}\right) d y+\left(1+y^2\right) e^x d x=0\)

⇒ \(\frac{d y}{1+y^2}+\frac{e^x d x}{1+e^{2 x}}=0 \Rightarrow \int \frac{d y}{1+y^2}+\int \frac{e^x d x}{1+e^{2 x}}=C\)

⇒ \(\tan ^{-1} y+\int \frac{e^x d x}{1+e^{2 x}}=C\)…..(1)

Let \(\mathrm{e}^{\mathrm{x}}=\mathrm{t} \Rightarrow \mathrm{e}^{2 \mathrm{x}}=\mathrm{t}^2\)

⇒ \(\frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{e}^{\mathrm{x}}\right)=\frac{\mathrm{dt}}{\mathrm{dx}}\)

⇒ \(\mathrm{e}^{\mathrm{x}}=\frac{\mathrm{dt}}{\mathrm{dx}} \Rightarrow \mathrm{e}^{\mathrm{x}} \mathrm{dx}=\mathrm{dt}\)

Substituting these values in equation (1), we get: \(\tan ^{-1} \mathrm{y}+\int \frac{\mathrm{dt}}{1+\mathrm{t}^2}=\mathrm{C}\)

⇒ \(\tan ^{-1} \mathrm{y}+\tan ^{-1} \mathrm{t}=\mathrm{C} \Rightarrow \tan ^{-1} \mathrm{y}+\tan ^{-1}\left(\mathrm{e}^{\mathrm{x}}\right)=\mathrm{C}\)….(2)

Now, y=1 at x=0.

Therefore, equation (2) becomes: \(\tan ^{-1} 1+\tan ^{-1} 1=\mathrm{C} \Rightarrow \frac{\pi}{4}+\frac{\pi}{4}=\mathrm{C} \Rightarrow \mathrm{C}=\frac{\pi}{2}\)

Substituting \(C=\frac{\pi}{2}\) in equation (2), we get: \(\tan ^{-1} y+\tan ^{-1}\left(e^x\right)=\frac{\pi}{2}\)

This is the required particular solution of the given differential equation.

Question 8. Solve the differential equation \(y e^{\frac{x}{y}} d x=\left(x e^{\frac{x}{y}}+y^2\right) d y(y \neq 0)\)
Solution:

⇒ \(y e^{\frac{x}{y}} d x=\left(x e^{\frac{x}{y}}+y^2\right) d y \Rightarrow y e^{\frac{x}{y}} \frac{d x}{d y}=x e^{\frac{x}{y}}+y^2\)

⇒ \(e^{\frac{x}{y}}\left[y \cdot \frac{d x}{d y}-x\right]=y^2\)…..(1)

Let \(\mathrm{e}^{\frac{\mathrm{x}}{y}}=\mathrm{z}\)

Differentiating it with respect to y, we get:

⇒ \(\frac{d}{d y}\left(e^{\frac{x}{y}}\right)=\frac{d z}{d y} \Rightarrow e^{\frac{x}{y}} \cdot \frac{d}{d y}\left(\frac{x}{y}\right)=\frac{d z}{d y}\)

⇒ \(e^{\frac{x}{y}} \cdot \frac{\left.y \cdot \frac{d x}{d y}-x\right]}{y^2}=\frac{d z}{d y}\)…..(2)

From equation (1) and equation (2), we get : \(\frac{\mathrm{dz}}{\mathrm{dy}}=1 \Rightarrow \mathrm{dz}=\mathrm{dy}\)

Integrating both sides, we get : \(\int d z=\int d y \Rightarrow z=y+C \Rightarrow e^{\frac{x}{y}}=y+C\)

Question 9. Find a particular solution of the differential equation (x-y)(d x+d y)=d x-d y, given that y=-1, when x=0(Hint : put x-y=t)
Solution:

(x-y)(d x+d y)=d x-d y

⇒ (x-y+1) d y=(1-x+y) d x

⇒ \(\frac{d y}{d x}=\frac{1-x+y}{x-y+1} \quad \Rightarrow \frac{d y}{d x}=\frac{1-(x-y)}{1+(x-y)}\)

Let x-y=t

⇒ \(\frac{d}{d x}(x-y)=\frac{d t}{d x} \Rightarrow 1-\frac{d y}{d x}=\frac{d t}{d x}\)….(1)

⇒ \(1-\frac{d t}{d x}=\frac{d y}{d x}\)

Substituting the values of x-y and \(\frac{d y}{d x}\) in equation (1), we get :

⇒ \(1-\frac{d t}{d x}=\frac{1-t}{1+t}\)

⇒ \(\frac{d t}{d x}=1-\left(\frac{1-t}{1+t}\right) \Rightarrow \frac{d t}{d x}=\frac{(1+t)-(1-t)}{1+t} \Rightarrow \frac{d t}{d x}=\frac{2 t}{1+t}\)

⇒ \(\left(\frac{1+t}{t}\right) d t=2 d x \Rightarrow\left(1+\frac{1}{t}\right) d t=2 d x\)

Integrating both sides, we get: \(\int\left(1+\frac{1}{t}\right) d t=2 \int 1 \cdot d x\)

t + \(\log |t|=2 x+C \Rightarrow(x-y)+\log |x-y|=2 x+C\)

⇒ log |x-y|=x+y+C…..(2)

Now, y=-1 at x=0

Therefore, equation (2) becomes: log 1=0-1+C

⇒ C=1

Substituting C=1 in equation (2) we get: log |x-y|=x+y+1

This is the required particular solution of the given differential equation.

Question 10. Solve the differential equation \(\left[\frac{e^{-2 \sqrt{x}}}{\sqrt{x}}-\frac{y}{\sqrt{x}}\right] \frac{d x}{d y}=1(x \neq 0)\)
Solution:

⇒ \(\left[\frac{e^{-2 \sqrt{x}}}{\sqrt{x}}-\frac{y}{\sqrt{x}}\right] \frac{d x}{d y}=1 \Rightarrow \frac{d y}{d x}\)

= \(\frac{e^{-2 \sqrt{x}}}{\sqrt{x}}-\frac{y}{\sqrt{x}} \Rightarrow \frac{d y}{d x}+\frac{y}{\sqrt{x}}=\frac{e^{-2 \sqrt{x}}}{\sqrt{x}}\)

This equation is a linear differential equation of the form \(\frac{d y}{d x}+P y=Q\), where P = \(\frac{1}{\sqrt{x}}\) and Q = \(\frac{e^{-2 \sqrt{x}}}{\sqrt{x}}\)

Now, I.F. = \(\mathrm{e}^{\int \mathrm{Pdx}}=\mathrm{e}^{\int \frac{1}{\sqrt{x}} \mathrm{dx}}=\mathrm{e}^{2 \sqrt{x}}\)

The general solution of the given differential equation is given by,

y(I.F.) = \(\int(\text { Q } \times \text { I.F. }) \mathrm{dx}+\mathrm{C}\)

⇒ \(\mathrm{ye}^{2 \sqrt{x}}=\int\left(\frac{\mathrm{e}^{-2 \sqrt{x}}}{\sqrt{\mathrm{x}}} \times \mathrm{e}^{2 \sqrt{\mathrm{x}}}\right) \mathrm{dx}+\mathrm{C}\)

⇒ \(\mathrm{ye}^{2 \sqrt{\mathrm{x}}}=\int \frac{1}{\sqrt{\mathrm{x}}} \mathrm{dx}+\mathrm{C} \Rightarrow \mathrm{ye}^{2 \sqrt{x}}=2 \sqrt{\mathrm{x}}+\mathrm{C}\)

Question 11. Find a particular solution of the differential equation \(\frac{d y}{d x}+y \cot x=4 x \mathrm{cosec} x(x \neq 0)\), given that y=0 where \(x=\frac{\pi}{2}\).
Solution:

The given differential equation is : \(\frac{d y}{d x}+y \cot x=4 x \mathrm{cosec} x\)

This equation is a linear differential equation of the form \(\frac{d y}{d x}+P y=0\), where P = cot x and Q = 4x cosec x

Now, I.F, \(=e^{\int P d x}=e^{\int \cot x d x}=e^{\log \sin x x}=\sin x\)

The general solution of the given differential equation is given by, \(y(\mathrm{I} . \mathrm{F} .)=\int(\mathrm{Q} \times \mathrm{I} . \mathrm{F} .) \mathrm{dx}+\mathrm{C}\)

⇒ \(\mathrm{y} \sin \mathrm{x}=\int(4 \mathrm{x} \mathrm{cosec} \mathrm{x} \cdot \sin \mathrm{x}) \mathrm{dx}+\mathrm{C}\)

⇒ \(\mathrm{y} \sin \mathrm{x}=4 \int \mathrm{xdx}+\mathrm{C}\)

⇒ \(\mathrm{y} \sin \mathrm{x}=4 \cdot \frac{\mathrm{x}^2}{2}+\mathrm{C} \Rightarrow \mathrm{y} \sin \mathrm{x}=2 \mathrm{x}^2+\mathrm{C}\)…..(1)

Now, y=0 at x = \(\frac{\pi}{2}\)

Therefore, equation (1) becomes : 0 = \(2 \times \frac{\pi^2}{4}+\mathrm{C} \Rightarrow \mathrm{C}=-\frac{\pi^2}{2}\)

Substituting C = \(-\frac{\pi^2}{2}\) in equation (1), we get: \(y \sin x=2 x^2-\frac{\pi^2}{2}\)

This is the required particular solution of the given differential equation.

Question 12. Find a particular solution of the differential equation \((x+1) \frac{d y}{d x}=2 e^{-y}-1\), given that y=0 when x=0
Solution:

(x+1) \(\frac{d y}{d x}=2 e^{-y}-1 \Rightarrow \frac{d y}{2 e^{-y}-1}=\frac{d x}{x+1} \Rightarrow \frac{e^y d y}{2-e^y}=\frac{d x}{x+1}\)

Integrating both sides, we get : \(\int \frac{e^y d y}{2-e^y}=\int \frac{d x}{x+1}\)

⇒ \(\int \frac{\mathrm{e}^y \mathrm{dy}}{2-\mathrm{e}^y}=\log |\mathrm{x}+1|+\log \mathrm{C}\)…..(1)

Let \(2-e^{y}=\mathrm{t} \Rightarrow e^y d y=-d t\)

Substituting this value in equation (1), we get : \(-\int \frac{\mathrm{dt}}{\mathrm{t}}=\log |\mathrm{x}+1|+\log \mathrm{C}\)

⇒ \(-\log |\mathrm{t}|=\log |\mathrm{C}(\mathrm{x}+1)| \Rightarrow-\log \left|2-\mathrm{e}^{\mathrm{y}}\right|\)

= \(\log |\mathrm{C}(\mathrm{x}+1)| \Rightarrow \frac{1}{2-\mathrm{e}^{\mathrm{y}}}=\mathrm{C}(\mathrm{x}+1)\)

⇒ \(2-\mathrm{e}^{\mathrm{y}}=\frac{1}{\mathrm{C}(\mathrm{x}+1)}\)……(2)

Now, at x=0 and y=0, equation (2) becomes : 2-1 = \(\frac{1}{C}\)

C=1

Substituting C=1 in equation (2), we get : \(2-e^y=\frac{1}{x+1}\)

⇒ \(e^y=2-\frac{1}{x+1} \Rightarrow e^y=\frac{2 x+2-1}{x+1} \Rightarrow e^y=\frac{2 x+1}{x+1}\)

⇒ \(y=\log \left|\frac{2 x+1}{x+1}\right|,(x \neq-1)\)

This is the required particular solution of the given differential equation.

Choose The Correct Answer

Question 13. The general solution of the differential equation \(\frac{y d x-x d y}{y}=0\) is

1. xy=C
2. \(x=C y^2\)
3. y=Cx
4. \(\mathrm{y}=\mathrm{Cx}^2\)

Solution: 3. y=Cx

The given differential equation is: \(\frac{y \mathrm{dx}-\mathrm{x} d \mathrm{y}}{\mathrm{y}}=0\)

⇒ \(\frac{1}{\mathrm{x}} \mathrm{dx}-\frac{1}{\mathrm{y}} \mathrm{dy}=0\)

Integrating both sides, we get : \(\int \frac{1}{x} d x-\int \frac{1}{y} d y=0 \Rightarrow \log |x|-\log |y|=\log k \Rightarrow \log \left|\frac{x}{y}\right|=\log k\)

⇒ \(\frac{x}{y}=k \Rightarrow y=\frac{1}{k} x \Rightarrow y=C x\) where c = \(\frac{1}{k}\)

Hence, the correct answer is (3).

Question 14. The general solution of a differential equation of the type \(\frac{d x}{d y}+P_1 x=Q_1\) is

1. \(y \mathrm{e}^{\int P_1 d y}=\int\left(Q_1 e^{\int P_1 d y}\right) d y+C\)
2. \(y \cdot e^{\int P_1 d x}=\int\left(Q_1 e^{\int P_1{d x}}\right) d y+C\)
3. \(x e^{\int P_1 d y}=\int\left(Q_1 e^{\int P_1 d y}\right) d y+C\)
4. \(x e^{\int P_1 d x}=\int\left(Q_1 e^{\int P_1 d x}\right) d y+C\)

Solution: 3. \(x e^{\int P_1 d y}=\int\left(Q_1 e^{\int P_1 d y}\right) d y+C\)

The integrating factor of the given differential equation \(\frac{d x}{d y}+P_1 x=Q_1\) is \(e^{\int P_1 d y}\).

The general solution of the differential equation is given by,

x(I .F.) = \(\int\left(Q_1 \times \text { I.F. }\right) d y+C \Rightarrow x \cdot e^{\int P_1 d y}=\int\left(Q_1 e^{\int P_1 d y}\right) d y+C\)

Hence, the correct answer is (3).

Question 15. The general solution of the differential equation \(e^x d y+\left(y e^x+2 x\right) d x=0\) is

1. \(x e^y+x^2=C\)
2. \(x e^y+y^2=C\)
3. \(y \mathrm{e}^{\mathrm{x}}+\mathrm{x}^2=\mathrm{C}\)
4. \(\mathrm{ye}^y+\mathrm{x}^2=\mathrm{C}\)

Solution: 3. \(y \mathrm{e}^{\mathrm{x}}+\mathrm{x}^2=\mathrm{C}\)

The given differential equation is: \(e^x d y+\left(y e^x+2 x\right) d x=0\)

⇒ \(e^x \frac{d y}{d x}+y e^x+2 x=0 \quad \Rightarrow \quad \frac{d y}{d x}+y=-2 x e^{-x}\)

This is a linear differential equation of the form

⇒ \(\frac{\mathrm{dy}}{\mathrm{dx}}+\mathrm{Py}=\mathrm{Q}\) where P =1 and Q=-2 \(\mathrm{x} \mathrm{e}^{-\mathrm{t}}\)

Now, I.F. = \(\mathrm{e}^{\int P d x}=\mathrm{e}^{\int d x}=\mathrm{e}^\pi\)

The general solution of the given differential equation is given by, \(y(\text { I.F. })=\int(\mathrm{Q} \times \text { I.F. }) \mathrm{dx}+C\)

⇒ \(\mathrm{ye}^{\mathrm{x}}=\int\left(-2 \mathrm{xe}^{-\mathrm{x}} \cdot \mathrm{e}^{\mathrm{x}}\right) \mathrm{dx}+\mathrm{C}\)

⇒ \(\mathrm{ye}^{\mathrm{x}}=-\int 2 \mathrm{xdx}+\mathrm{C}\)

⇒ \(\mathrm{ye}^{\mathrm{x}}=-\mathrm{x}^2+\mathrm{C}\)

⇒ \(\mathrm{ye}^{\mathrm{x}}+\mathrm{x}^2=\mathrm{C}\)

Hence, the correct answer is 3.

 

 

 

 

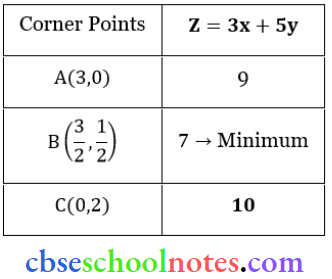
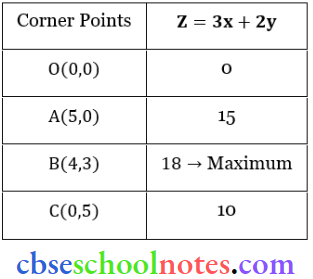
Integrals Exercise 7.1

Find An antiderivative (Or Integral) Of the following function by the method of inspection.

Question 1. sin2x
Solution:

The anti-derivate of sin2x is a function of x whose derivative is sin2x. It is known that,

∵ \(\frac{d}{d x}(\cos 2 x)=-2 \sin 2 x \Rightarrow-\frac{1}{2} \frac{d}{d x}(\cos 2 x)=\sin 2 x \Rightarrow \frac{d}{d x}\left(-\frac{1}{2} \cos 2 x\right)=\sin 2 x\)

Therefore, the anti-derivates of sin2x is –\(\frac{1}{2}\) cos2x

Question 2. cos 3x
Solution:

The anti-derviative of cos 3x is a function of x whose derivative is cos 3x.

∵ \(\frac{d}{d x}(\sin 3 x)=3 \cos 3 x \Rightarrow \frac{1}{3} \frac{d}{d x}(\sin 3 x)=\cos 3 x \Rightarrow \frac{d}{d x}\left(\frac{1}{3} \sin 3 x\right)=\cos 3 x\)

Therefore, the anti-derivates of sos 3x is –\(\frac{1}{3}\) sin 3x.

Question 3. e^2x
Solution:

The anti-derviative of cos 3x is a function of x whose derivative is cos 3x.

∵ \(\frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{e}^{2 \mathrm{x}}\right)=2 \mathrm{e}^{2 \mathrm{x}} \Rightarrow \frac{1}{2} \frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{e}^{2 \mathrm{x}}\right)=\mathrm{e}^{2 \mathrm{x}} \Rightarrow \frac{\mathrm{d}}{\mathrm{dx}}\left(\frac{1}{2} \mathrm{e}^{2 \mathrm{x}}\right)=\mathrm{e}^{2 \mathrm{x}}\)

Therefore, the anti-derivates of e^2x is –\(\frac{1}{2}\) e^2x.

Question 4. (ax + b)²
Solution:

The anti-derviative of (ax+b)² is a function of x whose derivative is (ax+b)².

It is known that,

∵ \(\frac{d}{d x}(a x+b)^3=3 a(a x+b)^2 \Rightarrow \frac{1}{3 a} \frac{d}{d x}(a x+b)^3\)

= \((a x+b)^2 \Rightarrow \frac{d}{d x}\left(\frac{1}{3 a}(a x+b)^3\right)=(a x+b)^2\)

Therefore, the anti-derivates of (ax+b) is –\(\frac{1}{3a}\) (ax+b).

Question 5. sin 2x – 4e^3x
Solution:

The anti-derivative of (sin 2x – 4e^3x) is a function of x whose derivative is (sin 2x – 4e^3x).

∵ \(\frac{d}{d x}(\cos 2 x)=-2 \sin 2 x \Rightarrow-\frac{1}{2} \frac{d}{d x}(\cos 2 x)=\sin 2 x \Rightarrow \frac{d}{d x}\left(-\frac{1}{2} \cos 2 x\right)=\sin 2 x\)

Similarly, \(\frac{d}{d x}\left(\frac{4}{3} e^{3 x}\right)=4 e^{3 x}\)

∵ \(\frac{d}{d x}\left(-\frac{1}{2} \cos 2 x\right)-\frac{d}{d x}\left(\frac{4}{3} e^{3 x}\right)=\sin 2 x-4 e^{3 x} \Rightarrow \frac{d}{d x}\left(-\frac{1}{2} \cos 2 x-\frac{4}{3} e^{3 x}\right)=\sin 2 x-4 e^{3 x}\)

Therefore, the antiderivative of \(\left(\sin 2 x-4 e^{3 x}\right)\) is \(\left(-\frac{1}{2} \cos 2 x-\frac{4}{3} e^{3 x}\right)\)

Find The Following Integrals

Question 6. \(\int\left(4 e^{3 x}+1\right) d x\)
Solution:

Let \(\mathrm{I}=\int\left(4 \mathrm{e}^{3 \mathrm{x}}+1\right) \mathrm{dx}\)

= \(4 \int e^{3 x} d x+\int 1 d x=4\left(\frac{e^{3 x}}{3}\right)+x+C=\frac{4}{3} e^{3 x}+x+C\)

Question 7. \(\int x^2\left(1-\frac{1}{x^2}\right) d x\)
Solution:

⇒ \(\int x^2\left(1-\frac{1}{x^2}\right) d x=\int\left(x^2-1\right) d x=\int x^2 d x-\int 1 d x=\frac{x^3}{3}-x+C\)

Read and Learn More Class 12 Maths Chapter Wise with Solutions

Question 8. \(\int\left(a x^2+b x+c\right) d x\)
Solution:

Let \(I=\int\left(a x^2+b x+c\right) d x=a \int x^2 d x+b \int x d x+c \int 1 . d x\)

= \(a\left(\frac{x^3}{3}\right)+b\left(\frac{x^2}{2}\right)+c x+C=\frac{a x^3}{3}+\frac{b x^2}{2}+c x+C\)

Question 9. \(\int\left(2 x^2+e^x\right) d x\)
Solution:

Let \(\mathrm{I}=\int\left(2 \mathrm{x}^2+\mathrm{e}^{\mathrm{x}}\right) \mathrm{dx}=2 \int \mathrm{x}^2 \mathrm{dx}+\int \mathrm{e}^{\mathrm{x}} \mathrm{dx}=2\left(\frac{\mathrm{x}^3}{3}\right)+\mathrm{e}^{\mathrm{x}}+\mathrm{C}=\frac{2}{3} \mathrm{x}^3+\mathrm{e}^{\mathrm{x}}+\mathrm{C}\)

Question 10. \(\int\left(\sqrt{\mathrm{x}}-\frac{1}{\sqrt{\mathrm{x}}}\right)^2 \mathrm{dx}\)
Solution:

Let \(I=\int\left(\sqrt{x}-\frac{1}{\sqrt{x}}\right)^2 d x=\int\left(x+\frac{1}{x}-2\right) d x\)

= \(\int x d x+\int \frac{1}{x} d x-2 \int 1, d x=\frac{x^2}{2}+\log |x|-2 x+C\)

Question 11. \(\int \frac{x^3+5 x^2-4}{x^2} d x\)
Solution:

Let \(I=\int \frac{x^3+5 x^2-4}{x^2} d x=\int\left(x+5-4 x^{-2}\right) d x=\int x d x+5 \int 1 . d x-4 \int x^{-2} d x\)

= \(\frac{x^2}{2}+5 x-4\left(\frac{x^{-1}}{-1}\right)+C=\frac{x^2}{2}+5 x+\frac{4}{x}+C\)

Question 12. \(\int \frac{x^3+3 x+4}{\sqrt{x}} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{x}^3+3 \mathrm{x}+4}{\sqrt{\mathrm{x}}} \mathrm{dx}=\int\left(\mathrm{x}^{\frac{5}{2}}+3 \mathrm{x}^{\frac{1}{2}}+4 \mathrm{x}^{\frac{-1}{2}}\right) \mathrm{dx}\)

(because \(\int \mathrm{x}^{\mathrm{n}} \mathrm{dx}=\frac{\mathrm{x}^{\mathrm{n}+1}}{\mathrm{n}+1}+\mathrm{C}\))

= \(\frac{x^{\left(\frac{7}{2}\right)}}{\frac{7}{2}}+\frac{3\left(x^{\frac{3}{2}}\right)}{\frac{3}{2}}+\frac{4\left(x^{\frac{1}{2}}\right)}{\frac{1}{2}}+C=\frac{2}{7} x^{\frac{7}{2}}+2 x^{\frac{3}{2}}+8 x^{\frac{1}{2}}+C=\frac{2}{7} x^{\frac{7}{2}}+2 x^{\frac{3}{2}}+8 \sqrt{x}+C\)

Question 13. \(\int \frac{x^3-x^2+x-1}{x-1} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{x}^3-\mathrm{x}^2+\mathrm{x}-1}{\mathrm{x}-1} \mathrm{dx}\)

On dividing, we obtain

I = \(\int \frac{x^2(x-1)+1(x-1)}{x-1} d x=\int \frac{(x-1)\left(x^2+1\right)}{(x-1)} d x=\int x^2 d x+\int 1 \cdot d x=\frac{x^3}{3}+x+C\)

Question 14. \(\int(1-x) \sqrt{x} d x\)
Solution:

Let \(I=\int(1-x) \sqrt{x} d x=\int\left(\sqrt{x}-x^{\frac{3}{2}}\right) d x=\int x^{\frac{1}{2}} d x-\int x^{\frac{3}{2}} d x\)

=\(\frac{x^{\frac{3}{2}}}{3 / 2}-\frac{x^{\frac{5}{2}}}{5 / 2}+C=\frac{2}{3} x^{3 / 2}-\frac{2}{5} x^{5 / 2}+C\)

Question 15. \(\int \sqrt{\mathrm{x}}\left(3 \mathrm{x}^2+2 \mathrm{x}+3\right) \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \sqrt{\mathrm{x}}\left(3 \mathrm{x}^2+2 \mathrm{x}+3\right) \mathrm{dx}=\int\left(3 \mathrm{x}^{\frac{5}{2}}+2 \mathrm{x}^{\frac{3}{2}}+3 \mathrm{x}^{\frac{1}{2}}\right) \mathrm{dx}\)

= \(3 \int \mathrm{x}^{-\frac{5}{2}} \mathrm{dx}+2 \int \mathrm{x}^{\frac{3}{2}} \mathrm{dx}+3 \int \mathrm{x}^{\frac{1}{2}} d x\)

= \(3\left(\frac{x^{\frac{7}{2}}}{\frac{7}{2}}\right)+2\left(\frac{x^{\frac{5}{2}}}{\frac{5}{2}}\right)+3\left(\frac{x^{\frac{3}{2}}}{\frac{3}{2}}\right)+C=\frac{6}{7} x^{\frac{7}{2}}+\frac{4}{5} x^{\frac{5}{2}}+2 x^{\frac{3}{2}}+C\)

Question 16. \(\int\left(2 x-3 \cos x+e^x\right) d x\)
Solution:

Let \(I=\int\left(2 x-3 \cos x+e^x\right) d x=2 \int x d x-3 \int \cos x d x+\int e^x d x\)

= \(\frac{2 \mathrm{x}^2}{2}-3(\sin \mathrm{x})+\mathrm{e}^{\mathrm{x}}+\mathrm{C}=\mathrm{x}^2-3 \sin \mathrm{x}+\mathrm{e}^{\mathrm{x}}+\mathrm{C}\)

Question 17. \(\int\left(2 x^2-3 \sin x+5 \sqrt{x}\right) d x\)
Solution:

Let \(I=\int\left(2 x^2-3 \sin x+5 \sqrt{x}\right) d x=2 \int x^2 d x-3 \int \sin x d x+5 \int x^{\frac{1}{2}} d x\)

= \(\frac{2 x^3}{3}-3(-\cos x)+5\left(\frac{x^{\frac{3}{2}}}{\frac{3}{2}}\right)+C=\frac{2}{3} x^3+3 \cos x+\frac{10}{3} x^{\frac{3}{2}}+C\)

Question 18. \(\int \sec x(\sec x+\tan x) d x\)
Solution:

Let \(I=\int \sec x(\sec x+\tan x) d x=\int\left(\sec ^2 x+\sec x \tan x\right) d x\)

= \(\tan \mathrm{x}+\sec \mathrm{x}+\mathrm{C}\)

Question 19. \(\int \frac{\sec ^2 x}{\mathrm{cosec}^2 x} d x\)
Solution:

Let \(I=\int \frac{\sec ^2 x}{\mathrm{cosec}^2 x} d x=\int \frac{\frac{1}{\cos ^2 x}}{\frac{1}{\sin ^2 x}} d x=\int \frac{\sin ^2 x}{\cos ^2 x} d x\)

= \(\int \tan ^2 x d x=\int\left(\sec ^2 x-1\right) d x=\int \sec ^2 x d x-\int 1 d x=\tan x-x+C\)

Question 20. \(\int \frac{2-3 \sin x}{\cos ^2 x} d x\)
Solution:

Let I = \(\int \frac{2-3 \sin x}{\cos ^2 x} d x=\int\left(\frac{2}{\cos ^2 x}-\frac{3 \sin x}{\cos ^2 x}\right) d x\)

= \(\int 2 \sec ^2 x d x-3 \int \tan x \sec x d x=2 \tan x-3 \sec x+C\)

Choose The Correct Answer In The Following

Question 21. The anti derivative of \(\left(\sqrt{\mathrm{x}}+\frac{1}{\sqrt{\mathrm{x}}}\right)\) equals?

1. \(\frac{1}{3} \mathrm{x}^{\frac{1}{3}}+2 \mathrm{x}^{\frac{1}{2}}+C\)
2. \(\frac{2}{3} \mathrm{x}^{\frac{2}{3}}+\frac{1}{2} \mathrm{x}^2+\mathrm{C}\)
3. \(\frac{2}{3} \mathrm{x}^{\frac{3}{2}}+2 \mathrm{x}^{\frac{1}{2}}+\mathrm{C}\)
4. \(\frac{3}{2} \mathrm{x}^{\frac{3}{2}}+\frac{1}{2} \mathrm{x}^{\frac{1}{2}}+\mathrm{C}\)

Solution: 3. \(\frac{2}{3} \mathrm{x}^{\frac{3}{2}}+2 \mathrm{x}^{\frac{1}{2}}+\mathrm{C}\)

Let \(\mathrm{I}=\int\left(\sqrt{\mathrm{x}}+\frac{1}{\sqrt{\mathrm{x}}}\right) \mathrm{dx}=\int \mathrm{x}^{\frac{1}{2}} \mathrm{dx}+\int \mathrm{x}^{-\frac{1}{2}} \mathrm{dx}=\frac{\mathrm{x}^{\frac{3}{2}}}{3 / 2}+\frac{\mathrm{x}^{\frac{1}{2}}}{1 / 2}+\mathrm{C}=\frac{2}{3} \mathrm{x}^{\frac{3}{2}}+2 \mathrm{x}^{\frac{1}{2}}+\mathrm{C}\)

Hence, the correct Answer is 3.

Question 22. If \(\frac{\mathrm{d}}{\mathrm{dx}}(f(\mathrm{x}))=4 \mathrm{x}^3-\frac{3}{\mathrm{x}^4}\) such that f(2)=0, then f(x) is?

1. \(x^4+\frac{1}{x^3}-\frac{129}{8}\)
2. \(x^3+\frac{1}{x^4}+\frac{129}{8}\)
3. \(\mathrm{x}^4+\frac{1}{\mathrm{x}^3}+\frac{129}{8}\)
4. \(\mathrm{x}^3+\frac{1}{\mathrm{x}^4}-\frac{129}{8}\)

Solution: 1. \(x^4+\frac{1}{x^3}-\frac{129}{8}\)

It is given that, \(\frac{\mathrm{d}}{\mathrm{dx}}(f(\mathrm{x}))=4 \mathrm{x}^3-\frac{3}{\mathrm{x}^4}\)

∴ Integrating both sides with respect to x

∴ \(f(\mathrm{x})=\int\left(4 \mathrm{x}^3-\frac{3}{\mathrm{x}^4}\right) \mathrm{dx} \Rightarrow f(\mathrm{x})=4 \int \mathrm{x}^3 \mathrm{dx}-3 \int\left(\mathrm{x}^{-4}\right) \mathrm{dx}\)

⇒ \(f(\mathrm{x})=4\left(\frac{\mathrm{x}^4}{4}\right)-3\left(\frac{\mathrm{x}^{-3}}{-3}\right)+\mathrm{C}\)

∴ \(f(\mathrm{x})=\mathrm{x}^4+\frac{1}{\mathrm{x}^3}+\mathrm{C}\)

Also, f(2)=0

∴ \(f(2)=(2)^4+\frac{1}{(2)^3}+\mathrm{C}=0 \Rightarrow 16+\frac{1}{8}+\mathrm{C}=0 \Rightarrow \mathrm{C}=-\left(16+\frac{1}{8}\right) \Rightarrow \mathrm{C}=-\frac{129}{8}\)

Put the value of C in equation (1)

f(x) = \(x^4+\frac{1}{x^3}-\frac{129}{8} \text {. }\)

Hence, the correct answer is (1).

Integrals Exercise 7.2

IntegrateThe Functions

Question 1. \(\int \frac{2 \mathrm{x}}{1+\mathrm{x}^2} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \frac{2 \mathrm{x}}{1+\mathrm{x}^2} \mathrm{dx}\)

Put \(1+\mathrm{x}^2=\mathrm{t} \Rightarrow 2 \mathrm{xdx}=\mathrm{dt}\)

⇒ \(\mathrm{I}=\int_{\mathrm{t}}^1 \frac{1}{\mathrm{dt}}=\log |\mathrm{t}|+\mathrm{C}=\log \left|1+\mathrm{x}^2\right|+\mathrm{C}=\log \left(1+\mathrm{x}^2\right)+\mathrm{C}\)

Question 2. \(\int \frac{(\log |\mathrm{x}|)^2}{\mathrm{x}} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \frac{(\log |\mathrm{x}|)^2}{\mathrm{x}} \mathrm{dx}\)

Put \(\log |x|=t \Rightarrow \frac{1}{x} d x=d t \Rightarrow I=\int t^2 d t=\frac{t^3}{3}+C=\frac{(\log |x|)^3}{3}+C\)

Question 3. \(\int \frac{1}{x+x \log x} d x\)
Solution:

Let \(I=\int \frac{1}{x+x \log x} d x=\int \frac{1}{x(1+\log x)} d x\)

Put \(1+\log \mathrm{x}=\mathrm{t} \Rightarrow \frac{1}{\mathrm{x}} \mathrm{dx}=\mathrm{dt}\)

Question 4. \(\int \sin x \cdot \sin (\cos x) d x\)
Solution:

Let \(\mathrm{I}=\int \sin \mathrm{x}, \sin (\cos \mathrm{x}) \mathrm{dx}\)

Put \(\cos \mathrm{x}=\mathrm{t} \Rightarrow-\sin \mathrm{x} \mathrm{dx}=\mathrm{dt}\)

⇒ \(\mathrm{I}=-\int \sin \mathrm{tdt}=-[-\cos \mathrm{t}]+\mathrm{C}=\cos (\cos \mathrm{x})+\mathrm{C}\)

Question 5.\(\int \sin (a x+b) \cos (a x+b) d x\)
Solution:

Let \(\mathrm{I}=\int \sin (a \mathrm{ax}+\mathrm{b}) \cos (a \mathrm{ax}+\mathrm{b}) \mathrm{dx}=\frac{1}{2} \int \sin 2(a \mathrm{a}+\mathrm{b}) \mathrm{dx}\)

Put \(2(a x+b)=t \Rightarrow d x=\frac{d t}{2 a}\)

⇒ I = \(\frac{1}{2} \int \frac{\sin t d t}{2 a}=\frac{1}{4 a}[-\cos t]+C=\frac{-1}{4 a} \cos 2(a x+b)+C\)

Question 6. \(\int \sqrt{a x+b} d x\)
Solution:

Let \(I=\int \sqrt{a x+b} d x\)

Put \(a x+b=t^2 \Rightarrow a d x=2 t d t\)

∴ I = \(\int t \cdot \frac{2 t}{a} d t=\frac{2}{a} \int t^2 d t=\frac{2}{a} \cdot \frac{t^3}{3}+C=\frac{2}{3 a}(a x+b)^{3 / 2}+C\)

Question 7. \(\int x \sqrt{x+2} d x\)
Solution:

Let \(\mathrm{I}=\int \mathrm{x} \sqrt{\mathrm{x}+2} \mathrm{dx}\)

Put \(x+2=t^2 \Rightarrow d x=2 t d t\)

∴ \(\mathrm{I}=\int\left(\mathrm{t}^2-2\right) \cdot \mathrm{t} \cdot 2 \mathrm{tdt}=\int\left(2 \mathrm{t}^4-4 \mathrm{t}^2\right) \mathrm{dt}\)

= \(\frac{2}{5} \mathrm{t}^5-\frac{4}{3} \mathrm{t}^3+\mathrm{C}=\frac{2}{5}(\mathrm{x}+2)^{5 / 2}-\frac{4}{3}(\mathrm{x}+2)^{3 / 2}+\mathrm{C}\)

Question 8. \(\int x \sqrt{1+2 x^2} d x\)
Solution:

Let \(\mathrm{I}=\int \mathrm{x} \sqrt{1+2 \mathrm{x}^2} \mathrm{dx}\)

Put \(1+2 x^2=t^2 \Rightarrow 4 x d x=2 t d t \Rightarrow x d x=\frac{t}{2} d t\)

∴ I = \(\int t \cdot \frac{t}{2} d t=\frac{1}{2} \int t^2 d t=\frac{1}{6} t^3+C=\frac{1}{6}\left(1+2 x^2\right)^{3 / 2}+C\)

Question 9. \(\int(4 x+2) \sqrt{x^2+x+1} d x\)
Solution:

Let \(\mathrm{I}=\int(4 \mathrm{x}+2) \sqrt{\mathrm{x}^2+\mathrm{x}+1} \mathrm{dx}\)

Put \(x^2+x+1=t^2 \Rightarrow(2 x+1) d x=2 t d t\)

⇒ \(\mathrm{I}=2 \int \mathrm{t} \cdot 2 \mathrm{tdt}=4 \int \mathrm{t}^2 \mathrm{dt}=\frac{4}{3} \mathrm{t}^3+\mathrm{C}=\frac{4}{3}\left(\mathrm{x}^2+\mathrm{x}+1\right)^{3 / 2}+\mathrm{C}\)

Question 10. \(\int \frac{1}{x-\sqrt{x}} d x\)
Solution:

Let \(I=\int \frac{1}{x-\sqrt{x}} d x=\int \frac{1}{\sqrt{x}(\sqrt{x}-1)} d x\)

Put \((\sqrt{\mathrm{x}}-1)=\mathrm{t} \Rightarrow \frac{1}{2 \sqrt{\mathrm{x}}} \mathrm{dx}=\mathrm{dt} \Rightarrow \frac{1}{\sqrt{\mathrm{x}}} \mathrm{dx}=2 \mathrm{dt}\)

∴ I = \(\int \frac{1}{t} \cdot 2 d t=2 \log |t|+C=2 \log |\sqrt{x}-1|+C\)

Question 11. \(\int \frac{\mathrm{x}}{\sqrt{\mathrm{x}+4}} \cdot \mathrm{dx}, \mathrm{x}>0\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{x}}{\sqrt{\mathrm{x}+4}} \cdot \mathrm{dx}, \mathrm{x}>0\)

Put \(x+4=t^2 \Rightarrow x=t^2-4 \Rightarrow d x=2 t d t\)

∴ I = \(\int \frac{\left(t^2-4\right)}{t} \cdot 2 t d t=2 \int\left(t^2-4\right) d t=2\left[\frac{t^3}{3}-4 t\right]+C\)

= \(\frac{2}{3} t\left[t^2-12\right]+C=\frac{2}{3} \sqrt{x+4}(x-8)+C\)

Question 12. \(\int\left(x^3-1\right)^{\frac{1}{3}} x^5 d x\)
Solution:

Let \(I=\int\left(x^3-1\right)^{\frac{1}{3}} x^5 d x\)

I = \(\int\left(x^3-1\right)^{1 / 3} \cdot x^3 \cdot x^2 d x\)

Put \(x^3-1=t^3 \Rightarrow x^3=t^3+1 \Rightarrow x^2 d x=t^2 d t\)

∴ I = \(\int t \cdot\left(t^3+1\right) \cdot t^2 d t=\int\left(t^6+t^3\right) d t=\frac{t^7}{7}+\frac{t^4}{4}+C=\frac{1}{7}\left(x^3-1\right)^{7 / 3}+\frac{1}{4}\left(x^3-1\right)^{4 / 3}+C\)

Question 13. \(\int \frac{x^2}{\left(2+3 x^3\right)^3} d x\)
Solution:

Let \(I=\int \frac{x^2}{\left(2+3 x^3\right)^3} d x\)

Put \(2+3 x^3=t \Rightarrow 9 x^2 d x=d t\)

∴ \(\mathrm{I}=\int \frac{1}{\mathrm{t}^3}, \frac{\mathrm{dt}}{9}=\frac{1}{9} \int \frac{1}{\mathrm{t}^3} \mathrm{dt}=\frac{1}{9}\left[\frac{\mathrm{t}^{-2}}{-2}\right]+\mathrm{C}=-\frac{1}{18}\left[\frac{1}{\mathrm{t}^2}\right]+\mathrm{C}=-\frac{1}{18\left(2+3 \mathrm{x}^3\right)^2}+\mathrm{C}\)

Question 14. \(\int \frac{1}{x(\log x)^m} d x, x>0, m \neq 1\)
Solution:

Let \(\mathrm{I}=\int \frac{1}{\mathrm{x}(\log \mathrm{x})^{\mathrm{m}}} \mathrm{dx}\)

Put \(\log \mathrm{x}=\mathrm{t} \Rightarrow \frac{1}{\mathrm{x}} \mathrm{dx}=\mathrm{dt}\)

⇒ \(\mathrm{I}=\int \frac{\mathrm{dt}}{(\mathrm{t})^{\mathrm{m}}}=\int \mathrm{t}^{-\mathrm{m}} \mathrm{dt}=\left(\frac{\mathrm{t}^{1-\mathrm{m}}}{1-\mathrm{m}}\right)+\mathrm{C}=\frac{(\log \mathrm{x})^{1-\mathrm{m}}}{(1-\mathrm{m})}+\mathrm{C}\)

Question 15. \(\int \frac{x}{9-4 x^2} d x\)
Solution:

Let \(I=\int \frac{x}{9-4 x^2} d x\)

Put \(9-4 \mathrm{x}^2=\mathrm{t} \Rightarrow-8 \mathrm{xdx}=\mathrm{dt}\)

∴ I = \(\frac{-1}{8} \int_t^1 \frac{1}{t}=\frac{-1}{8} \log |t|+C=\frac{-1}{8} \log \left|9-4 x^2\right|+C\)

Question 16. \(\int \mathrm{e}^{2 \mathrm{x}+3} \mathrm{dx}\)
Solution:

Let \(I=\int e^{2 x+3} d x\)

Put \(2 \mathrm{x}+3=\mathrm{t} \Rightarrow \mathrm{dx}=\frac{\mathrm{dt}}{2}\)

∴ \(\mathrm{I}=\frac{1}{2} \int \mathrm{e}^{\mathrm{t}} \mathrm{dt}=\frac{1}{2}\left(\mathrm{e}^{\mathrm{t}}\right)+\mathrm{C}=\frac{1}{2} \mathrm{e}^{(2 \mathrm{x}+3)}+\mathrm{C}\)

Question 17. \(\int \frac{\mathrm{x}}{\mathrm{e}^{\mathrm{x}^2}} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{x}}{\mathrm{e}^{\mathrm{x}^2}} \mathrm{dx}\)

Put \(\mathrm{x}^2=\mathrm{t} \Rightarrow 2 \mathrm{xdx}=\mathrm{dt}\)

∴ \(\mathrm{I}=\frac{1}{2} \int \frac{1}{\mathrm{e}^{\mathrm{t}}} \mathrm{dt}=\frac{1}{2} \int \mathrm{e}^{-t} \mathrm{dt}=\frac{1}{2}\left(\frac{\mathrm{e}^{-1}}{-1}\right)+\mathrm{C}=-\frac{1}{2} \mathrm{e}^{-\mathrm{x}^2}+\mathrm{C}=\frac{-1}{2 \mathrm{e}^{\mathrm{x}^2}}+\mathrm{C}\)

Question 18. \(\int \frac{e^{\sin -1 x}}{1+x^2} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{e}^{\mathrm{lin}^{-1} \mathrm{x}}}{1+\mathrm{x}^2} \mathrm{dx}\)

Put \(\tan ^{-1} \mathrm{x}=\mathrm{t} \Rightarrow \frac{1}{1+\mathrm{x}^2} \mathrm{dx}=\mathrm{dt}\)

∴ \(\mathrm{I}=\int \mathrm{e}^t \mathrm{dt}=\mathrm{e}^{\mathrm{t}}+\mathrm{C}=\mathrm{e}^{\tan ^{-1} \mathrm{x}}+\mathrm{C}
\)

Question 19. \(\int \frac{\mathrm{e}^{2 \mathrm{x}}-1}{\mathrm{e}^{2 \mathrm{x}}+1} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{e}^{2 \mathrm{x}}-1}{\mathrm{e}^{2 \mathrm{x}}+1} \mathrm{dx} \Rightarrow \mathrm{I}=\int \frac{\mathrm{e}^{\mathrm{x}}-\mathrm{e}^{-\mathrm{x}}}{\mathrm{e}^{\mathrm{x}}+\mathrm{e}^{-\mathrm{x}}} \mathrm{dx}\)

Dividing numerator and denominator by \(\mathrm{e}^{\mathrm{x}}\)

Put \(\mathrm{e}^{\mathrm{x}}+\mathrm{e}^{-\mathrm{x}}=\mathrm{t} \Rightarrow\left(\mathrm{e}^{\mathrm{x}}-\mathrm{e}^{-x}\right) \mathrm{dx}=\mathrm{dt}\)

∴ \(I=\int \frac{d t}{t}=\log |t|+C=\log \left|e^x+e^{-x}\right|+C\)

Question 20. \(\int \frac{e^{2 x}-e^{-2 x}}{e^{2 x}+e^{-2 x}} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{e}^{2 \mathrm{x}}-\mathrm{e}^{-2 \mathrm{x}}}{\mathrm{e}^{2 \mathrm{x}}+\mathrm{e}^{-2 \mathrm{x}}} \mathrm{dx}\)

Put \(\mathrm{e}^{2 \mathrm{x}}+\mathrm{e}^{-2 \mathrm{x}}=\mathrm{t} \Rightarrow\left(2 \mathrm{e}^{2 \mathrm{x}}-2 \mathrm{e}^{-2 \mathrm{x}}\right) \mathrm{dx}=\mathrm{dt} \Rightarrow\left(\mathrm{e}^{2 \mathrm{x}}-\mathrm{e}^{-2 \mathrm{x}}\right) \mathrm{dx}=\frac{\mathrm{dt}}{2}\)

∴ \(\mathrm{I}=\frac{1}{2} \int_{\mathrm{t}}^1 \mathrm{dt}=\frac{1}{2} \log |\mathrm{t}|+\mathrm{C}=\frac{1}{2} \log \left|\mathrm{e}^{2 \mathrm{x}}+\mathrm{e}^{-2 \mathrm{x}}\right|+\mathrm{C}\)

Question 21. \(\int \tan ^2(2 x-3) d x\)
Solution:

Let \(\mathrm{I}=\int \tan ^2(2 \mathrm{x}-3) \mathrm{dx}\)

Put \(2 \mathrm{x}-3=\mathrm{t} \Rightarrow \mathrm{dx}=\frac{\mathrm{dt}}{2}\)

∴ I = \(\frac{1}{2} \int \tan ^2 t \cdot d t=\frac{1}{2} \int\left(\sec ^2 t-1\right) d t\)

= \(\frac{1}{2}[\tan t-t]+C_1=\frac{1}{2}[\tan (2 x-3)-(2 x-3)]+C_1\)

= \(\frac{1}{2} \tan (2 x-3)-x+\frac{3}{2}+C_1=\frac{1}{2} \tan (2 x-3)-x+C \text {; where } C_1=C_1+\frac{3}{2}\)

Question 22. \(\int \sec ^2(7-4 x) d x\)
Solution:

Let \(\mathrm{I}=\int \sec ^2(7-4 \mathrm{x}) \mathrm{dx}\)

Put \(7-4 \mathrm{x}=\mathrm{t} \Rightarrow-4 \mathrm{dx}=\mathrm{dt}\)

∴ \(\mathrm{I}=-\frac{1}{4} \int \sec ^2 \mathrm{tdt}=\frac{-1}{4}(\tan \mathrm{t})+\mathrm{C}=\frac{-1}{4} \tan (7-4 \mathrm{x})+\mathrm{C}\)

Question 23. \(\int \frac{\sin ^{-1} x}{\sqrt{1-x^2}} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\sin ^{-1} \mathrm{x}}{\sqrt{1-\mathrm{x}^2}} \mathrm{dx}\)

Put \(\sin ^{-1} \mathrm{x}=\mathrm{t} \Rightarrow \frac{1}{\sqrt{1-\mathrm{x}^2}} \mathrm{dx}=\mathrm{dt}\)

∴ \(\mathrm{I}=\int \mathrm{tdt}=\frac{\mathrm{t}^2}{2}+\mathrm{C}=\frac{\left(\sin ^{-1} \mathrm{x}\right)^2}{2}+\mathrm{C}\)

Question 24. \(\int \frac{2 \cos x-3 \sin x}{6 \cos x+4 \sin x} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{2 \cos \mathrm{x}-3 \sin \mathrm{x}}{6 \cos \mathrm{x}+4 \sin \mathrm{x}} \mathrm{dx}=\frac{1}{2} \int \frac{2 \cos \mathrm{x}-3 \sin \mathrm{x}}{3 \cos \mathrm{x}+2 \sin \mathrm{x}} \mathrm{dx}\)

Put \(3 \cos x+2 \sin x=t \Rightarrow(-3 \sin x+2 \cos x) d x=d t\)

⇒ \((2 \cos x-3 \sin x) d x=d t\)

⇒ I = \(\frac{1}{2} \int_t^1 \frac{d t}{t}=\frac{1}{2} \log |t|+C=\frac{1}{2} \log |2 \sin x+3 \cos x|+C\)

Question 25. \(\int \frac{1}{\cos ^2 x(1-\tan x)^2} d x\)
Solution:

Let \(I=\int \frac{1}{\cos ^2 x(1-\tan x)^2} d x=\int \frac{\sec ^2 x}{(1-\tan x)^2} d x\)

Put \((1-\tan x)=t \Rightarrow \sec ^2 x d x=-d t\)

∴ I = \(\int \frac{-d t}{t^2}=-\int t^{-2} d t=\frac{1}{t}+C=\frac{1}{(1-\tan x)}+C\)

Question 26. \(\int \frac{\cos \sqrt{x}}{\sqrt{x}} d x\)
Solution:

Let \(I=\int \frac{\cos \sqrt{x}}{\sqrt{x}} d x\)

Put \(\sqrt{\mathrm{x}}=\mathrm{t} \Rightarrow \frac{1}{2 \sqrt{\mathrm{x}}} \mathrm{dx}=\mathrm{dt}\)

∴ I = \(2 \int \cos t d t=2 \sin \mathrm{t}+C=2 \sin \sqrt{x}+C\)

Question 27. \(\int \sqrt{\sin 2 x} \cos 2 x d x\)
Solution:

Let \(\mathrm{I}=\int \sqrt{\sin 2 \mathrm{x}} \cos 2 \mathrm{x} d \mathrm{x}\)

Put \(\sin 2 x=t^2 \Rightarrow 2 \cos 2 x d x=2 t d t \Rightarrow \cos 2 x d x=t d t\)

∴ \(\mathrm{I}=\int \mathrm{t} \cdot \mathrm{tdt}=\int \mathrm{t}^2 \mathrm{dt}=\frac{\mathrm{t}^3}{3}+\mathrm{C}=\frac{1}{3}(\sin 2 \mathrm{x})^{3 / 2}+\mathrm{C}\)

Question 28. \(\int \frac{\cos x}{\sqrt{1+\sin x}} d x\)
Solution:

Let \(I=\int \frac{\cos x}{\sqrt{1+\sin x}} d x\)

Put \(1+\sin x=t^2 \Rightarrow \cos x d x=2 t d t\)

∴ \(\mathrm{I}=\int \frac{1}{\mathrm{t}} \cdot 2 \mathrm{tdt}=2 \int 1 \cdot \mathrm{dt}=2 \mathrm{t}+\mathrm{C}=2 \sqrt{1+\sin \mathrm{x}}+\mathrm{C}\)

Question 29. \(\int \cot x \log \sin x d x\)
Solution:

Let \(\mathrm{I}=\int \cot \mathrm{x} \log \sin \mathrm{x} \mathrm{dx}\)

Put \(\log \sin x=t \Rightarrow \frac{1}{\sin x} \cdot \cos x d x=d t \Rightarrow \cot x d x=d t\)

∴ \(\mathrm{I}=\int \mathrm{tdt}=\frac{\mathrm{t}^2}{2}+\mathrm{C}=\frac{1}{2}(\log \sin \mathrm{x})^2+\mathrm{C}\)

Question 30. \(\int \frac{\sin x}{1+\cos x} d x\)
Solution:

Let \(I=\int \frac{\sin x}{1+\cos x} d x\)

Put \(1+\cos x=t \Rightarrow-\sin x d x=d t\)

∴ I =\(\int-\frac{d t}{t}=-\log |t|+C=-\log |1+\cos x|+C\)

Question 31. \(\int \frac{\sin x}{(1+\cos x)^2} d x\)
Solution:

Let \(I=\int \frac{\sin x}{(1+\cos x)^2} d x\)

Put \(1+\cos \mathrm{x}=\mathrm{t} \Rightarrow-\sin \mathrm{x} d \mathrm{x}=\mathrm{dt}\)

∴ I = \(\int-\frac{d t}{t^2}=-\int t^{-2} d t=\frac{1}{t}+C=\frac{1}{1+\cos x}+C\)

Question 32. \(\int \frac{1}{1+\cot x} d x\)
Solution:

Let \(I=\int \frac{1}{1+\cot x} d x=\int \frac{1}{1+\left(\frac{\cos x}{\sin x}\right)} d x=\int \frac{\sin x}{\sin x+\cos x} d x=\frac{1}{2} \int \frac{2 \sin x}{\sin x+\cos x} d x\)

= \(\frac{1}{2} \int \frac{(\sin x+\cos x)+(\sin x-\cos x)}{(\sin x+\cos x)} d x=\frac{1}{2} \int 1 d x-\frac{1}{2} \int \frac{\cos x-\sin x}{\sin x+\cos x} d x\)

= \(\frac{x}{2}-\frac{1}{2} \log |\sin x+\cos x|+C\) (because \(\int \frac{f^{\prime}(x)}{f(x)} d x=\log |f(x)|+C\))

Question 33. \(\int \frac{1}{1-\tan x} d x\)
Solution:

Let I = \(\int \frac{1}{1-\tan x} d x=\int \frac{1}{1-\left(\frac{\sin x}{\cos x}\right)} d x=\int \frac{\cos x}{\cos x-\sin x} d x=\frac{1}{2} \int \frac{2 \cos x}{\cos x-\sin x} d x\)

= \(\frac{1}{2} \int \frac{(\cos x-\sin x)+(\cos x+\sin x)}{(\cos x-\sin x)} d x=\frac{1}{2} \int 1 d x-\frac{1}{2} \int \frac{(-\sin x-\cos x)}{(\cos x-\sin x)} d x\)

= \(\frac{x}{2}-\frac{1}{2} \log |\cos x-\sin x|+C\) (because \(\int \frac{f^{\prime}(x)}{f(x)} d x=\log |f(x)|+C\))

Question 34. \(\int \frac{\sqrt{\tan x}}{\sin x \cos x} d x\)
Solution:

Let \(I=\int \frac{\sqrt{\tan x}}{\sin x \cos x} d x=\int \frac{\sqrt{\tan x}}{\frac{\sin x}{\cos x} \cdot \cos ^2 x} d x=\int \frac{\sqrt{\tan x}}{\tan x \cdot \cos ^2 x} d x\)

⇒\(\mathrm{I}=\int \frac{\sec ^2 x d x}{\sqrt{\tan x}}\)

Let \(\tan x=t^2 \Rightarrow \sec ^2 x d x=2 t d t\)

∴ I = \(\int \frac{1}{t} \cdot 2 t d t=2 \int 1 d t=2 t+C=2 \sqrt{\tan x}+C\)

Question 35. \(\int \frac{(1+\log x)^2}{x} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{(1+\log \mathrm{x})^2}{\mathrm{x}} \mathrm{dx}\)

Put \(1+\log x=t \Rightarrow \frac{1}{x} d x=d t\)

∴ I = \(\int t^2 d t=\frac{t^3}{3}+C=\frac{(1+\log x)^3}{3}+C\)

Question 36. \(\int \frac{(x+1)(x+\log x)^2}{x} d x\)
Solution:

Let \(I=\int \frac{(x+1)(x+\log x)^2}{x} d x\)

Put \((x+\log x)=t \Rightarrow\left(1+\frac{1}{x}\right) d x=d t \Rightarrow\left(\frac{x+1}{x}\right) d x=d t\)

∴ \(\mathrm{I}=\int \mathrm{t}^2 \mathrm{dt}=\frac{\mathrm{t}^3}{3}+\mathrm{C}=\frac{1}{3}(\mathrm{x}+\log \mathrm{x})^3+\mathrm{C}\)

Question 37. \(\int \frac{x^3 \sin \left(\tan ^{-1} x^4\right)}{1+x^8} d x\)
Solution:

Let \(I=\int \frac{x^3 \sin \left(\tan ^{-1} x^4\right)}{1+x^8} d x\)

Put \(\tan ^{-1} x^4=t \Rightarrow \frac{4 x^3}{\left(1+x^8\right)} d x=d t \Rightarrow \frac{x^3}{\left(1+x^8\right)} d x=\frac{d t}{4}\)

∴ \(I=\frac{1}{4} \int \sin t \cdot d t=\frac{1}{4}(-\cos t)+C=-\frac{1}{4} \cos \left(\tan ^{-1} x^4\right)+C\)

Choose The Correct Answer

Question 38. \(\int \frac{10 x^9+10^x \log _e 10}{x^{10}+10^x} d x\) equals:

1. \(10^x-\mathrm{x}^{10}+\mathrm{C}\)
2. \(10^5+\mathrm{x}^{10}+\mathrm{C}\)
3. \(\left(10^5-x^{10}\right)^{-1}+C\)
4. \(\log \left(10^x+x^{10}\right)+C\)

Solution: 4. \(\log \left(10^x+x^{10}\right)+C\)

Let \(\mathrm{I}=\int \frac{10 \mathrm{x}^9+10^{\mathrm{x}} \log _{\mathrm{c}} 10}{\mathrm{x}^{10}+10^{\mathrm{x}}} \mathrm{dx}\)

Put \(x^{10}+10^x=t \Rightarrow\left(10 x^9+10^x \log , 10\right) d x=d t\)

∴ \(I=\int \frac{d t}{t}=\log |t|+C=\log \left|10^x+x^{10}\right|+C\)

Hence, the correct answer is (4)

Question 39. \(\int \frac{d x}{\sin ^2 x \cos ^2 x}\) equals?

1. \(\tan x+\cot x+C\)
2. \(\tan x-\cot x+C\)
3. \(\tan \mathrm{x} \cdot \cot \mathrm{x}+\mathrm{C}\)
4. \(\tan x-\cot 2 x+C\)

Solution: 2. \(\tan x-\cot x+C\)

Let I = \(\int \frac{d x}{\sin ^2 x \cos ^2 x}=\int \frac{1}{\sin ^2 x \cos ^2 x} d x\)

= \(\int \frac{\sin ^2 x+\cos ^2 x}{\sin ^2 x \cos ^2 x} d x\)

= \(\int \frac{\sin ^2 x}{\sin ^2 x \cos ^2 x} d x+\int \frac{\cos ^2 x}{\sin ^2 x \cos ^2 x} d x\)

= \(\int \sec ^2 x d x+\int \mathrm{cosec}^2 x d x\)

= \(\tan x-\cot x+C\) (because \(\sin ^2 x+\cos ^2=1\))

Hence, the correct answer is (2).

Integrals Exercise 7.3

Find The Integrals Of The Function

Question 1. \(\int \sin ^2(2 x+5) d x\)
Solution:

Let I = \(\int \sin ^2(2 x+5) d x=\frac{1}{2} \int 2 \sin ^2(2 x+5) d x\)

I = \(\frac{1}{2} \int\{1-\cos (4 x+10)\} d x=\frac{1}{2} \int 1 d x-\frac{1}{2} \int \cos (4 x+10) d x\)

= \(\frac{1}{2} x-\frac{1}{2}\left(\frac{\sin (4 x+10)}{4}\right)+C=\frac{1}{2} x-\frac{1}{8} \sin (4 x+10)+C\)

Question 2. \(\int \sin 3 x \cos 4 x d x\)
Solution:

Let \(\mathrm{I}=\int \sin 3 \mathrm{x} \cos 4 \mathrm{xdx}=\frac{1}{2} \int 2 \sin 3 \mathrm{x} \cos 4 \mathrm{xdx}=\frac{1}{2} \int\{\sin 7 \mathrm{x}+\sin (-\mathrm{x})\} \mathrm{dx}\)

= \(\frac{1}{2} \int\{\sin 7 x-\sin x\} d x=\frac{1}{2} \int \sin 7 x d x-\frac{1}{2} \int \sin x d x=\frac{1}{2}\left(\frac{-\cos 7 x}{7}\right)-\frac{1}{2}(-\cos x)+C\)

= \(\frac{-\cos 7 x}{14}+\frac{\cos x}{2}+C\)

Question 3. \(\int \cos 2 x \cos 4 x \cos 6 x d x\)
Solution:

Let \(I=\int \cos 2 x \cos 4 x \cos 6 x d x=\frac{1}{2} \int \cos 2 x(2 \cos 4 x \cos 6 x) d x\)

= \(\frac{1}{2} \int \cos 2 x[\cos (4 x+6 x)+\cos (4 x-6 x)] d x\)

= \(\frac{1}{2} \int\{\cos 2 x \cos 10 x+\cos 2 x \cos (-2 x)\} d x\) (because \(\cos (-\theta)=\cos \theta\))

= \(\frac{1}{2} \int\left\{\cos 2 x \cos 10 x+\cos ^2 2 x\right\} d x=\frac{1}{4} \int\left\{2 \cos 2 x \cos 10 x+2 \cos ^2 2 x\right\} d x\)

= \(\frac{1}{4} \int(\cos 12 x+\cos 8 x+1+\cos 4 x) d x=\frac{1}{4}\left[\frac{\sin 12 x}{12}+\frac{\sin 8 x}{8}+x+\frac{\sin 4 x}{4}\right]+C\)

Question 4. \(\int \sin ^3(2 x+1) \mathrm{d} x\)
Solution:

Let \(\mathrm{I}=\int \sin ^3(2 \mathrm{x}+1) \mathrm{dx}=\int\left(1-\cos ^2(2 \mathrm{x}+1)\right\} \sin (2 \mathrm{x}+1) \mathrm{dx}\)

Put \(\cos (2 \mathrm{x}+1)=\mathrm{t} \Rightarrow-2 \sin (2 \mathrm{x}+1) \mathrm{dx}=\mathrm{dt} \Rightarrow \sin (2 \mathrm{x}+1) \mathrm{dx}=-\frac{\mathrm{dt}}{2}\)

∴ I = \(\frac{-1}{2} \int\left(1-t^2\right) d t=\frac{-1}{2}\left\{t-\frac{t^3}{3}\right\}+C=\frac{-1}{2}\left\{\cos (2 x+1)-\frac{\cos ^3(2 x+1)}{3}\right\}+C\)

= \(\frac{-\cos (2 x+1)}{2}+\frac{\cos ^3(2 x+1)}{6}+C\)

Question 5. \(\int \sin ^3 x \cos ^3 x d x\)
Solution:

Let \(I=\int \sin ^3 x \cos ^3 x \cdot d x=\int \cos ^3 x \cdot \sin ^2 x \cdot \sin x \cdot d x=\int \cos ^3 x\left(1-\cos ^2 x\right) \sin x \cdot d x\)

Put \(\cos \mathrm{x}=\mathrm{t} \Rightarrow-\sin \mathrm{x} \cdot \mathrm{dx}=\mathrm{dt}\)

∴ I = \(-\int t^3\left(1-t^2\right) d t=-\int\left(t^3-t^5\right) d t=-\left\{\frac{t^4}{4}-\frac{t^6}{6}\right\}+C\)

= \(-\left\{\frac{\cos ^4 x}{4}-\frac{\cos ^6 x}{6}\right\}+C=\frac{\cos ^6 x}{6}-\frac{\cos ^4 x}{4}+C\)

Question 6. \(\int \sin x \sin 2 x \sin 3 x d x\)
Solution:

Let I = \(\int \sin x \sin 2 x \sin 3 x d x=\frac{1}{2} \int \sin x\{2 \sin 2 x \sin 3 x\} d x\)

∴ I = \(\frac{1}{2} \int[\sin x \cdot\{\cos (2 x-3 x)-\cos (2 x+3 x)\}] d x\)

= \(\frac{1}{2} \int(\sin x \cos (-x)-\sin x \cos 5 x) d x=\frac{1}{2} \int(\sin x \cos x-\sin x \cos 5 x) d x\)

= \(\frac{1}{4} \int(2 \sin x \cos x-2 \sin x \cos 5 x) d x=\frac{1}{4} \int \sin 2 x d x-\frac{1}{4} \int\{\sin 6 x+\sin (-4 x)\} d x\)

= \(\frac{1}{4} \int \sin 2 x d x-\frac{1}{4} \int\{\sin 6 x-\sin 4 x\} d x\)

= \(\frac{-\cos 2 x}{8}-\frac{1}{4}\left[\frac{-\cos 6 x}{6}+\frac{\cos 4 x}{4}\right]+C=-\frac{\cos 2 x}{8}-\frac{1}{8}\left[-\frac{\cos 6 x}{3}+\frac{\cos 4 x}{2}\right]+C\)

= \(\frac{1}{8}\left[\frac{\cos 6 x}{3}-\frac{\cos 4 x}{2}-\cos 2 x\right]+C\)

Question 7. \(\int \sin 4 x \sin 8 x d x\)
Solution:

Let \(I=\int \sin 4 x \sin 8 x d x=\frac{1}{2} \int 2 \sin 4 x \sin 8 x d x\)

∴ I = \(\frac{1}{2} \int\{\cos (4 x-8 x)-\cos (4 x+8 x)\} d x=\frac{1}{2} \int(\cos (-4 x)-\cos 12 x) d x\)

= \(\frac{1}{2} \int(\cos 4 x-\cos 12 x) d x=\frac{1}{2}\left[\frac{\sin 4 x}{4}-\frac{\sin 12 x}{12}\right]+C\)

Question 8. \(\int \frac{1-\cos x}{1+\cos x} d x\)
Solution:

Let \(I=\int \frac{1-\cos x}{1+\cos x} d x=\int \frac{2 \sin ^2 x / 2}{2 \cos ^2 x / 2} d x=\int \tan ^2 \frac{x}{2} d x=\int\left(\sec ^2 \frac{x}{2}-1\right) d x\)

= \(\left[\frac{\tan \frac{x}{2}}{\frac{1}{2}}-x\right]+C=2 \tan \frac{x}{2}-x+C\)

Question 9. \(\int \frac{\cos x}{1+\cos x} d x\)
Solution:

Let \(I=\int \frac{\cos x}{1+\cos x} d x=\int \frac{\cos ^2 \frac{x}{2}-\sin ^2 \frac{x}{2}}{2 \cos ^2 \frac{x}{2}} d x\)

∴ I = \(\frac{1}{2} \int\left(1-\tan ^2 \frac{x}{2}\right) d x=\frac{1}{2} \int\left(1-\sec ^2 \frac{x}{2}+1\right) d x=\frac{1}{2} \int\left(2-\sec ^2 \frac{x}{2}\right) d x\)

= \(\frac{1}{2}\left[2 x-\frac{\tan \frac{x}{2}}{1 / 2}\right]+C=x-\tan \frac{x}{2}+C\)

Question 10. \(\int \sin ^4 x d x\)
Solution:

Let \(I=\int \sin ^4 x d x=\int \sin ^2 x \sin ^2 x d x=\int\left(\frac{1-\cos 2 x}{2}\right)\left(\frac{1-\cos 2 x}{2}\right) d x\)

= \(\int \frac{1}{4}(1-\cos 2 x)^2 d x=\frac{1}{4} \int\left[1+\cos ^2 2 x-2 \cos 2 x\right] d x\)

= \(\frac{1}{4} \int\left[1+\left(\frac{1+\cos 4 x}{2}\right)-2 \cos 2 x\right] d x\)

= \(\frac{1}{4} \int\left[1+\frac{1}{2}+\frac{1}{2} \cos 4 x-2 \cos 2 x\right] d x=\frac{1}{4} \int\left[\frac{3}{2}+\frac{1}{2} \cos 4 x-2 \cos 2 x\right] d x\)

= \(\frac{3 x}{8}+\frac{1}{32} \sin 4 x-\frac{1}{4} \sin 2 x+C\)

Question 11. \(\int \cos ^4 2 x d x\)
Solution:

Let \(I=\int \cos ^4 2 x d x=\int\left(\cos ^2 2 x\right)^2 d x=\int\left(\frac{1+\cos 4 x}{2}\right)^2 d x\)

= \(\frac{1}{4} \int\left[1+\cos ^2 4 x+2 \cos 4 x\right] d x\)

= \(\frac{1}{4} \int\left[1+\left(\frac{1+\cos 8 x}{2}\right)+2 \cos 4 x\right] d x=\frac{1}{4} \int\left[1+\frac{1}{2}+\frac{\cos 8 x}{2}+2 \cos 4 x\right] d x\)

= \(\frac{1}{4} \int\left[\frac{3}{2}+\frac{\cos 8 x}{2}+2 \cos 4 x\right] d x=\int\left(\frac{3}{8}+\frac{\cos 8 x}{8}+\frac{\cos 4 x}{2}\right) d x\)

= \(\frac{3}{8} x+\frac{\sin 8 x}{64}+\frac{\sin 4 x}{8}+C\)

Question 12. \(\int \frac{\sin ^2 x}{1+\cos x} d x\)
Solution:

Let \(I=\int \frac{\sin ^2 x}{1+\cos x} d x=\int \frac{\left(1-\cos ^2 x\right)}{(1+\cos x)} d x=\int(1-\cos x) d x=x-\sin x+C\)

Question 13. \(\int \frac{\cos 2 x-\cos 2 \alpha}{\cos x-\cos \alpha} d x\)
Solution:

Let, I=\(\int \frac{\cos 2 x-\cos 2 \alpha}{\cos x-\cos \alpha} d x=\int \frac{\left(2 \cos ^2 x-1\right)-\left(2 \cos ^2 \alpha-1\right)}{(\cos x-\cos \alpha)} d x=\int \frac{2\left(\cos ^2 x-\cos ^2 \alpha\right)}{(\cos x-\cos \alpha)} d x\)

= \(2 \int(\cos x+\cos \alpha) d x=2 \sin x+2 x \cos \alpha+C=2[\sin x+x \cos \alpha]+C\)

Question 14. \(\int \frac{\cos x-\sin x}{1+\sin 2 x} d x\)
Solution:

Let \(I=\int \frac{\cos x-\sin x}{1+\sin 2 x} d x=\int \frac{\cos x-\sin x}{(\sin x+\cos x)^2} d x\) (because \(1+\sin 2 x=(\sin x+\cos x)^2\))

Put \(\sin \mathrm{x}+\cos \mathrm{x}=\mathrm{t} \Rightarrow(\cos \mathrm{x}-\sin \mathrm{x}) \mathrm{dx}=\mathrm{dt}\)

∴ I = \(\int \frac{1}{t^2} d t=-\frac{1}{t}+C=-\frac{1}{(\sin x+\cos x)}+C\)

Question 15. \(\int \tan ^3 2 x \sec 2 x d x\)
Solution:

Let \(I=\int \tan ^3 2 x \sec 2 x d x=\int \tan ^2 2 x \cdot \sec 2 x \tan 2 x d x\)

= \(\int\left(\sec ^2 2 x-1\right) \sec 2 x \tan 2 x d x\)

Put sec 2x=t

⇒ sec 2x tan 2x dx = \(\frac{d t}{2}\)

∴ I = \(\int\left(t^2-1\right) \cdot \frac{d t}{2}=\frac{1}{2} \int\left(t^2-1\right) d t=\frac{1}{2}\left[\frac{t^3}{3}-t\right]+C\)

= \(\frac{1}{6}(\sec 2 x)^3-\frac{1}{2}(\sec 2 x)+C=\frac{1}{6} \sec ^3 2 x-\frac{1}{2} \sec 2 x+C\)

Question 16. \(\int \tan ^4 x d x\)
Solution:

Let \(I=\int \tan ^4 x d x=\int \tan ^2 x \cdot \tan ^2 x d x=\int\left(\sec ^2 x-1\right) \tan ^2 x d x\)

= \(\int\left(\tan ^2 x \sec ^2 x-\tan ^2 x\right) d x=\int \tan ^2 x \sec ^2 x d x-\int \sec ^2 x d x+\int 1 d x\)

= \(\frac{\tan ^3 x}{3}-\tan x+x+C\) (because \(\int\{f(x)\}^{\prime \prime} \cdot f^{\prime}(x) d x=\frac{\{f(x)\}^{n+1}}{n+1}+C\))

Question 17. \(\int \frac{\sin ^3 x+\cos ^3 x}{\sin ^2 x \cos ^2 x} d x\)
Solution:

Let \(I=\int \frac{\sin ^3 x+\cos ^3 x}{\sin ^2 x \cos ^2 x} d x=\int\left(\frac{\sin ^3 x}{\sin ^2 x \cos ^2 x}+\frac{\cos ^3 x}{\sin ^2 x \cos ^2 x}\right) d x\)

= \(\int\left(\frac{\sin x}{\cos ^2 x}+\frac{\cos x}{\sin ^2 x}\right) d x=\int(\tan x \sec x+\cot x \mathrm{cosec} x) d x=\sec x-\mathrm{cosec} x+C\)

Question 18. \(\int \frac{\cos 2 x+2 \sin ^2 x}{\cos ^2 x} d x\)
Solution:

Let \(I=\int \frac{\cos 2 x+2 \sin ^2 x}{\cos ^2 x} d x=\int \frac{\left(1-2 \sin ^2 x\right)+2 \sin ^2 x}{\cos ^2 x} d x\)

= \(\int \frac{1}{\cos ^2 x} d x=\int \sec ^2 x d x=\tan x+C\)

Question 19. \(\int \frac{1}{\sin x \cos ^3 x} d x\)
Solution:

Let \(I=\int \frac{1}{\sin x \cos ^3 x} d x=\int \frac{\sin ^2 x+\cos ^2 x}{\sin x \cos ^3 x} d x=\int\left(\frac{\sin x}{\cos ^3 x}+\frac{1}{\sin x \cos x}\right) d x\)

= \(\int\left(\tan x \sec ^2 x+\frac{\sec ^2 x}{\tan x}\right) d x\)

∴ I = \(\int \tan x \sec ^2 x d x+\int \frac{\sec ^2 x}{\tan x} d x\)

Put tan x = \(t \Rightarrow \sec ^2 \mathrm{x} d \mathrm{x}=\mathrm{dt}\)

⇒ \(\mathrm{I}=\int \mathrm{tdt}+\int \frac{1}{\mathrm{t}} \mathrm{dt}=\frac{\mathrm{t}^2}{2}+\log |\mathrm{t}|+\mathrm{C}=\frac{1}{2} \tan ^2 \mathrm{x}+\log |\tan \mathrm{x}|+\mathrm{C}\)

Question 20. \(\int \frac{\cos 2 x}{(\cos x+\sin x)^2} d x\)
Solution:

Let \(I=\int \frac{\cos 2 x}{(\cos x+\sin x)^2} d x=\int \frac{\left(\cos ^2 x-\sin ^2 x\right)}{(\cos x+\sin x)^2} d x=\int \frac{(\cos x+\sin x)(\cos x-\sin x)}{(\cos x+\sin x)^2} d x\)

= \(\int \frac{(\cos x-\sin x)}{(\cos x+\sin x)} d x=\log |\sin x+\cos x|+C\) (because \(\int \frac{f^{\prime}(x)}{f(x)} d x=\log |f(x)|+C\))

Question 21. \(\int \sin ^{-1}(\cos x) d x\)
Solution:

Let \(I=\int \sin ^{-1}(\cos x) d x=\int \sin ^{-1}\left[\sin \left(\frac{\pi}{2}-x\right)\right] d x=\int\left(\frac{\pi}{2}-x\right) d x=\frac{\pi}{2} x-\frac{x^2}{2}+C\)

Question 22. \(\int \frac{1}{\cos (x-a) \cos (x-b)} d x\)
Solution:

Let \(I=\int \frac{1}{\cos (x-a) \cos (x-b)} d x=\frac{1}{\sin (a-b)} \int\left[\frac{\sin (a-b)}{\cos (x-a) \cos (x-b)}\right] d x\)

= \(\frac{1}{\sin (a-b)} \int\left[\frac{\sin [(x-b)-(x-a)]}{\cos (x-a) \cos (x-b)}\right] d x\)

= \(\frac{1}{\sin (a-b)} \int\left[\frac{[\sin (x-b) \cos (x-a)-\cos (x-b) \sin (x-a)]}{\cos (x-a) \cos (x-b)}\right] d x\)

= \(\frac{1}{\sin (a-b)} \int[\tan (x-b)-\tan (x-a)] d x\)

= \(\frac{1}{\sin (a-b)}[-\log |\cos (x-b)|+\log |\cos (x-a)|]\)

= \(\frac{1}{\sin (a-b)}\left[\log \left|\frac{\cos (x-a)}{\cos (x-b)}\right|\right]+C\)

Choose The Correct Answer

Question 23. \(\int \frac{\sin ^2 x-\cos ^2 x}{\sin ^2 x \cos ^2 x} d x\) is equal to ?

1. \(\tan x+\cot x\)+C
2. \(\tan x+\mathrm{cosec}x\)+C
3. \(-\tan x+\cot x\)+C
4. \(\tan x+\sec x+\)C

Solution: 1. \(\tan x+\cot x\)+C

Let \(I=\int \frac{\sin ^2 x-\cos ^2 x}{\sin ^2 x \cos ^2 x} d x=\int\left(\frac{\sin ^2 x}{\sin ^2 x \cos ^2 x}-\frac{\cos ^2 x}{\sin ^2 x \cos ^2 x}\right) d x\)

= \(\int\left(\sec ^2 \mathrm{x}-\mathrm{cosec}^2 \mathrm{x}\right) \mathrm{dx}=\tan \mathrm{x}+\cot \mathrm{x}+\mathrm{C}\)

Hence, the correct answer is (1).

Question 24. \(\int \frac{e^x(1+x)}{\cos ^2\left(e^x x\right)} d x\) equals ?

1. \(-\cot \left(e x^x\right)+C\)
2. \(\tan \left(\mathrm{xe}^x\right)+C\)
3. \(\tan \left(e^x\right)+C\)
4. \(\cot \left(e^x\right)+C\)

Solution: 2. \(\tan \left(\mathrm{xe}^x\right)+C\)

Let I = \(\int \frac{e^x(1+x)}{\cos ^2\left(e^x x\right)} d x\)

Put \(e^x \cdot x=t \Rightarrow\left(e^x \cdot x+e^x \cdot 1\right) d x=d t\)

⇒ \(e^x(x+1) d x=d t\)

∴ \(I=\int \frac{d t}{\cos ^2 t}=\int \sec ^2 t d t\)

= \(\tan t+C=\tan \left(e^x \cdot x\right)+C=\tan \left(x \cdot e^x\right)+C\)

Hence, the correct answer is (2).

Integrals Exercise 7.4

Integrate The Function

Question 1. \(\int \frac{3 x^2}{x^6+1} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{3 \mathrm{x}^2}{\mathrm{x}^6+1} \mathrm{dx}\)

Put \(\mathrm{x}^3=\mathrm{t} \Rightarrow 3 \mathrm{x}^2 \mathrm{dx}=\mathrm{dt}\)

∴ I = \(\int \frac{3 x^2}{\left(x^3\right)^2+1} d x=\int \frac{d t}{t^2+1}=\tan ^{-1} t+C=\tan ^{-1}\left(x^3\right)+C\)

Question 2. \(\int \frac{1}{\sqrt{1+4 \mathrm{x}^2}} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \frac{1}{\sqrt{1+4 \mathrm{x}^2}} \mathrm{dx}\)

Put \(2 \mathrm{x}=\mathrm{t} \Rightarrow 2 \mathrm{dx}=\mathrm{dt}\)

∴ \(\mathrm{I}=\int \frac{1}{\sqrt{1+(2 \mathrm{x})^2}} \mathrm{dx}=\frac{1}{2} \int \frac{\mathrm{dt}}{\sqrt{1+\mathrm{t}^2}}=\frac{1}{2}\left[\log \left|\mathrm{t}+\sqrt{\mathrm{t}^2+1}\right|\right]+\mathrm{C}=\frac{1}{2} \log \left|2 \mathrm{x}+\sqrt{4 \mathrm{x}^2+1}\right|+\mathrm{C}\)

Question 3. \(\int \frac{1}{\sqrt{(2-x)^2+1}} d x\)
Solution:

Let \(I=\int \frac{1}{\sqrt{(2-x)^2+1}} d x\)

Put \(2-\mathrm{x}=\mathrm{t} \Rightarrow-\mathrm{dx}=\mathrm{dt}\)

∴ I = \(-\int \frac{1}{\sqrt{t^2+1}} d t=-\log \left|t+\sqrt{t^2+1}\right|+C=-\log \left|(2-x)+\sqrt{(2-x)^2+1}\right|+C\)

= \(\log \left|\frac{1}{(2-x)+\sqrt{x^2-4 x+5}}\right|+C\)

Question 4. \(\int \frac{1}{\sqrt{9-25 x^2}} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{1}{\sqrt{9-25 \mathrm{x}^2}} \mathrm{dx}\)

Put \(5 \mathrm{x}=\mathrm{t} \Rightarrow 5 \mathrm{dx}=\mathrm{dt}\)

∴ \(I=\frac{1}{5} \int \frac{1}{\sqrt{9-t^2}} d t=\frac{1}{5} \int \frac{1}{\sqrt{3^2-t^2}} d t=\frac{1}{5} \sin ^{-1}\left(\frac{t}{3}\right)+C=\frac{1}{5} \sin ^{-1}\left(\frac{5 x}{3}\right)+C\)

Question 5. \(\int \frac{3 x}{1+2 x^4} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{3 \mathrm{x}}{1+2 \mathrm{x}^4} \mathrm{dx}\)

Put \(\sqrt{2} \mathrm{x}^2=\mathrm{t} \Rightarrow 2 \sqrt{2} \mathrm{xdx}=\mathrm{dt}\)

∴ \(\mathrm{I}=\frac{3}{2 \sqrt{2}} \int \frac{\mathrm{dt}}{1+\mathrm{t}^2}=\frac{3}{2 \sqrt{2}}\left(\tan ^{-1} \mathrm{t}\right)+\mathrm{C}=\frac{3}{2 \sqrt{2}} \tan ^{-1}\left(\sqrt{2} \mathrm{x}^2\right)+\mathrm{C}\)

Question 6. \(\int \frac{x^2}{1-x^6} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{x}^2}{1-\mathrm{x}^6} \mathrm{dx} \Rightarrow \mathrm{I}=\int \frac{\mathrm{x}^2}{1-\left(\mathrm{x}^3\right)^2} \mathrm{dx}\)

Put \(\mathrm{x}^3=\mathrm{t} \Rightarrow 3 \mathrm{x}^2 \mathrm{dx}=\mathrm{dt}\)

= \(\frac{1}{3} \int \frac{\mathrm{dt}}{1-\mathrm{t}^2}=\frac{1}{3}\left[\frac{1}{2} \log \left|\frac{1+\mathrm{t}}{1-\mathrm{t}}\right|\right]+\mathrm{C}=\frac{1}{6} \log \left|\frac{1+\mathrm{x}^3}{1-\mathrm{x}^3}\right|+\mathrm{C}\)

Question 7. \(\int \frac{\mathrm{x}-1}{\sqrt{\mathrm{x}^2-1}} \mathrm{dx}\)
Solution:

Let \(I=\int \frac{x-1}{\sqrt{x^2-1}} d x \Rightarrow \int \frac{x}{\sqrt{x^2-1}} d x-\int \frac{1}{\sqrt{x^2-1}} d x\)

= \(\int \frac{x}{\sqrt{x^2-1}} d x-\log \left|x+\sqrt{x^2-1}\right|\)

Put \(x^2-1=t^2 \Rightarrow 2 x d x=2 t d t\)

∴ I = \(\int \frac{t d t}{t}-\log \left|x+\sqrt{x^2-1}\right|=\int 1 d t-\log \left|x+\sqrt{x^2-1}\right|\)

= \(t-\log \left|x+\sqrt{x^2-1}\right|=\sqrt{x^2-1}-\log \left|x+\sqrt{x^2-1}\right|+C\)

Question 8. \(\int \frac{x^2}{\sqrt{x^6+a^6}} d x\)
Solution:

Let \(I=\int \frac{x^2}{\sqrt{x^6+a^6}} d x \Rightarrow \int \frac{x^2}{\sqrt{\left(x^3\right)^2+\left(a^3\right)^2}} d x\)

Put \(\mathrm{x}^3=\mathrm{t} \Rightarrow 3 \mathrm{x}^2 \mathrm{dx}=\mathrm{dt}\)

= \(\frac{1}{3} \int \frac{\mathrm{dt}}{\sqrt{\mathrm{t}^2+\left(\mathrm{a}^3\right)^2}}=\frac{1}{3} \log \left|\mathrm{t}+\sqrt{\mathrm{t}^2+\mathrm{a}^6}\right|+\mathrm{C}=\frac{1}{3} \log \left|\mathrm{x}^3+\sqrt{\mathrm{x}^6+\mathrm{a}^6}\right|+\mathrm{C}\)

Question 9. \(\int \frac{\sec ^2 x}{\sqrt{\tan ^2 x+4}} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\sec ^2 \mathrm{x}}{\sqrt{\tan ^2 \mathrm{x}+4}} \mathrm{dx}\)

Put \(\tan \mathrm{x}=\mathrm{t} \Rightarrow \sec ^2 \mathrm{x} d \mathrm{x}=\mathrm{dt}\)

∴ \(I=\int \frac{d t}{\sqrt{t^2+2^2}}=\log \left|t+\sqrt{t^2+4}\right|+C=\log \left|\tan x+\sqrt{\tan ^2 x+4}\right|+C\)

Question 10. \(\int \frac{1}{\sqrt{\mathrm{x}^2+2 \mathrm{x}+2}} \mathrm{dx}\)
Solution:

Let \(I=\int \frac{1}{\sqrt{x^2+2 x+2}} d x=\int \frac{1}{\sqrt{(x+1)^2+1}} d x\)

Put \(\mathrm{x}+1=\mathrm{t} \Rightarrow \mathrm{dx}=\mathrm{dt}\)

∴ I = \(\int \frac{1}{\sqrt{t^2+1}} d t=\log \left|t+\sqrt{t^2+1}\right|+C\)

= \(\log \left|(x+1)+\sqrt{(x+1)^2+1}\right|+C\)

= \(\log \left|(x+1)+\sqrt{x^2+2 x+2}\right|+C\)

Question 11. \(\int \frac{1}{\left(9 x^2+6 x+5\right)} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{1}{\left(9 \mathrm{x}^2+6 \mathrm{x}+5\right)} \mathrm{dx}=\int \frac{1}{(3 \mathrm{x}+1)^2+4} \mathrm{dx}\)

Put \((3 \mathrm{x}+1)=\mathrm{t} \Rightarrow 3 \mathrm{dx}=\mathrm{dt}\)

∴ \(\mathrm{I}=\frac{1}{3} \int \frac{1}{\mathrm{t}^2+2^2} \mathrm{dt}=\frac{1}{3}\left[\frac{1}{2} \tan ^{-1}\left(\frac{\mathrm{t}}{2}\right)\right]+\mathrm{C}=\frac{1}{6} \tan ^{-1}\left(\frac{3 \mathrm{x}+1}{2}\right)+\mathrm{C}\)

Question 12. \(\int \frac{1}{\sqrt{7-6 x-x^2}} d x\)
Solution:

Let \(I=\int \frac{1}{\sqrt{7-6 x-x^2}} d x=\int \frac{1}{\sqrt{16-(x+3)^2}} d x\)

Put \(\mathrm{x}+3=\mathrm{t} \Rightarrow \mathrm{dx}=\mathrm{dt}\)

∴ I = \(\int \frac{1}{\sqrt{(4)^2-(t)^2}} d t=\sin ^{-1}\left(\frac{t}{4}\right)+C=\sin ^{-1}\left(\frac{x+3}{4}\right)+C\)

Question 13. \(\int \frac{1}{\sqrt{(x-1)(x-2)}} d x\)
Solution:

Let \(I=\int \frac{1}{\sqrt{(x-1)(x-2)}} d x=\int \frac{1}{\sqrt{x^2-3 x+2}} d x=\int \frac{1}{\sqrt{\left(x-\frac{3}{2}\right)^2-\frac{1}{4}}} d x\)

Put \(x-\frac{3}{2}=t \Rightarrow d x=d t\)

∴ I = \(\int \frac{1}{\sqrt{t^2-\left(\frac{1}{2}\right)^2}} d t=\log \left|t+\sqrt{t^2-\left(\frac{1}{2}\right)^2}\right|+C=\log \left|\left(x-\frac{3}{2}\right)+\sqrt{x^2-3 x+2}\right|+C\)

Question 14. \(\int \frac{1}{\sqrt{8+3 \mathrm{x}-\mathrm{x}^2}} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \frac{1}{\sqrt{8+3 \mathrm{x}-\mathrm{x}^2}} \mathrm{dx} \Rightarrow \mathrm{I}=\int \frac{1}{\sqrt{\frac{41}{4}-\left(\mathrm{x}-\frac{3}{2}\right)^2}} \mathrm{dx}\)

Put \(\mathrm{x}-\frac{3}{2}=\mathrm{t} \Rightarrow \mathrm{dx}=\mathrm{dt}\)

∴ I = \(\int \frac{1}{\left(\frac{\sqrt{41}}{2}\right)^2-t^2} d t=\sin ^{-1}\left(\frac{t}{\frac{\sqrt{41}}{2}}\right)+C=\sin ^{-1}\left(\frac{x-3 / 2}{\frac{\sqrt{41}}{2}}\right)+C=\sin ^{-1}\left(\frac{2 x-3}{\sqrt{41}}\right)+C\)

Question 15. \(\int \frac{1}{\sqrt{(x-a)(x-b)}} d x\)
Solution:

Let \(I=\int \frac{1}{\sqrt{(x-a)(x-b)}} d x=\int \frac{1}{\sqrt{x^2-(a+b) x+a b}} d x=\int \frac{1}{\sqrt{\left\{x-\left(\frac{a+b}{2}\right)\right\}^2-\left(\frac{a-b}{2}\right)^2}} d x\)

Put \(\mathrm{x}-\left(\frac{\mathrm{a}+\mathrm{b}}{2}\right)=\mathrm{t} \Rightarrow \mathrm{dx}=\mathrm{dt}\)

⇒ \(\int \frac{1}{\sqrt{\left\{x-\left(\frac{a+b}{2}\right)\right\}^2-\left(\frac{a-b}{2}\right)^2}} d x=\int \frac{1}{\sqrt{t^2-\left(\frac{a-b}{2}\right)^2}} d t\)

= \(\log \left|t+\sqrt{t^2-\left(\frac{a-b}{2}\right)^2}\right|+C=\log \left|\left(x-\frac{a+b}{2}\right)+\sqrt{\left\{x-\left(\frac{a+b}{2}\right)\right\}^2-\left(\frac{a-b}{2}\right)^2}\right|+C\)

= \(\log \left|\left\{x-\left(\frac{a+b}{2}\right)\right\}+\sqrt{(x-a)(x-b)}\right|+C\)

Question 16. \(\int \frac{4 \mathrm{x}+1}{\sqrt{2 \mathrm{x}^2+\mathrm{x}-3}} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \frac{4 \mathrm{x}+1}{\sqrt{2 \mathrm{x}^2+\mathrm{x}-3}} \mathrm{dx}\)

Put \(2 \mathrm{x}^2+\mathrm{x}-3=\mathrm{t}^2 \Rightarrow(4 \mathrm{x}+1) \mathrm{dx}=2 \mathrm{t} d \mathrm{t}\)

∴ \(\mathrm{I}=\int \frac{2 \mathrm{t}}{\mathrm{t}} \mathrm{dt}=2 \int 1 \mathrm{dt}=2 \mathrm{t}+\mathrm{C}=2 \sqrt{2 \mathrm{x}^2+\mathrm{x}-3}+\mathrm{C}\)

Question 17. \(\int \frac{x+2}{\sqrt{x^2-1}} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{x}+2}{\sqrt{\mathrm{x}^2-1}} \mathrm{dx}\)

Let x+2=A \(\frac{d}{d x}\left(x^2-1\right)+B\)

⇒ \(\mathrm{x}+2=\mathrm{A}(2 \mathrm{x})+\mathrm{B}\)

Equating the coefficients of x and constant term on both sides, we get 2A=1 \(A=\frac{1}{2}, B=2\)

From (1), we obtain, \((x+2)=\frac{1}{2}(2 x)+2\)

∴ I = \(\int \frac{\frac{1}{2}(2 x)+2}{\sqrt{x^2-1}} d x=\frac{1}{2} \int \frac{2 x}{\sqrt{x^2-1}} d x+\int \frac{2}{\sqrt{x^2-1}} d x\)

= \(\frac{1}{2} \int \frac{2 x}{\sqrt{x^2-1}} d x+2 \int \frac{1}{\sqrt{x^2-1}} d x=\frac{1}{2} \int \frac{2 x}{\sqrt{x^2-1}} d x+2 \log \left|x+\sqrt{x^2-1}\right|\)

Put \(x^2-1=t^2 \Rightarrow 2 x d x=2 t d t\)

∴ \(\mathrm{I}=\frac{1}{2} \int \frac{2 t}{t} \mathrm{dt}+2 \log \left|\mathrm{x}+\sqrt{\mathrm{x}^2-1}\right|=\int 1 \mathrm{dt}+2 \log \left|\mathrm{x}+\sqrt{\mathrm{x}^2-1}\right|\)

= \(\mathrm{t}+2 \log \left|\mathrm{x}+\sqrt{\mathrm{x}^2-1}\right|+\mathrm{C}=\sqrt{\mathrm{x}^2-1}+2 \log \left|\mathrm{x}+\sqrt{\mathrm{x}^2-1}\right|+\mathrm{C}\)

Question 18. \(\int \frac{5 x-2}{1+2 x+3 x^2} d x\)
Solution:

Let \(I=\int \frac{5 x-2}{1+2 x+3 x^2} d x\)

Let \(5 \mathrm{x}-2=\mathrm{A} \frac{\mathrm{d}}{\mathrm{dx}}\left(1+2 \mathrm{x}+3 \mathrm{x}^2\right)+\mathrm{B} \Rightarrow 5 \mathrm{x}-2=\mathrm{A}(2+6 \mathrm{x})+\mathrm{B}\)

Equating the coefficients of x and the constant term on both sides, we get

6\(\mathrm{~A}=5 \Rightarrow \mathrm{A}=\frac{5}{6}, 2 \mathrm{~A}+\mathrm{B}=-2 \Rightarrow \mathrm{B}=-2-2 \mathrm{~A}=-\frac{11}{3} \Rightarrow 5 \mathrm{x}-2=\frac{5}{6}(2+6 \mathrm{x})-\frac{11}{3}\)

∴ \(\mathrm{I}=\int \frac{\frac{5}{6}(2+6 \mathrm{x})-\frac{11}{3}}{1+2 \mathrm{x}+3 \mathrm{x}^2} \mathrm{dx}=\frac{5}{6} \int \frac{2+6 \mathrm{x}}{1+2 \mathrm{x}+3 \mathrm{x}^2} \mathrm{dx}-\frac{11}{3} \int \frac{1}{1+2 \mathrm{x}+3 \mathrm{x}^2} \mathrm{dx}\)

Let \(I_1=\int \frac{2+6 x}{1+2 x+3 x^2} d x\) and \(I_2=\int \frac{1}{1+2 x+3 x^2} d x\)

∴ \(\mathrm{I}=\frac{5}{6} \mathrm{I}_1-\frac{11}{3} \mathrm{I}_2\)……(1)

Now, \(I_1=\int \frac{2+6 x}{1+2 x+3 x^2} d x=\log \left|1+2 x+3 x^2\right|+C_1\)….(2)

(because \(\int \frac{f^{\prime}(x)}{f(x)} d x=\log |f(x)|+C\))

⇒ \(I_2=\int \frac{1}{1+2 x+3 x^2} d x=\int \frac{1}{3\left[x^2+\frac{2 x}{3}+\frac{1}{3}\right]} d x=\frac{1}{3} \int \frac{1}{\left[\left(x+\frac{1}{3}\right)^2+\frac{2}{9}\right]} d x\)

= \(\frac{1}{3} \int \frac{1}{\left(x+\frac{1}{3}\right)^2+\left(\frac{\sqrt{2}}{3}\right)} d x=\frac{1}{3} \cdot \frac{1}{(\sqrt{2} / 3)} \tan ^{-1}\left\{\frac{(x+1 / 3)}{\sqrt{2} / 3}\right\}+C_2\)

⇒ \(I_2=\frac{1}{\sqrt{2}} \tan ^{-1}\left(\frac{3 x+1}{\sqrt{2}}\right)+C_2\)

Using eq. (2) and (3) in eq. (1)

∴ \(\mathrm{I}=\frac{5}{6} \log \left|1+2 \mathrm{x}+3 \mathrm{x}^2\right|+\frac{5}{6} \mathrm{C}_1-\frac{11}{3}\left[\frac{1}{\sqrt{2}} \tan ^{-1}\left(\frac{3 \mathrm{x}+1}{\sqrt{2}}\right)\right]-\frac{11}{3} \mathrm{C}_2\)

= \(\frac{5}{6} \log \left|1+2 \mathrm{x}+3 \mathrm{x}^2\right|-\frac{11}{3 \sqrt{2}} \tan ^{-1}\left(\frac{3 \mathrm{x}+1}{\sqrt{2}}\right)+\mathrm{C}\)

where \(\mathrm{C}=\frac{5}{6} \mathrm{C}_1-\frac{11}{3} \mathrm{C}_2\)

Question 19. \(\int \frac{6 x+7}{\sqrt{(x-5)(x-4)}} d x\)
Solution:

Let \(I=\int \frac{6 x+7}{\sqrt{(x-5)(x-4)}} d x=\int \frac{6 x+7}{\sqrt{x^2-9 x+20}} d x\)

Let \(6 x+7=A \frac{d}{d x}\left(x^2-9 x+20\right)+B \Rightarrow 6 x+7=A(2 x-9)+B\)

Equating the coefficients of x and the constant term, we get

⇒ \(2 \mathrm{~A}=6 \Rightarrow \mathrm{A}=3\)

⇒ \(-9 \mathrm{~A}+\mathrm{B}=7 \Rightarrow \mathrm{B}=7+9 \mathrm{~A}=34\)

⇒ \(6 \mathrm{x}+7=3(2 \mathrm{x}-9)+34\)

∴ \(\mathrm{I}=\int \frac{3(2 \mathrm{x}-9)+34}{\sqrt{\mathrm{x}^2-9 x+20}} d x=3 \int \frac{2 \mathrm{x}-9}{\sqrt{\mathrm{x}^2-9 \mathrm{x}+20}} \mathrm{dx}+34 \int \frac{1}{\sqrt{\mathrm{x}^2-9 \mathrm{x}+20}} \mathrm{dx}\)

Let \(I_1=\int \frac{2 x-9}{\sqrt{x^2-9 x+20}} d x\) and \(I_2=\int \frac{1}{\sqrt{x^2-9 x+20}} d x\)

∴ \(\mathrm{I}=3 \mathrm{I}_1+34 \mathrm{I}_2\)….(1)

Now, \(I_1=\int \frac{2 x-9}{\sqrt{x^2-9 x+20}} d x\)

Put \(\mathrm{x}^2-9 \mathrm{x}+20=\mathrm{t}^2 \Rightarrow(2 \mathrm{x}-9) \mathrm{dx}=2 \mathrm{t} d \mathrm{t}\)

∴ \(I_1=\int \frac{2 t}{t} d t=2 \int 1 d t=2 t+C_1=2 \sqrt{x^2-9 x+20}+C_1\)….(2)

and \(I_2=\int \frac{1}{\sqrt{x^2-9 x+20}} d x=\int \frac{1}{\sqrt{\left(x-\frac{9}{2}\right)^2-\frac{1}{4}}} d x\)

⇒ \(I_2=\log \left|\left(x-\frac{9}{2}\right)+\sqrt{x^2-9 x+20}\right|+C_2\)

Using equations (2) and (3) in (1), we get

I = \(3\left[2 \sqrt{x^2-9 x+20}\right]+3 C_1+34 \log \left[\left(x-\frac{9}{2}\right)+\sqrt{x^2-9 x+20}\right]+34 C_2\)

∴ I = \(6 \sqrt{x^2-9 x+20}+34 \log \left[\left(x-\frac{9}{2}\right)+\sqrt{x^2-9 x+20}\right]+ \) where \(C=3 C_1+34 C_2\)

Question 20. \(\int \frac{\mathrm{x}+2}{\sqrt{4 \mathrm{x}-\mathrm{x}^2}} \mathrm{dx}\)
Solution:

Let \(I=\int \frac{x+2}{\sqrt{4 x-x^2}} d x\)

Let \(\mathrm{x}+2=\mathrm{A} \frac{\mathrm{d}}{\mathrm{dx}}\left(4 \mathrm{x}-\mathrm{x}^2\right)+\mathrm{B} \Rightarrow \mathrm{x}+2=\mathrm{A}(4-2 \mathrm{x})+\mathrm{B}\)

Equating the coefficients of x and constant term on both sides, we get \(-2 \mathrm{~A}=1 \Rightarrow \mathrm{A}=\frac{-1}{2} \Rightarrow 4 \mathrm{~A}+\mathrm{B}=2 \Rightarrow \mathrm{B}=2-4 \mathrm{~A}=4 \Rightarrow(\mathrm{x}+2)=-\frac{1}{2}(4-2 \mathrm{x})+4\)

∴ \(\mathrm{I}=\int \frac{-\frac{1}{2}(4-2 \mathrm{x})+4}{\sqrt{4 \mathrm{x-x^{2 }}}} \mathrm{dx}=-\frac{1}{2} \int \frac{4-2 \mathrm{x}}{\sqrt{4 \mathrm{x}-\mathrm{x}^2}} \mathrm{dx}+4 \int \frac{1}{\sqrt{4 \mathrm{x}-\mathrm{x}^2}} d x\)

Let \(I_1=\int \frac{4-2 x}{\sqrt{4 x-x^2}} d x\) and \(I_2=\int \frac{1}{\sqrt{4 x-x^2}} d x\)

∴ I = \(-\frac{1}{2} I_1+4 I_2\)….(1)

Now, \(I_1=\int \frac{4-2 x}{\sqrt{4 x-x^2}} d x\)

Put \(4 \mathrm{x}-\mathrm{x}^2=\mathrm{t}^2 \Rightarrow(4-2 \mathrm{x}) \mathrm{dx}=2 \mathrm{tdt}\)

∴ \(I_1=\int \frac{2 t}{t} d t=2 \int 1 d t=2 t+C_1=2 \sqrt{4 x-x^2}+C_1\)….(2)

Again, \(I_2=\int \frac{1}{\sqrt{4 x-x^2}} d x=\int \frac{1}{\sqrt{4-(x-2)^2}} d x\)

= \(\int \frac{1}{\sqrt{(2)^2-(x-2)^2}} d x=\sin ^{-1}\left(\frac{x-2}{2}\right)+C_2\)

Using equations (2) and (3) in (1), we get

I = \(-\frac{1}{2}\left(2 \sqrt{4 x-x^2}\right)-\frac{1}{2} C_1+4 \sin ^{-1}\left(\frac{x-2}{2}\right)+4 C_2\)

= \(-\sqrt{4 x-x^2}+4 \sin ^{-1}\left(\frac{x-2}{2}\right)+C\) where C=4 \(C_2-\frac{1}{2} C_1\)

Question 21. \(\int \frac{\mathrm{x}+2}{\sqrt{\mathrm{x}^2+2 \mathrm{x}+3}} \mathrm{dx}\)
Solution:

Let \(I=\int \frac{x+2}{\sqrt{x^2+2 x+3}} d x=\frac{1}{2} \int \frac{2(x+2)}{\sqrt{x^2+2 x+3}} d x=\frac{1}{2} \int \frac{(2 x+2)+2}{\sqrt{x^2+2 x+3}} d x\)

= \(\frac{1}{2} \int \frac{2 \mathrm{x}+2}{\sqrt{\mathrm{x}^2+2 \mathrm{x}+3}} \mathrm{dx}+\frac{1}{2} \int \frac{2}{\sqrt{\mathrm{x}^2+2 \mathrm{x}+3}} \mathrm{dx}=\frac{1}{2} \int \frac{2 \mathrm{x}+2}{\sqrt{\mathrm{x}^2+2 \mathrm{x}+3}} \mathrm{dx}+\int \frac{1}{\sqrt{\mathrm{x}^2+2 \mathrm{x}+3}} \mathrm{dx}\)

Let \(I_1=\int \frac{2 x+2}{\sqrt{x^2+2 x+3}} d x\) and \(I_2=\int \frac{1}{\sqrt{x^2+2 x+3}} d x\)

∴ I=\(\frac{1}{2} I_1+I_2\)…..(1)

Now, \(\mathrm{I}_1=\int \frac{2 \mathrm{x}+2}{\sqrt{\mathrm{x}^2+2 \mathrm{x}+3}} \mathrm{dx}\)

Put \(\mathrm{x}^2+2 \mathrm{x}+3=\mathrm{t}^2 \Rightarrow(2 \mathrm{x}+2) \mathrm{dx}=2 \mathrm{tdt}\)

∴ \(I_1=\int \frac{2 t}{t} d t=2 \int 1 d t=2 t+C_1 \Rightarrow I_1=2 \sqrt{x^2+2 x+3}+C_1\)…..(2)

Again, \(\mathrm{J}_2=\int \frac{1}{\sqrt{\mathrm{x}^2+2 \mathrm{x}+3}} \mathrm{dx}=\int \frac{1}{\sqrt{(\mathrm{x}+1)^2+(\sqrt{2})^2}} \mathrm{dx}\)

∴ \(I_2=\log \left|(x+1)+\sqrt{x^2+2 x+3}\right|+C_2\)…..(3)

Using equations (2) and (3) in (1), we get

I = \(\frac{1}{2}\left[2 \sqrt{x^2+2 x+3}\right]+\frac{1}{2} C_1+\log \left|(x+1)+\sqrt{x^2+2 x+3}\right|+C_2\)

= \(\sqrt{x^2+2 x+3}+\log \left|(x+1)+\sqrt{x^2+2 x+3}\right|+C\) where C = \(\frac{1}{2} C_1+C_2\)

Question 22. \(\int \frac{x+3}{x^2-2 x-5} d x\)
Solution:

Let \(I=\int \frac{x+3}{x^2-2 x-5} d x\)

Let \(\mathrm{x}+3=\mathrm{A} \frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{x}^2-2 \mathrm{x}-5\right)+\mathrm{B} \Rightarrow \mathrm{x}+3=\mathrm{A}(2 \mathrm{x}-2)+\mathrm{B}\)

Equating the coefficients of x and the constant term on both sides, we obtain

2\(\mathrm{~A}=1 \Rightarrow \mathrm{A}=\frac{1}{2} \Rightarrow-2 \mathrm{~A}+\mathrm{B}=3 \Rightarrow \mathrm{B}=3+2 \mathrm{~A}=4\)

therefore \((\mathrm{x}+3)=\frac{1}{2}(2 \mathrm{x}-2)+4\)

∴ \(\mathrm{I}=\int \frac{\frac{1}{2}(2 \mathrm{x}-2)+4}{\mathrm{x}^2-2 \mathrm{x}-5} \mathrm{dx}=\frac{1}{2} \int \frac{(2 \mathrm{x}-2)}{\mathrm{x}^2-2 \mathrm{x}-5} \mathrm{dx}+4 \int \frac{1}{\mathrm{x}^2-2 \mathrm{x}-5} \mathrm{dx}\)

Let \(I_1=\int \frac{2 x-2}{x^2-2 x-5} d x\) and \(I_2=\int \frac{1}{x^2-2 x-5} d x\)

∴ \(\mathrm{I}=\frac{1}{2} \mathrm{I}_1+4 \mathrm{I}_2\)…..(1)

Now, \(I_1=\int \frac{2 x-2}{x^2-2 x-5} d x\)

Put \(x^2-2 x-5=t \Rightarrow(2 x-2) d x=d t=\int \frac{d t}{t}=\log |t|+C_1\)

∴ \(I_1=\log \left|x^2-2 x-5\right|+C_1\)….(2)

Again, \(I_2=\int \frac{1}{x^2-2 x-5} d x=\int \frac{1}{\left(x^2-2 x+1\right)-6} d x=\int \frac{1}{(x-1)^2-(\sqrt{6})^2} d x\)

= \(\frac{1}{2 \sqrt{6}} \log \left(\frac{x-1-\sqrt{6}}{x-1+\sqrt{6}}\right)+C_2\)….(3)

Using equations (2) and (3) in (1), we get:

I = \(\frac{1}{2} \log \left|x^2-2 x-5\right|+\frac{1}{2} C_1+\frac{4}{2 \sqrt{6}} \log \left|\frac{x-1-\sqrt{6}}{x-1+\sqrt{6}}\right|+4 C_2\)

= \(\frac{1}{2} \log \left|x^2-2 x-5\right|+\frac{2}{\sqrt{6}} \log \left|\frac{x-1-\sqrt{6}}{x-1+\sqrt{6}}\right|+C \text { where } C=\frac{1}{2} C_1+4 C_2\)

Question 23. \(\int \frac{5 x+3}{\sqrt{x^2+4 x+10}} d x\)
Solution:

Let \(I=\int \frac{5 x+3}{\sqrt{x^2+4 x+10}} d x\)

Let \(5 \mathrm{x}+3=\mathrm{A} \frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{x}^2+4 \mathrm{x}+10\right)+\mathrm{B} \Rightarrow 5 \mathrm{x}+3=\mathrm{A}(2 \mathrm{x}+4)+\mathrm{B}\)

Equating the coefficients of x and the constant term, we get

⇒ \(2 \mathrm{~A}=5 \Rightarrow \mathrm{A}=\frac{5}{2}, 4 \mathrm{~A}+\mathrm{B}=3\)

⇒ \(\mathrm{B}=3-4\left(\frac{5}{2}\right)=-7 \Rightarrow 5 \mathrm{x}+3=\frac{5}{2}(2 \mathrm{x}+4)-7\)

∴ \(\mathrm{I}=\int \frac{\frac{5}{2}(2 \mathrm{x}+4)-7}{\sqrt{\mathrm{x}^2+4 \mathrm{x}+10}} \mathrm{dx}=\frac{5}{2} \int \frac{2 \mathrm{x}+4}{\sqrt{\mathrm{x}^2+4 \mathrm{x}+10}} \mathrm{dx}-7 \int \frac{1}{\sqrt{\mathrm{x}^2+4 \mathrm{x}+10}} \mathrm{dx}\)

Let, \(I_1=\int \frac{2 x+4}{\sqrt{x^2+4 x+10}} d x\) and \(I_2=\int \frac{1}{\sqrt{x^2+4 x+10}} d x\)

∴ \(\mathrm{I}=\frac{5}{2} \mathrm{I}_1-7 \mathrm{I}_2\)

Now, \(\mathrm{I}_1=\int \frac{2 \mathrm{x}+4}{\sqrt{\mathrm{x}^2+4 \mathrm{x}+10}} \mathrm{dx}\)

Put \(x^2+4 x+10=t^2 \Rightarrow(2 x+4) d x=2 t d t\)

∴ \(I_1=\int \frac{2 t}{t} d t=2 \int 1 d t=2 t+C_1=2 \sqrt{x^2+4 x+10}+C_1\)

Again, \(I_2=\int \frac{1}{\sqrt{x^2+4 x+10}} d x=\int \frac{1}{\sqrt{\left(x^2+4 x+4\right)+6}} d x=\int \frac{1}{\sqrt{(x+2)^2+(\sqrt{6})^2}} d x\)

= \(\log \left|(\mathrm{x}+2)+\sqrt{\mathrm{x}^2+4 \mathrm{x}+10}\right|+\mathrm{C}_2\)

Using equations (2) and (3) in (1), we get

I = \(\frac{5}{2}\left[2 \sqrt{x^2+4 x+10}\right]+\frac{5}{2} C_1-7 \log \left|(x+2)+\sqrt{x^2+4 x+10}\right|-7 C_2\),

= \(5 \sqrt{x^2+4 x+10}-7 \log \left|(x+2)+\sqrt{x^2+4 x+10}\right|+C ; \text { where } C=\frac{5}{2} C_1-7 C_2\)

Choose The Correct Answer In The Following

Question 24. \(\int \frac{\mathrm{dx}}{\mathrm{x}^2+2 \mathrm{x}+2}\) equals ?

1. \(x \tan ^{-1}(x+1)+C\)
2. \(\tan ^{-1}(x+1)+C\)
3. \((x+1) \tan ^{-1} x+C\)
4. \(\tan ^{-1} x+C\)

Solution: 2. \(\tan ^{-1}(x+1)+C\)

Let \(\tan ^{-1}(x+1)+C\)

I = \(\int \frac{\mathrm{dx}}{\mathrm{x}^2+2 \mathrm{x}+2}\)

= \(\int \frac{\mathrm{dx}}{\left(\mathrm{x}^2+2 \mathrm{x}+1\right)+1}=\int \frac{1}{(\mathrm{x}+1)^2+(1)^2} \mathrm{dx}\)

= \(\left[\tan ^{-1}(\mathrm{x}+1)\right]+C\)

⇒ \(\tan ^{-1}(x+1)+C\)

Hence, the correct answer is (2).

Question 25. \(\int \frac{\mathrm{dx}}{\sqrt{9 \mathrm{x}-4 \mathrm{x}^2}}\) { equals?

1. \(\frac{1}{9} \sin ^{-1}\left(\frac{9 x-8}{8}\right)+C\)
2. \(\frac{1}{2} \sin ^{-1}\left(\frac{8 x-9}{9}\right)+C\)
3. \(\frac{1}{3} \sin ^{-1}\left(\frac{9 x-8}{8}\right)+C\)
4. \(\frac{1}{2} \sin ^{-1}\left(\frac{9 x-8}{9}\right)+C\)

Solution: 2. \(\frac{1}{2} \sin ^{-1}\left(\frac{8 x-9}{9}\right)+C\)

Let I = \(\int \frac{d x}{\sqrt{9 x-4 x^2}}=\int \frac{1}{\sqrt{-4\left(x^2-\frac{9}{4} x\right)}} d x=\int \frac{1}{\sqrt{-4\left(x^2-\frac{9}{4} x+\frac{81}{64}-\frac{81}{64}\right)}} d x\)

= \(\int \frac{1}{\left.\sqrt{-4\left[\left(x-\frac{9}{8}\right)^2-\left(\frac{9}{8}\right)^2\right.}\right]} d x=\frac{1}{2} \int \frac{1}{\sqrt{\left(\frac{9}{8}\right)^2-\left(x-\frac{9}{8}\right)^2}} d x\)

= \(\frac{1}{2}\left[\sin ^{-1}\left(\frac{x-\frac{9}{8}}{\frac{9}{8}}\right)\right]+C=\frac{1}{2} \sin ^{-1}\left(\frac{8 x-9}{9}\right)+C\)

Hence, the correct answer is (2).

Integrals Exercise 7.5

Integrate The Rational Functions

Question 1. \(\int \frac{x}{(x+1)(x+2)} d x\)
Solution:

Let \(I=\int \frac{x}{(x+1)(x+2)} d x\)

Let \(\frac{x}{(x+1)(x+2)}=\frac{A}{(x+1)}+\frac{B}{(x+2)}\)

x=A(x+2)+B(x+1)…..(1)

In equation (1)

Put x=-1 ⇒ A=-1

x=-2 ⇒ -B=-2 ⇒ B=2

∴ \(\frac{x}{(x+1)(x+2)}=\frac{-1}{(x+1)}+\frac{2}{(x+2)}\)

∴ I = \(\int \frac{-1}{(x+1)} d x+\int \frac{2}{(x+2)} d x\)

= \(-\log |x+1|+2 \log |x+2|+C=\log (x+2)^2-\log |x+1|+C=\log \left|\frac{(x+2)^2}{(x+1)}\right|+C\)

Question 2. \(\int \frac{1}{x^2-9} d x\)
Solution:

Let \(I=\int \frac{1}{x^2-9} d x=\int \frac{1}{(x+3)(x-3)} d x\)

Let \(\frac{1}{(x+3)(x-3)}=\frac{A}{(x+3)}+\frac{B}{(x-3)} \Rightarrow 1=A(x-3)+B(x+3)\)…..(1)

From equation (1)

Put \(x=-3 \Rightarrow-6 A=1 \Rightarrow A=\frac{-1}{6}\) and \(x=3 \Rightarrow 6 B=1 \Rightarrow B=\frac{1}{6}\)

∴ \(\frac{1}{(x+3)(x-3)}=\frac{-1}{6(x+3)}+\frac{1}{6(x-3)}\)

∴ I = \(\int\left(\frac{-1}{6(x+3)}+\frac{1}{6(x-3)}\right) d x\)

= \(-\frac{1}{6} \log |x+3|+\frac{1}{6} \log |x-3|+C=\frac{1}{6} \log \left|\frac{(x-3)}{(x+3)}\right|+C\)

Question 3. \(\int \frac{3 x-1}{(x-1)(x-2)(x-3)} d x\)
Solution:

Let \(I=\int \frac{3 x-1}{(x-1)(x-2)(x-3)} d x\)

Let \(\frac{3 x-1}{(x-1)(x-2)(x-3)}=\frac{A}{(x-1)}+\frac{B}{(x-2)}+\frac{C}{(x-3)}\)

3\(\mathrm{x}-1=\mathrm{A}(\mathrm{x}-2)(\mathrm{x}-3)+\mathrm{B}(\mathrm{x}-1)(\mathrm{x}-3)+\mathrm{C}(\mathrm{x}-1)(\mathrm{x}-2)\)….(1)

Put x=1,2, and 3 in equation (1), we get

A=1, \(\mathrm{~B}=-5\), and \(\mathrm{C}=4\) respectively

Now, \(\int \frac{3 x-1}{(x-1)(x-2)(x-3)} d x=\int\left(\frac{1}{(x-1)}-\frac{5}{(x-2)}+\frac{4}{(x-3)}\right) d x\)

= \(\log |\mathrm{x}-1|-5 \log |\mathrm{x}-2|+4 \log |\mathrm{x}-3|+\mathrm{C}\)

Question 4. \(\int \frac{x}{(x-1)(x-2)(x-3)} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{x}}{(\mathrm{x}-1)(\mathrm{x}-2)(\mathrm{x}-3)} \mathrm{dx}\)

Let \(\frac{x}{(x-1)(x-2)(x-3)}=\frac{A}{(x-1)}+\frac{B}{(x-2)}+\frac{C}{(x-3)}\)

x= \(\mathrm{A}(\mathrm{x}-2)(\mathrm{x}-3)+\mathrm{B}(\mathrm{x}-1)(\mathrm{x}-3)+\mathrm{C}(\mathrm{x}-1)(\mathrm{x}-2)\)……(1)

Put x=1,2 and 3 in equation (1), and we get \(\mathrm{A}=\frac{1}{2}, \mathrm{~B}=-2\), and \(\mathrm{C}=\frac{3}{2}\) respectively

∴ \(\frac{x}{(x-1)(x-2)(x-3)}=\frac{1}{2(x-1)}-\frac{2}{(x-2)}+\frac{3}{2(x-3)}\)

∴ \(I=\int\left\{\frac{1}{2(x-1)}-\frac{2}{(x-2)}+\frac{3}{2(x-3)}\right\} d x=\frac{1}{2} \log |x-1|-2 \log |x-2|+\frac{3}{2} \log |x-3|+C\)

Question 5. \(\int \frac{2 x}{x^2+3 x+2} d x\)
Solution:

Let \(I=\int \frac{2 x}{x^2+3 x+2} d x, \frac{2 x}{x^2+3 x+2}=\frac{2 x}{(x+1)(x+2)}=\frac{A}{(x+1)}+\frac{B}{(x+2)}\)

⇒ \(2 \mathrm{x}=\mathrm{A}(\mathrm{x}+2)+\mathrm{B}(\mathrm{x}+1)\)….(1)

Put x=-1 and -2 equation (1), we get A=-2 and B=4 respectively

⇒ \(\frac{2 x}{(x+1)(x+2)}=\frac{-2}{(x+1)}+\frac{4}{(x+2)}\)

⇒ I = \(\int\left\{\frac{4}{(x+2)}-\frac{2}{(x+1)}\right\} d x=4 \log |x+2|-2 \log |x+1|+C\)

Question 6. \(\int \frac{1-x^2}{x(1-2 x)} d x\)
Solution:

Let \(I=\int \frac{1-x^2}{x(1-2 x)} d x\)

Given integrand is an improper rational function. So, on dividing we get \(\frac{1-x^2}{x(1-2 x)}=\frac{1}{2}+\frac{1}{2}\left(\frac{2-x}{x(1-2 x)}\right)\)….(1)

Let \(\frac{2-x}{x(1-2 x)}=\frac{A}{x}+\frac{B}{(1-2 x)} \Rightarrow 2-x=A(1-2 x)+B x\)

Put x=0 and \(\frac{1}{2}\) in equation (1), we get A=2 and B=3 respectively

∴ \(\frac{2-x}{x(1-2 x)}=\frac{2}{x}+\frac{3}{1-2 x}\)

∴ I = \(\int\left\{\frac{1}{2}+\frac{1}{2}\left(\frac{2}{x}+\frac{3}{1-2 x}\right)\right\} d x\)

= \(\frac{x}{2}+\log |x|+\frac{3}{2(-2)} \log |1-2 x|+C=\frac{x}{2}+\log |x|-\frac{3}{4} \log |1-2 x|+C\)

Question 7. \(\int \frac{x}{\left(x^2+1\right)(x-1)} d x\)
Solution:

Let \(I=\int \frac{x}{\left(x^2+1\right)(x-1)} d x\)

Put \(\frac{x}{\left(x^2+1\right)(x-1)}=\frac{A x+B}{\left(x^2+1\right)}+\frac{C}{(x-1)}, x=(A x+B)(x-1)+C\left(x^2+1\right)\)….(1)

In eq. (1), Put x=1 \(\Rightarrow C=\frac{1}{2}\)

Equating the coefficients of x² and the constant term, we get

A+C = \(0 \Rightarrow A=-C=-\frac{1}{2},-B+C=0 \Rightarrow B=C=\frac{1}{2}\)

∴ \(\frac{x}{\left(x^2+1\right)(x-1)}=\frac{\left(-\frac{1}{2} x+\frac{1}{2}\right)}{x^2+1}+\frac{\frac{1}{2}}{(x-1)}\)

∴ I = \(-\frac{1}{2} \int \frac{x}{x^2+1} d x+\frac{1}{2} \int \frac{1}{x^2+1} d x+\frac{1}{2} \int \frac{1}{x-1}\)

= \(-\frac{1}{4} \int \frac{2 x}{x^2+1} d x+\frac{1}{2} \tan ^{-1} x+\frac{1}{2} \log |x-1|+C\)

= \(-\frac{1}{4} \log \left|\mathrm{x}^2+1\right|+\frac{1}{2} \tan ^{-1} \mathrm{x}+\frac{1}{2} \log |\mathrm{x}-1|+\mathrm{C}\)

= \(\frac{1}{2} \log |\mathrm{x}-1|-\frac{1}{4} \log \left|\mathrm{x}^2+1\right|+\frac{1}{2} \tan ^{-1} \mathrm{x}+\mathrm{C}\)

Question 8. \(\int \frac{x}{(x-1)^2(x+2)} d x\)
Solution:

Let \(I=\int \frac{x}{(x-1)^2(x+2)} d x\)

Let \(\frac{x}{(x-1)^2(x+2)}=\frac{A}{(x-1)}+\frac{B}{(x-1)^2}+\frac{C}{(x+2)}\)

x = \(=\mathrm{A}(\mathrm{x}-1)(\mathrm{x}+2)+\mathrm{B}(\mathrm{x}+2)+\mathrm{C}(\mathrm{x}-1)^2\)

In equation (1)

Put x = \(1 \Rightarrow \mathrm{B}=\frac{1}{3}, \mathrm{x}=-2 \Rightarrow \mathrm{C}=-\frac{2}{9}\)

On equating the coefficients of \(\mathrm{x}^2, \mathrm{~A}+\mathrm{C}=0 \Rightarrow \mathrm{A}=-\mathrm{C}=\frac{2}{9}\)

∴ \(\frac{x}{(x-1)^2(x+2)}=\frac{2}{9(x-1)}+\frac{1}{3(x-1)^2}-\frac{2}{9(x+2)}\)

∴ I = \(\frac{2}{9} \int \frac{1}{(x-1)} d x+\frac{1}{3} \int \frac{1}{(x-1)^2} d x-\frac{2}{9} \int \frac{1}{(x+2)} d x\)

= \(\frac{2}{9} \log |x-1|+\frac{1}{3}\left(\frac{-1}{x-1}\right)-\frac{2}{9} \log |x+2|+C=\frac{2}{9} \log \left|\frac{x-1}{x+2}\right|-\frac{1}{3(x-1)}+C\)

Question 9. \(\int \frac{3 x+5}{x^3-x^2-x+1} d x\)
Solution:

Let \(I=\int \frac{3 x+5}{x^3-x^2-x+1} d x\)

Let \(\frac{3 x+5}{(x-1)^2(x+1)}=\frac{A}{(x-1)}+\frac{B}{(x-1)^2}+\frac{C}{(x+1)}\)

3 \(\mathrm{x}+5=\mathrm{A}(\mathrm{x}-1)(\mathrm{x}+1)+\mathrm{B}(\mathrm{x}+1)+\mathrm{C}(\mathrm{x}-1)^2\)…..(1)

Put x=1 and x=-1 in equation (1), we get B=4 and C = \(\frac{1}{2}\)

Equating the coefficients of \(x^2\) we get A+C=0 ⇒ A=-C=\(-\frac{1}{2}\)

∴ \(\frac{3 x+5}{(x-1)^2(x+1)}=\frac{-1}{2(x-1)}+\frac{4}{(x-1)^2}+\frac{1}{2(x+1)}\)

∴ I = \(-\frac{1}{2} \int \frac{1}{x-1} d x+4 \int \frac{1}{(x-1)^2} d x+\frac{1}{2} \int \frac{1}{(x+1)} d x\)

= \(-\frac{1}{2} \log |x-1|+4\left(\frac{-1}{x-1}\right)+\frac{1}{2} \log |x+1|+C\)

= \(\frac{1}{2} \log \left|\frac{x+1}{x-1}\right|-\frac{4}{(x-1)}+C\)

Question 10. \(\int \frac{2 \mathrm{x}-3}{\left(\mathrm{x}^2-1\right)(2 \mathrm{x}+3)} \mathrm{dx}\)
Solution:

Let I = \(\int \frac{2 x-3}{\left(x^2-1\right)(2 x+3)} d x=\int \frac{2 x-3}{(x+1)(x-1)(2 x+3)} \cdot d x\)

Let \(\frac{2 x-3}{(x+1)(x-1)(2 x+3)}=\frac{A}{(x+1)}+\frac{B}{(x-1)}+\frac{C}{(2 x+3)}\)

⇒ \((2 \mathrm{x}-3)=\mathrm{A}(\mathrm{x}-1)(2 \mathrm{x}+3)+\mathrm{B}(\mathrm{x}+1)(2 \mathrm{x}+3)+\mathrm{C}(\mathrm{x}+1)(\mathrm{x}-1)\)….(1)

Put x=-1,1 and \(-\frac{3}{2}\) in eq. (1), we get

∴ \(\mathrm{A}=\frac{5}{2}, \mathrm{~B}=-\frac{1}{10}, \mathrm{C}=-\frac{24}{5}\) respectively

∴ \(\frac{2 x-3}{(x+1)(x-1)(2 x+3)}=\frac{5}{2(x+1)}-\frac{1}{10(x-1)}-\frac{24}{5(2 x+3)} \)

∴ \(\mathrm{I}=\frac{5}{2} \int \frac{1}{(\mathrm{x}+1)} \mathrm{dx}-\frac{1}{10} \int \frac{1}{\mathrm{x}-1} \mathrm{dx}-\frac{24}{5} \int \frac{1}{(2 \mathrm{x}+3)} \mathrm{dx}\)

= \(\frac{5}{2} \log |\mathrm{x}+1|-\frac{1}{10} \log |\mathrm{x}-1|-\frac{24}{5 \times 2} \log |2 \mathrm{x}+3|+\mathrm{C}\)

= \(\frac{5}{2} \log |x+1|-\frac{1}{10} \log |x-1|-\frac{12}{5} \log |2 x+3|+C\)

Question 11. \(\int \frac{5 x}{(x+1)\left(x^2-4\right)} d x\)
Solution:

Let \(I=\int \frac{5 x}{(x+1)\left(x^2-4\right)} d x=\int \frac{5 x}{(x+1)(x+2)(x-2)} d x\)

Let \(\frac{5 x}{(x+1)(x+2)(x-2)}=\frac{A}{(x+1)}+\frac{B}{(x+2)}+\frac{C}{(x-2)}\)

5\(\mathrm{x}=\mathrm{A}(\mathrm{x}+2)(\mathrm{x}-2)+\mathrm{B}(\mathrm{x}+1)(\mathrm{x}-2)+\mathrm{C}(\mathrm{x}+1)(\mathrm{x}+2)\)…..(1)

Put x=-1,-2, and 2 in equation (1), we get

∴ \(\mathrm{A}=\frac{5}{3}, \mathrm{~B}=-\frac{5}{2}\) and \(\mathrm{C}=\frac{5}{6}\) respectively

∴ \(\frac{5 x}{(x+1)(x+2)(x-2)}=\frac{5}{3(x+1)}-\frac{5}{2(x+2)}+\frac{5}{6(x-2)}\)

I = \(\frac{5}{3} \int \frac{1}{(x+1)} d x-\frac{5}{2} \int \frac{1}{(x+2)} d x+\frac{5}{6} \int \frac{1}{(x-2)} d x\)

= \(\frac{5}{3} \log |x+1|-\frac{5}{2} \log |x+2|+\frac{5}{6} \log |x-2|+C\)

Question 12. \(\int \frac{x^3+x+1}{x^2-1} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{x}^3+\mathrm{x}+1}{\mathrm{x}^2-1} \mathrm{dx}\)

Given integrand is an improper rational function.

So, on dividing \(\left(x^3+x+1\right)\) by \(x^2-1\), we get \(\frac{x^3+x+1}{x^2-1}=x+\frac{2 x+1}{x^2-1}\)

Let \(\frac{2 x+1}{x^2-1}=\frac{A}{(x+1)}+\frac{B}{(x-1)} \Rightarrow 2 x+1=A(x-1)+B(x+1)\)

Put x=-1 and 1 in equation (1), we get \(A=\frac{1}{2}\) and \(B=\frac{3}{2}\)

∴ \(\frac{x^3+x+1}{x^2-1}=x+\frac{1}{2(x+1)}+\frac{3}{2(x-1)}\)

∴ I = \(\int x d x+\frac{1}{2} \int \frac{1}{(x+1)} d x+\frac{3}{2} \int \frac{1}{(x-1)} d x=\frac{x^2}{2}+\frac{1}{2} \log |x+1|+\frac{3}{2} \log |x-1|+C\)

Question 13. \(\int \frac{2}{(1-\mathrm{x})\left(1+\mathrm{x}^2\right)} \mathrm{dx}\)
Solution:

Let \(I=\int \frac{2}{(1-x)\left(1+x^2\right)} d x=\int \frac{2}{(1-x)\left(1+x^2\right)} d x\)

Let \(\frac{2}{(1-x)\left(1+x^2\right)}=\frac{A}{(1-x)}+\frac{B x+C}{\left(1+x^2\right)}\)

2 = \(A\left(1+x^2\right)+(B x+C)(1-x)\)….(1)

In equation (1), Put x=1 ⇒ A=1

Now, on equating the coefficient of \(x^2\) and constant term, we get

∴ \(\mathrm{A}-\mathrm{B}=0 \Rightarrow \mathrm{B}=\mathrm{A}=1\)

∴ \(\mathrm{~A}+\mathrm{C}=2 \Rightarrow \mathrm{C}=2-\mathrm{A}=1\)

∴ \(\frac{2}{(1-\mathrm{x})\left(1+\mathrm{x}^2\right)}=\frac{1}{1-\mathrm{x}}+\frac{\mathrm{x}+1}{1+\mathrm{x}^2}\)

⇒ \(\mathrm{I}=\int \frac{1}{1-\mathrm{x}} \mathrm{dx}+\int \frac{\mathrm{x}}{1+\mathrm{x}^2} \mathrm{dx}+\int \frac{1}{1+\mathrm{x}^2} \mathrm{dx}\)

= \(-\int \frac{1}{\mathrm{x}-1} \mathrm{dx}+\frac{1}{2} \int \frac{2 \mathrm{x}}{1+\mathrm{x}^2} \mathrm{dx}+\int \frac{1}{1+\mathrm{x}^2} \mathrm{dx}\)

= \(-\log |\mathrm{x}-1|+\frac{1}{2} \log \left|1+\mathrm{x}^2\right|+\tan ^{-1} \mathrm{x}+\mathrm{C}\)

Question 14. \(\int \frac{3 x-1}{(x+2)^2} d x\)
Solution:

Let \(I=\int \frac{3 x-1}{(x+2)^2} d x=\int \frac{3 x-1}{(x+2)^2} d x\)

Let \(\frac{3 x-1}{(x+2)^2}=\frac{A}{(x+2)}+\frac{B}{(x+2)^2} \Rightarrow 3 x-1=A(x+2)+B\)

In eq. (1), Put x=-2 ⇒ B=-7

On equating the coefficients of x, we get A=3

∴ \(\frac{3 x-1}{(x+2)^2}=\frac{3}{(x+2)}-\frac{7}{(x+2)^2}\)

⇒ I = \(3 \int \frac{1}{(x+2)} d x-7 \int \frac{1}{(x+2)^2} d x=3 \log |x+2|-7\left(\frac{-1}{(x+2)}\right)+C=3 \log |x+2|+\frac{7}{(x+2)}+C\)

Question 15. \(\int \frac{1}{\mathrm{x}^4-1} \mathrm{dx}\)
Solution:

Let \(I=\int \frac{1}{x^4-1} d x \Rightarrow \frac{1}{\left(x^4-1\right)}=\frac{1}{\left(x^2-1\right)\left(x^2+1\right)}=\frac{1}{(x+1)(x-1)\left(1+x^2\right)}\)

Let \(\frac{1}{(x+1)(x-1)\left(x^2+1\right)}=\frac{A}{(x+1)}+\frac{B}{(x-1)}+\frac{C x+D}{\left(x^2+1\right)}\)

I = \(A(x-1)\left(x^2+1\right)+B(x+1)\left(x^2+1\right)+(C x+D)\left(x^2-1\right)\)….(1)

In equation (1)

Put x=-1 and 1 we get \(A=-\frac{1}{4}\) and \(B=\frac{1}{4}\)

On equating the coefficients of x³ and the constant term, we get

A+B+C =0 ⇒ C=-(A+B)=0

-A+B-D=1 ⇒ D=-A+B-1= \(-\frac{1}{2}\)

∴ \(\frac{1}{x^4-1}=\frac{-1}{4(x+1)}+\frac{1}{4(x-1)}-\frac{1}{2\left(x^2+1\right)}\)

∴ I = \(-\frac{1}{4} \int \frac{1}{x+1} d x+\frac{1}{4} \int \frac{1}{x-1} d x-\frac{1}{2} \int \frac{1}{x^2+1} d x\)

= \(-\frac{1}{4} \log |x+1|+\frac{1}{4} \log |x-1|-\frac{1}{2} \tan ^{-1} x+C=\frac{1}{4} \log \left|\frac{x-1}{x+1}\right|-\frac{1}{2} \tan ^{-1} x+C\)

Question 16. \(\int \frac{1}{x\left(x^n+1\right)} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{1}{\mathrm{x}\left(\mathrm{x}^{\mathrm{n}}+1\right)} \mathrm{dx}\)

⇒ \(\frac{1}{\mathrm{x}\left(\mathrm{x}^n+1\right)}=\frac{\mathrm{x}^{n-1}}{\mathrm{x}^n\left(\mathrm{x}^n+1\right)}\) (because Multiplying numerator and denominator by \(\mathrm{x}^{n-1}\))

Put \(\mathrm{x}^{\mathrm{t}}=\mathrm{t} \Rightarrow \mathrm{nx}^{\mathrm{s}-1} \mathrm{dx}=\mathrm{dt}\)

∴ I = \(\int \frac{x^{n-1}}{x^n\left(x^n+1\right)} d x=\frac{1}{n} \int \frac{1}{t(t+1)} d t\)

⇒ \(\frac{1}{n} \int\left\{\frac{1}{t}-\frac{1}{(t+1)}\right\} d x=\frac{1}{n}[\log |t|-\log |t+1|]+C\)

= \(\frac{1}{n}\left[\log \left|x^n\right|-\log \left|x^n+1\right|\right]+C=\frac{1}{n} \log \left|\frac{x^n}{x^n+1}\right|+C\)

Alternate :

I = \(\int \frac{1}{x\left(x^n+1\right)} d x=\int \frac{1}{x^{n+1}\left(1+x^{-n}\right)} d x\)

Put \(1+x^{-n}=t \Rightarrow-n x^{-a-1} d x=d t \Rightarrow \frac{1}{x^{n+1}} d x=-\frac{d t}{n}\)

∴ I = \(-\frac{1}{n} \int_t^1 \frac{d t}{t}=-\frac{1}{n} \log |t|+C=-\frac{1}{n} \log \left|1+x^{-n}\right|+C=\frac{1}{n} \log \left|\frac{x^n}{x^n+1}\right|+C\)

Question 17. \(\int \frac{\cos x}{(1-\sin x)(2-\sin x)} d x\)
Solution:

Let I = \(\int \frac{\cos x}{(1-\sin x)(2-\sin x)} d x\)

Put sin x=t ⇒ cos x dx = dt

∴ I = \(\int \frac{d t}{(1-t)(2-t)}\)

Let \(\frac{1}{(1-t)(2-t)}=\frac{A}{(1-t)}+\frac{B}{(2-t)}\)

I = \(\mathrm{A}(2-\mathrm{t})+\mathrm{B}(1-\mathrm{t})\)….(1)

Put t=1 and t=2 in equation (1), we get A=1 and B=-1 respectively

∴ \(\frac{1}{(1-t)(2-t)}=\frac{1}{(1-t)}-\frac{1}{(2-t)}\)

⇒ \(I=\int\left\{\frac{1}{1-t}-\frac{1}{(2-t)}\right\} d x=-\log |1-t|+\log |2-t|+C\)

= \(\log \left|\frac{2-t}{1-t}\right|+C=\log \left|\frac{2-\sin x}{1-\sin x}\right|+C\)

Question 18. \(\int \frac{\left(x^2+1\right)\left(x^2+2\right)}{\left(x^2+3\right)\left(x^2+4\right)} d x\)
Solution:

Let \(I=\int \frac{\left(x^2-1\right)\left(x^2+2\right)}{\left(x^2+3\right)\left(x^2+4\right)} d x, x^2=y\), then \(\frac{(y+1)(y+2)}{(y+3)(y+4)}=1+\frac{(-4 y-10)}{(y+3)(y+4)}=1+\frac{A}{y+3}+\frac{B}{y+4}\)

y+1)(y+2)=(y+3)(y+4)+A(y+4)+B(y+3) …..(1)

In equation (1)

Put y=-3 and y=-4, we get A=2 and B=-6 respectively

∴ \(\frac{(y+1)(y+2)}{(y+3)(y+4)}=1+\frac{2}{(y+3)}-\frac{6}{(y+4)}\)

∴ \(\frac{\left(x^2+1\right)\left(x^2+2\right)}{\left(x^2+3\right)\left(x^2+4\right)}\)

= \(1+\frac{2}{x^2+3}-\frac{6}{x^2+4}\) (because \(x^2=y\))

I = \(\int 1 d x+2 \int \frac{1}{x^2+3} d x-6 \int \frac{1}{x^2+4} d x\)

= \(x+2 \cdot \frac{1}{\sqrt{3}} \tan ^{-1} \frac{x}{\sqrt{3}}-6 \cdot \frac{1}{2} \tan ^{-1} \frac{x}{2}+C=x+\frac{2}{\sqrt{3}} \tan ^{-1} \frac{x}{\sqrt{3}}-3 \tan ^{-1} \frac{x}{2}+C\)

Question 19. \(\int \frac{2 \mathrm{x}}{\left(\mathrm{x}^2+1\right)\left(\mathrm{x}^2+3\right)} \mathrm{dx}\)
Solution:

Let \(I=\int \frac{2 x}{\left(x^2+1\right)\left(x^2+3\right)} d x\)

Put \(\mathrm{x}^2=\mathrm{t} \Rightarrow 2 \mathrm{xdx}=\mathrm{dt}\)

∴ I = \(\int \frac{d t}{(t+1)(t+3)}=\int \frac{1}{(t+1)(t+3)} d x\)

Let \(\frac{A}{t+1}+\frac{B}{t+3}\)

I = \((t+3) A+(t+1) B, 1=(A+B) t+(3 t+B)\)

⇒ A = \(\frac{1}{2} \text { and } B=-\frac{1}{2}\)

∴ I = \(\int \frac{1}{2}\left[\frac{1}{(t+1)}-\frac{1}{(t+3)}\right] d t=\frac{1}{2} \int \frac{1}{t+1} d t-\frac{1}{2} \int \frac{1}{t+3} d t=\frac{1}{2} \log |t+1|-\frac{1}{2} \log |t+3|+C\)

= \(\frac{1}{2} \log \left|\frac{t+1}{t+3}\right|+C=\frac{1}{2} \log \left|\frac{x^2+1}{x^2+3}\right|+C\)

Question 20. \(\int \frac{1}{\mathrm{x}\left(\mathrm{x}^4-1\right)} \mathrm{dx}\)
Solution:

Let \(I=\int \frac{1}{x\left(x^4-1\right)} d x=\int \frac{x^3}{x^4\left(x^4-1\right)} d x \) (Multiply Nr. and Dr. by \(x^3\))

Put \(\mathrm{x}^4=\mathrm{t} \Rightarrow 4 \mathrm{x}^3 \mathrm{dx}=\mathrm{dt}\)

∴\(\int \frac{1}{x\left(x^4-1\right)} d x =\frac{1}{4} \int \frac{d t}{t(t-1)}=\frac{1}{4} \int\left[\frac{1}{t-1}-\frac{1}{t}\right] d t=\frac{1}{4} \int \frac{1}{t-1} d t-\frac{1}{4} \int \frac{1}{t} d t\)

= \(\frac{1}{4} \log |t-1|-\frac{1}{4} \log |t|+C=\frac{1}{4} \log \left|\frac{t-1}{t}\right|+C=\frac{1}{4} \log \left|\frac{x^4-1}{x^4}\right|+C\)

Question 21. \(\int \frac{1}{\left(e^{\mathrm{x}}-1\right)} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \frac{1}{\left(\mathrm{e}^{\mathrm{x}}-1\right)} \mathrm{dx}\)

Let \(\mathrm{e}^{\mathrm{x}}=\mathrm{t} \Rightarrow \mathrm{e}^{\mathrm{x}} \mathrm{dx}=\mathrm{dt} \Rightarrow \mathrm{dx}=\frac{\mathrm{dt}}{\mathrm{t}}\)

∴ I = \(\int \frac{1}{t-1} \times \frac{d t}{t}=\int \frac{1}{t(t-1)} d t=\int\left[\frac{1}{t-1}-\frac{1}{t}\right] d t=\log |t-1|-\log |t|+C\)

= \(\log \left|\frac{t-1}{t}\right|+C=\log \left|\frac{e^x-1}{e^x}\right|+C\)

Choose The Correct Answer

Question 22. \(\int \frac{x d x}{(x-1)(x-2)}\) equals ?

1. \(\log \left|\frac{(x-1)^2}{x-2}\right|+C\)
2. \(\log \left|\frac{(x-2)^2}{x-1}\right|+C\)
3. \(\log \left|\left(\frac{(x-1)}{x-2}\right)^2\right|+C\)
4. \(\log |(x-1)(x-2)|+C\)

Solution: 2. \(\log \left|\frac{(x-2)^2}{x-1}\right|+C\)

Let \(I=\int \frac{x d x}{(x-1)(x-2)}\)

x = \(\mathrm{A}(\mathrm{x}-2)+\mathrm{B}(\mathrm{x}-1)\)

Put x=1 and 2 in (1), we get A=-1 and B=2 respectively,

∴ \(\frac{x}{(x-1)(x-2)}=-\frac{1}{(x-1)}+\frac{2}{(x-2)}\)

I = \(\int\left\{\frac{-1}{(x-1)}+\frac{2}{(x-2)}\right\} d x=-\log |x-1|+2 \log |x-2|+C=\log \left|\frac{(x-2)^2}{(x-1)}\right|+C\)

Hence, the correct answer is (2).

Question 23. \(\int \frac{\mathrm{dx}}{\mathrm{x}\left(\mathrm{x}^2+1\right)}\) equals?

1. \(\log |\mathrm{x}|-\frac{1}{2} \log \left(\mathrm{x}^2+1\right)+\mathrm{C}\)
2. \(\log |\mathrm{x}|+\frac{1}{2} \log \left(\mathrm{x}^2+1\right)+\mathrm{C}\)
3. –\(\log |x|+\frac{1}{2} \log \left(x^2+1\right)+C\)
4. \(\frac{1}{2} \log |\mathrm{x}|+\log \left(\mathrm{x}^2+1\right)+\mathrm{C}\)

Solution: 1. \(\log |\mathrm{x}|-\frac{1}{2} \log \left(\mathrm{x}^2+1\right)+\mathrm{C}\)

Let \(\mathrm{I}=\int \frac{\mathrm{dx}}{\mathrm{x}\left(\mathrm{x}^2+1\right)} \mathrm{dx}\)

Let \(\frac{1}{x\left(x^2+1\right)}=\frac{A}{x}+\frac{B x+C}{x^2+1}\)

I = \(\mathrm{A}\left(\mathrm{x}^2+1\right)+(\mathrm{Bx}+\mathrm{C}) \mathrm{x}\)

In equation (1), Put x=0 ⇒ A=1

On equating the coefficients of \(x^2, x\), we get A+B=0 ⇒ B=-A=-1, A=1 and C=0

∴ \(\frac{1}{x\left(x^2+1\right)}=\frac{1}{x}+\left(\frac{-x}{x^2+1}\right)\)

I = \(\int\left\{\frac{1}{x}-\frac{x}{x^2+1}\right\} d x=\log |x|-\frac{1}{2} \log \left|x^2+1\right|+C\)

Hence, the correct answer is (1).

Integrals Exercise 7.6

Integrate The Function

Question 1. \(\int x \sin x d x\)
Solution:

Let \(I=\int x \sin x d x\)

Taking x as the first function and sin x as the second function and integrating by parts, we obtain

I = \(x \int \sin x d x-\int\left\{\left(\frac{d}{d x}(x)\right) \int \sin x d x\right\} d x\)

= \(x(-\cos x)+\int 1 \cdot(\cos x) d x=-x \cos x+\sin x+C\)

Question 2. Let \(\int x \sin 3 x d x\)
Solution:

I = \(\int x \sin 3 x d x\)

Taking x as the first function and sin 3x as the second function and integrating by parts, we obtain

I = \(x \int \sin 3 x d x-\int\left\{\left(\frac{d}{d x}(x)\right) \int \sin 3 x d x\right\} d x=x\left(\frac{-\cos 3 x}{3}\right)-\int 1 \cdot\left(\frac{-\cos 3 x}{3}\right) d x\)

= \(\frac{-x \cos 3 x}{3}+\frac{1}{3} \int \cos 3 x d x=\frac{-x \cos 3 x}{3}+\frac{1}{9} \sin 3 x+C\)

Question 3. \(\int x^2 e^x d x\)
Solution:

Let \(\mathrm{I}=\int \mathrm{x}^2 \mathrm{e}^{\mathrm{x}} \mathrm{dx}\)

Taking \(x^2\) as first function and \(\mathrm{e}^{\mathrm{x}}\) as second function and integrating by parts, we obtain

I = \(x^2 \int e^x d x-\int\left\{\left(\frac{d}{d x}\left(x^2\right)\right) \int e^x d x\right\} d x=x^2 e^x-\int 2 x \cdot e^x d x=x^2 e^x-2 \int x \cdot e^x d x\)

Again integrating by parts, we obtain

I = \(x^2 e^x-2\left[x \int e^x d x-\int\left\{\left(\frac{d}{d x}(x)\right) \cdot \int e^x d x\right] d x\right]=x^2 e^x-2\left[x e^x-\int e^x d x\right]\)

= \(x^2 e^x-2\left[x e^x-e^x\right]=x^2 e^x-2 x e^x+2 e^x+C=e^x\left(x^2-2 x+2\right)+C\)

Question 4. \(\int x \log x d x\)
Solution:

Let \(\mathrm{I}=\int \mathrm{x} \log \mathrm{x} \mathrm{x}\)

Taking \(\log \mathrm{x}\) as first function and x as second function and integrating by parts, we obtain

I = \(\log x \int x d x-\int\left\{\left(\frac{d}{d x}(\log x)\right) \int x d x\right\} d x=\log x \cdot \frac{x^2}{2}-\int \frac{1}{x} \cdot \frac{x^2}{2} d x\)

= \(\frac{x^2 \log x}{2}-\int \frac{x}{2} d x=\frac{x^2 \log x}{2}-\frac{x^2}{4}+C\)

Question 5. \(\int x \log 2 x d x\)
Solution:

Let \(\mathrm{I}=\int \mathrm{x} \log 2 \mathrm{x} d \mathrm{x}\)

Taking log 2x as the first function and x as the second function and integrating by parts, we obtain

I = \(\log 2 x \int x d x-\int\left\{\left(\frac{d}{d x}(\log 2 x)\right) \int x d x\right\} d x=\log 2 x \cdot \frac{x^2}{2}-\int\left(\frac{2}{2 x} \cdot \frac{x^2}{2}\right) d x\)

= \(\frac{x^2 \log 2 x}{2}-\int \frac{x}{2} d x=\frac{x^2 \log 2 x}{2}-\frac{x^2}{4}+C\)

Question 6. \(\int x^2 \log x d x\)
Solution:

Let \(\mathrm{I}=\int \mathrm{x}^2 \log \mathrm{x} d \mathrm{x}\)

Taking log x as the first function and \(x^2\) as second function and integrating by parts, we obtain

I = \(\log x \int x^2 d x-\int\left\{\left(\frac{d}{d x}(\log x)\right) \int x^2 d x\right\} d x=\log x\left(\frac{x^3}{3}\right)-\int \frac{1}{x} \cdot \frac{x^3}{3} d x\)

= \(\frac{x^3 \log x}{3}-\int \frac{x^2}{3} d x=\frac{x^3 \log x}{3}-\frac{x^3}{9}+C\)

Question 7. \(\int x \sin ^{-1} x d x\)
Solution:

Let \(I=\int x \sin ^{-1} x d x\)

put \(\mathrm{x}=\sin \mathrm{t}, \mathrm{dx}=\cos \mathrm{dt}\)

= \(\int \sin t \sin ^{-1}(\sin t) \cos t d t=\int t \sin t \cos t d t\)

= \(\frac{1}{2} \int t(2 \sin t \cos t) d t=\frac{1}{2} \int t \sin 2 t d t\)

Taking t as the first function and sin 2t as the second function and integrating by parts, we obtain

= \(\frac{1}{2}\left[t \int \sin 2 t d t-\int\left(\frac{d(t)}{d t} \int \sin 2 t d t\right) d t\right]\)

= \(\frac{1}{2}\left[-t \frac{\cos 2 t}{2}+\int \frac{\cos 2 t}{2} d t\right]\)

= \(\frac{1}{2}\left[-\frac{t}{2} \cos 2 t+\frac{1}{4} \sin 2 t\right]+C=\frac{-t}{4} \cos 2 t+\frac{1}{8} \sin 2 t+C\)

= \(\frac{-t}{4}\left[1-2 \sin ^2 t\right]+\frac{1}{8} 2 \sin t \cos t+C=\frac{-t}{4}\left(1-2 \sin ^2 t\right)+\frac{1}{4} \sin t \sqrt{1-\sin ^2 t}+C\)

= \(\frac{-\sin ^{-1} x}{4}\left(1-2 x^2\right)+\frac{x}{4} \sqrt{1-x^2}+C=\frac{1}{4}\left(2 x^2-1\right) \sin ^{-1} x+\frac{x}{4} \sqrt{1-x^2}+C\)

Question 8. \(\int x \tan ^{-1} x d x\)
Solution:

I = \(\int x \tan ^{-1} x d x\)

Taking \(\tan ^{-1} \mathrm{x}\) as first function and x as second function and integrating by parts, we obtain

I = \(\tan ^{-1} x \int x d x-\int\left\{\left(\frac{d}{d x}\left(\tan ^{-1} x\right)\right) \int x d x\right\} d x=\tan ^{-1} x\left(\frac{x^2}{2}\right)-\int \frac{1}{1+x^2} \cdot \frac{x^2}{2} d x\)

= \(\frac{x^2 \tan ^{-1} x}{2}-\frac{1}{2} \int \frac{x^2}{1+x^2} d x=\frac{x^2 \tan ^{-1} x}{2}-\frac{1}{2} \int\left(\frac{x^2+1}{1+x^2}-\frac{1}{1+x^2}\right) d x\)

= \(\frac{x^2 \tan ^{-1} x}{2}-\frac{1}{2} \int\left(1-\frac{1}{1+x^2}\right) d x=\frac{x^2 \tan ^{-1} x}{2}-\frac{1}{2}\left(x-\tan ^{-1} x\right)+C\)

= \(\frac{x^2}{2} \tan ^{-1} x-\frac{x}{2}+\frac{1}{2} \tan ^{-1} x+C\)

Question 9. \(\int x \cos ^{-1} x d x\)
Solution:

Let \(I=\int x \cos ^{-1} x d x\)

put \(\mathrm{x}=\cos \mathrm{t}, \mathrm{dx}=-\sin \mathrm{dt}\)

I = \(-\int \cos t \cos ^{-1}(\cos t) \sin t d t=-\int t \sin t \cos t d t\)

= \(-\frac{1}{2} \int t(2 \sin t \cos t) d t=-\frac{1}{2} \int t(\sin 2 t) d t\)

Taking t as the first function and sin2 t as the second function and integrating by parts, we obtain

= \(-\frac{1}{2}\left[t \int \sin 2 t d t-\int\left(\frac{d(t)}{d t} \int \sin 2 t d t\right) d t=-\frac{1}{2}\left[-t \frac{\cos 2 t}{2}+\int \frac{\cos 2 t}{2} d t\right]\right. \)

= \(-\frac{1}{2}\left[-t \frac{\cos 2 t}{2}+\frac{\sin 2 t}{4}\right]+C=\frac{t}{4} \cos 2 t-\frac{\sin 2 t}{8}+C\)

= \(\frac{t}{4}\left(2 \cos ^2 t-1\right)-\frac{1}{8} 2 \sin t \cos t+C=\frac{t}{4}\left(2 \cos ^2 t-1\right)-\frac{1}{4} \cos t \sqrt{1-\cos ^2} t+C\)

= \(\frac{\cos ^{-1} x}{4}\left(2 x^2-1\right)-\frac{1}{4} x \sqrt{1-x^2}+C=\frac{\left(2 x^2-1\right)}{4} \cos ^{-1} x-\frac{x}{4} \sqrt{1-x^2}+C\)

Question 10. \(\int\left(\sin ^{-1} x\right)^2 \mathrm{dx}\)
Solution:

Let \(I=\int\left(\sin ^{-1} x\right)^2 \cdot 1 d x\)

Taking \(\left(\sin ^{-1} x\right)^2\) as the first function and 1 as the second function and integrating by parts, we obtain

I = \(\left(\sin ^{-1} x\right)^2 \int 1 d x-\int\left\{\frac{d}{d x}\left(\sin ^{-1} x\right)^2 \cdot \int 1 \cdot d x\right\} d x=x\left(\sin ^{-1} x\right)^2-2 \int \frac{x \sin ^{-1} x}{\sqrt{1-x^2}} d x\)

= \(x\left(\sin ^{-1} x\right)^2-2 \int t \cdot \sin t d t\)

∴ \(\left(\begin{array}{l}
\text { Put } \sin ^{-1} x=t \Rightarrow x=\sin t \\
\frac{1}{\sqrt{1-x^2}} d x=d t
\end{array}\right)\)

Again integrating by parts, we obtain

I = \(x\left(\sin ^{-1} x\right)^2-2\left[-t \cos t-\int(-\cos t) d t\right]=x\left(\sin ^{-1} x\right)^2+2 t \cos t-2 \sin t+C\)

I = \(x\left(\sin ^{-1} x\right)^2+2 t \sqrt{1-\sin ^2 t}-2 \sin t+C=x\left(\sin ^{-1} x\right)^2+2 \sin ^{-1} x \sqrt{1-x^2}-2 x+C\)

Question 11. \(\int \frac{x \cos ^{-1} x}{\sqrt{1-x^2}} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{x} \cos ^{-1} \mathrm{x}}{\sqrt{1-\mathrm{x}^2}} \mathrm{dx}\)

Put \(\cos ^{-1} \mathrm{x}=\mathrm{t} \Rightarrow \mathrm{x}=\cos \mathrm{t}\)

⇒ \(\frac{1}{\sqrt{1-x^2}} d x=-d t\)

⇒ I = \(\int t \cos t d t\) (Using interagration by parts)

= \(-\left[t \sin t-\int \sin t d t\right]=-[t \sin t+\cos t]+C\)

⇒I = \(-\left[t \sqrt{1-\cos ^2 t}+\cos t\right]+C=-\left[\sqrt{1-x^2} \cos ^{-1} x+x\right]+C\)

Question 12. \(\int x \sec ^2 x d x\)
Solution:

Let \(\mathrm{I}=\int \mathrm{x} \sec ^2 \mathrm{xdx}\)

Taking x as first function and \(\sec ^2 \mathrm{x}\) as second function and integrating by parts, we obtain

I = \(x \int \sec ^2 x d x-\int\left\{\left\{\frac{d}{d x}(x)\right\} \int \sec ^2 x d x\right\} d x\)

= \(x \tan x-\int 1 \cdot \tan x d x=x \tan x+\log |\cos x|+C\)

Question 13. \(\int \tan ^{-1} x d x\)
Solution:

Let \(I=\int 1 \cdot \tan ^{-1} x d x\)

Taking \(\tan ^{-1} x\) as the first function and 1 as the second function and integrating by parts, we obtain

I = \(\tan ^{-1} x \int 1 d x-\int\left\{\left(\frac{d}{d x}\left(\tan ^{-1} x\right)\right) \int 1 \cdot d x\right\} d x\)

= \(\tan ^{-1} x \cdot x-\int \frac{1}{1+x^2} \cdot x d x=x \tan ^{-1} x-\frac{1}{2} \int \frac{2 x}{1+x^2} d x\)

Let \(1+\mathrm{x}^2=\mathrm{t} \Rightarrow 2 \mathrm{xdx}=\mathrm{dt}\)

⇒ I = \(x \tan ^{-1} x-\frac{1}{2} \int \frac{1}{t} d t=x \tan ^{-1} x-\frac{1}{2} \log |t|+C=x \tan ^{-1} x-\frac{1}{2} \log \left|1+x^2\right|+C\)

Question 14. \(\int x(\log x)^2 d x\)
Solution:

Let \(\mathrm{I}=\int \mathrm{x}(\log \mathrm{x})^2 \mathrm{dx}\)

Taking \((\log x)^2\) as first function and x as second function and integrating by parts, we obtain

I = \((\log x)^2 \int x d x-\int\left\{\left(\frac{d}{d x}(\log x)^2\right) \int x d x\right\} d x\)

= \(\frac{x^2}{2}(\log x)^2-\int\left[2 \log x \cdot \frac{1}{x} \cdot \frac{x^2}{2}\right] d x=\frac{x^2}{2}(\log x)^2-\int x \log x d x\)

Again integrating by parts, we obtain

I = \(\frac{x^2}{2}(\log x)^2-\left[\log x \int x d x-\int\left\{\left(\frac{d}{d x}(\log x)\right) \int x d x\right\} d x\right]\)

= \(\frac{x^2}{2}(\log x)^2-\left[\frac{x^2}{2} \log x-\int \frac{1}{x} \cdot \frac{x^2}{2} d x\right]=\frac{x^2}{2}(\log x)^2-\frac{x^2}{2} \log x+\frac{1}{2} \int x d x\)

= \(\frac{x^2}{2}(\log x)^2-\frac{x^2}{2} \log x+\frac{x^2}{4}+C\)

Question 15. \(\int\left(x^2+1\right) \log x d x\)
Solution:

Let \(I=\int\left(x^2+1\right) \log x d x=\int x^2 \log x d x+\int \log x d x\)

Let \(\mathrm{I}=\mathrm{I}_1+\mathrm{I}_2\)…..(1)

Where, \(I_1=\int x^2 \log x d x\) and \(I_2=\int \log x d x\)

⇒ \(I_1=\int x^2 \log x d x\)

Taking logx as first function and \(\mathrm{x}^2\) as second function and integrating by parts, we obtain

⇒ \(I_1=\log x \cdot \int x^2 d x-\int\left\{\left(\frac{d}{d x}(\log x)\right) \int x^2 d x\right\} d x=\log x \cdot \frac{x^3}{3}-\int \frac{1}{x} \cdot \frac{x^3}{3} d x\)

= \(\frac{x^3}{3} \log x-\frac{1}{3}\left(\int x^2 d x\right)=\frac{x^3}{3} \log x-\frac{x^3}{9}+C_1\)

∴ \(I_2=\int \log x d x\)

Taking log x as the first function and 1 as the second function and integrating by parts, we obtain

⇒ \(I_2 =\log x \int 1 \cdot d x-\int\left\{\left(\frac{d}{d x}(\log x)\right) \int 1 \cdot d x\right\} d x\)

= \(\log x \cdot x-\int \frac{1}{x} \cdot x d x=x \log x-\int 1 d x\)

= \(x \log x-x+C_2\)

Using equations (2) and (3) in (1), we obtain

I = \(\frac{x^3}{3} \log x-\frac{x^3}{9}+C_1+x \log x-x+C_2=\frac{x^3}{3} \log x-\frac{x^3}{9}+x \log x-x+\left(C_1+C_2\right)\)

= \(\left(\frac{x^3}{3}+x\right) \log x-\frac{x^3}{9}-x+C\) (because \(C_1+C_2=C\))

Question 16. \(\int e^x(\sin x+\cos x) d x\)
Solution:

Let \(I=\int e^x(\sin x+\cos x) d x\)

Let \(\mathrm{f}(\mathrm{x})=\sin \mathrm{x}\)

⇒ \(\mathrm{f}(\mathrm{x})=\cos \mathrm{x}\)

⇒ \(\mathrm{I}=\int \mathrm{e}^{\mathrm{x}}\left\{\mathrm{f}(\mathrm{x})+\mathrm{f}^{\prime}(\mathrm{x})\right\} \mathrm{dx}\)

It is known that, \(\int \mathrm{e}^{\mathrm{x}}\left\{\mathrm{f}(\mathrm{x})+\mathrm{f}^{\prime}(\mathrm{x})\right\} \mathrm{dx}=\mathrm{e}^{\mathrm{x}} \mathrm{f}(\mathrm{x})+\mathrm{C}\)

∴ \(\mathrm{I}=\mathrm{e}^{\mathrm{x}} \sin \mathrm{x}+\mathrm{C}\)

Question 17. \(\int \frac{\mathrm{xe}^{\mathrm{x}}}{(1+\mathrm{x})^2} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{xe}}{(1+\mathrm{x})^2} \mathrm{dx}=\int \mathrm{e}^x\left\{\frac{\mathrm{x}}{(1+\mathrm{x})^2}\right\} \mathrm{dx}=\int \mathrm{e}^{\mathrm{x}}\left\{\frac{1+\mathrm{x}-1}{(1+\mathrm{x})^2}\right\} \mathrm{dx}=\int \mathrm{e}^{\mathrm{x}}\left\{\frac{1}{1+\mathrm{x}}-\frac{1}{(1+\mathrm{x})^2}\right\} \mathrm{dx}\)

Let \(f(x)=\frac{1}{1+x} \Rightarrow f^{\prime}(x)=\frac{-1}{(1+x)^2} \Rightarrow \int \frac{x e^x}{(1+x)^2} d x=\int e^x\left\{f(x)+f^{\prime}(x)\right\} d x\)

It is known that, \(\int \mathrm{e}^{\mathrm{x}}\left\{\mathrm{f}(\mathrm{x})+\mathrm{f}^{\prime}(\mathrm{x})\right\} \mathrm{dx}=\mathrm{e}^{\mathrm{x}} \mathrm{f}(\mathrm{x})+\mathrm{C}\)

∴ \(\int \frac{x e^x}{(1+x)^2} d x=\frac{e^x}{1+x}+C\)

Question 18. \(\int e^x\left(\frac{1+\sin x}{1+\cos x}\right) d x\)
Solution:

⇒ \(e^x\left(\frac{1+\sin x}{1+\cos x}\right)=e^x\left(\frac{\sin ^2 \frac{x}{2}+\cos ^2 \frac{x}{2}+2 \sin \frac{x}{2} \cos \frac{x}{2}}{2 \cos ^2 \frac{x}{2}}\right)\)

= \(\frac{e^x\left(\sin \frac{x}{2}+\cos \frac{x}{2}\right)^2}{2 \cos ^2 \frac{x}{2}}\)

= \(\frac{1}{2} e^x \cdot\left(\frac{\sin \frac{x}{2}+\cos \frac{x}{2}}{\cos \frac{x}{2}}\right)^2=\frac{1}{2} e^x\left[\tan \frac{x}{2}+1\right]^2=\frac{1}{2} e^x\left[1+\tan \frac{x}{2}\right]^2\)

= \(\frac{1}{2} e^x\left[1+\tan ^2 \frac{x}{2}+2 \tan \frac{x}{2}\right]=\frac{1}{2} e^x\left[\sec ^2 \frac{x}{2}+2 \tan \frac{x}{2}\right]\)

⇒ \(\int \frac{e^x(1+\sin x) d x}{(1+\cos x)}=\int e^x\left[\tan \frac{x}{2}+\frac{1}{2} \sec ^2 d x\right] d x \ldots .(1)\)

Let \(f(x)=\tan \frac{x}{2} \Rightarrow f^{\prime}(x)=\frac{1}{2} \sec ^2 \frac{x}{2}\)

It is known that, \(\int \mathrm{e}^{\mathrm{x}}\left\{\mathrm{f}(\mathrm{x})+\mathrm{f}^{\prime}(\mathrm{x})\right\} \mathrm{dx}=\mathrm{e}^{\mathrm{x}} \mathrm{f}(\mathrm{x})+\mathrm{C}\)

From equation (1), we obtain, \(\int \frac{e^x(1+\sin x)}{(1+\cos x)} d x=e^x \tan \frac{x}{2}+C\)

Question 19. \(\int e^x\left(\frac{1}{x}-\frac{1}{x^2}\right) d x\)
Solution:

Let \(I=\int e^x\left[\frac{1}{x}-\frac{1}{x^2}\right] d x\)

Also, let \(f(x)=\frac{1}{x} \Rightarrow f^{\prime}(x)=\frac{-1}{x^2}\)

It is known that, \(\int \mathrm{e}^{\mathrm{x}}\left\{\mathrm{f}(\mathrm{x})+\mathrm{f}^{\prime}(\mathrm{x})\right\} \mathrm{dx}=\mathrm{e}^{\mathrm{x}} \mathrm{f}(\mathrm{x})+\mathrm{C}\)

∴ \(\mathrm{I}=\frac{\mathrm{e}^{\mathrm{x}}}{\mathrm{x}}+\mathrm{C}\)

Question 21. \(\int e^{2 x} \sin x d x\)
Solution:

Let \(\mathrm{I}=\int \mathrm{e}^{2 \mathrm{x}} \sin \mathrm{x} d \mathrm{x}\)

Integrating by parts, we obtain. \(I=\sin x \int e^{2 x} d x-\int\left\{\left(\frac{d}{d x}(\sin x)\right) \int e^{2 x} d x\right\} d x\)

⇒ \(\mathrm{I}=\sin \mathrm{x} \cdot \frac{\mathrm{e}^{2 \mathrm{x}}}{2}-\int \cos \mathrm{x} \cdot \frac{\mathrm{e}^{2 \mathrm{x}}}{2} \mathrm{dx} \Rightarrow \mathrm{I}\)

= \(\frac{\mathrm{e}^{2 \mathrm{x}} \sin \mathrm{x}}{2}-\frac{1}{2} \int \mathrm{e}^{2}\)

Again integrating by parts, we obtain \(I=\frac{e^{2 x} \sin x}{2}-\frac{1}{2}\left[\cos x \int e^{2 x} d x-\int\left\{\left(\frac{d}{d x}(\cos x)\right) \int e^{2 x} d x\right\} d x\right]\)

⇒ \(\mathrm{I}=\frac{\mathrm{e}^{2 \mathrm{x}} \sin \mathrm{x}}{2}-\frac{1}{2}\left[\cos \mathrm{x} \cdot \frac{\mathrm{e}^{2 \mathrm{x}}}{2}-\int(-\sin \mathrm{x}) \frac{\mathrm{e}^{2 \mathrm{x}}}{2} \mathrm{dx}\right]\)

⇒ \(\mathrm{I}=\frac{\mathrm{e}^{2 \mathrm{x}} \cdot \sin \mathrm{x}}{2}-\frac{1}{2}\left[\frac{\mathrm{e}^{2 \mathrm{x}} \cos \mathrm{x}}{2}+\frac{1}{2} \int \mathrm{e}^{2 \mathrm{x}} \sin \mathrm{x} d \mathrm{x}\right]\)

⇒ \(\mathrm{I}=\frac{\mathrm{e}^{2 \mathrm{x}} \sin \mathrm{x}}{2}-\frac{\mathrm{e}^{2 \mathrm{x}} \cos \mathrm{x}}{4}-\frac{1}{4} \mathrm{I}\)

⇒ \(\mathrm{I}+\frac{1}{4} \mathrm{I}\)

= \(\frac{\mathrm{e}^{2 \mathrm{x}} \cdot \sin \mathrm{x}}{2}-\frac{\mathrm{e}^{2 \mathrm{x}} \cos \mathrm{x}}{4} \Rightarrow \frac{5}{4} \mathrm{I}=\frac{\mathrm{e}^{2 \mathrm{x}} \sin \mathrm{x}}{2}-\frac{\mathrm{e}^{2 \mathrm{x}} \cos \mathrm{x}}{4}\)

⇒ I = \(\frac{4}{5}\left[\frac{e^{2 x} \sin x}{2}-\frac{e^{2 x} \cos x}{4}\right]+C \Rightarrow I=\frac{e^{2 x}}{5}[2 \sin x-\cos x]+C\)

Question 22. \(\int \sin ^{-1}\left(\frac{2 x}{1+x^2}\right) d x\)
Solution:

Let \(\mathrm{x}=\tan \theta \Rightarrow d x=\sec ^2 \theta d \theta\)

∴ \(\sin ^{-1}\left(\frac{2 x}{1+x^2}\right)=\sin ^{-1}\left(\frac{2 \tan \theta}{1+\tan ^2 \theta}\right)=\sin ^{-1}(\sin 2 \theta)=2 \theta\)

⇒ \(\mathrm{I}=\int \sin ^{-1}\left(\frac{2 \mathrm{x}}{1+\mathrm{x}^2}\right) \mathrm{dx}\)

= \(\int 2 \theta \cdot \sec ^2 \theta \mathrm{d} \theta=2 \int \theta \cdot \sec ^2 \theta \mathrm{d} \theta\)

Integrating by parts, we obtain

I = \(2\left[\theta \cdot \int \sec ^2 \theta d \theta-\int\left\{\left(\frac{d}{d \theta}(\theta)\right) \int \sec ^2 \theta d \theta\right\} d \theta\right]\)

= \(2\left[\theta \cdot \tan \theta-\int \tan \theta d \theta\right]\)

= \(2[\theta \tan \theta+\log |\cos \theta|+C]=2\left[x \tan ^{-1} x+\log \left|\frac{1}{\sqrt{1+x^2}}\right|+C\right]\)

= \(2 x \tan ^{-1} x+2 \log \left(1+x^2\right)^{-\frac{1}{2}}+C\)

= \(2 x \tan ^{-1} x+2\left[-\frac{1}{2} \log \left(1+x^2\right)\right]+C=2 x \tan ^{-1} x-\log \left(1+x^2\right)+C\)

Choose The Correct Answer

Question 23. \(\int x^2 e^{x^7} d x\) equals

1. \(\frac{1}{3} \mathrm{e}^{\mathrm{x}^3}+\mathrm{C}\)
2. \(\frac{1}{3} \mathrm{e}^{\mathrm{x}^2}+\mathrm{C}\)
3. \(\frac{1}{2} \mathrm{e}^{\mathrm{x}^1}+\mathrm{C}\)
4. \(\frac{1}{2} e^{x^2}+C\)

Solution: 1. \(\frac{1}{3} \mathrm{e}^{\mathrm{x}^3}+\mathrm{C}\)

Let \(\mathrm{I}=\int \mathrm{x}^2 \mathrm{e}^{\mathrm{x}^3} \mathrm{dx}\)

Put \(x^3=t \Rightarrow 3 x^2 d x=d t \Rightarrow I=\frac{1}{3} \int e^t d t=\frac{1}{3}\left(e^t\right)+C=\frac{1}{3} e^{x^3}+C\)

Hence, the correct answer is (1).

Question 24. \(\int e^x \sec x(1+\tan x) d x\)

1. \(\mathrm{e}^{\mathrm{x}} \cos \mathrm{x}+\mathrm{C}\)
2. \(e^x \sec x+C\)
3. \(e^x \sin x+C\)
4. \(\mathrm{e}^{\mathrm{x}} \tan \mathrm{x}+\mathrm{C}\)

Solution: 2. \(e^x \sec x+C\)

Let \(I=\int e^x \sec x(1+\tan x) d x\)

= \(\int \mathrm{e}^x(\sec x+\sec x \tan x) d x\)

Also, if sec x = f(x) ⇒ \(\sec \mathrm{x} \tan \mathrm{x}=\mathrm{f}^{\prime}(\mathrm{x})\)

It is known that, \(\int \mathrm{e}^x\left\{f(x)+f^{\prime}(x)\right\} d x=e^x f(x)+C\)

I = \(e^x \sec x+C\)

Hence, the correct answer is (2).

Integrals Exercise 7.7

Integrate The Functions

Question 1. \(\int \sqrt{4-x^2} d x\)
Solution:

Let \(\mathrm{I}=\int \sqrt{4-\mathrm{x}^2} \mathrm{dx}=\int \sqrt{(2)^2-(\mathrm{x})^2} \mathrm{dx}\)

It is known that, \(\int \sqrt{a^2-x^2} d x=\frac{x}{2} \sqrt{a^2-x^2}+\frac{a^2}{2} \sin ^{-1}\left(\frac{x}{a}\right)+C\)

∴ I = \(\frac{x}{2} \sqrt{4-x^2}+\frac{4}{2} \sin ^{-1}\left(\frac{x}{2}\right)+C=\frac{x}{2} \sqrt{4-x^2}+2 \sin ^{-1}\left(\frac{x}{2}\right)+C
\)

Question 2. \(\int \sqrt{1-4 \mathrm{x}^2} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \sqrt{1-4 \mathrm{x}^2} \mathrm{dx}=\int \sqrt{(1)^2-(2 \mathrm{x})^2} \mathrm{dx}\), Let \(2 \mathrm{x}=\mathrm{t} \Rightarrow 2 \mathrm{dx}=\mathrm{dt}\)

I = \(\frac{1}{2} \int \sqrt{(1)^2-(t)^2} d t\)

It is known that, (because \(\int \sqrt{a^2-x^2} d x=\frac{x}{2} \sqrt{a^2-x^2}+\frac{a^2}{2} \sin ^{-1}\left(\frac{x}{a}\right)+C\))

⇒ I = \(\frac{1}{2}\left[\frac{\mathrm{t}}{2} \sqrt{1-\mathrm{t}^2}+\frac{1}{2} \sin ^{-1} \mathrm{t}\right]+\mathrm{C}=\frac{\mathrm{t}}{4} \sqrt{1-\mathrm{t}^2}+\frac{1}{4} \sin ^{-1} \mathrm{t}+\mathrm{C}\)

= \(\frac{2 \mathrm{x}}{4} \sqrt{1-4 \mathrm{x}^2}+\frac{1}{4} \sin ^{-1}(2 \mathrm{x})+\mathrm{C}=\frac{\mathrm{x}}{2} \sqrt{1-4 \mathrm{x}^2}+\frac{1}{4} \sin ^{-1}(2 \mathrm{x})+\mathrm{C}\)

Question 3. \(\int \sqrt{x^2+4 x+6} d x\)
Solution:

Let \(I=\int \sqrt{x^2+4 x+6} d x=\int \sqrt{x^2+4 x+4+2} d x\)

= \(\int \sqrt{\left(x^2+4 x+4\right)+2} d x=\int \sqrt{(x+2)^2+(\sqrt{2})^2} d x\)

It is known that, (because \(\int \sqrt{\mathrm{x}^2+\mathrm{a}^2} \mathrm{dx}=\frac{\mathrm{x}}{2} \sqrt{\mathrm{x}^2+\mathrm{a}^2}+\frac{\mathrm{a}^2}{2} \log \left|\mathrm{x}+\sqrt{\mathrm{x}^2+\mathrm{a}^2}\right|+\mathrm{C}\))

∴ I = \(\frac{(x+2)}{2} \sqrt{x^2+4 x+6}+\frac{2}{2} \log \left|(x+2)+\sqrt{x^2+4 x+6}\right|+C\)

= \(\frac{(x+2)}{2} \sqrt{x^2+4 x+6}+\log \left|(x+2)+\sqrt{x^2+4 x+6}\right|+C\)

Question 4. \(\int \sqrt{x^2+4 x+1} d x\)
Solution:

Let \(I=\int \sqrt{x^2+4 x+1} d x=\int \sqrt{\left(x^2+4 x+4\right)-3} d x=\int \sqrt{(x+2)^2-(\sqrt{3})^2} d x\)

It is known that, (because \(\int \sqrt{x^2-a^2} d x=\frac{x}{2} \sqrt{x^2-a^2}-\frac{a^2}{2} \log \left|x+\sqrt{x^2-a^2}\right|+C\))

∴ I = \(\frac{(x+2)}{2} \sqrt{x^2+4 x+1}-\frac{3}{2} \log \left|(x+2)+\sqrt{x^2+4 x+1}\right|+C\)

Question 5. \(\int \sqrt{1-4 x-x^2} d x\)
Solution:

Let \(\mathrm{I}=\int \sqrt{1-4 \mathrm{x}-\mathrm{x}^2} d \mathrm{x}=\int \sqrt{1-\left(\mathrm{x}^2+4 \mathrm{x}+4-4\right)} \mathrm{dx}\)

= \(\int \sqrt{1+4-(\mathrm{x}+2)^2} \mathrm{dx}=\int \sqrt{(\sqrt{5})^2-(\mathrm{x}+2)^2} \mathrm{dx}\)

It is known that, \(\int \sqrt{a^2-x^2} d x=\frac{x}{2} \sqrt{a^2-x^2}+\frac{a^2}{2} \sin ^{-1}\left(\frac{x}{a}\right)+C\)

∴ I = \(\frac{(x+2)}{2} \sqrt{1-4 x-x^2}+\frac{5}{2} \sin ^{-1}\left(\frac{x+2}{\sqrt{5}}\right)+C\)

Question 6. \(\int \sqrt{x^2+4 x-5} d x\)
Solution:

Let \(I=\int \sqrt{x^2+4 x-5} d x=\int \sqrt{\left(x^2+4 x+4-9\right)} d x=\int \sqrt{(x+2)_{-}^2-(3)^2} d x\)

It is known that, \(\int \sqrt{x^2-a^2} d x=\frac{x}{2} \sqrt{x^2-a^2}-\frac{a^2}{2} \log \left|x+\sqrt{x^2-a^2}\right|+C\)

∴ I = \(\frac{(x+2)}{2} \sqrt{x^2+4 x-5}-\frac{9}{2} \log \left|(x+2)+\sqrt{x^2+4 x-5}\right|+C\)

Question 7. \(\int \sqrt{1+3 \mathrm{x}-\mathrm{x}^2} \mathrm{dx}\)
Solution:

Let \(I=\int \sqrt{1+3 x-x^2} d x=\int \sqrt{1-\left(x^2-3 x+\frac{9}{4}-\frac{9}{4}\right)} d x\)

= \(\int \sqrt{\left(1+\frac{9}{4}\right)-\left(x-\frac{3}{2}\right)^2} d x=\int \sqrt{\left(\frac{\sqrt{13}}{2}\right)^2-\left(x-\frac{3}{2}\right)^2} d x\)

It is known that, \(\left\{\int \sqrt{a^2-x^2} d x=\frac{x}{2} \sqrt{a^2-x^2}+\frac{a^2}{2} \sin ^{-1}\left(\frac{x}{a}\right)+C\right\}\)

∴ I = \(\frac{\left(x-\frac{3}{2}\right)}{2} \sqrt{1+3 x-x^2}+\frac{13}{4 \times 2} \sin ^{-1}\left(\frac{x-\frac{3}{2}}{\frac{\sqrt{13}}{2}}\right)+C\)

= \(\frac{2 x-3}{4} \sqrt{1+3 x-x^2}+\frac{13}{8} \sin ^{-1}\left(\frac{2 x-3}{\sqrt{13}}\right)+C\)

Question 8. \(\int \sqrt{\mathrm{x}^2+3 \mathrm{x}} d \mathrm{x}\)
Solution:

Let \(I=\int \sqrt{x^2+3 x} d x=\int \sqrt{x^2+3 x+\frac{9}{4}-\frac{9}{4}} d x=\int \sqrt{\left(x+\frac{3}{2}\right)^2-\left(\frac{3}{2}\right)^2} d x\)

It is known that, (because \(\int \sqrt{\mathrm{x}^2-\mathrm{a}^2} \mathrm{dx}=\frac{\mathrm{x}}{2} \sqrt{\mathrm{x}^2-\mathrm{a}^2}-\frac{\mathrm{a}^2}{2} \log \left|\mathrm{x}+\sqrt{\mathrm{x}^2-\mathrm{a}^2}\right|+\mathrm{C}\}\)

∴I = \(\frac{\left(x+\frac{3}{2}\right)}{2} \sqrt{x^2+3 x}-\frac{9}{2} \log \left|\left(x+\frac{3}{2}\right)+\sqrt{x^2+3 x}\right|+C\)

= \(\frac{(2 x+3)}{4} \sqrt{x^2+3 x}-\frac{9}{8} \log \left|\left(x+\frac{3}{2}\right)+\sqrt{x^2+3 x}\right|+C\)

Question 9. \(\int \sqrt{1+\frac{x^2}{9}} d x\)
Solution:

Let \(\mathrm{I}=\int \sqrt{1+\frac{\mathrm{x}^2}{9}} \mathrm{dx}=\frac{1}{3} \int \sqrt{9+\mathrm{x}^2} d x=\frac{1}{3} \int \sqrt{(3)^2+\mathrm{x}^2} d x\)

It is known that, \(\int \sqrt{x^2+a^2} d x=\frac{x}{2} \sqrt{x^2+a^2}+\frac{a^2}{2} \log \left|x+\sqrt{x^2+a^2}\right|+C\)

∴ I = \(\frac{1}{3}\left[\frac{x}{2} \sqrt{x^2+9}+\frac{9}{2} \log \left|x+\sqrt{x^2+9}\right|\right]+C=\frac{x}{6} \sqrt{x^2+9}+\frac{3}{2} \log \left|x+\sqrt{x^2+9}\right|+C\)

Choose The Correct Answer

Question 10. \(\int \sqrt{1+\mathrm{x}^2} \mathrm{dx}\) is equal to?

1. \(\frac{\mathrm{x}}{2} \sqrt{1+\mathrm{x}^2}+\frac{1}{2} \log \left|\mathrm{x}+\sqrt{1+\mathrm{x}^2}\right|+\mathrm{C}\)
2. \(\frac{2}{3}\left(1+x^2\right)^{\frac{3}{2}}+C\)
3. \(\frac{2}{3} x\left(1+x^2\right)^{\frac{3}{2}}+C\)
4. \(\frac{x^2}{2} \sqrt{1+x^2}+\frac{1}{2} x^2 \log \left|x+\sqrt{1+x^2}\right|+C\)

Solution:

It is known that, \(\int \sqrt{a^2+x^2} d x=\frac{x}{2} \sqrt{a^2+x^2}+\frac{a^2}{2} \log \left|x+\sqrt{x^2+a^2}\right|+C\)

∴ \(\int \sqrt{1+\mathrm{x}^2} \mathrm{dx}=\frac{\mathrm{x}}{2} \sqrt{1+\mathrm{x}^2}+\frac{1}{2} \log \left|\mathrm{x}+\sqrt{1+\mathrm{x}^2}\right|+\mathrm{C}\).

Hence, the correct answer is (1).

Question 11. \(\int \sqrt{x^2-8 x+7} d x\) is equal to ?

1. \(\frac{1}{2}(x-4) \sqrt{x^2-8 x+7}+9 \log \left|x-4+\sqrt{x^2-8 x+7}\right|+C\)
2. \(\frac{1}{2}(x+4) \sqrt{x^2-8 x+7}+9 \log \left|x+4+\sqrt{x^2-8 x+7}\right|+C\)
3. \(\frac{1}{2}(x-4) \sqrt{x^2-8 x+7}-3 \sqrt{2} \log \left|x-4+\sqrt{x^2-8 x+7}\right|+C\)
4. \(\frac{1}{2}(x-4) \sqrt{x^2-8 x+7}-\frac{9}{2} \log \left|x-4+\sqrt{x^2-8 x+7}\right|+C\)

Solution:

Let \(\mathrm{I}=\int \sqrt{\mathrm{x}^2-8 \mathrm{x}+7} \mathrm{dx}=\int \sqrt{\left(\mathrm{x}^2-8 \mathrm{x}+16\right)-9} \mathrm{dx}=\int \sqrt{(\mathrm{x}-4)^2-(3)^2} \mathrm{dx}\)

It is known that, \(\int \sqrt{\mathrm{x}^2-\mathrm{a}^2} \mathrm{dx}=\frac{\mathrm{x}}{2} \sqrt{\mathrm{x}^2-\mathrm{a}^2}-\frac{\mathrm{a}^2}{2} \log \left|\mathrm{x}+\sqrt{\mathrm{x}^2-\mathrm{a}^2}\right|+\mathrm{C}\)

∴ \(I=\frac{(x-4)}{2} \sqrt{x^2-8 x+7}-\frac{9}{2} \log \left|(x-4)+\sqrt{x^2-8 x+7}\right|+C\).

Hence, the correct answer is (4).

Integrals Exercise 7.8

Evaluate The Definite Integrals

Question 1. \(\int_{-1}^1(x+1) d x\)
Solution:

Let \(\int_{-1}^1(x+1) d x\)=\(\left(\frac{\mathrm{x}^2}{2}+\mathrm{x}\right)_{-1}^1=\left(\frac{1}{2}+1\right)-\left(\frac{1}{2}-1\right)=\frac{1}{2}+1-\frac{1}{2}+\mathrm{I}=2\)

Question 2. \(\int_2^3 \frac{1}{\mathrm{x}} \mathrm{dx}\)
Solution:

Let \(I=\int_2^3 \frac{1}{x} d x=[\log |x|]_2^3=\log |3|-\log |2|=\log \frac{3}{2}\)

Question 3. \(\int_1^2\left(4 x^3-5 x^2+6 x+9\right) d x\)
Solution:

Let \(\mathrm{I}=\int_1^2\left(4 \mathrm{x}^3-5 \mathrm{x}^2+6 \mathrm{x}+9\right) \mathrm{dx}\)

⇒ \(\int_1^2\left(4 x^3-5 x^2+6 x+9\right) d x=\left[4\left(\frac{x^4}{4}\right)-5\left(\frac{x^3}{3}\right)+6\left(\frac{x^2}{2}\right)+9(x)\right]_1^2\)

I = \(\left\{2^4-\frac{5 \cdot(2)^3}{3}+3(2)^2+9(2)\right\}-\left\{(1)^4-\frac{5(1)^3}{3}+3(1)^2+9(1)\right\}\)

= \(\left(16-\frac{40}{3}+12+18\right)-\left(1-\frac{5}{3}+3+9\right)=16-\frac{40}{3}+12+18-1+\frac{5}{3}-3-9\)

= \(33-\frac{35}{3}=\frac{99-35}{3}=\frac{64}{3}\)

Question 4. \(\int_0^{\frac{\pi}{4}} \sin 2 x d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{4}} \sin 2 x d x=\left(\frac{-\cos 2 x}{2}\right)_0^{\frac{\pi}{4}}=-\frac{1}{2}\left[\cos 2\left(\frac{\pi}{4}\right)-\cos 0\right]=-\frac{1}{2}\left[\cos \left(\frac{\pi}{2}\right)-\cos 0\right]\)

= \(-\frac{1}{2}[0-1]=\frac{1}{2}\)

Question 5. \(\int_0^{\frac{\pi}{2}} \cos 2 x d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{2}} \cos 2 x d x=\left(\frac{\sin 2 x}{2}\right)_0^{\frac{\pi}{2}}=\frac{1}{2}\left[\sin 2\left(\frac{\pi}{2}\right)-\sin 0\right]=\frac{1}{2}[\sin \pi-\sin 0]=\frac{1}{2}[0-0]=0\)

Question 6. \(\int_4^5 e^x d x\)
Solution:

Let \(\mathrm{I}=\int_4^5 \mathrm{e}^{\mathrm{x}} \mathrm{dx}=\left(\mathrm{e}^{\mathrm{x}}\right)_4^5=\mathrm{e}^5-\mathrm{e}^4=\mathrm{e}^4(\mathrm{e}-1)\)

Question 7. \(\int_0^{\frac{\pi}{4}} \tan x d x\)
Solution:

Let I = \(\int_0^{\frac{\pi}{4}} \tan x d x=[-\log |\cos x|]_0^{\frac{\pi}{4}}=-\log \left|\cos \frac{\pi}{4}\right|+\log |\cos 0|\)

= \(-\log \left|\frac{1}{\sqrt{2}}\right|+\log |1|\) (because log (1)=0)

= \(-\log (2)^{-\frac{1}{2}}=\frac{1}{2} \log 2\)

Question 8. \(\int_{\frac{\pi}{6}}^{\frac{\pi}{4}} \mathrm{cosec} x d x\)
Solution:

Let \(I =\int_{\frac{\pi}{6}}^{\frac{\pi}{4}} \mathrm{cosec} x d x=[\log |\mathrm{cosec} x-\cot x|]_{\frac{\pi}{6}}^{\frac{\pi}{4}}\)

= \(\log \left|\mathrm{cosec} \frac{\pi}{4}-\cot \frac{\pi}{4}\right|-\log \left|\mathrm{cosec} \frac{\pi}{6}-\cot \frac{\pi}{6}\right|\)

= \(\log |\sqrt{2}-1|-\log |2-\sqrt{3}|=\log \left(\frac{\sqrt{2}-1}{2-\sqrt{3}}\right)\)

Question 9. \(\int_0^1 \frac{\mathrm{dx}}{\sqrt{1-\mathrm{x}^2}}\)
Solution:

Let \(\mathrm{I}=\int_0^1 \frac{\mathrm{dx}}{\sqrt{1-\mathrm{x}^2}}=\left[\sin ^{-1} \mathrm{x}\right]_0^{\mathrm{t}}=\sin ^{-1}(1)-\sin ^{-1}(0)=\frac{\pi}{2}-0=\frac{\pi}{2}\)

Question 10. \(\int_0^1 \frac{\mathrm{dx}}{1+\mathrm{x}^2}\)
Solution:

Let \(\mathrm{I}=\int_0^1 \frac{\mathrm{dx}}{1+\mathrm{x}^2}=\left[\tan ^{-1} \mathrm{x}\right]_0^1\)

= \(\tan ^{-1}(1)-\tan ^{-1}(0)=\frac{\pi}{4}\)

Question 11. \(\int_2^3 \frac{\mathrm{dx}}{\mathrm{x}^2-1}\)
Solution:

Let \(I=\int_2^3 \frac{d x}{x^2-1}=\left[\frac{1}{2} \log \left|\frac{x-1}{x+1}\right|\right]_2^3\)

= \(\frac{1}{2}\left[\log \left|\frac{3-1}{3+1}\right|-\log \left|\frac{2-1}{2+1}\right|\right]=\frac{1}{2}\left[\log \left|\frac{2}{4}\right|-\log \left|\frac{1}{3}\right|\right]\)

= \(\frac{1}{2}\left[\log \frac{1}{2}-\log \frac{1}{3}\right]=\frac{1}{2}\left[\log \frac{3}{2}\right]\)

Question 12. \(\int_0^{\frac{\pi}{2}} \cos ^2 x d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{2}} \cos ^2 x d x\)

I = \(\int_0^{\frac{\pi}{2}}\left(\frac{1+\cos 2 x}{2}\right) d x=\frac{1}{2}\left(x+\frac{\sin 2 x}{2}\right)_0^{\frac{\pi}{2}}\)

= \(\frac{1}{2}\left[\left(\frac{\pi}{2}+\frac{\sin \pi}{2}\right)-\left(0+\frac{\sin 0}{2}\right)\right]=\frac{1}{2}\left[\frac{\pi}{2}+0-0-0\right]=\frac{\pi}{4}\)

Question 13. \(\int_2^3 \frac{x d x}{x^2+1}\)
Solution:

Let \(I=\int_2^3 \frac{\mathrm{x}}{\mathrm{x}^2+1} \mathrm{dx}=\frac{1}{2} \int_2^3 \frac{2 \mathrm{x}}{\mathrm{x}^2+1} \mathrm{dx}=\frac{1}{2}\left[\log \left(1+\mathrm{x}^2\right)\right]_2^3\)

(because \(\mathrm{x}^2+1=\mathrm{t}, 2 \mathrm{x} d \mathrm{x}=\mathrm{dt}\))

= \(\frac{1}{2}\left[\log \left(1+(3)^2\right)-\log \left(1+(2)^2\right)\right]=\frac{1}{2}[\log (10)-\log (5)]=\frac{1}{2} \log \left(\frac{10}{5}\right)=\frac{1}{2} \log 2\)

Question 14. \(\int_0^1 \frac{2 x+3}{5 x^2+1} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int_0^1 \frac{2 \mathrm{x}+3}{5 \mathrm{x}^2+1} \mathrm{dx}=\frac{1}{5} \int_0^1 \frac{5(2 \mathrm{x}+3)}{5 \mathrm{x}^2+1} \mathrm{dx}=\frac{1}{5} \int_0^1 \frac{(10 \mathrm{x}+15)}{5 \mathrm{x}^2+1} \mathrm{dx}\)

= \(\frac{1}{5} \int_0^1 \frac{10 \mathrm{x}}{5 \mathrm{x}^2+1} \mathrm{dx}+3 \int_0^1 \frac{1}{5 \mathrm{x}^2+1} \mathrm{dx}\)

= \(\frac{1}{5} \int_0^1 \frac{10 \mathrm{x}}{5 \mathrm{x}^2+1} \mathrm{dx}+3 \int_0^1 \frac{1}{5\left(\mathrm{x}^2+\left(\frac{1}{\sqrt{5}}\right)^2\right)} \mathrm{dx}\)

= \(\frac{1}{5}\left[\log \left(5 \mathrm{x}^2+1\right)\right]_0^1+\frac{3}{5} \cdot \frac{1}{\frac{1}{\sqrt{5}}}\left[\tan ^{-1}\left(\frac{\mathrm{x}}{\frac{1}{\sqrt{5}}}\right)\right]_0^1\)

= \(\frac{1}{5}\left[\log \left(5 \mathrm{x}^2+1\right)\right]_0^1+\frac{3}{\sqrt{5}}\left[\tan ^{-1}(\sqrt{5} \mathrm{x})\right]_1^1\)

= \(\left\{\frac{1}{5} \log (5+1)-\frac{1}{5} \log (1)\right\}+\left\{\frac{3}{\sqrt{5}} \tan ^{-1}(\sqrt{5})-\frac{3}{\sqrt{5}} \tan ^{-1}(0)\right\}\)

= \(\frac{1}{5} \log 6+\frac{3}{\sqrt{5}} \tan ^{-1} \sqrt{5}\) (because log (1)=0 and \(\tan ^{-1}(0)=0\))

Question 15. \(\int_0^1 x e^{x^2} d x\)
Solution:

Let \(\mathrm{I}=\int_0^1 x \mathrm{e}^{x^2} \mathrm{dx}\)

Put \(\mathrm{x}^2=\mathrm{t} \Rightarrow 2 \mathrm{xdx}=\mathrm{dt}\)

As \(x \rightarrow 0, t \rightarrow 0\) and as \(x \rightarrow 1, t \rightarrow 1\)

∴ \(\mathrm{I}=\frac{1}{2} \int_0^1 \mathrm{e}^{\mathrm{t}} \mathrm{dt}=\frac{1}{2}\left(\mathrm{e}^{\mathrm{t}}\right)_0^1=\frac{1}{2} \mathrm{e}-\frac{1}{2} \mathrm{e}^0=\frac{1}{2}(\mathrm{e}-1)\)

Question 16. \(\int_1^2 \frac{5 x^2}{x^2+4 x+3} d x\)
Solution:

Let \(\mathrm{I}=\int_1^2 \frac{5 \mathrm{x}^2}{\mathrm{x}^2+4 \mathrm{x}+3} \mathrm{dx}\)

Dividing \(5 x^2\) by \(x^2+4 x+3\), we obtain

I = \(\int_1^2\left\{5-\frac{20 x+15}{x^2+4 x+3}\right\} d x=\int_1^2 5 d x-\int_1^2 \frac{20 x+15}{x^2+4 x+3} d x=[5 x]_1^2-\int_1^2 \frac{20 x+15}{x^2+4 x+3} d x\)

⇒ \(\mathrm{I}=5-\mathrm{I}_1\), where \(\mathrm{I}_{\mathrm{t}}=\int_1^2 \frac{20 \mathrm{x}+15}{\mathrm{x}^2+4 \mathrm{x}+3} \mathrm{dx}\)….(1)

Consider \(I_1=\int_1^2 \frac{20 x+15}{x^2+4 x+3} d x\)

Let \(20 \mathrm{x}+15=\mathrm{A} \frac{\mathrm{d}}{\mathrm{dx}}\left(\mathrm{x}^2+4 \mathrm{x}+3\right)+\mathrm{B}=2 \mathrm{Ax}+(4 \mathrm{~A}+\mathrm{B})\)

Equating the coefficients of x and the constant term, we obtain

A = 10 and B=-25

⇒ \(I_1=10 \int_1^2 \frac{2 x+4}{x^2+4 x+3} d x-25 \int_1^2 \frac{d x}{x^2+4 x+3}\)

Let \(\mathrm{x}^2+4 \mathrm{x}+3=\mathrm{t}\)

⇒ \((2 \mathrm{x}+4) \mathrm{dx}=\mathrm{dt}\)

⇒ \(\mathrm{I}_1=10 \int_8^{15} \frac{\mathrm{dt}}{\mathrm{t}}-25 \int_1^2 \frac{\mathrm{dx}}{(\mathrm{x}+2)^2-1^2}=10[\log \mathrm{t}]_{\mathrm{s}}^{15}-25\left[\frac{1}{2} \log \left(\frac{\mathrm{x}+2-1}{\mathrm{x}+2+1}\right)\right]_1^2\)

= \([10 \log 15-10 \log 8]-25\left[\frac{1}{2} \log \frac{3}{5}-\frac{1}{2} \log \frac{2}{4}\right]\)

= \([10 \log (5 \times 3)-10 \log (4 \times 2)]-\frac{25}{2}[\log 3-\log 5-\log 2+\log 4] \)

= \([10 \log 5+10 \log 3-10 \log 4-10 \log 2]-\frac{25}{2}[\log 3-\log 5-\log 2+\log 4]\)

= \(\left[10+\frac{25}{2}\right] \log 5+\left[-10-\frac{25}{2}\right] \log 4+\left[10-\frac{25}{2}\right] \log 3+\left[-10+\frac{25}{2}\right] \log 2\)

= \(\frac{45}{2} \log 5-\frac{45}{2} \log 4-\frac{5}{2} \log 3+\frac{5}{2} \log 2=\frac{45}{2} \log \frac{5}{4}-\frac{5}{2} \log \frac{3}{2}\)

Substituting the value of \(I_1\) in (1), we obtain

I = \(5-\left[\frac{45}{2} \log \frac{5}{4}-\frac{5}{2} \log \frac{3}{2}\right]=5-\frac{5}{2}\left[9 \log \frac{5}{4}-\log \frac{3}{2}\right]\)

Question 17. \(\int_0^{\frac{\pi}{4}}\left(2 \sec ^2 x+x^3+2\right) d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{4}}\left(2 \sec ^2 x+x^3+2\right) d x=\left(2 \tan x+\frac{x^4}{4}+2 x\right)_0^{\frac{\pi}{4}}\)

= \(\left\{\left(2 \tan \frac{\pi}{4}+\frac{1}{4}\left(\frac{\pi}{4}\right)^4+2\left(\frac{\pi}{4}\right)\right)-(2 \tan 0+0+0)\right\}=2 \tan \frac{\pi}{4}+\frac{\pi^4}{4^5}+\frac{\pi}{2}=2+\frac{\pi}{2}+\frac{\pi^4}{1024}\)

Question 18. \(\int_0^\pi\left(\sin ^2 \frac{x}{2}-\cos ^2 \frac{x}{2}\right) d x\)
Solution:

Let \(I=\int_0^\pi\left(\sin ^2 \frac{x}{2}-\cos ^2 \frac{x}{2}\right) d x=-\int_0^\pi\left(\cos ^2 \frac{x}{2}-\sin ^2 \frac{x}{2}\right) d x\)

= \(-\int_0^\pi \cos x d x=(-\sin x)_0^\pi=-[\sin \pi-\sin 0]=0\)

Question 19. \(\int_0^2 \frac{6 x+3}{x^2+4} d x\)
Solution:

Let \(I=\int_0^2 \frac{6 x+3}{x^2+4} d x=3 \int_0^2 \frac{2 x+1}{x^2+4} d x=3 \int_0^2 \frac{2 x}{x^2+4} d x+3 \int_0^2 \frac{1}{x^2+2^2} d x\)

(because \(\int \frac{d x}{a^2+x^2}=\frac{1}{a} \tan ^{-1} \frac{x}{a}\))

= \(3\left[\log \left(x^2+4\right)\right]_0^2+\frac{3}{2}\left(\tan ^{-1} \frac{x}{2}\right)_0^2\)

= \(\left\{3 \log \left(2^2+4\right)+\frac{3}{2} \tan ^{-1}\left(\frac{2}{2}\right)\right\}-\left\{3 \log (0+4)+\frac{3}{2} \tan ^{-1}\left(\frac{0}{2}\right)\right\}\)

= \(3 \log 8+\frac{3}{2} \tan ^{-1} 1-3 \log 4-\frac{3}{2} \tan ^{-1} 0=3 \log 8+\frac{3}{2}\left(\frac{\pi}{4}\right)-3 \log 4-0\)

= \(3 \log \left(\frac{8}{4}\right)+\frac{3 \pi}{8}=3 \log 2+\frac{3 \pi}{8}\)

Question 20. \(\int_0^1\left(x e^x+\sin \frac{\pi x}{4}\right) d x\)
Solution:

Let \(\mathrm{I}=\int_0^1\left(x e^x+\sin \frac{\pi x}{4}\right) \mathrm{dx}=\left(x \mathrm{e}^{\mathrm{x}}\right)_0^1-\int_0^1 \mathrm{e}^{\mathrm{x}} \mathrm{dx}-\left[\frac{4}{\pi} \cos \frac{\pi \mathrm{x}}{4}\right]_0^1\)

= \(\left[x e^x-e^x-\frac{4}{\pi} \cos \frac{\pi x}{4}\right]_0^1=\left(1 \cdot e^1-e^1-\frac{4}{\pi} \cos \frac{\pi}{4}\right)-\left(0 \cdot e^0-e^0-\frac{4}{\pi} \cos 0\right)\)

= \(e-e-\frac{4}{\pi}\left(\frac{1}{\sqrt{2}}\right)+1+\frac{4}{\pi}=1+\frac{4}{\pi}-\frac{2 \sqrt{2}}{\pi}\)

Choose The Correct Answer

Question 21. \(\int_1^{\sqrt{3}} \frac{\mathrm{dx}}{1+\mathrm{x}^2}\) equals

1. \(\frac{\pi}{3}\)
2. \(\frac{2 \pi}{3}\)
3. \(\frac{\pi}{6}\)
4. \(\frac{\pi}{12}\)

Solution: 4. \(\frac{\pi}{12}\)

I = \(\int_1^{\sqrt{3}} \frac{\mathrm{dx}}{1+\mathrm{x}^2}=\left(\tan ^{-1} \mathrm{x}\right)_1^{\sqrt{3}}=\tan ^{-1} \sqrt{3}-\tan ^{-1} 1=\frac{\pi}{3}-\frac{\pi}{4}=\frac{\pi}{12}\).

Hence, the correct answer is (4).

Question 22. \(\int_0^{\frac{2}{3}} \frac{\mathrm{dx}}{4+9 \mathrm{x}^2}\) equals?

1. \(\frac{\pi}{6}\)
2. \(\frac{\pi}{12}\)
3. \(\frac{\pi}{24}\)
4. \(\frac{\pi}{4}\)

Solution: 3. \(\frac{\pi}{24}\)

I = \(\int_0^{\frac{2}{3}} \frac{d x}{4+9 x^2}=\int_0^{\frac{2}{3}} \frac{d x}{(2)^2+(3 x)^2}=\frac{1}{3}\left[\frac{1}{2} \tan ^{-1} \frac{3 x}{2}\right]_0^{\frac{2}{3}}=\frac{1}{6}\left[\tan ^{-1}\left(\frac{3 x}{2}\right)\right]_0^{\frac{2}{3}}\)

= \(\frac{1}{6} \tan ^{-1}\left(\frac{3}{2} \cdot \frac{2}{3}\right)-\frac{1}{6} \tan ^{-1} 0=\frac{1}{6} \tan ^{-1} 1-0=\frac{1}{6} \times \frac{\pi}{4}=\frac{\pi}{24}\).

Hence, the correct answer is (3).

Integrals Exercise 7.9

Evaluate The Integrals

Question 1. \(\int_0^1 \frac{x}{x^2+1} d x\)
Solution:

Let \(I=\int_0^1 \frac{x}{x^2+1} d x\)

Put \(\mathrm{x}^2+1=\mathrm{t} \Rightarrow 2 \mathrm{xdx}=\mathrm{dt}\)

When x=0, t=1 and when x=1, t=2

⇒ \(\int_0^1 \frac{\mathrm{x}}{\mathrm{x}^2+1} \mathrm{dx}=\frac{1}{2} \int_1^2 \frac{\mathrm{dt}}{\mathrm{t}}=\frac{1}{2}[\log |\mathrm{t}|]_1^2=\frac{1}{2}[\log 2-\log 1]=\frac{1}{2} \log 2\)

Question 2. \(\int_0^{\frac{\pi}{2}} \sqrt{\sin \phi} \cos ^5 \phi \mathrm{d} \phi\)
Solution:

Let \(I=\int_0^{\frac{\pi}{2}} \sqrt{\sin \phi} \cos ^5 \phi \mathrm{d} \phi=\int_0^{\frac{\pi}{2}} \sqrt{\sin \phi} \cos ^4 \phi \cos \phi \mathrm{d} \phi\)

= \(\int_0^{\pi / 2} \sqrt{\sin \phi}\left(1-\sin ^2 \phi\right)^2 \cdot \cos \phi d \phi\)

(because \(\cos ^2 x=1-\sin ^2 x\))

Put \(\sin \phi=\mathrm{t} \Rightarrow \cos \phi \mathrm{d} \phi=\mathrm{dt}\)

When \(\phi=0, t=0\) and when \(\phi=\frac{\pi}{2}, \mathrm{t}=1\)

∴ I = \(\int_0^1 \sqrt{t}\left(1-t^2\right)^2 d t=\int_0^1 t^{\frac{1}{2}}\left(1+t^4-2 t^2\right) d t=\int_0^1\left[t^{\frac{1}{2}}+t^{\frac{9}{2}}-2 t^{\frac{5}{2}}\right] d t\)

= \(\left[\frac{t^{\frac{3}{2}}}{\frac{3}{2}}+\frac{t^{\frac{11}{2}}}{\frac{11}{2}}-\frac{2 t^{\frac{7}{2}}}{\frac{7}{2}}\right]_0^1=\frac{2}{3}+\frac{2}{11}-\frac{4}{7}=\frac{154+42-132}{231}=\frac{64}{231}\)

Question 3. \(\int_0^1 \sin ^{-1}\left(\frac{2 x}{1+x^2}\right) d x\)
Solution:

Let \(I=\int_0^1 \sin ^{-1}\left(\frac{2 x}{1+x^2}\right) d x\)

Put \(x=\tan \theta \Rightarrow d x=\sec ^2 \theta d \theta\)

When \(\mathrm{x}=0, \theta=0\) and when \(\mathrm{x}=1, \theta=\frac{\pi}{4}\)

∴ I = \(\int_0^{\frac{\pi}{4}} \sin ^{-1}\left(\frac{2 \tan \theta}{1+\tan ^2 \theta}\right) \sec ^2 \theta d \theta \Rightarrow I=\int_0^{\frac{\pi}{4}} \sin ^{-1}(\sin 2 \theta) \sec ^2 \theta d \theta\)

I = \(\int_0^{\frac{\pi}{4}} 2 \theta \cdot \sec ^2 \theta d \theta=2 \int_0^{\frac{\pi}{4}} \theta \cdot \sec ^2 \theta d \theta\)

Taking \(\theta\) as first function and \(\sec ^2 \theta\) as second function and integrating by parts, we obtain

I = \(2\left[\theta \int \sec ^2 \theta d \theta-\int\left\{\left(\frac{d}{d \theta}(\theta)\right) \int \sec ^2 \theta d \theta\right\} d \theta\right]_0^{\frac{\pi}{4}}=2\left[\theta \tan \theta-\int \tan \theta d \theta\right]_0^{\pi / 4}\)

= \(2[\theta \tan \theta+\log |\cos \theta|]_0^{\frac{\pi}{4}}=2\left[\frac{\pi}{4} \tan \frac{\pi}{4}+\log \left|\cos \frac{\pi}{4}\right|-\log |\cos 0|\right]\)

= \(2\left[\frac{\pi}{4}+\log \left(\frac{1}{\sqrt{2}}\right)-\log 1\right]=2\left[\frac{\pi}{4}-\frac{1}{2} \log 2\right]=\frac{\pi}{2}-\log 2\)

Question 4. \(\int_0^2 x \sqrt{x+2} d x\).
Solution:

Let \(\mathrm{I}=\int_0^2 \mathrm{x} \sqrt{\mathrm{x}+2} \mathrm{dx}\)

Put \(x+2=t^2 \Rightarrow d x=2 t d t\), when, \(x=0, t=\sqrt{2}\) and when \(x^2=2, t=2\)

∴ \(\int_1^2 x \sqrt{x+2} d x=\int_{\sqrt{2}}^2\left(t^2-2\right) \sqrt{t^2} 2 t d t=2 \int_{\sqrt{2}}^2\left(t^2-2\right) t^2 d t=2 \int_{\sqrt{2}}^2\left(t^4-2 t^2\right) d t\)

= \(2\left[\frac{t^5}{5}-\frac{2 t^3}{3}\right]_{\sqrt{2}}^2=2\left[\frac{32}{5}-\frac{16}{3}-\frac{4 \sqrt{2}}{5}+\frac{4 \sqrt{2}}{3}\right]=2\left[\frac{96-80-12 \sqrt{2}+20 \sqrt{2}}{15}\right]\)

= \(2\left[\frac{16+8 \sqrt{2}}{15}\right]=\frac{16(2+\sqrt{2})}{15}=\frac{16 \sqrt{2}(\sqrt{2}+1)}{15}\)

Question 5. \(\int_0^{\frac{\pi}{2}} \frac{\sin x}{1+\cos ^2 x} d x\)
Solution:

Let \(\mathrm{I}=\int_0^{\frac{\pi}{2}} \frac{\sin \mathrm{x}}{1+\cos ^2 \mathrm{x}} \mathrm{dx}\)

Put cos x=t \Rightarrow-sin x d x=d t

When x=0, t=1 and when \(x=\frac{\pi}{2}, t=0\)

⇒ \(\int_0^{\frac{\pi}{2}} \frac{\sin \mathrm{x}}{1+\cos ^2 \mathrm{x}} \mathrm{dx}\)

= \(-\int_1^0 \frac{\mathrm{dt}}{1+\mathrm{t}^2}=-\left[\tan ^{-1} \mathrm{t}\right]_1^0=-\left[\tan ^{-1} 0-\tan ^{-1} 1\right]=-\left[-\frac{\pi}{4}\right]=\frac{\pi}{4}\)

Question 6. \(\int_0^2 \frac{d x}{x+4-x^2}\)
Solution:

Let \(\mathrm{I}=\int_0^2 \frac{\mathrm{dx}}{\mathrm{x}+4-\mathrm{x}^2}=\int_0^2 \frac{\mathrm{dx}}{-\left(\mathrm{x}^2-\mathrm{x}-4\right)}\)

= \(\int_0^2 \frac{d x}{-\left(x^2-x+\frac{1}{4}-\frac{1}{4}-4\right)}=\int_0^2 \frac{d x}{-\left[\left(x-\frac{1}{2}\right)^2-\frac{17}{4}\right]}\)

= \(\int_0^2 \frac{d x}{\left(\frac{\sqrt{17}}{2}\right)^2-\left(x-\frac{1}{2}\right)^2}\)

Question 7. \(\int_{-1}^1 \frac{d x}{x^2+2 x+5}\)
Solution:

Let \(I=\int_{-1}^1 \frac{d x}{x^2+2 x+5}=\int_{-1}^1 \frac{d x}{\left(x^2+2 x+1\right)+4}=\int_{-1}^1 \frac{d x}{(x+1)^2+(2)^2}\)

Put \(\mathrm{x}+1=\mathrm{t} \Rightarrow \mathrm{dx}=\mathrm{dt}\)

When x=-1, t=0 and when x=1, t=2

∴ \(\int_{-1}^1 \frac{\mathrm{dx}}{(\mathrm{x}+1)^2+(2)^2}=\int_0^2 \frac{\mathrm{dt}}{\mathrm{t}^2+2^2}\)

= \(\left[\frac{1}{2} \tan ^{-1} \frac{\mathrm{t}}{2}\right]_0^2=\frac{1}{2} \tan ^{-1} 1-\frac{1}{2} \tan ^{-1} 0=\frac{1}{2}\left(\frac{\pi}{4}\right)=\frac{\pi}{8}\)

Question 8. \(\int_1^2\left(\frac{1}{x}-\frac{1}{2 x^2}\right) e^{2 x} d x\)
Solution:

Let \(I=\int_1^2\left(\frac{1}{x}-\frac{1}{2 x^2}\right) e^{2 x} d x\)

Put \(2 \mathrm{x}=\mathrm{t} \Rightarrow 2 \mathrm{dx}=\mathrm{d} t\)

When x=1, t=2 and when x=2, t=4

∴ \(\int_1^2\left(\frac{1}{x}-\frac{1}{2 x^2}\right) e^{2 x} d x=\frac{1}{2} \int_2^4\left(\frac{2}{t}-\frac{2}{t^2}\right) e^t d t\)

= \(\int_2^4\left(\frac{1}{t}-\frac{1}{t^2}\right) e^t d t\) (therefore \(\int e^t\left[f(t)+f^{\prime}(t)\right] d t=e^1 f(t)+C\))

= \(\left[\frac{e^t}{t}\right]_2^4=\frac{e^4}{4}-\frac{e^2}{2}=\frac{e^2\left(e^2-2\right)}{4}\)

Choose The Correct Answer

Question 9. The value of the integral \(\int_{\frac{1}{3}}^1 \frac{\left(x-x^3\right)^{\frac{1}{3}}}{x^4} d x\) is ?

1. 6
2. 0
3. 3
4. 4

Solution:

Let \(I=\int_{\frac{1}{3}}^1 \frac{\left(x-x^3\right)^{\frac{1}{3}}}{x^4} d x \Rightarrow I=\int_{\frac{1}{3}}^1 \frac{x\left(\frac{1}{x^2}-1\right)^{\frac{1}{3}}}{x^4} d x \Rightarrow I=\int_{\frac{1}{3}}^1 \frac{\left(\frac{1}{x^2}-1\right)^{\frac{1}{3}}}{x^3} d x\)

Put \(\frac{1}{x^2}-1=t^3,-\frac{2}{x^3} \cdot d x=3 t^2 d t, \quad \frac{d x}{x^3}=\frac{-3}{2} t^2 d t\)

When x=1 then t=0 and when \(x=\frac{1}{3}\) then t=2

= \(-\frac{3}{2} \int_2^0\left(\mathrm{t}^3\right)^{1 / 3} \cdot \mathrm{t}^2 \mathrm{dt}=\frac{3}{2} \int_0^2 \mathrm{t}^3 \mathrm{dt}=\frac{3}{2}\left[\frac{\mathrm{t}^4}{4}\right]_0^2=\frac{3}{2} \times \frac{1}{4}\left[2^4-0\right]=\frac{3}{8} \times 16=6\)

Hence, the correct answer is (1).

Question 10. If \(f(x)=\int_0^x t \sin t d t\), then \(f^{\prime}(x)\) is

1. \(\cos \mathrm{x}+\mathrm{x} \sin \mathrm{x}\)
2. \(x \sin \mathrm{x}\)
3. \(x \cos x\)
4. \(\sin x+x \cos x\)

Solution: 2. \(x \sin \mathrm{x}\)

f(x) = \(\int_0^x t \sin t d t\)

Differentiation on both sides \(f^4(x)=(t \sin t)_0^x\)

∴ \(f^4(x)=x \sin x\)

Hence, the correct answer is (2).

Integrals Exercise 7.10

By using the properties of definite integrals.

Question 1. \(\int_0^{\frac{\pi}{2}} \cos ^2 x d x\)
Solution:

I = \(\int_0^{\frac{\pi}{2}} \cos ^2 x d x\)….(1)

⇒ I = \(\int_0^{\frac{\pi}{2}} \cos ^2\left(\frac{\pi}{2}-x\right) d x\) (because \(\int_0^a f(x) d x=\int_0^a f(a-x) d x\))

⇒ I = \(\int_0^{\frac{\pi}{2}} \sin ^2 x d x\)….(2)

Adding (1) and (2), we obtain

2I = \(\int_0^{\frac{\pi}{2}}\left(\sin ^2 x+\cos ^2 x\right) d x \Rightarrow 2 I=\int_0^{\frac{\pi}{2}} 1 \cdot d x\)

2I = \([x]_0^{\frac{\pi}{2}} \Rightarrow 2 I=\frac{\pi}{2} \Rightarrow I=\frac{\pi}{4}\)

Question 2. \(\int_0^{\frac{\pi}{2}} \frac{\sqrt{\sin x}}{\sqrt{\sin x}+\sqrt{\cos x}} d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{2}} \frac{\sqrt{\sin x}}{\sqrt{\sin x}+\sqrt{\cos x}} d x\)….(1)

I = \(\int_0^{\frac{\pi}{2}} \frac{\sqrt{\sin \left(\frac{\pi}{2}-x\right)}}{\sqrt{\sin \left(\frac{\pi}{2}-x\right)+\sqrt{\cos \left(\frac{\pi}{2}-x\right)}}} d x\)

⇒ I = \(\int_0^{\frac{\pi}{2}} \frac{\sqrt{\cos x}}{\sqrt{\cos x}+\sqrt{\sin x}} d x\)….(2)

Adding (1) and (2), we obtain

2I = \(int_0^{\frac{\pi}{2}} \frac{\sqrt{\sin x}+\sqrt{\cos x}}{\sqrt{\sin x}+\sqrt{\cos x}} d x\)

2I = \(\int_0^{\frac{\pi}{2}} 1 \cdot d x \Rightarrow 2 I=[x]_0^{\frac{\pi}{2}} \Rightarrow 2 I=\frac{\pi}{2} \Rightarrow I=\frac{\pi}{4}\)

Question 3. \(\int_0^{\frac{\pi}{2}} \frac{\sin ^{\frac{3}{2}} x d x}{\sin ^{\frac{3}{2}} x+\cos ^{\frac{3}{2}} x}\)
Solution:

Let \(I=\int_0^{\frac{\pi}{2}} \frac{\sin ^{\frac{3}{2}} x}{\sin ^{\frac{3}{2}} x+\cos ^{\frac{3}{2}} x} d x\)….(1)

⇒ I = \(\int_0^{\frac{\pi}{2}} \frac{\sin ^{\frac{3}{2}}\left(\frac{\pi}{2}-x\right)}{\sin ^{\frac{3}{2}}\left(\frac{\pi}{2}-x\right)+\cos ^{\frac{3}{2}}\left(\frac{\pi}{2}-x\right)} d x\)

(because \(\int_0^a f(x) d x=\int_0^a f(a-x) d x\))

⇒ I = \(\int_0^{\frac{\pi}{2}} \frac{\cos ^{\frac{3}{2}} \mathrm{x}}{\sin ^{\frac{3}{2}} \mathrm{x}+\cos ^{\frac{3}{2}} \mathrm{x}} \mathrm{dx}\)….(2)

Adding (1) and (2), we obtain

2I = \(int_0^{\frac{\pi}{2}} \frac{\sin ^{\frac{3}{2}} x+\cos ^{\frac{3}{2}} x}{\sin ^{\frac{3}{2}} x+\cos ^{\frac{3}{2}} x} d x \Rightarrow 2 I=\int_0^{\frac{\pi}{2}} 1 \cdot d x=[x]_0^{\frac{\pi}{2}} \Rightarrow 2 I=\frac{\pi}{2} \Rightarrow I=\frac{\pi}{4}\)

Question 4. \(\int_0^{\frac{\pi}{2}} \frac{\cos ^5 x d x}{\sin ^5 x+\cos ^5 x}\)
Solution:

Let \(I=\int_0^{\frac{\pi}{2}} \frac{\cos ^5 x}{\sin ^5 x+\cos ^5 x} d x\)

⇒ I = \(\int_0^{\frac{\pi}{2}} \frac{\cos ^5\left(\frac{\pi}{2}-x\right)}{\sin ^5\left(\frac{\pi}{2}-x\right)+\cos ^5\left(\frac{\pi}{2}-x\right)} d x\)

(because \(\int_0^a f(x) d x=\int_0^a f(a-x) d x\))

⇒ I = \(\int_0^{\frac{\pi}{2}} \frac{\sin ^5 x}{\sin ^5 x+\cos ^5 x} d x\)

Adding (1) and (2), we obtain

2I = \(\int_0^\pi 2 \frac{\sin ^5 x+\cos ^5 x}{\sin ^5 x+\cos ^5 x} d x \Rightarrow 2 I=\int_0^{\frac{\pi}{2}} 1 \cdot d x \Rightarrow 2 I=[x]_0^{\frac{\pi}{2}} \Rightarrow 2 I=\frac{\pi}{2} \Rightarrow I=\frac{\pi}{4}\)

Question 5. \(\int_{-5}^5|x+2| d x\)
Solution:

Let \(I=\int_{-5}^5|x+2| d x\)

I = \(\int_{-5}^{-2}|x+2| d x+\int_{-2}^5|x+2| d x\)

∴ I = \(\int_{-5}^{-2}-(x+2) d x+\int_{-2}^5(x+2) d x\) (because \(\int_a^b f(x) d x=\int_a^c f(x) d x+\int_c^b f(x) d x\))

I = \(-\left[\frac{x^2}{2}+2 x\right]_{-5}^{-2}+\left[\frac{x^2}{2}+2 x\right]_{-2}^5\)

= \(-\left[\frac{(-2)^2}{2}+2(-2)-\frac{(-5)^2}{2}-2(-5)\right]+\left[\frac{(5)^2}{2}+2(5)-\frac{(-2)^2}{2}-2(-2)\right]\)

= \(-\left[2-4-\frac{25}{2}+10\right]+\left[\frac{25}{2}+10-2+4\right]=-2+4+\frac{25}{2}-10+\frac{25}{2}+10-2+4\)

= 29

Question 6. \(\int_2^8|x-5| d x\)
Solution:

Let \(\mathrm{I}=\int_2^8|\mathrm{x}-5| \mathrm{dx}\)

I = \(\int_2^5|x-5| d x+\int_5^8|x-5| d x\)

I = \(\int_2^5-(x-5) d x+\int_5^8(x-5) d x\) (because \(\int_a^b f(x) d x=\int_a^c f(x) d x+\int_c^b f(x) d x\))

= \(-\left[\frac{x^2}{2}-5 x\right]_2^5+\left[\frac{x^2}{2}-5 x\right]_5^8\)

= \(-\left[\frac{25}{2}-25-2+10\right]+\left[32-40-\frac{25}{2}+25\right]=9\)

Question 7. \(\int_0^1 x(1-x)^n d x\)
Solution:

Let \(\mathrm{I}=\int_0^1 \mathrm{x}(1-\mathrm{x})^n \mathrm{dx}\)

∴ I = \(\int_0^1(1-x)(1-(1-x))^n d x\) (because \(\int_0^a f(x) d x=\int_0^a f(a-x) d x\))

= \(\int_0^1(1-x)(x)^n d x=\int_0^1\left(x^n-x^{n+1}\right) d x\)

= \(\left[\frac{x^{n+1}}{n+1}-\frac{x^{n+2}}{n+2}\right]_0^1=\left[\frac{1}{n+1}-\frac{1}{n+2}\right]\)

= \(\frac{(n+2)-(n+1)}{(n+1)(n+2)}=\frac{1}{(n+1)(n+2)}\)

Question 8. \(\int_0^{\frac{\pi}{4}} \log (1+\tan x) d x\)
Solution:

Let \(\mathrm{I}=\int_0^{\frac{\pi}{4}} \log (1+\tan \mathrm{x}) \mathrm{dx}\)

∴ I = \(\int_0^{\frac{\pi}{4}} \log \left[1+\tan \left(\frac{\pi}{4}-x\right)\right] d x \)

(because \(\int_0^\pi f(x) d x=\int_0^a f(a-x) d x\))

⇒ I = \(\int_0^{\frac{\pi}{4}} \log \left\{1+\frac{\tan \frac{\pi}{4}-\tan x}{1+\tan \frac{\pi}{4} \tan x}\right\} d x \Rightarrow I=\int_0^{\frac{\pi}{4}} \log \left\{1+\frac{1-\tan x}{1+\tan x}\right\} d x\)

⇒ I = \(\int_0^{\frac{\pi}{4}}\left\{\log \frac{2}{(1+\tan x)}\right\} d x \Rightarrow I=\int_0^{\frac{\pi}{4}} \log 2 d x-\int_0^{\frac{\pi}{4}} \log (1+\tan x) d x\)

⇒ I = \(\int_0^{\frac{\pi}{4}} \log 2 d x-I\) [From (1)]

⇒ 2I = \([x \log 2]_0^{\frac{\pi}{4}} \Rightarrow 2 I=\frac{\pi}{4} \log 2 \Rightarrow I=\frac{\pi}{8} \log 2\)

Question 9. \(\int_0^{-2} x \sqrt{2-x} d x\)
Solution:

Let \(\mathrm{I}=\int_0^2 \mathrm{x} \sqrt{2-\mathrm{x}} \mathrm{dx}\)

I = \(\int_0^2(2-x) \sqrt{x} d x\) (because \(\int_0^{11} f(x) d x=\int_0^0 f(a-x) d x\))

= \(\int_0^2\left\{2 x^{\frac{1}{2}}-x^{\frac{3}{2}}\right\} d x=\left[2\left(\frac{x^{\frac{3}{2}}}{\frac{3}{2}}\right)-\frac{x^{\frac{5}{2}}}{\frac{5}{2}}\right]_0^2\)

= \(\left[\frac{4}{3} x^{\frac{3}{2}}-\frac{2}{5} x^{\frac{5}{2}}\right]_0^2=\frac{4}{3}(2)^{\frac{3}{2}}-\frac{2}{5}(2)^{\frac{5}{2}}\)

= \(\frac{4 \times 2 \sqrt{2}}{3}-\frac{2}{5} \times 4 \sqrt{2}=\frac{8 \sqrt{2}}{3}-\frac{8 \sqrt{2}}{5}=\frac{40 \sqrt{2}-24 \sqrt{2}}{15}=\frac{16 \sqrt{2}}{15}\)

Question 10. \(\int_0^{\frac{\pi}{2}}(2 \log \sin x-\log \sin 2 x) d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{2}}(2 \log \sin x-\log \sin 2 x) d x\)

I = \(\int_0^{\frac{\pi}{2}} \log \left(\frac{\sin ^2 x}{\sin 2 x}\right) d x=\int_0^{\frac{\pi}{2}} \log \left(\frac{\sin ^2 x}{2 \sin x \cos x}\right) d x\)

= \(\int_0^{\frac{\pi}{2}} \log \left(\frac{\tan x}{2}\right) d x=\int_0^{\frac{\pi}{2}} \log \tan x d x-\int_0^{\frac{\pi}{2}} \log 2 d x\)

I = \(I^{\prime}-\log 2(x)^{\frac{\pi}{2}} \Rightarrow I=I^{\prime}-\frac{\pi}{2} \log 2\)….(1)

Now, \(I^{\prime}=\int_0^{\frac{\pi}{2}} \log \tan x d x\)….(2)

= \(\int_0^{\frac{\pi}{2}} \log \tan \left(\frac{\pi}{2}-x\right) d x\) (because \(\int_0^2 f(x) d x=\int_0^\pi f(a-x) d x\))

= \(\int_0^{\frac{\pi}{2}} \log \cot x d x\)….(3)

Adding equation (2) and (3)

⇒ \(2 I^{\prime}=\int_0^{\frac{\pi}{2}}(\log \tan x+\log \cot x) d x=\int_0^{\frac{\pi}{2}}(\log \tan x \cot x) d x\)

= \(\int_0^{\frac{\pi}{2}} \log 1 d x\)

2I’ =0

I’=0 put in equation (1)

I = \(0-\frac{\pi}{2} \log 2\)

I = \(\frac{\pi}{2} \log \frac{1}{2}\)

Question 11. \(\int_{\frac{-\pi}{2}}^{\frac{\pi}{2}} \sin ^2 x d x\)
Solution:

Let \(I=\int_{\frac{-\pi}{2}}^{\frac{\pi}{2}} \sin ^2 x d x \Rightarrow 2 \int_0^{\frac{\pi}{2}} \sin ^2 x d x\)….(1)

(\(\int_{-a}^2 f(x) d x=2 \int_0^n f(x) d x\), when f(x) is even function

⇒ I = \(2 \int_0^{\frac{\pi}{2}} \sin ^2\left(\frac{\pi}{2}-x\right) d x\) (because \(\int_0^a f(x) d x=\int_0^a f(a-x) d x\))

⇒ I = \(2 \int_0^{\frac{\pi}{2}} \cos ^2 x d x\)….(2)

Adding equation (1) and (2),

2I = \(2 \int_0^{\frac{\pi}{2}}\left(\sin ^2 x+\cos ^2 x\right) d x=2 \int_0^{\frac{\pi}{2}} 1 d x=2(x)_0^{\frac{\pi}{2}}\)

= \(2 \cdot \frac{\pi}{2}=\pi \Rightarrow 2 I=\pi \Rightarrow I=\frac{\pi}{2} \Rightarrow I=\frac{\pi}{2}\)

Question 12. \(\int_0^\pi \frac{x}{1+\sin x} d x\)
Solution:

Let \(I=\int_0^\pi \frac{x}{1+\sin x} d x\)….(1)

⇒ I = \(\int_0^\pi \frac{(\pi-x)}{1+\sin (\pi-x)} d x\) (because \(\int_0^\pi f(x) d x=\int_0^a f(a-x) d x\))

⇒ I = \(\int_0^\pi \frac{(\pi-x)}{1+\sin x} d x\)….(2)

Adding eq. (1) and (2), we obtain

2I =\(\int_0^\pi \frac{\pi}{1+\sin x} d x \Rightarrow 2 I=\pi \int_0^\pi \frac{(1-\sin x)}{(1+\sin x)(1-\sin x)} d x \Rightarrow 2 I=\pi \int_0^\pi \frac{1-\sin x}{\cos ^2 x} d x\)

⇒ 2I = \(\pi \int_0^\pi\left\{\sec ^2 x-\tan x \sec x\right\} d x\)

⇒ 2I = \(\pi[\tan x-\sec x]_0^\pi=\pi[\tan \pi-\tan 0]-\pi[\sec \pi-\sec 0]=\pi[0-0]-\pi[-1-1]\)

⇒ 2I = \(2 \pi \Rightarrow I=\pi\)

Question 13. \(\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \sin ^7 x d x\)
Solution:

Let \(\mathrm{I}=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \sin ^7 \mathrm{xdx}\)

As \(\sin ^7(-x)=(\sin (-x))^7=(-\sin x)^7=-\sin ^7 x\), therefore, \(\sin ^7 x\) is an odd function.

It is known that, if f(x) is an odd function, then \(\int_{-\alpha}^a f(x) d x=0\)

∴ \(I=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \sin ^7 x d x=0\)

Question 14. \(\int_0^{2 \pi} \cos ^5 x d x\)
Solution:

Let \(I=\int_0^{2 \pi} \cos ^5 x d x\)

f(x) = \(\cos ^5 x \text {, then } f(2 \pi-x)=\cos ^5(2 \pi-x)=\cos ^5 x=f(x)\)

(because \(\int_0^{2 a} f(x) d x=2 \int_0^a f(x) d x \text {, if } f(2 a-x)=f(x)\))

∴ I = \(2 \int_0^\pi \cos ^5 x d x\)

I = \(2 \int_0^\pi \cos ^5(\pi-x) d x=-2 \int_0^\pi \cos ^3 x d x\) (because \(\int_0^a f(x) d x=\int_0^a f(a-x) d x\))

I = \(-2 \int_0^\pi \cos ^5 x d x=-I \Rightarrow 2 I=0 \Rightarrow I=0\) [From eq. (1)]

Question 15. \(\int_0^{\frac{\pi}{2}} \frac{\sin x-\cos x}{1+\sin x \cos x} d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{2}} \frac{\sin x-\cos x}{1+\sin x \cos x} d x\)….(1)

⇒ I = \(\int_0^{\frac{\pi}{2}} \frac{\sin \left(\frac{\pi}{2}-x\right)-\cos \left(\frac{\pi}{2}-x\right)}{1+\sin \left(\frac{\pi}{2}-x\right) \cos \left(\frac{\pi}{2}-x\right)} d x\)

⇒ I = \(\int_0^{\frac{\pi}{2}} \frac{\cos x-\sin x}{1+\sin x \cos x} d x\)…..(2)

Adding (1) and (2), we obtain, \(2 I=\int_0^{\frac{\pi}{2}} \frac{0}{1+\sin x \cos x} d x \Rightarrow I=0\)

Question 16. \(\int_0^\pi \log (1+\cos \mathrm{x}) \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int_0^\pi \log (1+\cos \mathrm{x}) \mathrm{dx}\)….(1)

⇒ \(\mathrm{I}=\int_0^\pi \log (1+\cos (\pi-\mathrm{x})) \mathrm{dx}\) (because \(\int_0^a f(x) d x=\int_0^a f(a-x) d x\))

⇒ \(\mathrm{I}=\int_0^\pi \log (1-\cos \mathrm{x}) \mathrm{dx}\)…..(2)

So, \(1+\sin x \cos x\)

Adding (1) and (2), we obtain

2I = \(\int_0^\pi\{\log (1+\cos x)+\log (1-\cos x)\} d x \Rightarrow 2 I=\int_0^\pi \log \left(1-\cos ^2 x\right) d x\)

⇒ 2I = \(\int_0^\pi \log \sin ^2 x d x \Rightarrow 2 I=2 \int_0^\pi \log \sin x d x\)

⇒ I = \(\int_0^\pi \log \sin x d x\) (because \(sin (\pi-x)=\sin x\))…(3)

⇒ I = \(2 \int_0^{\frac{\pi}{2}} \log \sin x d x\)….(4)

∴ I = \(2 \int_0^{\frac{\pi}{2}} \log \sin \left(\frac{\pi}{2}-x\right) d x=2 \int_0^{\frac{\pi}{2}} \log \cos x d x\)…(5)

Adding (4) and (5), we obtain

2I = \(2 \int_0^{\frac{\pi}{2}}(\log \sin \mathrm{x}+\log \cos \mathrm{x}) \mathrm{dx} \Rightarrow \mathrm{I}=\int_0^{\frac{\pi}{2}}(\log \sin \mathrm{x}+\log \cos \mathrm{x}+\log 2-\log 2) \mathrm{dx}\)

⇒ \(\mathrm{I}=\int_0^{\frac{\pi}{2}}(\log 2 \sin \mathrm{x} \cos \mathrm{x}-\log 2) \mathrm{dx} \Rightarrow \mathrm{I}=\int_0^{\frac{\pi}{2}} \log \sin 2 \mathrm{xdx}-\int_0^{\frac{\pi}{2}} \log 2 \mathrm{dx}\)

Let \(2 \mathrm{x}=\mathrm{t} \Rightarrow 2 \mathrm{dx}=\mathrm{dt}\)

When \(\mathrm{x}=0, \mathrm{t}=0\) and when \(\mathrm{x}=\frac{\pi}{2}, \mathrm{t}=\pi\)

∴ I = \(\frac{1}{2} \int_0^\pi \log \sin t d t-\frac{\pi}{2} \log 2 \Rightarrow I=\frac{1}{2} \int_0^\pi \log \sin x d x-\frac{\pi}{2} \log 2\)

⇒ I = \(\frac{1}{2} I-\frac{\pi}{2} \log 2 \Rightarrow \frac{I}{2}=-\frac{\pi}{2} \log 2 \Rightarrow I=-\pi \log 2\)

(because \(int_a^b f(x) d x=\int_a^b f(t) d t\)) [From eq.(3)]

Question 17. \(\int_0^2 \frac{\sqrt{\mathrm{x}}}{\sqrt{\mathrm{x}}+\sqrt{\mathrm{a}-\mathrm{x}}} \mathrm{dx}\)
Solution:

Let \(I=\int_0^a \frac{\sqrt{x}}{\sqrt{x}+\sqrt{a-x}} d x\)…..(1)

I = \(\int_0^a \frac{\sqrt{a-x}}{\sqrt{a-x}+\sqrt{x}} d x\)….(2) (because \(\int_0^a f(x) d x=\int_0^a f(a-x) d x\))

Adding eq. (1) and (2), we obtain

2I = \(\int_0^a \frac{\sqrt{x}+\sqrt{a-x}}{\sqrt{x}+\sqrt{a-x}} d x \Rightarrow 2 I=\int_0^\pi 1 d x \Rightarrow 2 I=[x]_0^\pi \Rightarrow 2 I=a \Rightarrow I=\frac{a}{2}\)

Question 18. \(\int_0^4|x-1| d x\)
Solution:

Let \(\mathrm{I}=\int_0^4|\mathrm{x}-1| \mathrm{dx}\)

I = \(\int_0^1|x-1| d x+\int_1^4|x-1| d x\)

= \(\int_0^1-(x-1) d x+\int_1^4(x-1) d x\) (because \(\int_a^b f(x) d x=\int_a^0 f(x) d x+\int_c^b f(x) d x\))

I = \(-\left[\frac{x^2}{2}-x\right]_0^1+\left[\frac{x^2}{2}-x\right]_1^4\)

= \(-\left[\left(\frac{1}{2}-1\right)-0\right]+\left[\left(\frac{16}{2}-4\right)-\left(\frac{1}{2}-1\right)\right]=\frac{1}{2}+8-4+\frac{1}{2}=5\)

Question 19. Show that \(\int_0^a f(x) g(x) d x=2 \int_0^a f(x) d x\), if f and g are defined as f(x)=f(a-x) and \(\mathrm{g}(\mathrm{x})+\mathrm{g}(\mathrm{a}-\mathrm{x})=4\)
Solution:

Let I = \(\int_0^a f(x) g(x) d x\)….(1)

⇒ \(\mathrm{I}=\int_0^{\mathrm{a}} \mathrm{f}(\mathrm{a}-\mathrm{x}) \mathrm{g}(\mathrm{a}-\mathrm{x}) \mathrm{dx}\)

⇒ \(\mathrm{I}=\int_0^{\mathrm{a}} \mathrm{f}(\mathrm{x}) \mathrm{g}(\mathrm{a}-\mathrm{x}) \mathrm{dx}\)

⇒ \(\left(\int_0^a f(x) d x=\int_0^a f(a-x) d x\right)\)….(2)

Adding eq. (1) and (2), we obtain

2I = \(\int_0^a\{f(x) g(x)+f(x) g(a-x)\} d x\)

⇒ 2I = \(\int_0^a f(x)\{g(x)+g(a-x)\} d x \Rightarrow 2 I=\int_0^a f(x) \times 4 d x\) (Given g(x)+g(a-x)=4)

⇒ \(2 I=4 \int_0^a f(x) d x\)

⇒ \(I=2 \int_0^a f(x) d x\)

Hence proved

Choose The Correct Answer

Question 20. The value of \(\int_{\frac{\pi}{2}}^{\frac{\pi}{2}}\left(x^3+x \cos x+\tan ^5 x+1\right) d x\) is?

1. 0
2. 2
3. \(\pi\)
4. 1

Solution: 3. \(\pi\)

Let \(I=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}}\left(x^3+x \cos x+\tan ^5 x+1\right) d x\)

⇒ I = \(\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} x^2 d x+\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} x \cos x+\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \tan ^5 x d x+\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} 1 . d x\)

It is known that if f(x) is an even function, then \(\int_{-a}^a f(x) d x=2 \int_0^a f(x) d x\) and if f(x) is an odd function, then \(\int_{-2}^a f(x) d x=0\)

⇒ \(\mathrm{I}=0+0+0+\int_{\frac{-\pi}{2}}^{\frac{\pi}{2}} 1 \cdot \mathrm{dx}\) (because \(x^3, x \cos x\), and \(\tan ^5 x\) are odd functions)

⇒ \(\mathrm{I}=[\mathrm{x}]_{\frac{-\pi}{2}}^{\frac{\pi}{2}}\)

⇒ \(\mathrm{I}=\left[\frac{\pi}{2}+\frac{\pi}{2}\right]=\frac{2 \pi}{2}=\pi\)

Hence, the correct answer is (3).

Question 21. The value of \(\int_0^{\frac{\pi}{2}} \log \left(\frac{4+3 \sin x}{4+3 \cos x}\right) d x\) is?

1. 2
2. \(\frac{3}{4}\)
3. 0
4. -2

Solution: 3. 0

Let \(I=\int_0^{\frac{\pi}{2}} \log \left(\frac{4+3 \sin x}{4+3 \cos x}\right) d x\)

⇒ I = \(\int_0^{\frac{\pi}{2}} \log \left[\frac{4+3 \sin \left(\frac{\pi}{2}-x\right)}{4+3 \cos \left(\frac{\pi}{2}-x\right)}\right] d x\) (because \(\int_0^a f(x) d x=\int_0^a f(a-x) d x\))

⇒ \(\mathrm{I}=\int_0^{\frac{\pi}{2}} \log \left(\frac{4+3 \cos \mathrm{x}}{4+3 \sin \mathrm{x}}\right) \mathrm{dx}\)

Adding (1) and (2), we obtain

2I = \(\int_0^{\frac{\pi}{2}}\left\{\log \left(\frac{4+3 \sin x}{4+3 \cos x}\right)+\log \left(\frac{4+3 \cos x}{4+3 \sin x}\right)\right\} d x\)

2I = \(\int_0^{\frac{\pi}{2}}\left\{\log \left(\frac{4+3 \sin x}{4+3 \cos x} \times \frac{4+3 \cos x}{4+3 \sin x}\right)\right\} d x\)

⇒ 2I = \(\int_0^{\frac{\pi}{2}} \log 1 d x\)

⇒ 2I = \(\int_0^{\frac{\pi}{2}} 0 d x \Rightarrow I=0\)

Hence, the correct answer is(3).

Miscellaneous Exercise Integrals

Integrated The Functions

Question 1. \(\int \frac{1}{x-x^3} d x\)
Solution:

Let \(I=\int \frac{1}{x-x^3} d x=\int \frac{1}{x\left(1-x^2\right)} d x=\int \frac{1}{x(1-x)(1+x)} d x\)

⇒ \(\frac{1}{x(1-x)(1+x)}=\frac{A}{x}+\frac{B}{(1-x)}+\frac{C}{(1+x)}\)

I = \(A\left(1-x^2\right)+B x(1+x)+C x(1-x)\)

I = \(A-A x^2+B x+B x^2+C x-C x^2\)…(1) (using partial fraction)

Equating the coefficients of \(\mathrm{x}^2, \mathrm{x}\), and constant term, we obtain

– \(\mathrm{A}+\mathrm{B}-\mathrm{C}=0, \mathrm{~B}+\mathrm{C}=0, \mathrm{~A}=1\)

On solving these equations, we obtain \(\mathrm{A}=1, \mathrm{~B}=\frac{1}{2}\), and \(\mathrm{C}=-\frac{1}{2}\)

From equation (1), we obtain

⇒ \(\frac{1}{x(1-x)(1+x)}=\frac{1}{x}+\frac{1}{2(1-x)}-\frac{1}{2(1+x)}\)

⇒ \(\int \frac{1}{x(1-x)(1+x)} d x=\int \frac{1}{x} d x+\frac{1}{2} \int \frac{1}{1-x} d x-\frac{1}{2} \int \frac{1}{1+x} d x\)

= \(\log |x|-\frac{1}{2} \log |(1-x)|-\frac{1}{2} \log |(1+x)|=\log |x|-\log \left|(1-x)^{\frac{1}{2}}\right|-\log \left|(1+x)^{\frac{1}{2}}\right|\)

= \(\log \left|\frac{x}{(1-x)^{\frac{1}{2}}(1+x)^{\frac{1}{2}}}\right|+C=\log \left|\left(\frac{x^2}{1-x^2}\right)^{\frac{1}{2}}\right|+C=\frac{1}{2} \log \left|\frac{x^2}{1-x^2}\right|+C\)

Question 2. \(\int \frac{1}{\sqrt{(x+a)}+\sqrt{(x+b)}} d x\)
Solution:

Let \(I=\int \frac{1}{\sqrt{(x+a)}+\sqrt{(x+b)}} d x\)

Now, \(\int \frac{1}{\sqrt{x+a}+\sqrt{x+b}} d x=\int \frac{1}{\sqrt{x+a}+\sqrt{x+b}} \times \frac{\sqrt{x+a}-\sqrt{x+b}}{\sqrt{x+a}-\sqrt{x+b}} d x\)

⇒ \(\int \frac{1}{\sqrt{x+a}+\sqrt{x+b}} d x=\frac{1}{a-b} \int(\sqrt{x+a}-\sqrt{x+b}) d x \)

⇒ I = \(\frac{1}{(a-b)}\left[\frac{(x+a)^{\frac{3}{2}}}{\frac{3}{2}}-\frac{(x+b)^{\frac{3}{2}}}{\frac{3}{2}}\right]+C=\frac{2}{3(a-b)}\left[(x+a)^{\frac{3}{2}}-(x+b)^{\frac{3}{2}}\right]+C\)

Question 3. \(\int \frac{1}{x \sqrt{a x-x^2}} d x\)
Solution:

Let \(I=\int \frac{1}{x \sqrt{a x-x^2}} d x\)

Put \(x=\frac{a}{t} \Rightarrow d x=-\frac{a}{t^2} d t\)

⇒ \(\int \frac{1}{x \sqrt{a x-x^2}} d x=\int \frac{1}{\frac{a}{t} \sqrt{a \cdot \frac{a}{t}-\left(\frac{a}{t}\right)^2}}\left(-\frac{a}{t^2} d t\right)=-\int \frac{1}{a t} \cdot \frac{1}{\sqrt{\frac{1}{t}-\frac{1}{t^2}}} d t=-\frac{1}{a} \int \frac{1}{\sqrt{\frac{t^2}{t}-\frac{t^2}{t^2}}} d t\)

= \(-\frac{1}{a} \int \frac{1}{\sqrt{t-1}} d t=-\frac{1}{a}[2 \sqrt{t-1}]+C=-\frac{1}{a}\left[2 \sqrt{\frac{a}{x}-1}\right]+C\)

= \(-\frac{2}{a}\left(\frac{\sqrt{a-x}}{\sqrt{x}}\right)+C=-\frac{2}{a}\left(\sqrt{\frac{a-x}{x}}\right)+C\)

Question 4. \(\int \frac{1}{x^2\left(x^4+1\right)^{\frac{3}{4}}} d x\)
Solution:

Let \(I=\int \frac{1}{x^2\left(x^4+1\right)^{\frac{3}{4}}} d x\)

I = \(\int \frac{d x}{x^2\left(x^4\right)^{3 / 4}\left(1+\frac{1}{x^4}\right)^{3 / 4}}=\int \frac{d x}{x^5\left(1+\frac{1}{x^4}\right)^{3 / 4}}\)

I = \(\int\left(1+x^{-4}\right)^{-3 / 4} x^{-5} d x\)

Put \(\left(1+x^{-4}\right)=t \Rightarrow-4 x^{-5} d x=d t\) or \(x^{-5} d x=\frac{-d t}{4}\)

= \(\int \mathrm{t}^{-3 / 4}\left(\frac{-\mathrm{dt}}{4}\right)=-\frac{1}{4} \int \mathrm{t}^{-3 / 4} \mathrm{dt}=-\frac{1}{4} \) \(\frac{\mathrm{t}^{-\frac{3}{4}+1}}{-\frac{3}{4}+1}+\mathrm{C}=\frac{1}{4}\left[\frac{\mathrm{t}^{1 / 4}}{\frac{1}{4}}\right]+\mathrm{C}=-\left(1+\frac{1}{\mathrm{x}^4}\right)^{\frac{1}{4}}+\mathrm{C}\)

Question 5. \(\int \frac{1}{x^{\frac{1}{2}}+x^{\frac{1}{3}}} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{1}{\mathrm{x}^{\frac{1}{2}}+\mathrm{x}^{\frac{1}{3}}} \mathrm{dx}\)

Put \(x=t^6 \Rightarrow d x=6 t^5 d t\)

∴ \(\int \frac{1}{x^{\frac{1}{2}}+x^{\frac{1}{3}}} d x=\int \frac{1}{t^{6 / 2}+t^{6 / 3}} \cdot\left(6 t^5\right) d t=\int \frac{6 t^3}{t^2(1+t)} d t=6 \int \frac{t^3}{(1+t)} d t\)

On dividing, we obtain \(\int \frac{1}{x^{\frac{1}{2}}+x^{\frac{1}{3}}} d x=6 \int\left[\left(t^2-t+1\right)-\frac{1}{1+t}\right] d t\)

= \(=6\left[\left(\frac{t^3}{3}\right)-\left(\frac{t^2}{2}\right)+t-\log |1+t|\right]+C\)

= \(2 x^{\frac{1}{2}}-3 x^{\frac{1}{3}}+6 x^{\frac{1}{6}}-6 \log \left(1+x^{\frac{1}{6}}\right)+C=2 \sqrt{x}-3 x^{\frac{1}{3}}+6 x^{\frac{1}{6}}-6 \log \left(1+x^{\frac{1}{6}}\right)+C\)

Question 6. \(\int \frac{5 x}{(x+1)\left(x^2+9\right)} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{5 \mathrm{x}}{(\mathrm{x}+1)\left(\mathrm{x}^2+9\right)} \mathrm{dx}\)

⇒ \(\frac{5 \mathrm{x}}{(\mathrm{x}+1)\left(\mathrm{x}^2+9\right)}=\frac{\mathrm{A}}{(\mathrm{x}+1)}+\frac{\mathrm{Bx}+\mathrm{C}}{\left(\mathrm{x}^2+9\right)} \quad \ldots(1)\) [using partial fraction]

⇒ \(5 \mathrm{x}=\mathrm{A}\left(\mathrm{x}^2+9\right)+(\mathrm{Bx}+\mathrm{C})(\mathrm{x}+1) \Rightarrow 5 \mathrm{x}=\mathrm{Ax}^2+9 \mathrm{~A}+\mathrm{Bx}^2+\mathrm{Bx}+\mathrm{Cx}+\mathrm{C}\)

Equating the coefficients of \(\mathrm{x}^2, \mathrm{x}\), and constant term, we obtain

⇒ \(\mathrm{A}+\mathrm{B}=0, \mathrm{~B}+\mathrm{C}=5,9 \mathrm{~A}+\mathrm{C}=0\)

On solving these equations, we obtain \(\mathrm{A}=-\frac{1}{2}, \mathrm{~B}=\frac{1}{2}\), and \(\mathrm{C}=\frac{9}{2}\)

From equation (1), we obtain

⇒ \(\frac{5 x}{(x+1)\left(x^2+9\right)}=\frac{-1}{2(x+1)}+\frac{\frac{x}{2}+\frac{9}{2}}{\left(x^2+9\right)}\)

⇒ \(\int \frac{5 x}{(x+1)\left(x^2+9\right)} d x=\int\left\{\frac{-1}{2(x+1)}+\frac{(x+9)}{2\left(x^2+9\right)}\right\} d x\)

= \(-\frac{1}{2} \log |x+1|+\frac{1}{2} \int \frac{x}{x^2+9} d x+\frac{9}{2} \int \frac{1}{x^2+9} d x\)

= \(-\frac{1}{2} \log |x+1|+\frac{1}{4} \int \frac{2 x}{x^2+9} d x+\frac{9}{2} \int \frac{1}{x^2+9} d x\)

= \(-\frac{1}{2} \log |x+1|+\frac{1}{4} \log \left|x^2+9\right|+\frac{9}{2} \cdot\left(\frac{1}{3} \tan ^{-1} \frac{x}{3}\right)+C\)

(because \(\int \frac{d x}{a^2+x^2}=\frac{1}{a} \tan ^{-1}\left(\frac{x}{a}\right)+C\))

= \(-\frac{1}{2} \log |x+1|+\frac{1}{4} \log \left(x^2+9\right)+\frac{3}{2} \tan ^{-1}\left(\frac{x}{3}\right)+C\)

Question 7. \(\int \frac{\sin x}{\sin (x-a)} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\sin \mathrm{x}}{\sin (\mathrm{x}-\mathrm{a})} \mathrm{dx}\)

Put x-a=t ⇒ d x=d t

⇒ \(\int \frac{\sin x}{\sin (x-a)} d x=\int \frac{\sin (t+a)}{\sin t} d t\)

= \(\int \frac{\sin t \cos a+\cos t \sin a}{\sin t} d t=\int(\cos a+\cot t \sin a) d t\)

= \(t \cos a+\sin a \log |\sin t|+C_1=(x-a) \cos a+\sin a \log |\sin (x-a)|+C_1\)

= \(x \cos a+\sin a \log |\sin (x-a)|-a \cos a+C_1\)

= \(\sin a \log |\sin (x-a)|+x \cos a+C\) (because-\(a \cos a+C_1=C_{1}\))

Question 8. \(\int \frac{e^{5 \log x}-e^{4 \log x}}{e^{3 \log x}-e^{2 \log x}} d x\)
Solution:

Let \(I=\int \frac{e^{\log x}-e^{4 \log x}}{e^{3 \log x}-e^{2 \log x}} d x=\int \frac{e^{\log x^3}-e^{\log x^4}}{e^{\log x^2}-e^{\log x^2}} d x=\int \frac{x^5-x^4}{x^3-x^2} d x=\int \frac{x^4(x-1)}{x^2(x-1)} d x=\int x^2 d x=\frac{x^3}{3}+C\)

Question 9. \(\int \frac{\cos x}{\sqrt{4-\sin ^2 x}} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\cos \mathrm{x}}{\sqrt{4-\sin ^2 \mathrm{x}}} \mathrm{dx}\)

Put sin x = \(t \Rightarrow \cos x d x=d t\)

⇒ I = \(\int \frac{\cos x}{\sqrt{4-\sin ^2 x}} d x=\int \frac{d t}{\sqrt{(2)^2-(t)^2}}=\sin ^{-1}\left(\frac{t}{2}\right)+C=\sin ^{-1}\left(\frac{\sin x}{2}\right)+C\)

Question 10. \(\int \frac{\sin ^8 x-\cos ^8 x}{1-2 \sin ^2 x \cos ^2 x} d x\)
Solution:

Let \(I=\int \frac{\sin ^8 x-\cos ^8 x}{1-2 \sin ^2 x \cos ^2 x} d x\)

= \(\int \frac{\left(\sin ^4 x+\cos ^4 x\right)\left(\sin ^4 x-\cos ^4 x\right)}{\sin ^2 x+\cos ^2 x-\sin ^2 x \cos ^2 x-\sin ^2 x \cos ^2 x} d x\)

= \(\int \frac{\left(\sin ^4 x+\cos ^4 x\right)\left(\sin ^2 x-\cos ^2 x\right)}{\sin ^2 x\left(1-\cos ^2 x\right)+\cos ^2 x\left(1-\sin ^2 x\right)} d x\)

= \(-\int \frac{\left(\sin ^4 x+\cos ^4 x\right)\left(\cos ^2 x-\sin ^2 x\right)}{\left(\sin ^4 x+\cos ^4 x\right)} d x\)

= \(-\int \cos 2 x d x=-\frac{\sin 2 x}{2}+C\)

Question 11. \(\int \frac{1}{\cos (x+a) \cos (x+b)} d x\)
Solution:

Let \(I=\int \frac{1}{\cos (x+a) \cos (x+b)} d x\)

Multiplying and dividing by sin (a-b), we obtain, \(\int \frac{1}{\cos (x+a) \cos (x+b)} d x=\frac{1}{\sin (a-b)} \int\left[\frac{\sin (a-b)}{\cos (x+a) \cos (x+b)}\right] d x\)

= \(\frac{1}{\sin (a-b)} \int\left[\frac{\sin [(x+a)-(x+b)]}{\cos (x+a) \cos (x+b)}\right] d x\)

= \(\frac{1}{\sin (a-b)} \int\left[\frac{\sin (x+a) \cos (x+b)-\cos (x+a) \cdot \sin (x+b)}{\cos (x+a) \cos (x+b)}\right] d x\)

= \(\frac{1}{\sin (a-b)} \int\left[\frac{\sin (x+a)}{\cos (x+a)}-\frac{\sin (x+b)}{\cos (x+b)}\right] d x=\frac{1}{\sin (a-b)} \int[\tan (x+a)-\tan (x+b)] d x\)

= \(\frac{1}{\sin (a-b)}[-\log |\cos (x+a)|+\log |\cos (x+b)|]+C=\frac{1}{\sin (a-b)} \log \left|\frac{\cos (x+b)}{\cos (x+a)}\right|+C\)

Question 12. \(\int \frac{\mathrm{x}^3}{\sqrt{1-\mathrm{x}^8}} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \frac{\mathrm{x}^3}{\sqrt{1-\mathrm{x}^8}} \mathrm{dx}\)

Put \(x^4=t \Rightarrow 4 x^3 d x=d t \Rightarrow I=\int \frac{x^3}{\sqrt{1-x^8}} d x=\frac{1}{4} \int \frac{d t}{\sqrt{1-t^2}}=\frac{1}{4} \sin ^{-1} t+C=\frac{1}{4} \sin ^{-1}\left(x^4\right)+C\)

Question 13. \(\int \frac{e^x}{\left(1+e^x\right)\left(2+e^x\right)} d x\)
Solution:

Let \(I=\int \frac{e^x}{\left(1+e^x\right)\left(2+e^x\right)} d x\)

Put \(\mathrm{e}^{\mathrm{x}}=\mathrm{t} \Rightarrow \mathrm{e}^{\mathrm{x}} \mathrm{dx}=\mathrm{dt}\)

⇒ \(\int \frac{e^x}{\left(1+e^x\right)\left(2+e^x\right)} d x=\int \frac{d t}{(t+1)(t+2)}=\frac{1}{(t+1)(t+2)}=\frac{A}{t+1}+\frac{B}{t+2}=1=A(t+2)+B(t+2)\)

put t=-2, B=-1, put t=-1, A=1

I = \(\int\left[\frac{1}{(t+1)}-\frac{1}{(t+2)}\right] d t=\log |t+1|-\log |t+2|+C=\log \left|\frac{t+1}{t+2}\right|+C=\log \left|\frac{1+e^x}{2+e^x}\right|+C\)

Question 14. \(\int \frac{1}{\left(\mathrm{x}^2+1\right)\left(\mathrm{x}^2+4\right)} \mathrm{dx}\)
Solution:

Here, \(\frac{1}{\left(x^2+1\right)\left(x^2+4\right)}=\frac{1}{(y+1)(y+4)}\) (put \(x^2=y\))

⇒ \(\frac{1}{(y+1)(y+4)}=\frac{A}{y+1}+\frac{B}{y+4}\)

⇒ \(\frac{1}{(y+1)(y+4)}=\frac{A(y+4)+B(y+1)}{(y+1)(y+4)} \Rightarrow 1=A(y+4)+B(y+1)\) [By using partial fraction]

Put y=-1, then A = \(\frac{1}{3}\) and put y=-4, then \(B=-\frac{1}{3}\)

⇒ \(\frac{1}{(y+1)(y+4)}=\frac{1}{3(y+1)}-\frac{1}{3(y+4)}\)

⇒ \(\frac{1}{\left(x^2+1\right)\left(x^2+4\right)}=\frac{1}{3\left(x^2+1\right)}-\frac{1}{3\left(x^2+4\right)}\)

⇒ \(\int \frac{1}{\left(x^2+1\right)\left(x^2+4\right)} d x=\frac{1}{3} \int \frac{1}{x^2+1} d x-\frac{1}{3} \int \frac{1}{x^2+4} d x=\frac{1}{3} \tan ^{-1} x-\frac{1}{3} \cdot \frac{1}{2} \tan ^{-1} \frac{x}{2}+C\)

= \(\frac{1}{3} \tan ^{-1} x-\frac{1}{6} \tan ^{-1} \frac{x}{2}+C\)

Question 15. \(\int \cos ^3 x e^{\log \sin x} d x\)
Solution:

Let \(I=\int \cos ^3 x e^{\log \sin x} d x \Rightarrow \int \cos ^3 x e^{\log \sin x} d x=\int \cos ^3 x \sin x \hat{d x}\)

Put \(\cos x=t \Rightarrow-\sin x d x=d t \Rightarrow I=-\int t^3 \cdot d t=-\frac{t^4}{4}+C=-\frac{\cos ^4 x}{4}+C\)

Question 16. \(\int \mathrm{e}^{3 \log x}\left(\mathrm{x}^4+1\right)^{-1} \mathrm{dx}\)
Solution:

Let \(\mathrm{I}=\int \mathrm{e}^{3 \log x}\left(\mathrm{x}^4+1\right)^{-1} \mathrm{dx}\)

⇒ \(\int e^{3 \log x}\left(x^4+1\right)^{-1} d x=\int e^{\log x^3}\left(x^4+1\right)^{-1} d x=\int \frac{x^3}{\left(x^4+1\right)} d x\)

Put \(x^4+1=t \Rightarrow 4 x^3 d x=d t \Rightarrow I=\frac{1}{4} \int \frac{d t}{t}=\frac{1}{4} \log |t|+C=\frac{1}{4} \log \left|x^4+1\right|+C\)

Question 17. \(\int f^{\prime}(a x+b)[f(a x+b)]^n d x\)
Solution:

Let \(I=\int f^{\prime}(a x+b)[f(a x+b)]^n d x\)

Put \(f(a x+b)=t \Rightarrow a f^{\prime}(a x+b) d x=d t\)

⇒ \(\int f^{\prime}(a x+b)[f(a x+b)]^n d x=\frac{1}{a} \int t^n d t=\frac{1}{a}\left[\frac{t^{n+1}}{n+1}\right]+C=\frac{1}{a(n+1)}(f(a x+b))^{n+1}+C\)

Question 18. \(\int \frac{1}{\sqrt{\sin ^3 x \sin (x+\alpha)}} d x\)
Solution:

Let \(I=\int \frac{1}{\sqrt{\sin ^3 x \sin (x+\alpha)}} d x=\int \frac{1}{\sqrt{\sin ^3 x(\sin x \cos \alpha+\cos x \sin \alpha)}} d x\)

= \(\int \frac{1}{\sqrt{\sin ^4 x \cos \alpha+\sin ^3 x \cos x \sin \alpha}} d x=\int \frac{1}{\sin ^2 x \sqrt{\cos \alpha+\cot x \sin \alpha}} d x\)

= \(\int \frac{\mathrm{cosec}^2 x}{\sqrt{\cos \alpha+\cot x \sin \alpha}} d x\)

Put \(\cos \alpha+\cot x \cdot \sin \alpha=\mathrm{t} \Rightarrow-\mathrm{cosec}^2 \mathrm{x} \sin \alpha \mathrm{d} x=\mathrm{dt}\)

⇒ \(\mathrm{I}=\frac{-1}{\sin \alpha} \int \frac{\mathrm{dt}}{\sqrt{\mathrm{t}}}=\frac{-1}{\sin \alpha}[2 \sqrt{\mathrm{t}}]+\mathrm{C}=\frac{-1}{\sin \alpha}[2 \sqrt{\cos \alpha+\cot \mathrm{x} \sin \alpha}]+\mathrm{C}\)

= \(\frac{-2}{\sin \alpha} \sqrt{\cos \alpha+\frac{\cos \mathrm{x} \sin \alpha}{\sin x}}+\mathrm{C}=\frac{-2}{\sin \alpha} \sqrt{\frac{\sin \mathrm{x} \cos \alpha+\cos \mathrm{x} \sin \alpha}{\sin x}}+\mathrm{C}=\frac{-2}{\sin \alpha} \sqrt{\frac{\sin (\mathrm{x}+\alpha)}{\sin x}}+\mathrm{C}\)

Question 19. \(\int \sqrt{\frac{1-\sqrt{x}}{1+\sqrt{x}}} d x\)
Solution:

Let \(\mathrm{I}=\int \sqrt{\frac{1-\sqrt{\mathrm{x}}}{1+\sqrt{\mathrm{x}}}} d \mathrm{x}\)

put \(x=\cos ^2 \theta \Rightarrow d x=-2 \sin \theta \cos \theta d \theta\)

⇒ I = \(\int \sqrt{\frac{1-\cos \theta}{1+\cos \theta}}(-2 \sin \theta \cos \theta) d \theta=-\int \sqrt{\frac{2 \sin ^2 \frac{\theta}{2}}{2 \cos ^2 \frac{\theta}{2}}} \sin 2 \theta d \theta=-\int \tan \frac{\theta}{2} \cdot 2 \sin \theta \cos \theta d \theta\)

= \(-2 \int \frac{\sin \frac{\theta}{2}}{\cos \frac{\theta}{2}}\left(2 \sin \frac{\theta}{2} \cos \frac{\theta}{2}\right) \cos \theta d \theta=-4 \int \sin ^2 \frac{\theta}{2} \cos \theta d \theta=-4 \int \sin ^2 \frac{\theta}{2} \cdot\left(2 \cos ^2 \frac{\theta}{2}-1\right) d \theta\)

= \(-4 \int\left(2 \sin ^2 \frac{\theta}{2} \cos ^2 \frac{\theta}{2}-\sin ^2 \frac{\theta}{2}\right) d \theta=-8 \int \sin ^2 \frac{\theta}{2} \cdot \cos ^2 \frac{\theta}{2} d \theta+4 \int \sin ^2 \frac{\theta}{2} d \theta\)

= \(-2 \int \sin ^2 \theta d \theta+4 \int \sin ^2 \frac{\theta}{2} d \theta=-2 \int\left(\frac{1-\cos 2 \theta}{2}\right) d \theta+4 \int \frac{1-\cos \theta}{2} d \theta\)

= \(-2\left[\frac{\theta}{2}-\frac{\sin 2 \theta}{4}\right]+4\left[\frac{\theta}{2}-\frac{\sin \theta}{2}\right]+C=-\theta+\frac{\sin 2 \theta}{2}+2 \theta-2 \sin \theta+C\)

= \(\theta+\frac{\sin 2 \theta}{2}-2 \sin \theta+C=\theta+\frac{2 \sin \theta \cos \theta}{2}-2 \sin \theta+C\)

= \(\theta+\sqrt{1-\cos ^2 \theta} \cdot \cos \theta-2 \sqrt{1-\cos ^2 \theta}+C=\cos ^{-1} \sqrt{x}+\sqrt{1-x} \cdot \sqrt{x}-2 \sqrt{1-x}+C\)

= \(-2 \sqrt{1-x}+\cos ^{-1} \sqrt{x}+\sqrt{x(1-x)}+C=-2 \sqrt{1-x}+\cos ^{-1} \sqrt{x}+\sqrt{x-x^2}+C\)

Question 20. \(\int\left(\frac{2+\sin 2 x}{1+\cos 2 x}\right) e^x d x\)
Solution:

Let \(I=\int\left(\frac{2+\sin 2 x}{1+\cos 2 x}\right) e^x d x=\int\left(\frac{2+2 \sin x \cos x}{2 \cos ^2 x}\right) e^x d x=\int\left(\frac{1+\sin x \cos x}{\cos ^2 x}\right) e^x d x\)

= \(\int\left(\sec ^2 x+\tan x\right) e^x d x=\int\left(\tan x+\sec ^2 x\right) e^x d x\)

Let f(x) = \(\tan x \Rightarrow f^{\prime}(x)=\sec ^2 x\)

I = \(\int\left[f(x)+f^{\prime}(x)\right] e^x d x=e^x \cdot f(x)+C=e^x \tan x+C\)

Question 21. \(\int \frac{x^2+x+1}{(x+1)^2(x+2)} d x\)
Solution:

Let \(I=\int \frac{x^2+x+1}{(x+1)^2(x+2)} d x\)

⇒ \(\frac{\mathrm{x}^2+\mathrm{x}+1}{(\mathrm{x}+1)^2(\mathrm{x}+2)}=\frac{\mathrm{A}}{(\mathrm{x}+1)}+\frac{\mathrm{B}}{(\mathrm{x}+1)^2}+\frac{\mathrm{C}}{(\mathrm{x}+2)} \ldots(1)\)

⇒ \(\mathrm{x}^2+\mathrm{x}+1=\mathrm{A}(\mathrm{x}+1)(\mathrm{x}+2)+\mathrm{B}(\mathrm{x}+2)+\mathrm{C}\left(\mathrm{x}^2+2 \mathrm{x}+1\right)\)

⇒ \(\mathrm{x}^2+\mathrm{x}+1=\mathrm{A}\left(\mathrm{x}^2+3 \mathrm{x}+2\right)+\mathrm{B}(\mathrm{x}+2)+\mathrm{C}\left(\mathrm{x}^2+2 \mathrm{x}+1\right)\)

⇒ \(\mathrm{x}^2+\mathrm{x}+1=(\mathrm{A}+\mathrm{C}) \mathrm{x}^2+(3 \mathrm{~A}+\mathrm{B}+2 \mathrm{C}) \mathrm{x}+(2 \mathrm{~A}+2 \mathrm{~B}+\mathrm{C})\) (Using partial fraction)

Equating the coefficients of \(\mathrm{x}^2, \mathrm{x}\), and constant term, we obtain \(\mathrm{A}+\mathrm{C}=1,3 \mathrm{~A}+\mathrm{B}+2 \mathrm{C}=1,2 \mathrm{~A}+2 \mathrm{~B}+\mathrm{C}=1\)

On solving these equations, we obtain A=-2, B=1, and C=3

From equation (1), we obtain \(\frac{x^2+x+1}{(x+1)^2(x+2)}=\frac{-2}{(x+1)}+\frac{1}{(x+1)^2}+\frac{3}{(x+2)}\)

⇒ \(\int \frac{x^2+x+1}{(x+1)^2(x+2)} d x=-2 \int \frac{1}{x+1} d x+\int \frac{1}{(x+1)^2} d x+3 \int \frac{1}{(x+2)} d x\)

= \(-2 \log |x+1|-\frac{1}{(x+1)}+3 \log |x+2|+C\)

Question 22. \(\int \tan ^{-1} \sqrt{\frac{1-\mathrm{x}}{1+\mathrm{x}}} \mathrm{dx}\)
Solution:

Let \(I=\tan ^{-1} \sqrt{\frac{1-x}{1+x}} d x\)

Put \(x=\cos \theta \Rightarrow d x=-\sin \theta d \theta\)

I = \(\int \tan ^{-1} \sqrt{\frac{1-\cos \theta}{1+\cos \theta}}(-\sin \theta d \theta)=-\int \tan ^{-1} \sqrt{\frac{2 \sin ^2 \frac{\theta}{2}}{2 \cos ^2 \frac{\theta}{2}}} \sin \theta d \theta=-\int \tan ^{-1} \tan \frac{\theta}{2} \cdot \sin \theta d \theta\)

= \(-\frac{1}{2} \int \theta \cdot \sin \theta d \theta=-\frac{1}{2}\left[\theta \cdot(-\cos \theta)-\int 1 \cdot(-\cos \theta) d \theta\right]+C=-\frac{1}{2}[-\theta \cos \theta+\sin \theta]+C\)

= \(+\frac{1}{2} \theta \cos \theta-\frac{1}{2} \sin \theta+C=\frac{1}{2} \cos ^{-1} x \cdot x-\frac{1}{2} \sqrt{1-x^2}+C=\frac{x}{2} \cos ^{-1} x-\frac{1}{2} \sqrt{1-x^2}+C\)

= \(\frac{1}{2}\left(x \cos ^{-1} x-\sqrt{1-x^2}\right)+C\)

Question 23. \(\int \frac{\sqrt{x^2+1} \log \left(x^2+1\right)-2 \log x}{x^4} d x\)
Solution:

Let \(\mathrm{I}=\int \frac{\sqrt{\mathrm{x}^2+1} \log \left(\mathrm{x}^2+1\right)-2 \log \mathrm{x}}{\mathrm{x}^4} \mathrm{dx}\)

⇒ \(\int \frac{\sqrt{x^2+1} \log \left(x^2+1\right)-2 \log x}{x^4} d x=\int \frac{\sqrt{x^2+1}}{x^4}\left[\log \left(x^2+1\right)-\log x^2\right] d x\)

= \(\int \frac{\sqrt{x^2+1}}{x^4}\left[\log \left(\frac{x^2+1}{x^2}\right)\right]=\int \frac{1}{x^3} \sqrt{\frac{x^2+1}{x^2}} \log \left(1+\frac{1}{x^2}\right) d x=\int \frac{1}{x^3} \sqrt{1+\frac{1}{x^2}} \log \left(1+\frac{1}{x^2}\right) d x\)

Put \(1+\frac{1}{x^2}=t \Rightarrow \frac{-2}{x^3} d x=d t \Rightarrow I=-\frac{1}{2} \int \sqrt{t} \log t d t=-\frac{1}{2} \int t^{\frac{1}{2}}+\log t d t\)

= \(-\frac{1}{2}[\log \mathrm{t} \cdot \frac{\mathrm{t}^{\frac{3}{2}}}{\frac{3}{2}}-\int \frac{1}{\mathrm{t}} \cdot \frac{\mathrm{t}^{\frac{3}{2}}}{\frac{3}{2}} \mathrm{dt}\) (Integrating by parts, we obtain)

= \(-\frac{1}{2}\left[\frac{2}{3} \mathrm{t}^{\frac{3}{2}} \log \mathrm{t}-\frac{2}{3} \int \mathrm{t}^{\frac{1}{2}} \mathrm{dt}\right]=-\frac{1}{2}\left[\frac{2}{3} \mathrm{t}^{\frac{3}{2}} \log \mathrm{t}-\frac{4}{9} \mathrm{t}^{\frac{3}{2}}\right]+\mathrm{C}=-\frac{1}{3} \mathrm{t}^{\frac{3}{2}} \log \mathrm{t}+\frac{2}{9} \mathrm{t}^{\frac{3}{2}}+\mathrm{C}\)

= \(-\frac{1}{3} \mathrm{t}^{\frac{3}{2}}\left[\log \mathrm{t}-\frac{2}{3}\right]+\mathrm{C}=-\frac{1}{3}\left(1+\frac{1}{\mathrm{x}^2}\right)^{\frac{3}{2}}\left[\log \left(1+\frac{1}{\mathrm{x}^2}\right)-\frac{2}{3}\right]+\mathrm{C}\)

Question 24. \(\int_{\frac{\pi}{2}}^\pi e^x\left(\frac{1-\sin x}{1-\cos x}\right) d x\)
Solution:

Let \(I=\int_{\frac{\pi}{2}}^\pi e^x\left(\frac{1-\sin x}{1-\cos x}\right) d x \Rightarrow I=\int_{\frac{\pi}{2}}^\pi e^x\left(\frac{1-2 \sin \frac{x}{2} \cos \frac{x}{2}}{2 \sin ^2 \frac{x}{2}}\right) d x\)

I = \(\int_{\frac{\pi}{2}}^\pi e^x\left(\frac{\mathrm{cosec}^2 \frac{x}{2}}{2}-\cot \frac{x}{2}\right) d x\)

Let f(x) = \(-\cot \frac{x}{2} \Rightarrow f^{\prime}(x)=-\left(-\frac{1}{2} \mathrm{cosec}^2 \frac{x}{2}\right)=\frac{1}{2} \mathrm{cosec}^2 \frac{x}{2}\)

I = \(\int_{\frac{\pi}{2}}^\pi e^x\left[f(x)+f^{\prime}(x)\right] d x=\left[e^x \cdot f(x) d x\right]_{\frac{\pi}{2}}^x=-\left[e^x \cdot \cot \frac{x}{2}\right]_{\frac{\pi}{2}}^\pi \)

= \(-\left[e^\pi \times \cot \frac{\pi}{2}-e^{\frac{\pi}{2}} \times \cot \frac{\pi}{4}\right]=-\left[e^x \times 0-e^{\frac{\pi}{2}} \times 1\right]=e^{\frac{\pi}{2}}\)

Question 25. \(\int_0^{\frac{\pi}{4}} \frac{\sin x \cos x}{\cos ^4 x+\sin ^4 x} d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{4}} \frac{\sin x \cos x}{\cos ^4 x+\sin ^4 x} d x \Rightarrow I=\int_0^{\frac{\pi}{4}} \frac{\frac{\sin x \cos x}{\cos ^4 x}}{\frac{\left(\cos ^4 x+\sin ^4 x\right)}{\cos ^4 x}} d x\) (Nr. and Dr. divided by \(\cos ^4 x\))

⇒ \(\mathrm{I}=\int_0^{\frac{\pi}{4}} \frac{\tan x \sec ^2 \mathrm{x}}{1+\tan ^4 \mathrm{x}} \mathrm{dx}\)

Put \(\tan ^2 x=t \Rightarrow 2 \tan x \sec ^2 x d x=d t\)

When x=0, t=0 and when \(x=\frac{\pi}{4}, t=1\)

⇒ \(\mathrm{I}=\frac{1}{2} \int_0^1 \frac{\mathrm{dt}}{1+\mathrm{t}^2}=\frac{1}{2}\left[\tan ^{-1} \mathrm{t}\right]_0^1=\frac{1}{2}\left[\tan ^{-1} 1-\tan ^{-1} 0\right]=\frac{1}{2}\left[\frac{\pi}{4}\right]=\frac{\pi}{8}\)

Question 26. \(\int_0^{\frac{\pi}{2}} \frac{\cos ^2 x}{\cos ^2 x+4 \sin ^2 x} d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{2}} \frac{\cos ^2 x}{\cos ^2 x+4 \sin ^2 x} d x\) (Nr. and Dr. divided by \(\cos ^2 x\))

I = \(\int_0^{\frac{\pi}{2}} \frac{d x}{1+4 \tan ^2 x} \Rightarrow I=\int_0^{\frac{\pi}{2}} \frac{\sec ^2 x d x}{\sec ^2 x\left(1+4 \tan ^2 x\right)}\)(multiplying and divide by \(\sec ^2 x\))

I = \(\int_0^{\frac{\pi}{2}} \frac{\sec ^2 x d x}{\left(1+\tan ^2 x\right)\left(1+4 \tan ^2 x\right)}\)

Put \(\tan x=t \Rightarrow \sec ^2 x d x=d t\)

when x=0, then t=0, when \(x=\frac{\pi}{2}\), then t=\(\infty\)

I \(=\int_0^{\infty} \frac{d t}{\left(1+t^2\right)\left(1+4 t^2\right)}\) (put } \(t^2=y\)

= \(\int_0^{\infty} \frac{d t}{(1+y)(1+4 y)} \Rightarrow \frac{1}{(1+y)(1+4 y)}=\frac{A}{1+y}+\frac{B}{1+4 y}\)

= \(\frac{1}{(1+y)(1+4 y)}=\frac{A(1+4 y)+B(1+y)}{(1+y)(1+4 y)}\)

I = \(A(1+4 y)+B(1+y)\) (By using partial fraction)

Put y=-1, then A = \(-\frac{1}{3}\)

Put \(\mathrm{y}=-\frac{1}{4}\), then \(\mathrm{B}=\frac{4}{3}\)

⇒ \(\mathrm{I}=-\frac{1}{3} \int_0^{\infty} \frac{\mathrm{dt}}{1+\mathrm{t}^2}+\frac{4}{3} \int_0^{\infty} \frac{\mathrm{dt}}{1+4 \mathrm{t}^2}=-\frac{1}{3}\left[\tan ^{-1} \mathrm{t}\right]_0^{\infty}+\frac{4}{3} \int_0^{\infty} \frac{\mathrm{dt}}{1+(2 \mathrm{t})^2}\)

= \(-\frac{1}{3}\left[\tan ^{-1} \infty-\tan ^{-1} 0\right]+\frac{4}{3} \times \frac{1}{2}\left[\tan ^{-1} 2 \mathrm{t}\right]_0^{\infty}=-\frac{1}{3}\left[\frac{\pi}{2}-0\right]+\frac{2}{3}\left[\tan ^{-1} \infty-\tan ^{-1} 0\right]\)

= \(-\frac{\pi}{6}+\frac{2}{3} \cdot \frac{\pi}{2}=-\frac{\pi}{6}+\frac{\pi}{3}=\frac{\pi}{6}\)

Question 27. \(\int_{\frac{\pi}{6}}^{\frac{\pi}{3}} \frac{\sin x+\cos x}{\sqrt{\sin 2 x}} d x\)
Solution:

Let \(I=\int_{\frac{\pi}{6}}^{\frac{\pi}{3}} \frac{\sin x+\cos x}{\sqrt{\sin 2 x}} d x=\int_{\frac{\pi}{6}}^{\frac{\pi}{3}} \frac{\sin x+\cos x}{\sqrt{1+(\sin 2 x-1)}} d x=\int_{\frac{\pi}{6}}^{\frac{\pi}{3}} \frac{\sin x+\cos x}{\sqrt{1-(1-\sin 2 x)}} d x\)

I = \(\int_{\frac{\pi}{6}}^{\frac{\pi}{3}} \frac{(\sin x+\cos x) d x}{\sqrt{1-(\sin x-\cos x)^2}}\)

Put \((\sin \mathrm{x}-\cos \mathrm{x})=\mathrm{t} \Rightarrow(\sin \mathrm{x}+\cos \mathrm{x}) \mathrm{dx}=\mathrm{dt}\)

When \(x=\frac{\pi}{6}, t=\left(\frac{1-\sqrt{3}}{2}\right)\) and when \(x=\frac{\pi}{3}, t=\left(\frac{\sqrt{3}-1}{2}\right)\)

I = \(\int_{\frac{1-\sqrt{3}}{2}}^{\frac{\sqrt{3}-1}{2}} \frac{d t}{\sqrt{1-t^2}} \Rightarrow I=\int_{-\left(\frac{\sqrt{3}-1}{2}\right)}^{\frac{\sqrt{3}-1}{2}} \frac{d t}{\sqrt{1-t^2}}\)

As \(\frac{1}{\sqrt{1-(-t)^2}}=\frac{1}{\sqrt{1-t^2}}\), therefore, \(\frac{1}{\sqrt{1-t^2}}\) is an even function.

It is known that if f(x) is an even function, then \(\int_{-a}^a f(x) d x=2 \int_0^a f(x) d x\)

⇒ \(\mathrm{I}=2 \int_0^{\frac{\sqrt{3}-1}{2}} \frac{\mathrm{dt}}{\sqrt{1-\mathrm{t}^2}}=\left[2 \sin ^{-1} \mathrm{t}\right]_0^{\frac{\sqrt{3}-1}{2}}=2 \sin ^{-1}\left(\frac{\sqrt{3}-1}{2}\right)\)

Question 28. \(\int_0^1 \frac{d x}{\sqrt{1+x}-\sqrt{x}}\)
Solution:

Let \(I=\int_0^1 \frac{d x}{\sqrt{1+x}-\sqrt{x}}\)

I = \(\int_0^1 \frac{d x}{\sqrt{1+x}-\sqrt{x}} \times \frac{(\sqrt{1+x}+\sqrt{x})}{(\sqrt{1+x}+\sqrt{x})} d x=\int_0^1 \frac{\sqrt{1+x}+\sqrt{x}}{1+x-x} d x=\int_0^1 \sqrt{1+x} d x+\int_0^1 \sqrt{x} d x\)

= \(\left[\frac{2}{3}(1+x)^{\frac{3}{2}}\right]_0^1+\left[\frac{2}{3}(x)^{\frac{3}{2}}\right]_0^1=\frac{2}{3}\left[(2)^{\frac{3}{2}}-1\right]+\frac{2}{3}[1]=\frac{2}{3}(2)^{\frac{3}{2}}=\frac{2 \cdot 2 \sqrt{2}}{3}=\frac{4 \sqrt{2}}{3}\)

Question 29. \(\int_0^{\frac{\pi}{4}} \frac{\sin x+\cos x}{9+16 \sin 2 x} d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{4}} \frac{\sin x+\cos x}{9+16 \sin 2 x} d x\)

Put \(\sin x-\cos x=t \Rightarrow(\cos x+\sin x) d x=d t\)

When \(\mathrm{x}=0, \mathrm{t}=-1\) and when \(\mathrm{x}=\frac{\pi}{4}, \mathrm{t}=0\)

⇒ \((\sin x-\cos x)^2=t^2 \Rightarrow \sin ^2 x+\cos ^2 x-2 \sin x \cos x=t^2\)

⇒ \(1-\sin 2 x=t^2 \Rightarrow \sin 2 x=1-t^2\)

∴ \(I=\int_{-1}^0 \frac{d t}{9+16\left(1-t^2\right)}=\int_{-1}^0 \frac{d t}{9+16-16 t^2}=\int_{-1}^0 \frac{d t}{25-16 t^2}=\int_{-1}^0 \frac{d t}{(5)^2-(4 t)^2}\)

= \(\frac{1}{4}\left[\frac{1}{2(5)} \log \left|\frac{5+4 t}{5-4 t}\right|\right]_{-1}^0=\frac{1}{40}\left[\log (1)-\log \left|\frac{1}{9}\right|\right]=\frac{1}{40} \log 9\)

Question 30. \(\int_0^{\frac{\pi}{2}} \sin 2 x \tan ^{-1}(\sin x) d x\)
Solution:

Let \(I=\int_0^{\frac{\pi}{2}} \sin 2 x \tan ^{-1}(\sin x) d x=\int_0^{\frac{\pi}{2}} 2 \sin x \cos x \tan ^{-1}(\sin x) d x\)

Put \(\sin \mathrm{x}=\mathrm{t} \Rightarrow \cos \mathrm{x} d \mathrm{x}=\mathrm{dt}\)

When x=0, t=0 and when \(x=\frac{\pi}{2}, t=1\)

⇒ \(\mathrm{I}=2 \int_0^{\mathrm{t}} \mathrm{t} \tan ^{-1}(\mathrm{t}) \mathrm{dt}\)

Consider \(\int \mathrm{t} \cdot \tan ^{-1} \mathrm{t} d \mathrm{t}=\tan ^{-1} \mathrm{t} \cdot \int \mathrm{t} \mathrm{dt}-\int\left\{\frac{\mathrm{d}}{\mathrm{dt}}\left(\tan ^{-1} \mathrm{t}\right) \int \mathrm{t} d \mathrm{t}\right\} \mathrm{dt}\)

= \(\tan ^{-1} \mathrm{t} \cdot \frac{\mathrm{t}^2}{2}-\int \frac{1}{1+\mathrm{t}^2} \cdot \frac{\mathrm{t}^2}{2} \mathrm{dt}=\frac{\mathrm{t}^2 \tan ^{-1} \mathrm{t}}{2}-\frac{1}{2} \int \frac{\mathrm{t}^2+1-1}{1+\mathrm{t}^2} \mathrm{dt}=\frac{\mathrm{t}^2 \tan ^{-1} \mathrm{t}}{2}-\frac{1}{2} \int 1 \mathrm{dt}+\frac{1}{2} \int \frac{1}{1+\mathrm{t}^2} \mathrm{dt}\)

= \(\frac{t^2 \tan ^{-1} t}{2}-\frac{1}{2} \cdot t+\frac{1}{2} \tan ^{-1} t\)

⇒ \(\int_0^1 \mathrm{t} \cdot \tan ^{-1} \mathrm{tdt}=\left[\frac{\mathrm{t}^2 \cdot \tan ^{-1} \mathrm{t}}{2}-\frac{\mathrm{t}}{2}+\frac{1}{2} \tan ^{-1} \mathrm{t}\right]_0^1=\frac{1}{2}\left[\frac{\pi}{4}-1+\frac{\pi}{4}\right]\)

= \(\frac{1}{2}\left[\frac{\pi}{2}-1\right]=\frac{\pi}{4}-\frac{1}{2}\)

From equation (1) and (2). We obtain: \(\mathrm{I}=2\left[\frac{\pi}{4}-\frac{1}{2}\right]=\frac{\pi}{2}-1\)

Question 31. \(\int_1^4[|x-1|+|x-2|+|x-3|] d x\)
Solution:

Let \(\mathrm{I}=\int_1^4[|\mathrm{x}-1|+|\mathrm{x}-2|+|\mathrm{x}-3|] \mathrm{dx}\)

= \(\int_1^2[(x-1)-(x-2)-(x-3)] d x+\int_2^3[(x-1)+(x-2)-(x-3)] d x\)

+ \(\int_3^4[(x-1)+(x-2)+(x-3)] d x\)

= \(\int_1^2(-x+4) d x+\int_2^3(x) d x+\int_3^4(3 x-6) d x\)

= \(\left[-\frac{x^2}{2}+4 x\right]_1^2+\left[\frac{x^2}{2}\right]_2^3+\left[\frac{3 x^2}{2}-6 x\right]_3^4\)

= \(\left[\left(-\frac{4}{2}+8\right)-\left(-\frac{1}{2}+4\right)\right]+\frac{1}{2}\left[3^2-2^2\right]+\left[\left(\frac{3 \times 16}{2}-6 \times 4\right)-\left(3 \times \frac{9}{2}-6 \times 3\right)\right]\)

= \(\left[6-\frac{7}{2}\right]+\frac{1}{2} \times 5+\left[(24-24)-\left(\frac{27}{2}-18\right)\right]=\frac{5}{2}+\frac{5}{2}+\frac{9}{2}=\frac{19}{2}\)

Question 32. Prove that: \(\int_1^3 \frac{\mathrm{dx}}{\mathrm{x}^2(\mathrm{x}+1)}=\frac{2}{3}+\log \frac{2}{3}\)
Solution:

Let \(I=\int_1^3 \frac{d x}{x^2(x+1)}\)

Let \(\frac{1}{x^2(x+1)}=\frac{A}{x}+\frac{B}{x^2}+\frac{C}{x+1}\)…..(1) (using partial fraction)

I = \(\mathrm{Ax}(\mathrm{x}+1)+\mathrm{B}(\mathrm{x}+1)+\mathrm{C}\left(\mathrm{x}^2\right) \Rightarrow 1=\mathrm{Ax}^2+\mathrm{Ax}+\mathrm{Bx}+\mathrm{B}+\mathrm{Cx}^2\)

Equating the coefficients of \(x^2, x\), and constant term, we obtain \(\mathrm{A}+\mathrm{C}=0, \mathrm{~A}+\mathrm{B}=0, \mathrm{~B}=1\)

On solving these equations, we obtain \(\mathrm{A}=-1, \mathrm{C}=1\), and \(\mathrm{B}=1\)

∴ \(\frac{1}{x^2(x+1)}=\frac{-1}{x}+\frac{1}{x^2}+\frac{1}{(x+1)}\) (from (1))

⇒ \(I=\int_1^3\left\{-\frac{1}{x}+\frac{1}{x^2}+\frac{1}{(x+1)}\right\} d x=\left[-\log x-\frac{1}{x}+\log (x+1)\right]_1^3=\left[\log \left(\frac{x+1}{x}\right)-\frac{1}{x}\right]_1^3\)

= \(\log \left(\frac{4}{3}\right)-\frac{1}{3}-\log \left(\frac{2}{1}\right)+1=\log 4-\log 3-\log 2+\frac{2}{3}=\log 2-\log 3+\frac{2}{3}=\log \left(\frac{2}{3}\right)+\frac{2}{3}\)

Hence, the given result is proved.

Question 33. Prove that: \(\int_0^1 x e^x d x=1\)
Solution:

Let \(\mathrm{I}=\int_0^1 \mathrm{xe}^{\mathrm{x}} \mathrm{dx}\)

Integrating by parts, we obtain

I = \(\left[x \int e^x d x\right]_0^1-\int_0^1\left\{\left(\frac{d}{d x}(x)\right) \int e^x d x\right\} d x=\left[x e^x\right]_0^1-\int_0^1 e^x d x=\left[x e^x\right]_0^1-\left[e^x\right]_0^1\)

= \(\mathrm{e}-\mathrm{e}+1=1\)

Hence, the given result is proved.

Question 34. Prove that: \(\int_{-1}^1 x^{17} \cos ^4 x d x=0\)
Solution:

Let \(\mathrm{I}=\int_{-1}^1 \mathrm{x}^{17} \cos ^4 \mathrm{x} d \mathrm{x}\)

Also, let \(f(x)=x^{17} \cos ^4 x \Rightarrow f(-x)=(-x)^{17} \cos ^4(-x)=-x^{17} \cos ^4 x=-f(x)\)

Therefore, f(x) is an odd function.

It is known that if f(x) is an odd function, then \(\int_{-a}^a f(x) d x=0\)

∴ \(\mathrm{I}=\int_{-1}^1 \mathrm{x}^{17} \cos ^4 \mathrm{x} \mathrm{dx}=0\)

Hence, the given result is proved.

Question 35. Prove that: \(\int_0^{\frac{\pi}{2}} \sin ^3 x d x=\frac{2}{3}\)
Solution:

Let \(\mathrm{I}=\int_0^{\frac{\pi}{2}} \sin ^3 \mathrm{xdx}\)

⇒ \(\mathrm{I}=\int_0^{\frac{\pi}{2}} \sin ^2 x \cdot \sin x d x=\int_0^{\frac{\pi}{2}}\left(1-\cos ^2 x\right) \sin x d x=\int_0^{\frac{\pi}{2}} \sin x d x-\int_0^{\frac{\pi}{2}} \cos ^2 x \cdot \sin x d x\)

= \([-\cos x]_0^{\frac{\pi}{2}}+\left[\frac{\cos ^3 x}{3}\right]_0^{\frac{\pi}{2}}=1+\frac{1}{3}[-1]=1-\frac{1}{3}=\frac{2}{3}\)

Hence, the given result is proved.

Question 36. Prove that: \(\int_0^{\frac{\pi}{4}} 2 \tan ^3 x d x=1-\log 2\)
Solution:

Let \(I=\int_0^{\frac{\pi}{4}} 2 \tan ^3 x d x\)

I = \(2 \int_0^{\frac{\pi}{4}} \tan ^2 x \tan x d x=2 \int_0^{\frac{\pi}{4}}\left(\sec ^2 x-1\right) \tan x d x=2 \int_0^{\frac{\pi}{4}} \sec ^2 x \tan x d x-2 \int_0^{\frac{\pi}{4}} \tan x d x\)

= \(2\left[\frac{\tan ^2 x}{2}\right]_0^{\frac{\pi}{4}}+2[\log \cos x]_0^{\frac{\pi}{4}} \quad\left[\tan ^2 \frac{\pi}{4}-\tan ^2 0\right]+2\left[\log \cos \frac{\pi}{4}-\log \cos 0\right]\)

= \(1+2\left[\log \frac{1}{\sqrt{2}}-\log 1\right]=1-2 \log \sqrt{2}-2 \log 1=1-\frac{2}{2} \log 2-0\)

= \(1-\log 2=1-\log 2\)

Hence, the given result is proved.

Question 37. Prove that: \(\int_0^1 \sin ^{-1} x d x=\frac{\pi}{2}-1\)
Solution:

Let \(\mathrm{I}=\int_0^1 \sin ^{-1} \mathrm{x} d x \Rightarrow \int_0^1 \sin ^{-1} \mathrm{x} \cdot 1 \mathrm{dx}\)

Integrating by parts, we obtain \(1=\left[\sin ^{-1} x \cdot x\right]_0^1-\int_0^1 \frac{1}{\sqrt{1-x^2}} \cdot x d x=\left[x \sin ^{-1} x\right]_0^1+\frac{1}{2} \int_0^1 \frac{(-2 x)}{\sqrt{1-x^2}} d x\)

Put \(1-x^2=t \Rightarrow-2 x d x=d t\); When x=0, t=1 and when x=1, t=0

I = \(\left[x \sin ^{-1} x\right]_0^1+\frac{1}{2} \int_1^0 \frac{d t}{\sqrt{t}}=\left[x \sin ^{-1} x\right]_0^1+\frac{1}{2}[2 \sqrt{t}]_1^0=\sin ^{-1}(1)+[-\sqrt{1}]=\frac{\pi}{2}-1\)

Hence, the given result is proved.

Choose The Correct Answers

Question 38. \(\int \frac{\mathrm{dx}}{\mathrm{e}^{\mathrm{x}}+\mathrm{e}^{-\mathrm{x}}}\) is equal to ?

1. \(\tan ^{-1}\left(e^x\right)+C\)
2. \(\tan ^{-1}\left(e^{-x}\right)+C\)
3. \(\log \left(e^x-e^{-x}\right)+C\)
4. \(\log \left(e^x+e^{-x}\right)+C\)

Solution: 1. \(\tan ^{-1}\left(e^x\right)+C\)

Let \(I=\int \frac{d x}{e^x+e^{-x}} d x=\int \frac{e^x}{e^{2 x}+1} d x\)

Put \(\mathrm{e}^{\mathrm{x}}=\mathrm{t} \Rightarrow \mathrm{e}^{\mathrm{x}} d \mathrm{x}=\mathrm{dt}\)

I = \(\int \frac{d t}{1+t^2}=\tan ^{-1} t+C=\tan ^{-1}\left(e^x\right)+C\)

Hence, the correct Answer is 1.

Question 39. \(\int \frac{\cos 2 x}{(\sin x+\cos x)^2} d x\) is equal to ?

1. \(\frac{-1}{\sin x+\cos x}+C\)
2. \(\log |\sin x+\cos x|+C\)
3. \(\log |\sin x-\cos x|+C\)
4. \(\frac{1}{(\sin x+\cos x)^2}\)

Solution:

Let I = \(\frac{\cos 2 x}{(\cos x+\sin x)^2}\)

I = \(\int \frac{\cos ^2 x-\sin ^2 x}{(\cos x+\sin x)^2} d x=\int \frac{(\cos x+\sin x)(\cos x-\sin x)}{(\cos x+\sin x)^2} d x=\int \frac{\cos x-\sin x}{\cos x+\sin x} d x\)

Put \(\cos \mathrm{x}+\sin \mathrm{x}=\mathrm{t} \Rightarrow(\cos \mathrm{x}-\sin \mathrm{x}) \mathrm{dx}=\mathrm{dt}\)

⇒ \(\mathrm{I}=\int \frac{\mathrm{dt}}{\mathrm{t}}=\log |\mathrm{t}|+\mathrm{C}=\log |\cos \mathrm{x}+\sin \mathrm{x}|+\mathrm{C}\)

Hence, the correct answer is (2).

Question 40. If f(a+b-x)=f(x), then \(\int_a^b x f(x) d x\) is equal to ?

1. \(\frac{a+b}{2} \int_a^b f(b-x) d x\)
2. \(\frac{a+b}{2} \int_a^b f(b+x) d x\)
3. \(\frac{b-a}{2} \int_a^b f(x) d x\)
4. \(\frac{a+b}{2} \int_a^b f(x) d x\)

Solution: Let \(I=\int_a^b x f(x) d x\)

I = \(\int_a^b(a+b-x) f(a+b-x) d x \quad\left(\int_a^b f(x) d x=\int_a^b f(a+b-x) d x\right)\)

⇒ \(I=\int_a^b(a+b-x) f(x) d x \Rightarrow I=(a+b) \int_a^b f(x) d x-I\)

⇒ \(I+I=(a+b) \int_a^b f(x) d x \Rightarrow 2 I=(a+b) \int_a^b f(x) d x\)

⇒ \(\mathrm{I}=\left(\frac{\mathrm{a}+\mathrm{b}}{2}\right) \int_{\mathrm{a}}^{\mathrm{b}} \mathrm{f}(\mathrm{x}) \mathrm{dx}\)

Hence, the correct answer is (4).