Class 10 Maths Some Applications of Trigonometry
1. The length of the Shadow of a vertical pole is \(\frac{1}{\sqrt{3}}\) times its height. Show that the angle of elevation of the Sun is 60°.
Solution:
Let PQ be a vertical pole whose height is h. Its Shadow is OQ whose height is \(\frac{h}{\sqrt{3}}\)
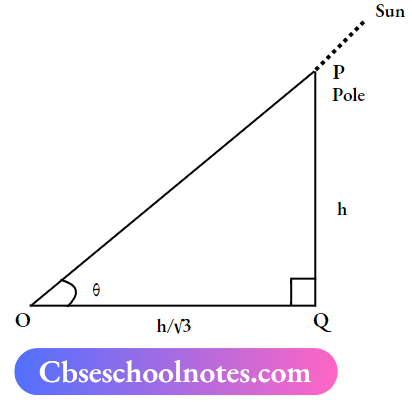
Let the angle of elevation of the Sun is ∠POQ = 0
In APOQ,
⇒ \(\tan \theta=\frac{P Q}{O Q}=\frac{h}{h / \sqrt{3}}=\sqrt{3}=\tan 60^{\circ}\)
θ = 60°
∴ The angle of elevation of Sun = 60°
Read and Learn More Class 10 Maths
2. If a tower 30m high, casts a shadow \(10 \sqrt{3} \mathrm{~m}\) long on the ground, then what is the angle of elevation of the Sun?
Solution:
It is given that AB = 30m be the tower and BC = \(10 \sqrt{3} \mathrm{~m}\) m be its shadow on the ground.
Let θ be the angle of elevation.
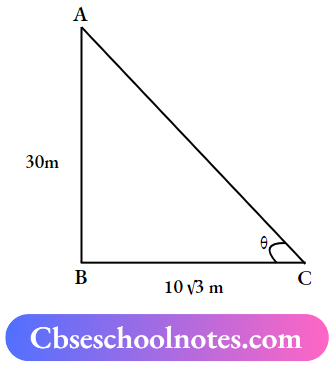
In a right triangle,
tan θ = \(\frac{AB}{BC}\)
⇒ \(\frac{30}{10 \sqrt{3}}=\frac{3}{\sqrt{3}}=\sqrt{3}\)
= tan 60°
θ = 60°
∴ Hence, the angle of elevation θ = 60°
3. A ladder 15 meters long just reaches the top of a vertical wall. If the ladder makes an angle of 60° with the wall, find the height of the wall.
Solution:
Let PR be a ladder of length 15m and QR, a wall of height h.
Given that ∠PRQ = 60°
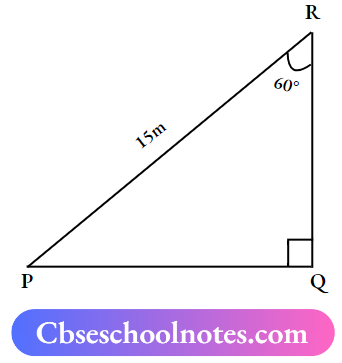
In ΔPQR,
Cos 60° = \(\frac{h}{PR}\) = \(\frac{1}{2}\) = \(\frac{h}{15}\)
⇒ h = \(\frac{15}{2}\)m
∴ Height of the wall = \(\frac{15}{2} m\)
4. A Circus artist is climbing a 20m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the Pole, if the angle made by the rope with the ground level is 30°.
Solution:
In ΔABC,
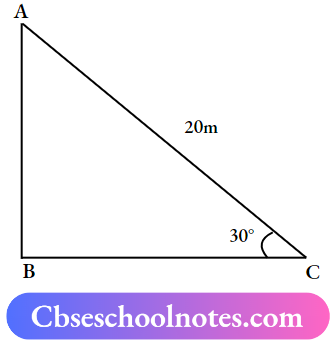
Sin 30°= \(\frac{A B}{A C}\)
⇒ \(\frac{1}{2}=\frac{A B}{20}\)
AB = 10
∴ Height of pole = 10m
5. A tree breaks due to stom and the broken part bends so that the top of the tree touches the ground making an angle 30° with it. The distance between the foot of the tree to the point where the top touches the ground is 8m. Find the height of the tree.
Solution:
Let the part CD of the tree BD broken in air and touches the ground at point A.
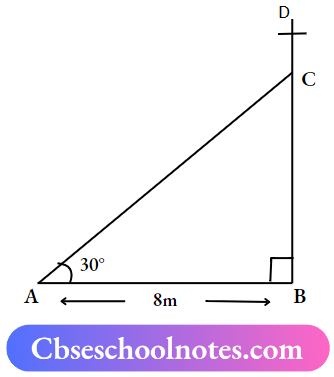
According to the problem,
AB = 8M
and ∠BAC = 30°
In ΔABC,
tan 30° = \(\frac{BC}{AB}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{B C}{8}\)
⇒ \(B=\frac{8}{\sqrt{3}} \mathrm{~m}\)
and Cos 30° = \(\frac{A B}{A C} \Rightarrow \frac{\sqrt{3}}{2}=\frac{8}{A C}\)
AC = \(\frac{16}{\sqrt{3}} m\)
CD = \(\frac{16}{\sqrt{3}} m\) (∵ AC = CD)
Now, the height of tree = BC + CD
⇒ \(\frac{8}{\sqrt{3}}+\frac{16}{\sqrt{3}}=\frac{24}{\sqrt{3}}=8 \sqrt{3} \mathrm{~m}\)
6. The angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30°: Find the height of the tower.
Solution:
Let AB be the tower.
The angle of elevation of the top of the tower from Point C, 30m away from A is 30°
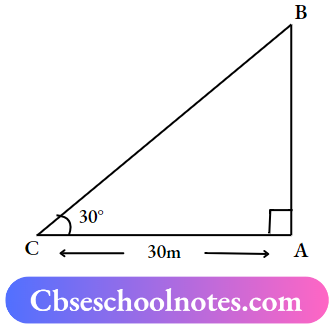
∴ In ABAC,
tan 30°= \(\frac{A B}{A C}\)
⇒ \(\frac{1}{\sqrt{3}}=\frac{A B}{A C}\)
AB = \(\frac{30}{\sqrt{3}}=10 \sqrt{3} \mathrm{~m}\)
∴ Height of the tower = \(10 \sqrt{3} \mathrm{~m}\)
7. From a point on the ground, the angles of elevation of the bottom and the top of a transmission tower fixed at the top of a 20m high building and 60° respectively. Find the height of the tower.
Solution:
Let, CD be the height of the transmission tower.
Here, the height of the building
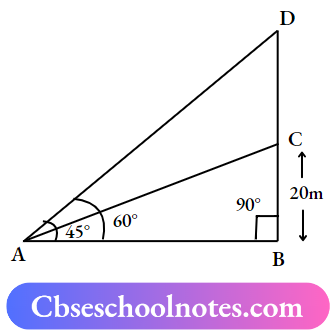
BC = 20m
In ΔABC,
tan 45° = \(\frac{B C}{A B}\)
1 = \(\frac{20}{A B}\)
AB = 20m
In ΔABD,
tan 60° = \(\frac{B D}{A B} \Rightarrow \sqrt{3}=\frac{B D}{20}\)
⇒ \(B D=20 \sqrt{3} \mathrm{~m}\)
⇒ \(B C+C D=20 \sqrt{3}\)
⇒ \(20+C D=20 \sqrt{3}\)
⇒ \(C D=20(\sqrt{3}-1) m\)
∴ Height of transmission tower = \(20(\sqrt{3}-1) m\)
8. The Shadow of a tower Standing on a level plane is found to be much longer when the Sun’s elevation is 30° than when it is 60°. Find the height of the tower.
Solution:
Let AB be a tower of height ‘h’ meters and BD and BC be its shadows when the angles of elevation of the sun are 30° and 60° respectively.
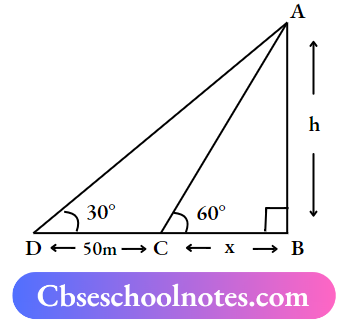
∴ ∠ADB =30°, ∠ACB = 60° and CD = 50m
Let BC = X meters.
In ΔABC
tan 60° = \(\frac{A B}{B C} \Rightarrow \sqrt{3}=\frac{h}{x}\)
⇒ \(x=\frac{h}{\sqrt{3}}\)
In ΔABD
tan 30° = \(\frac{A B}{B D} \Rightarrow \frac{1}{\sqrt{3}}=\frac{h}{x+50}\)
⇒ \(\sqrt{3} h=x+50 \Rightarrow \sqrt{3} h=\frac{h}{\sqrt{3}}+50\)
2h= \(50 \sqrt{3}\)
h = \(25 \sqrt{3}\)
∴ Height of the tower = \(25 \sqrt{3}\)m
9. The angle of elevation of the top of a tower from a point on the ground is 30: After walking nom towards the tower, the angle of elevation becomes 60° Find the height of the tower.
Solution:
Let AB be a tower of height ‘h’ meters. From points D and c on the ground, the angle of elevation of top A of the tower is 30° and 60° respectively.
Given that CD = 40m
let BC = x meters
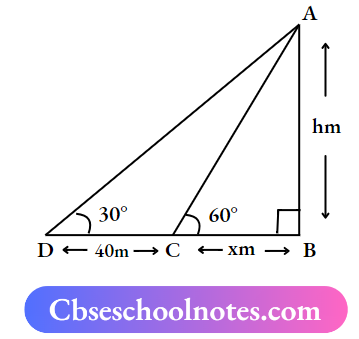
In ΔABC
tan 60° = \(\frac{A B}{B C} \Rightarrow \sqrt{3}=\frac{h}{x}\)
⇒ \(x=\frac{h}{\sqrt{3}}\)
In ΔABD
tan 30° =\(\frac{A B}{B D} \Rightarrow \frac{1}{\sqrt{3}}=\frac{h}{40+x}\)
⇒ \(\sqrt{3} h=40+x\)
⇒ \(\sqrt{3} h=40+\frac{h}{\sqrt{3}}\) [from (1)]
⇒ \(3 h=40 \sqrt{3}+h\)
⇒ \(2 h=40 \sqrt{3}\)
⇒ \(h=20 \sqrt{3}\)
∴ Height of the tower = \(20 \sqrt{3} \mathrm{~m}\) m